Forces in Physics

FORCES IN PHYSICS

Weight or “the force of gravity” is pervasive and gets acted on at all times. If not counteracted, things will fall because of their weight. Objects placed on a surface such as a table will have a restoring force exerted upon them (similar to that of a spring) and, unless it is a heavy object or a flimsy table, you will not notice the deforming nature of the table. The greater the deformation, the greater is the restoring force. Because of the restoring force, the net external force on the load will be zero. The normal force means the force perpendicular to a surface. It is an upward force that opposes the weight of the load but is not necessarily perpendicular to the ground as it can be angulated with respect to the ground (that is, not parallel to the ground). Like other forces, normal force is a vector.

As friction opposes motion between surfaces, the acceleration is smaller when there is friction present. If friction is negligible down an incline, the acceleration down an incline will be the force of gravity multiplied by the sine of the angle of the surface, regardless of the mass of the object. This goes with the idea that all objects will fall at the same rate, regardless of mass, if other things like air resistance are not a factor.

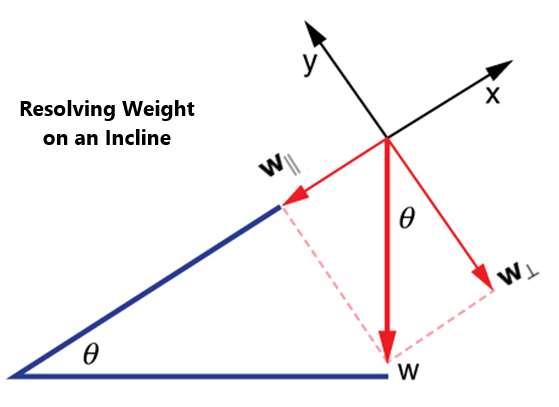
There are steps you already have an idea how to do that involves the way to resolve weights into their respective components. The first thing you must do is to set the x and y axes so that they align with the slant of the incline upon which the weight rests. You should also recognize that some of the weight will be parallel to the slant and some of the weight will be perpendicular to the slant. Figure 15 describes this differentiation of the weights:

Figure 15.

The weight parallel to the incline is the weight multiplied by the sine of theta, while the weight perpendicular to the incline is the weight multiplied by the cosine of theta. Remember that weight is equal to mass times the force of gravity g, which can be substituted into the equations just mentioned.

Understand that, in this case, parallel weight is weight along the x axis and perpendicular weight is weight along the y axis. This means that the usage of sine and cosine is the reverse of what it has been before because theta is now the angle between the y axis and the downward vector, which is ninety degrees minus the theta we’ve talked about in the past. You just need to know this in order to do these equations.

The perpendicular weight is equal in magnitude and the opposite in direction to the normal force. The parallel force would be the force that pushes the object down the incline. If there was no friction, the object would slide down the incline if the force is even at a minimum. The force of friction, however, opposes the motion of the object. In such cases, friction is a force that has a magnitude and direction that goes up the incline.