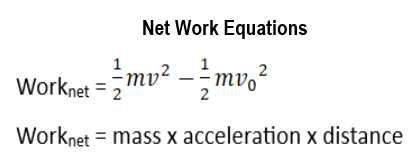
3 minute read
Gravity and Potential Energy
by AudioLearn
applied due to friction. Because force equals mass times acceleration, the net work will equal the mass times the acceleration times the distance. Another substitution that can be made involves velocity. The net work on a system can be described as half the mass times the square of the final velocity minus half the mass times the square of the initial velocity. This is explained visually in figure 32:
Figure 32.
Advertisement
The expression of work as it relates to mass and volume is called the work-energy theorem, which applies even if the forces vary in direction and magnitude. The quantity one-half mass times the velocity squared is referred to as the kinetic energy or KE of a mass moving at a certain speed. In actuality, this is referred to specifically as the “translational kinetic energy” because it is linear in nature and is different from the rotational kinetic energy, which will be covered later.
You should know that energy is going to be in the form of Joules, which will be kilogram-meters squared per second squared. Another way to look at it is that Kinetic Energy is force times distance so, in the case where you are given the net force and the distance, you need only multiply the two as one joule is one Newton-meter.
GRAVITY AND POTENTIAL ENERGY
All work done in climbing a mountain or lifting an object against gravity does work against the force of gravity. When there is work done, energy is transformed. The work required to take an object of a mass m through a height h at constant speed will be equal to its weight or mass times the force of gravity times the height. This is called the gravitational potential energy. The energy is stored in the gravitational field of the
earth. Potential energy is a property of a system rather than of a single object and is basically due to its physical position. For most systems in which gravitational potential energy is used, the arbitrary setpoint for zero potential energy is the Earth’s surface. This is an arbitrary setpoint and what’s important is the difference in gravitational potential energy.
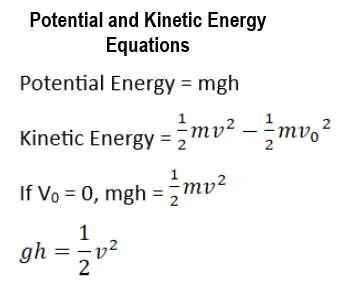
Because all energy is energy, there must be a way to convert gravitational potential energy and kinetic energy. The best way to do this is to consider the difference in potential energy and kinetic energy without considering the step involving the work involved. The change in potential energy of a weight can be described as the mass times the force of gravity times the change in height. The change in height can be positive or negative. As something falls, it gives up its gravitational potential energy in the fall. This potential energy is converted to kinetic energy.
It is important to note that this change in gravitational potential energy is independent of the path the object takes to get from point A to point B. It just depends on the height as it applies to a lower height on the y-axis, which might be the height above the level of the earth. The change in potential energy is equal to the kinetic energy so that the speed can be determined after a certain distance is covered. What you know about kinetic energy is that it equals one-half mass times velocity squared and that will equal mass times height times the force of gravity. Note that you don’t have to know the mass of the object because it cancels itself out in this equation. Figure 33 describes these equations: