The Four-Stroke Engine

THE FOUR-STROKE ENGINE

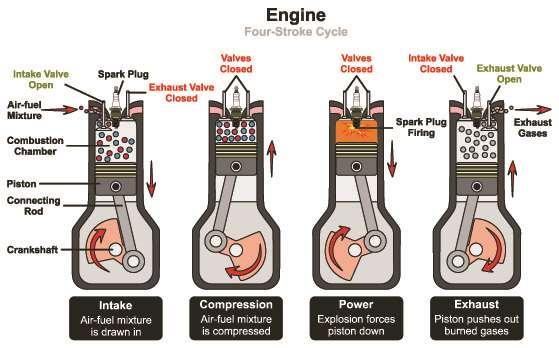
This represents a simple piston engine system, which involves the use of an ignitable gas that does work on the system and drives the engine. Figure 87 is a four-stroke internal combustion engine described visually:

Figure 87.

There are four cycles: intake-exhaust, compression, ignition, and power. The compression stroke involves work being done on the system, increasing the pressure and temperature. There is heat transfer in the next part, causing a pressure increase and temperature increase. This happens so quickly in a combustion engine that volume is nearly (but not completely) constant. The final part, power is is (nearly) adiabatic process so that work is done to a greater degree because the pressure is greater. Exhaust leaves the system and the volume is returned to its initial state.

During the first part, air is mixed with fuel during intake. In the second part, compression of the air-fuel mixture occurs in a process that is nearly adiabatic. Work is done on the compressed gas. In the power stroke, there is ignition of the air-fuel mixture, creating thermal energy that increases the pressure, pushing on the piston.

This also does work, and is nearly adiabatic. In the exhaust stroke, hot gas is expelled in order to prepare the engine for another cycle.

What you should note is that it is necessary for heat to transfer out of the system in order for there to be a net amount of work done. The pressure volume work done in this cycle, called the Otto cycle, is described in figure 88, which is defined as the work done in the cycle. If no heat transfer is done, then no work is done. The lower the temperature on the path A to B, the less work needs to be done to compress the gas. In addition, the higher the temperature along the path from C to D, the greater the work done.

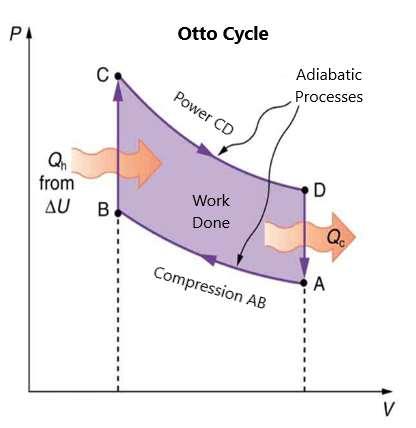
Figure 88.

In figure 88, paths A to B and C to D are adiabatic and are the compression strokes and power strokes, respectively, of the internal combustion engine. Paths BC and DA are isochoric and are the ignition and intake-exhaust aspects of a four-stroke engine (note that intake and exhaust happen at the same time). Work is done on the AB path but more work is done on the CD path, which allows a net amount of work output done. This increase in work occurs along the CD path because the temperature is increased along

this path versus the AB path. The isochoric processes are done so quickly that the volume of the piston does not have a chance to catch up.

In summary, these are the parts of the four-stroke engine:

• Exhaust-Intake D to A: The decrease in pressure when the gas-air mixture is added and exhaust takes place (isochoric).

• Compression A to B: The increase in pressure when the piston is pressed on (adiabatic).

• Ignition B to C: The ignition of the spark plugs and further increase in pressure (isochoric).

• Power C to D: The decrease in pressure and increase in volume (adiabatic).

This example of the Otto cycle and the four-cycle engine reflects what happens in normal fuel-powered engines that turn fuel into heat and finally into power that drives the engine. During the compression phase, the gases are compressed so they can be ignited, furthering the change in pressure that drives the power in the system, which is what does the work.

Heat engines cannot be 100 percent efficient. Why is this the case? Because, heat must be transferred to the environment. This heat transfer is referred to as waste heat. The Carnot engine and the Carnot cycle reflect the most efficient cyclical cycle process possible. This would technically be a never-ending engine that requires just a small amount of input to get the engine going. What defines the Carnot cycle versus the Otto cycle is that only reversible processes are at work.

This leads to a third aspect of the second law of thermodynamics. This is that a Carnot engine between two temperatures will have the greatest possible efficiency of any type of heat engine operating between the two different temperatures. All engines using just reversible processes will have the same maximum efficiency when operating between the same given temperatures. Figure 89 shows the Pressure-Volume diagram for a Carnot cycle engine. There are two isothermal and two adiabatic processes, both of which are reversible in theory:

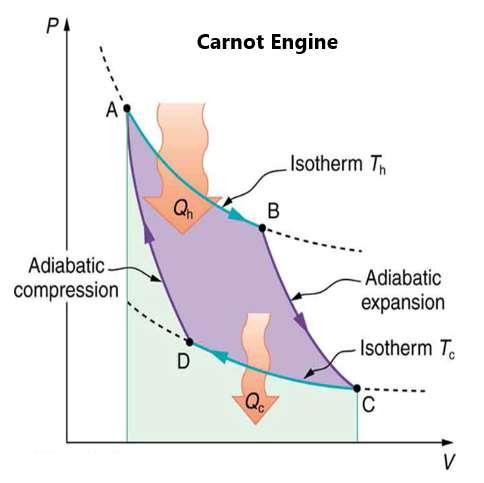
Figure 89.

Remember that the efficiency of a perfect heat engine is one minus the ratio of the heat output and the heat input. For a perfect heat engine, the ratio is equal to the ratio of the absolute temperatures of the heat reservoirs. It leads to the definition of the Carnot efficiency or maximum efficiency is related to the actual temperature differences between both aspects of the system. In reality, the actual efficiency is about 0.7 or 70 percent of the Carnot maximum. The greatest efficiency would happen when the temperature ratios are as small as possible.

The problem with the Carnot engine is that it has no ability to do any power. It would only be possible if the cold reservoir were at absolute zero, which is impossible. Because all of the engines operate at levels greater than absolute zero, the ability to be efficient is limited. The maximum efficiency could happen when one reservoir is at the highest possible temperature and the other reservoir were at the lowest possible temperature. This would increase the area under the Pressure-Volume curve.