Computational Odyssey 1.0

Balakumaran Ramalingam

Computational Odyssey 1.0

The ideology and practice of designing have changed through time and evolved into an array of iterations developed through parameters, saving time and energy allowing to experiment and experience more. This development thrilled me when I first used grasshopper which controls primitives merely with a slider. This changed my perception of design. Fromthen on, I investigated more on designing with parameters rather than translating human thoughts to computer. I would like to thank Meal*, Mula and Designmorphine for being a mentor, inspiration and pioneer in the field of computational thinking and designs. This portfolio is an initial compilation of my experimentation through computational and procedural softwares and tools, which I will publish as further series in near future.

FIBROIDPROBE

Materialising mathematics through grasshopper 01 02 03 04 05 06

Exploration of Shortwalk and Voronoi

ALIENLANDSCAPES

Experimentation with heightfields through grasshopper

CELLULAR AUTOMATA

Exploration of game of life through anemone

STOICHIOMETRICAGGREGATION

Exploration of aggregation of elements through wasp

HARDSURFACE MODELLING

Procedural modelling in Houdini

SINGULAR SURFACE

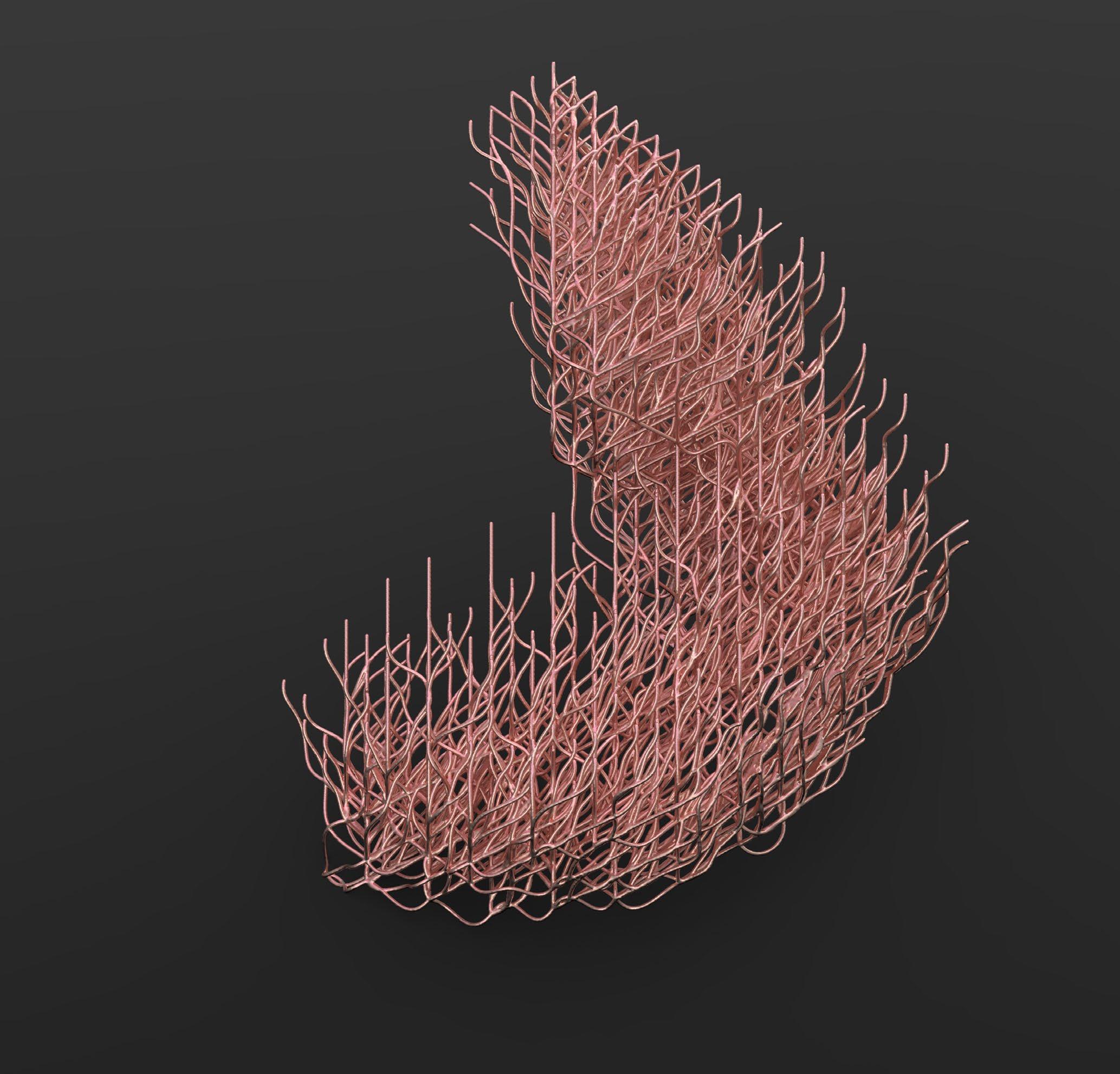
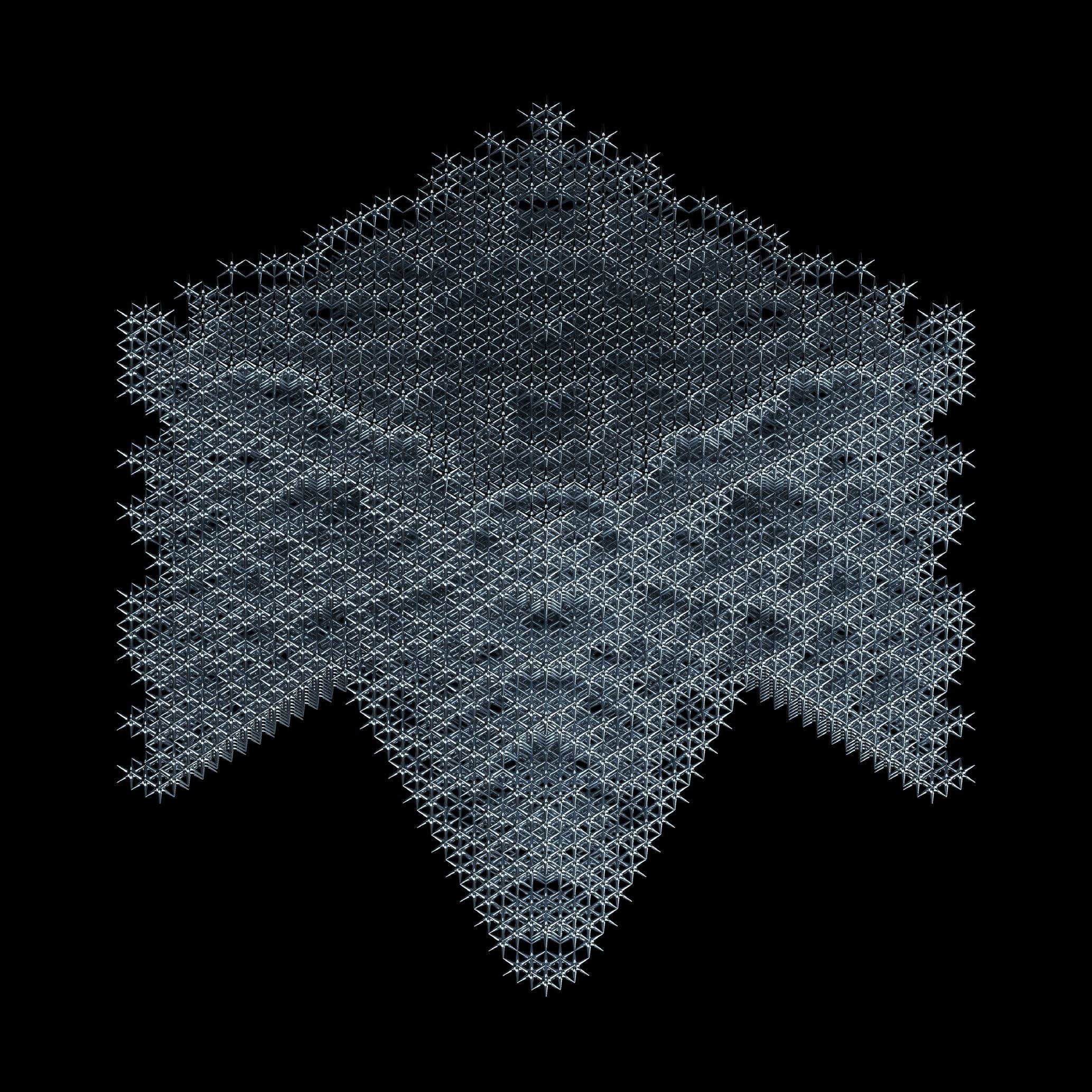
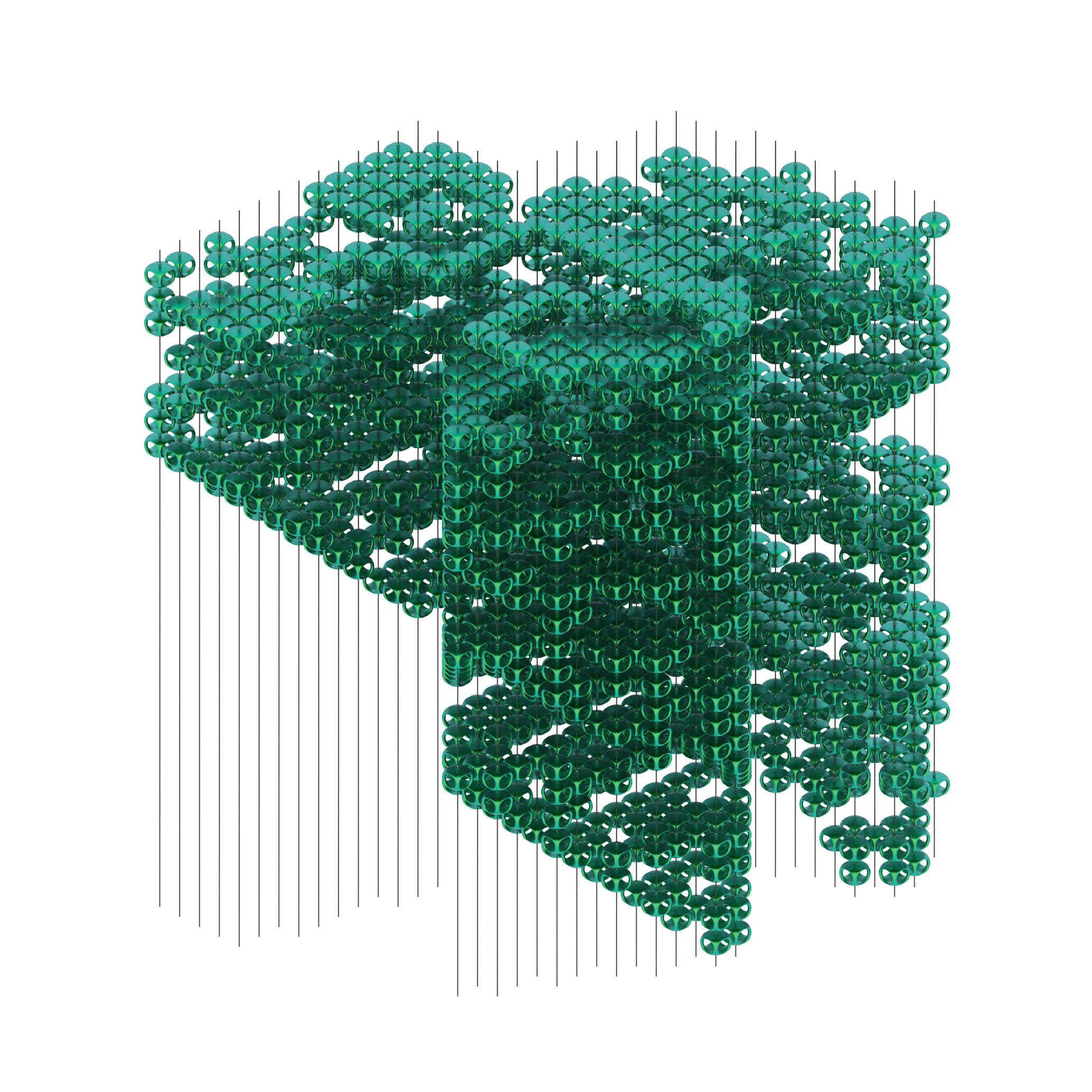
This chapter is an anthology of experiments that involve superabundance interpolation of voronoi and shortwalk through grasshopper. These outputs are results of workshop conducted by Filippo Nassetti through Meal* Live academy. The curvilinear primitives obtained through shortwalk path in voronoi 3d frames are converted to solids to give an interesting results of fibrous structures.

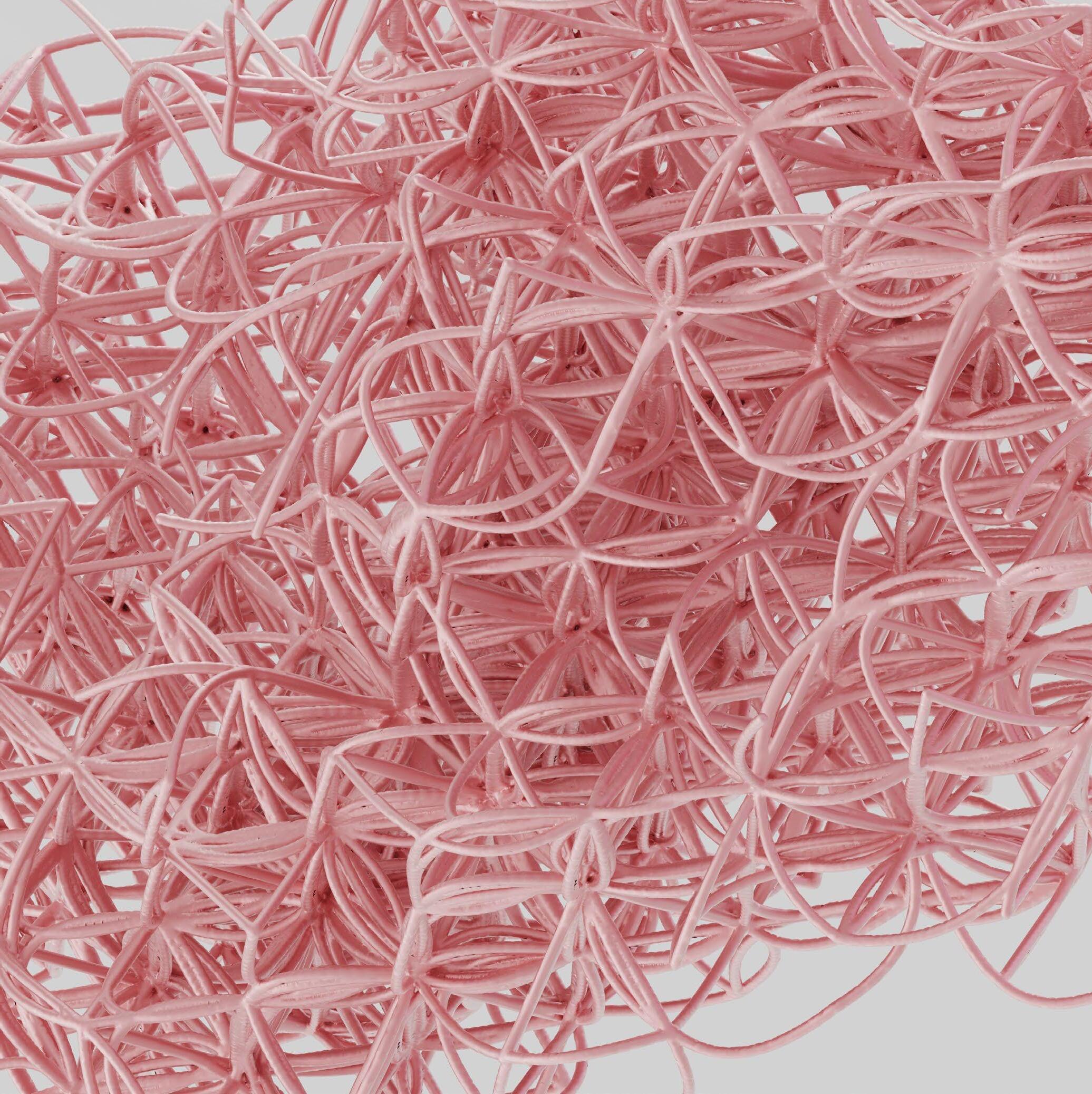
01

Fibroid Probe

Initial Hexagrid frame

Desired path

Shortwalk path through initial frame

Rebuilding Shortwalk Path

Initial Voronoi cell frame

Desired path

Shortwalk path through initial frame

Rebuilding Shortwalk Path

Dense city and one node

Shortwalk path with attractors

City and one node

City and two node

Initial State

Initial State

Initial State

Voronoi cell : 1000

Curve Division : 6

Voronoi cell : 2000

Curve Division : 8

Voronoi cell :2000

Curve Division : 8

Voronoi cell : 2000

Curve Division : 6

Voronoi cell : 1000

Curve Division : 8

Voronoi cell : 1000

Curve Division : 6

Voronoi cell : 1000

Curve Division : 8

Voronoi cell :1000

Curve Division :6

Voronoi cell : 1000

Curve Division : 8

Initial State

Voronoi cell :1000

Curve Division : 8

Voronoi cell : 2000

Curve Division : 6

Voronoi cell : 2000

Curve Division : 8

Voronoi cell : 2000

Curve Division : 8

Voronoi cell : 3000

Curve Division : 8

Voronoi cell : 3000

Curve Division : 8

Voronoi cell :3000

Curve Division : 8

This exercise is based on research by MHOX: (curated by Filipino Nasetti). This research studies the path of a spider along a hexagonal diagrid frame, frominitial stage to destiny, interpreting the fibres that it spuns through the path. The simulation produced amazing superabundance tissue structures that could emote a stereotypical geometries and objects. I experimented with a frame work , but with dfferent initial and destiny points, through randompaths. Some of the experiment outputs are here.

Image by MHOX:

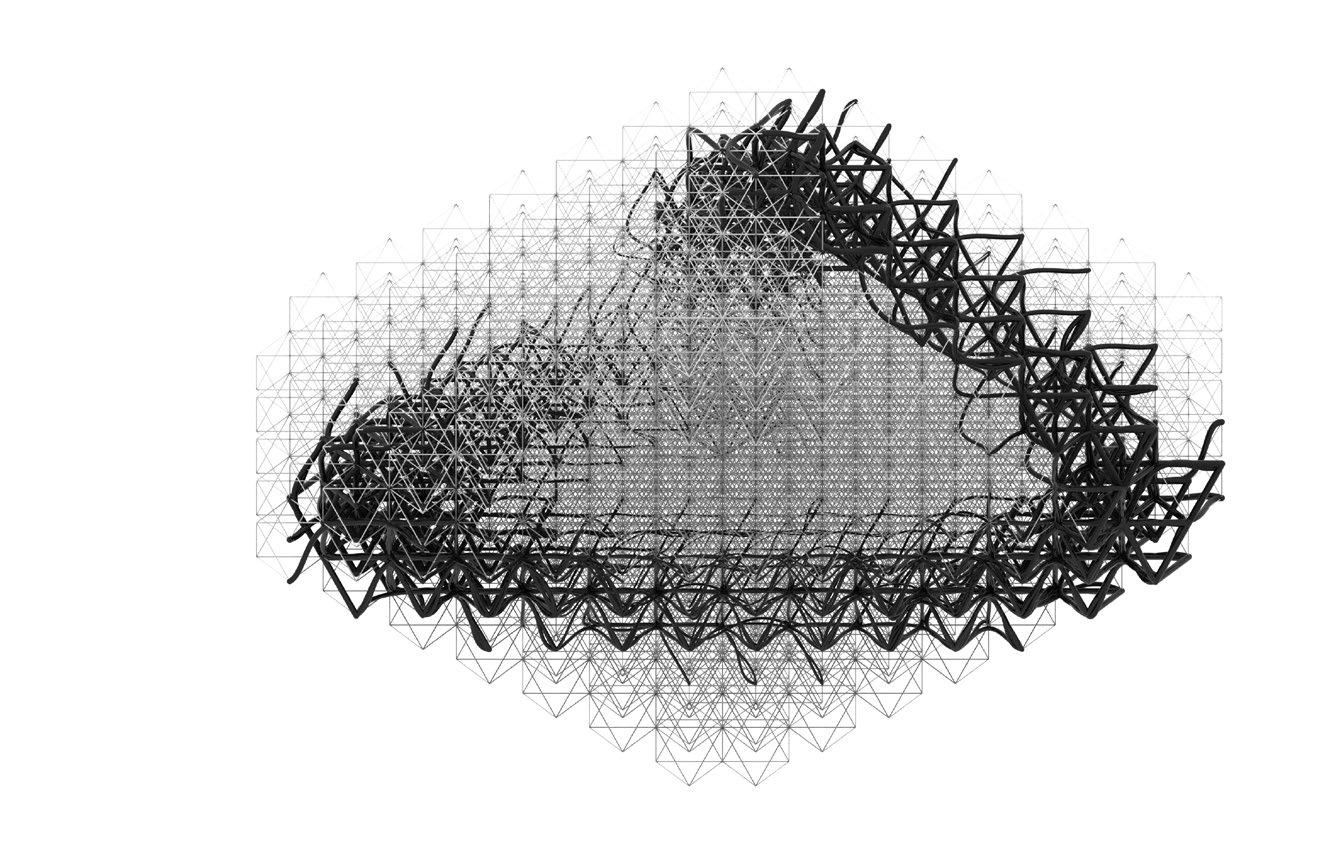
This series is an extended version of previous experiment. Here I fed five predefined curves and experimented with the outputs based on combination of voronoi and hexagrid frames. I have experimented with grid frame sizes to play with fibre like networks. These experimentation gave an inference that the solid forms would be a material exploitation, rather than finding stabular organic forms that serves the same purpose.

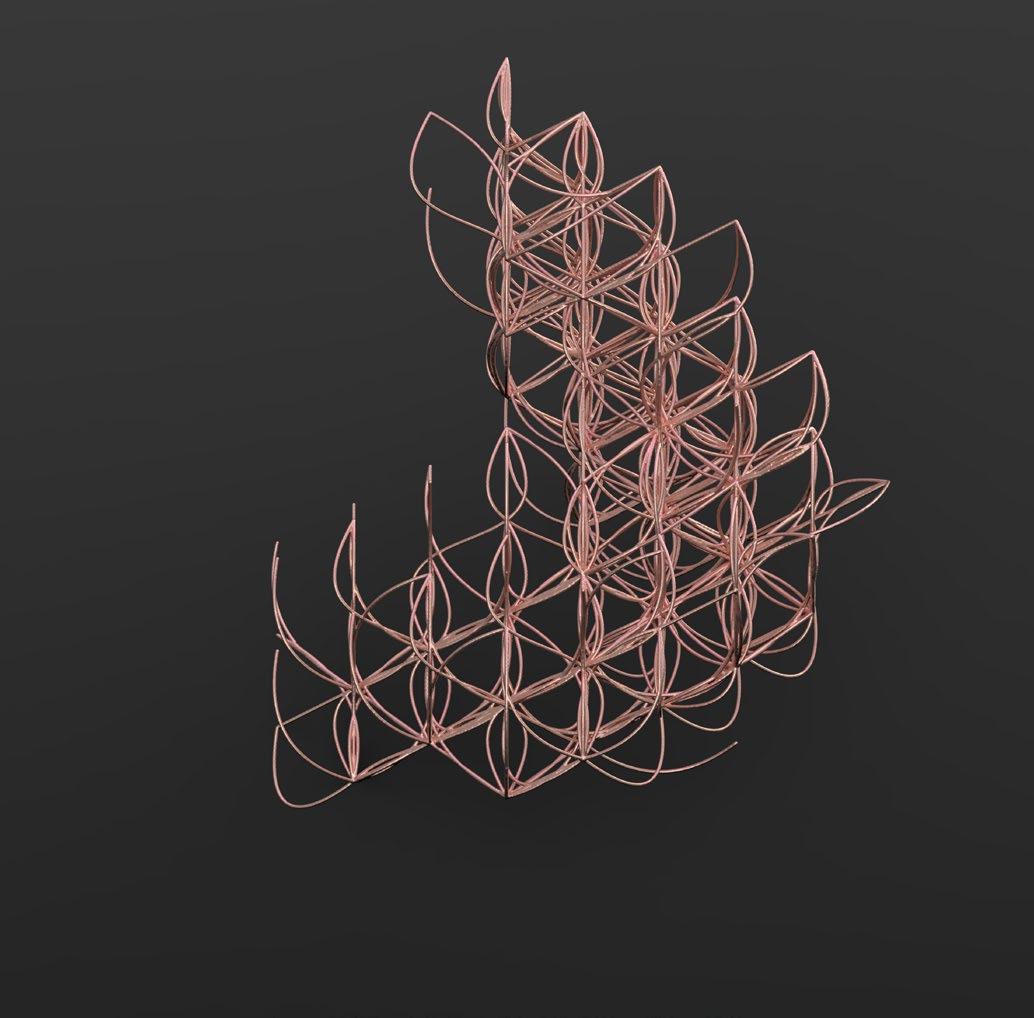
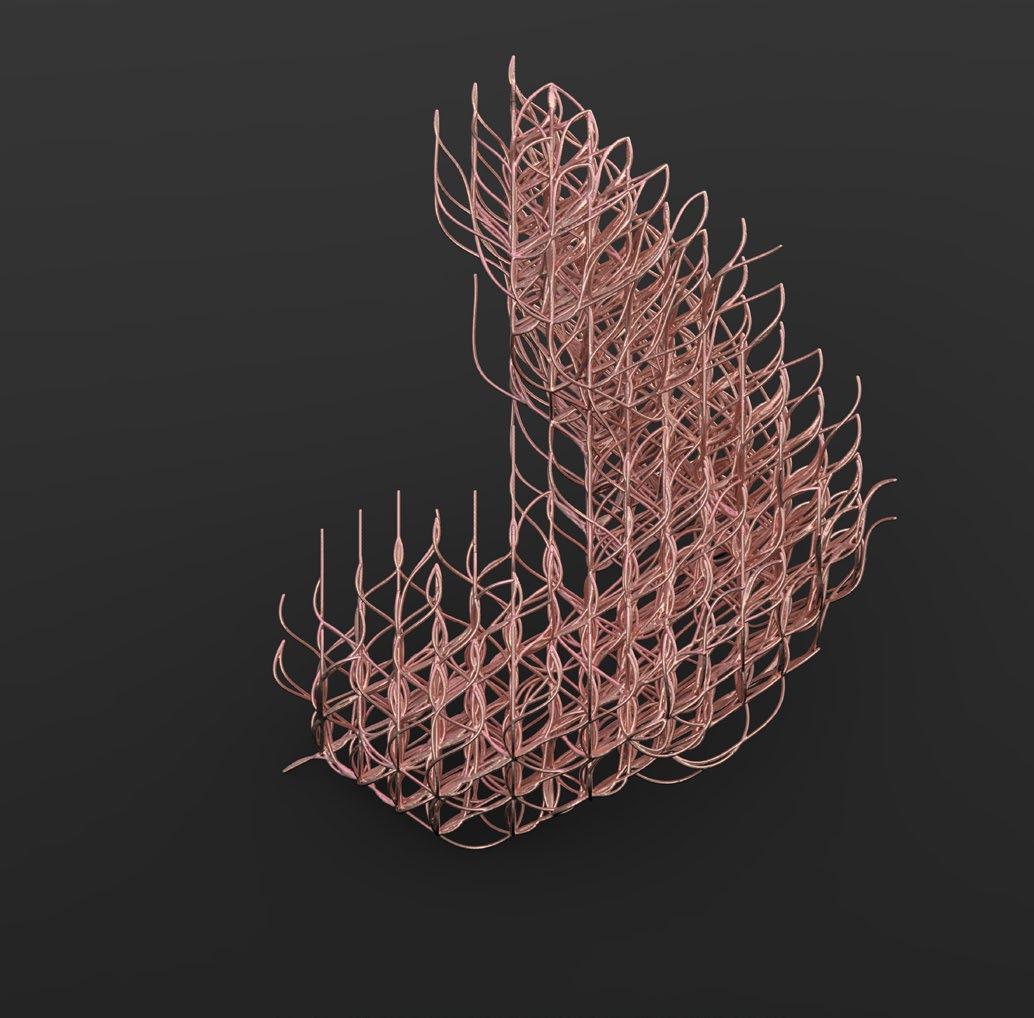
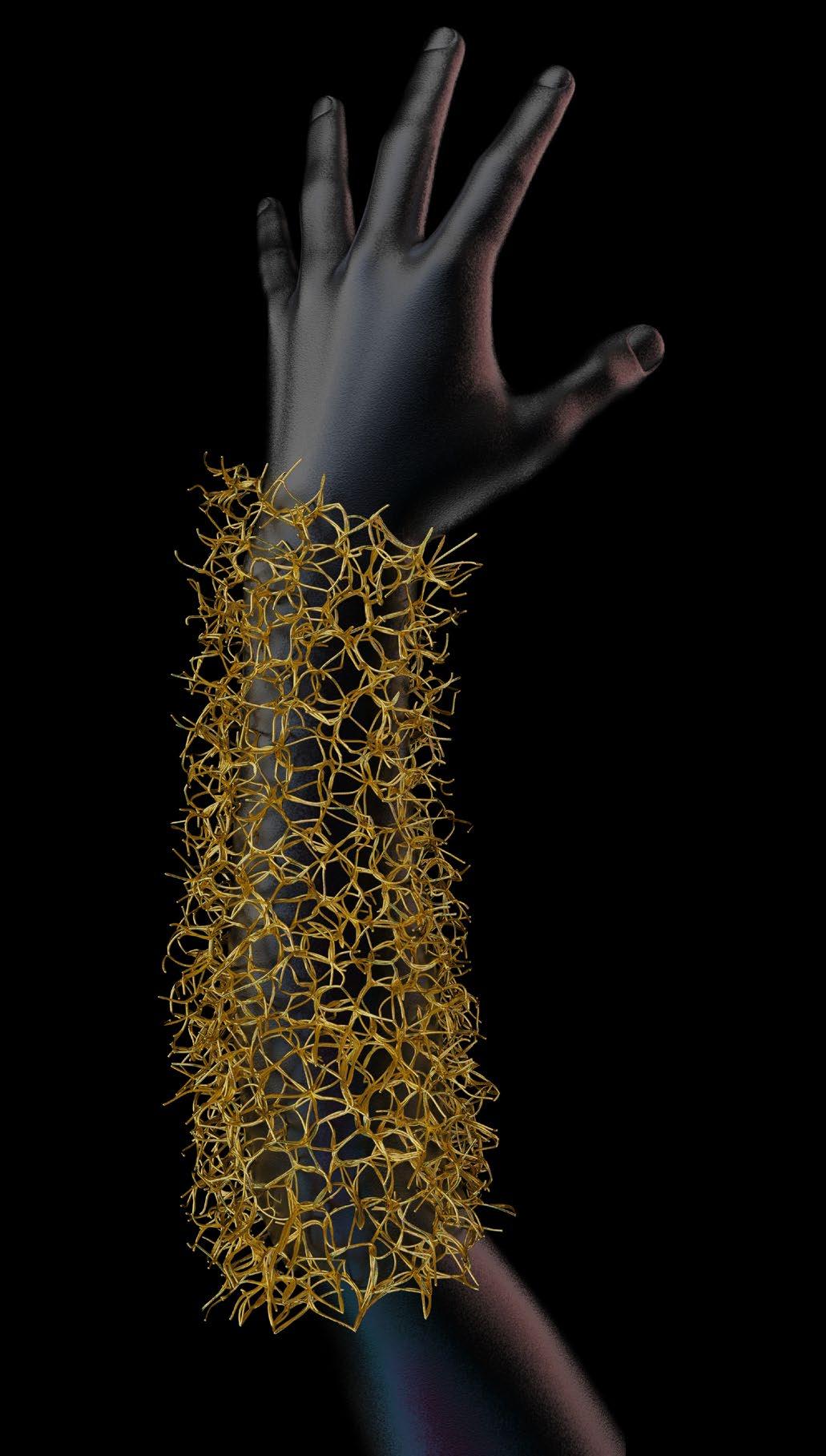
This experiment is a real life ideation. I ideated to use superabundance tissues to design fabrics, apparels and fashion elements that could be 3d printed instead of heavy craftmenship and abundance material usage.

02 Alien Landscapes

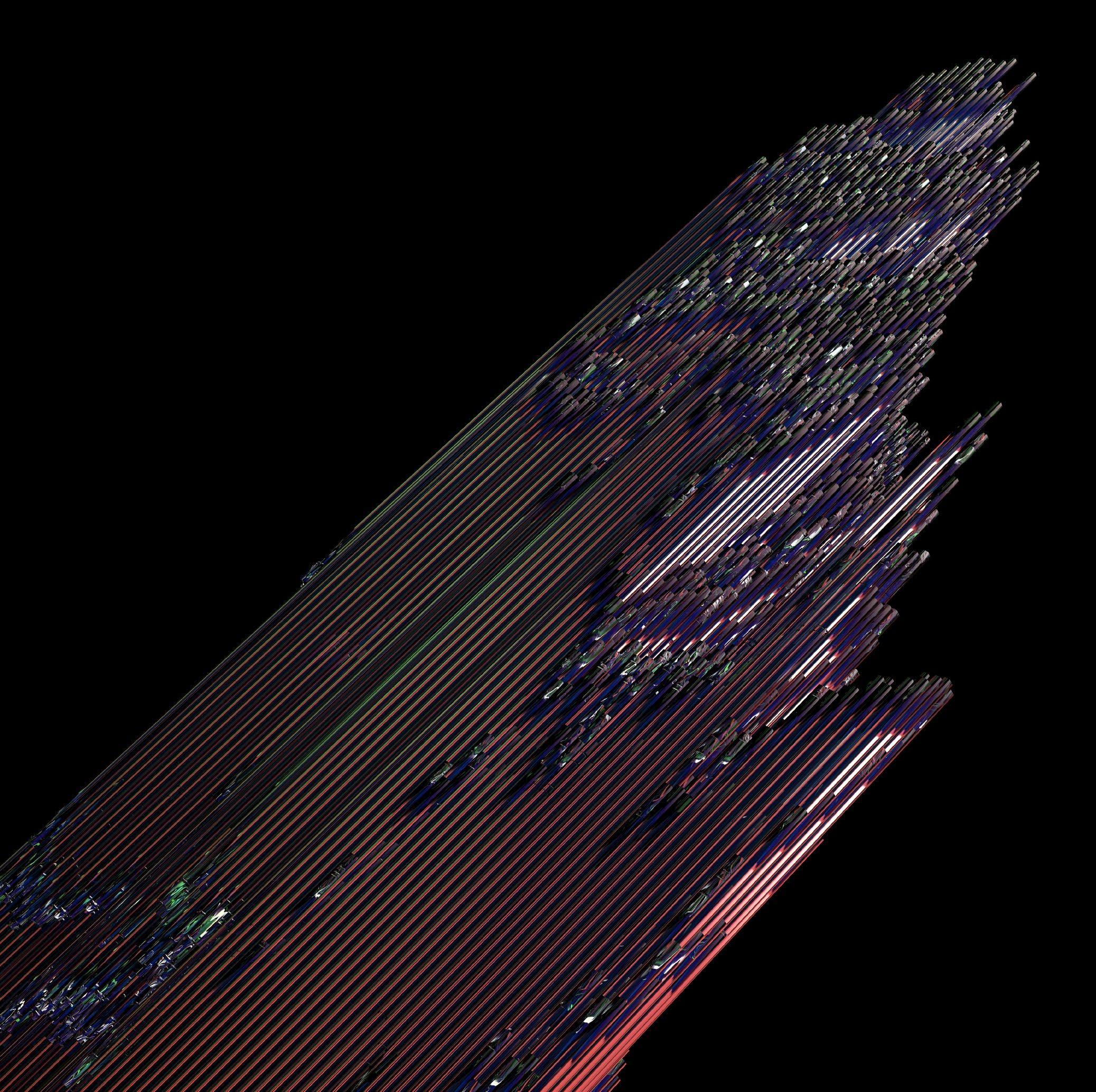
Working with noises and maps are always intriguing in terms of relatively known outputs. In addition, the ability to control the bounds always make thema playful tool. This chapter is a collection of such geometry play, with twisted and curled geometry fromthe maps and noises. The outputs are gripping and appealing with an artistic dialect. This series is the output of ‘Eroded Morphologies’ conducted by Filippo Nassetti through MEAL* academy.

03 Cellular Automata

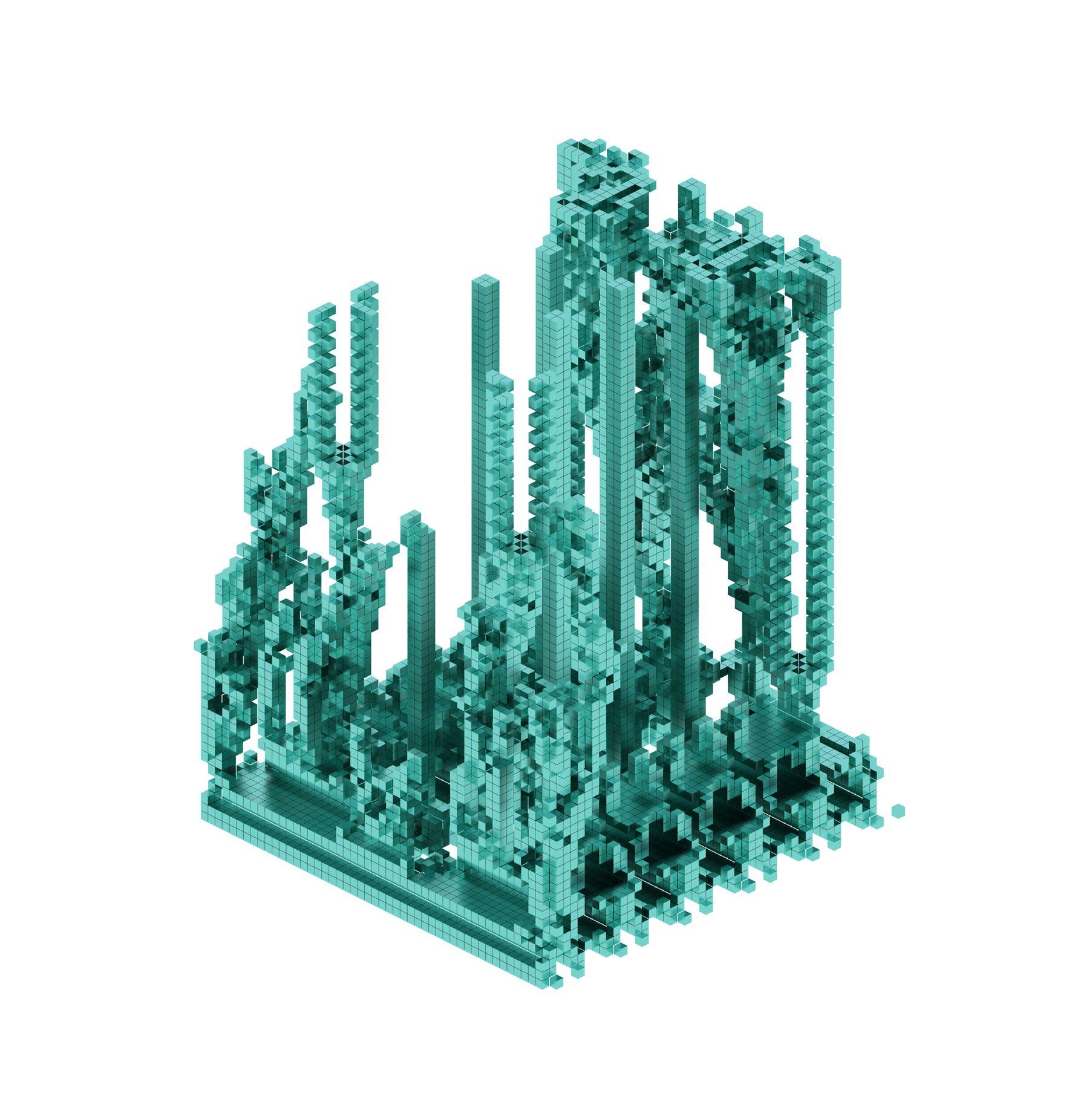
Acellular automato is an interpretation of cells’ behaviour on a grid of specified shape that evolves through a number of discrete time steps according to a set of rules based on the initial states of neighboring cells. The rules are then applied iteratively to study the feasibility and morphology of cells. The outputs here are the results after the workshop by Stepan kukharskiy.

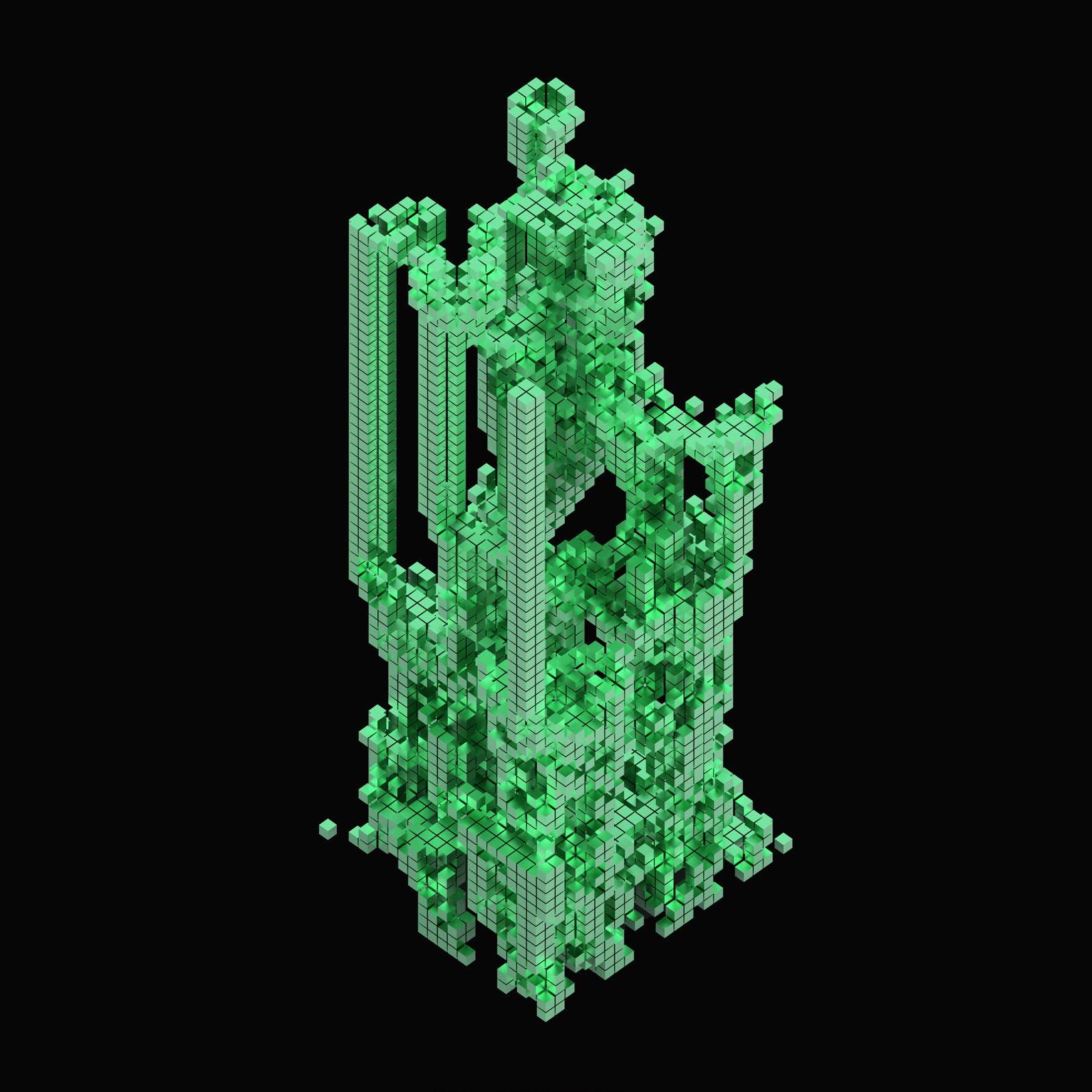
The universe of the Game of Life is an infinite, twodimensional orthogonal grid of square cells, each of which is in one of two possible states, active or dead. Conways game of life follows simple rules: Any live cell with 2/3 live neighbours survives \\ Any dead cell with 3 live neighbours becomes a live cell \\ All other live cells remain live and dead cell stay dead

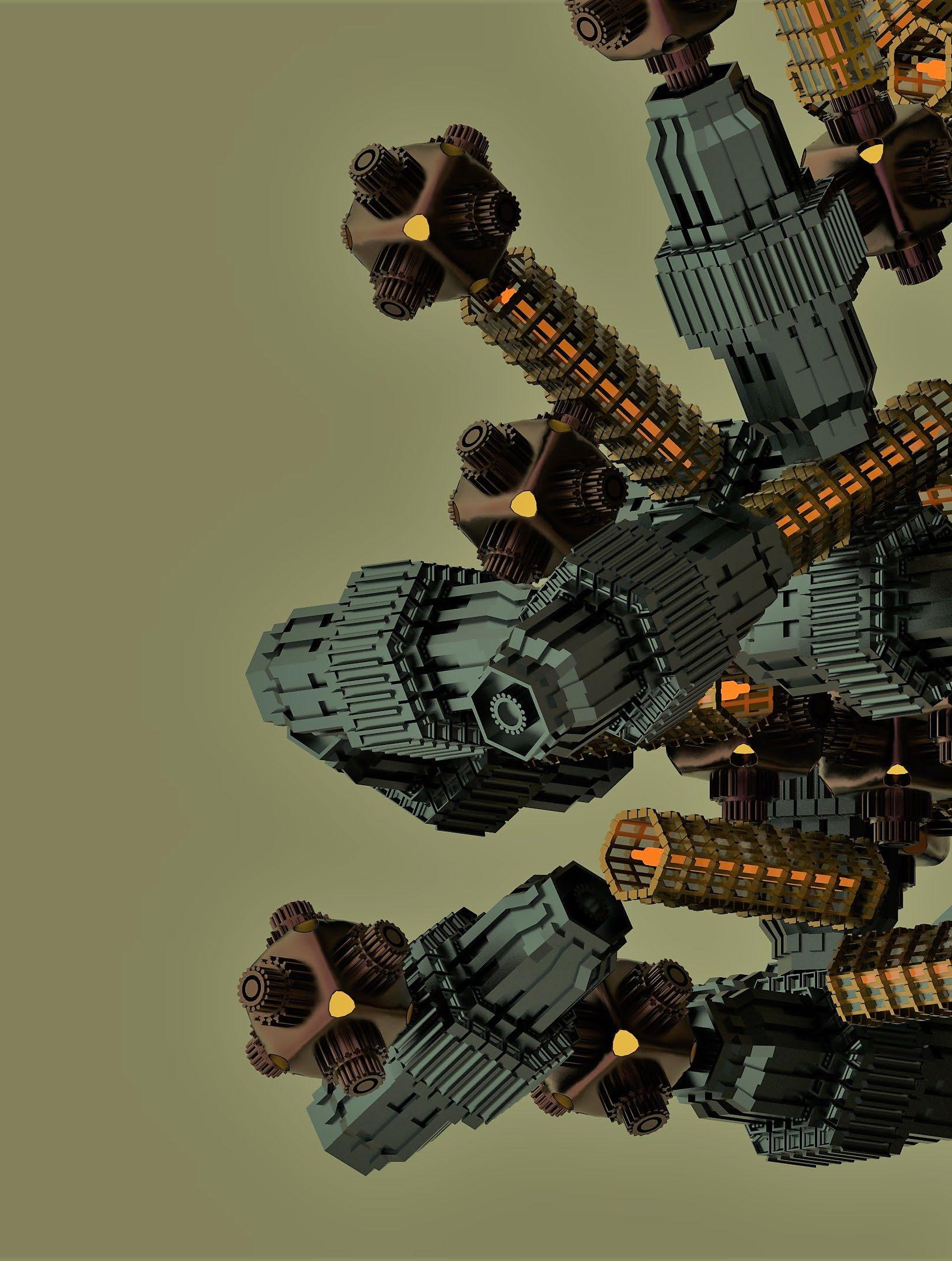
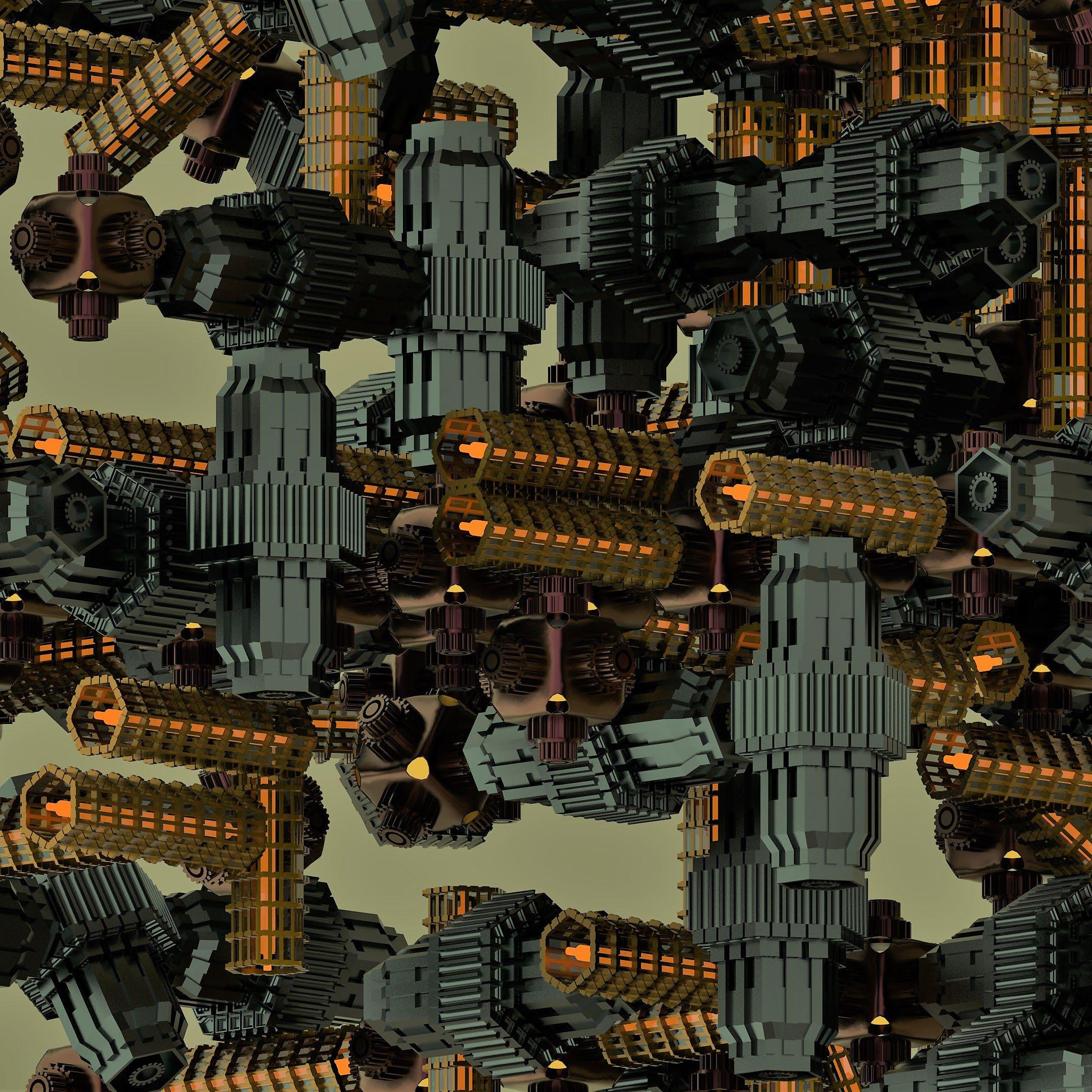
04 Stoichiometric Aggregation

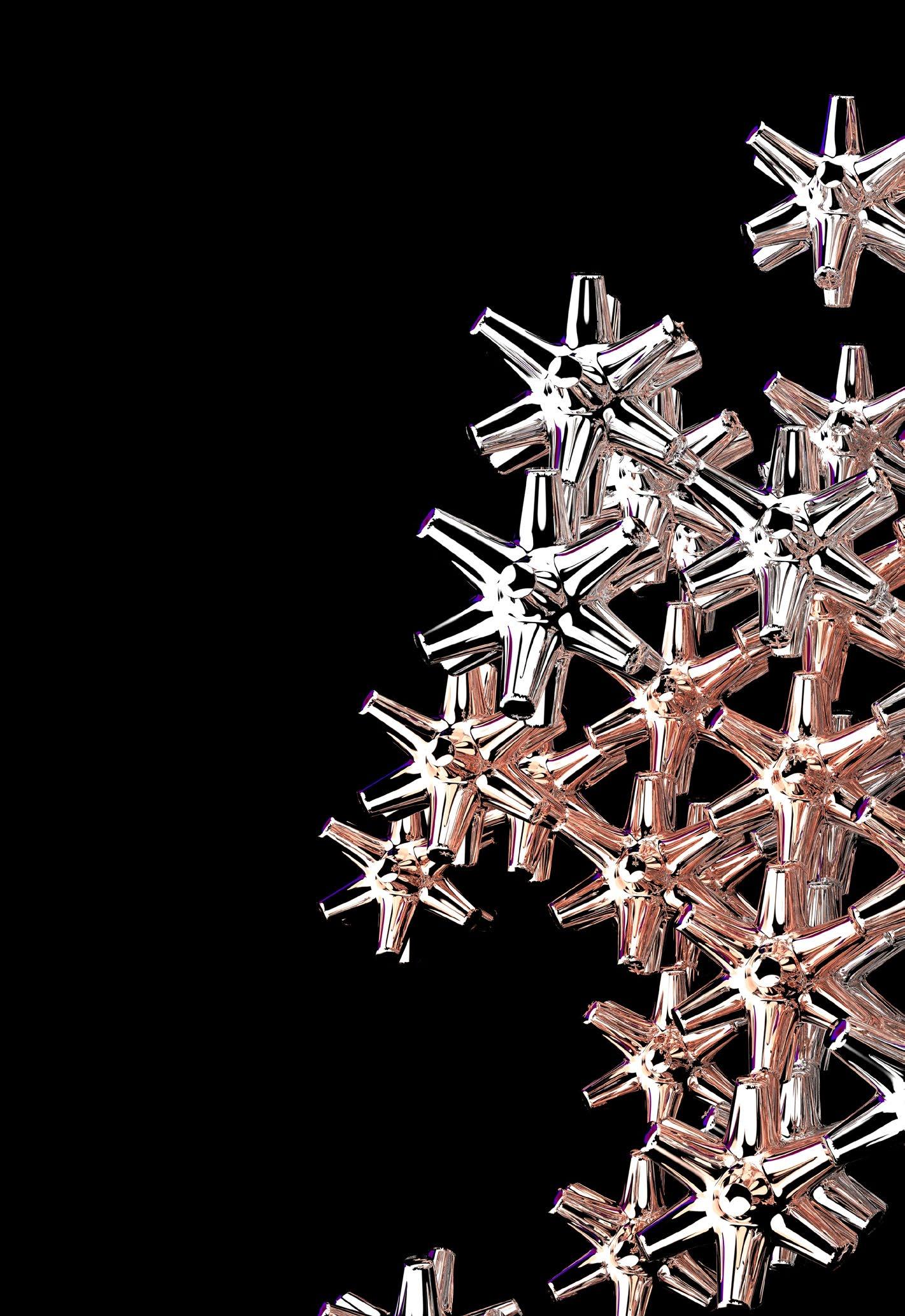
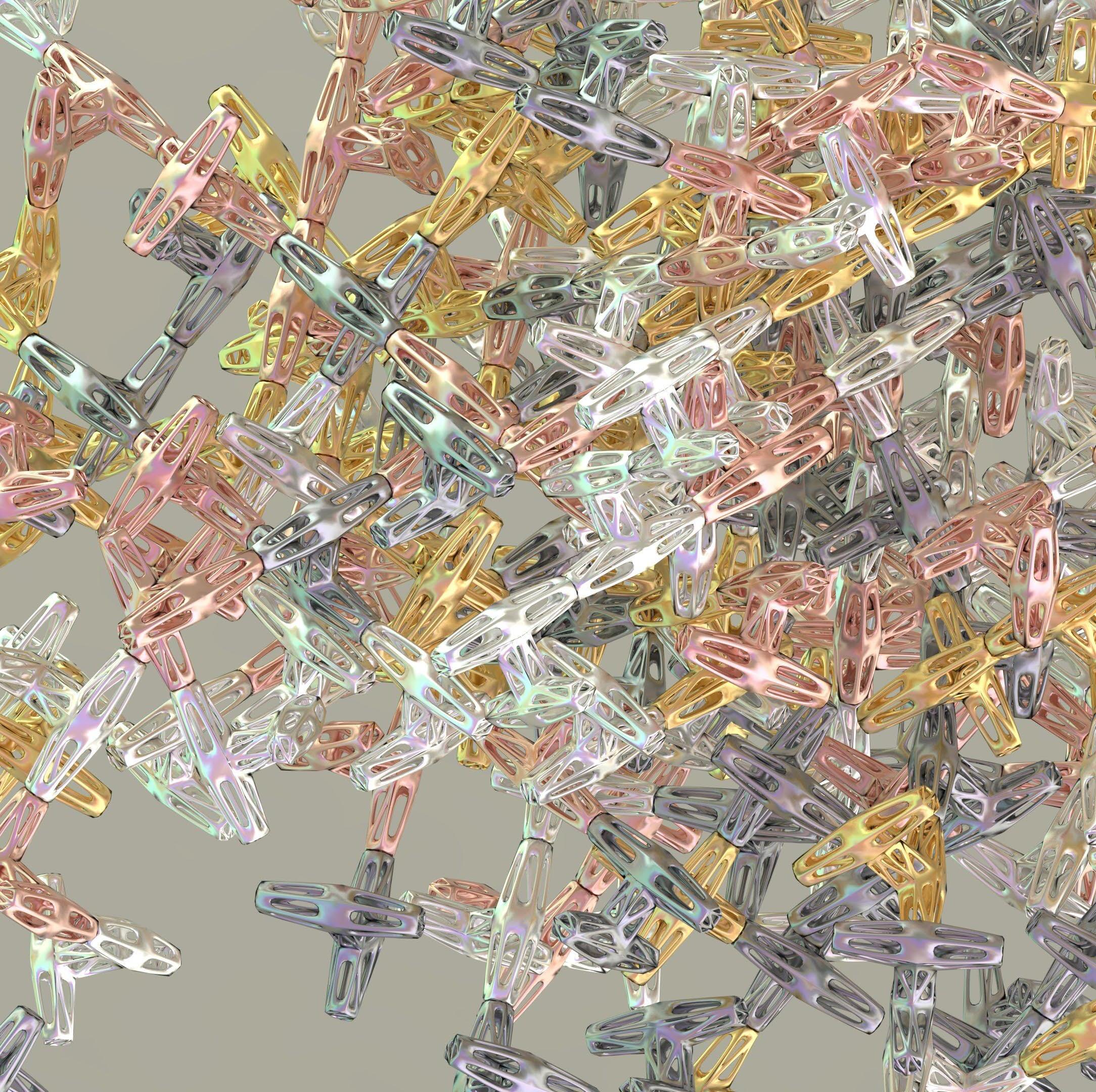
Compounds are formed through rational, calculated and definite reaction between initial reactants. The molecules do merge with elements adjoining in a specific structure and rules. ‘Wasp’ is a grasshopper plugin which allows geometry to be aggregated with user defined axial joints and conjuction elements. I have iterated modules to forman artistic story between elements that juxtapose simple geometries with complex aggregating mechanism.

05 Hardsurface Modelling

Through the journey, I find Houdini to be a more efficient tool in present day design development. Being a node based tool, combined with flexibility through VEX language, Houdini opened ‘n’ number of possiblities for parametric and programme based designs. As a beginner, I explored more about procedural polygonal modelling through Houdini, where some results of workshops by Rohan Dalvi is here.

06 Singular surfaces

The idea of modulating and texturing a surface through manipulation of the cartesian equation was an interesting experiment to play with. Through several attemps and play, transforming a regular interesting curve to shapes through equations produced interesting results, which some of them are here.