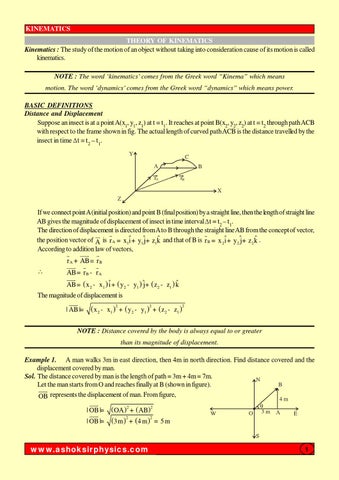
KINEMATICS THEORY OF KINEMATICS Kinematics : The study of the motion of an object without taking into consideration cause of its motion is called kinematics. NOTE : The word ‘kinematics’ comes from the Greek word “Kinema” which means motion. The word ‘dynamics’ comes from the Greek word “dynamics” which means power. BASIC DEFINITIONS Distance and Displacement Suppose an insect is at a point A(x1, y1, z1) at t = t1. It reaches at point B(x2, y2, z2) at t = t2 through path ACB with respect to the frame shown in fig. The actual length of curved path ACB is the distance travelled by the insect in time t = t2 – t1. Y
C A rA
B rB X
Z
If we connect point A (initial position) and point B (final position) by a straight line, then the length of straight line AB gives the magnitude of displacement of insect in time interval t = t2 – t1. The direction of displacement is directed from A to B through the straight line AB from the concept of vector, the position vector of A is r A = x1ˆi + y1ˆj+ z1kˆ and that of B is r B = x 2ˆi + y 2ˆj+ z 2 kˆ . According to addition law of vectors, r A + AB = r B AB = r B - r A AB = ( x 2 - x1 ) ˆi + ( y 2 - y1 ) ˆj+ ( z 2 - z1 ) kˆ The magnitude of displacement is 2 2 2 | AB |= ( x 2 - x1 ) + ( y 2 - y1 ) + ( z 2 - z1 ) NOTE : Distance covered by the body is always equal to or greater than its magnitude of displacement. Example 1. A man walks 3m in east direction, then 4m in north direction. Find distance covered and the displacement covered by man. Sol. The distance covered by man is the length of path = 3m + 4m = 7m. N B Let the man starts from O and reaches finally at B (shown in figure). OB represents the displacement of man. From figure, 4m 2 2 | OB |= ( OA) + ( AB) O 3m A W E 2 2 | OB |= (3m ) + (4 m ) = 5 m S
www.ashoksirphysics.com
1
KINEMATICS 4m 4 = 3m 3
and
tan q =
æ4 ö q = tan- 1ç ÷ è 3ø
æ ö - 1 4 The displacement is directed at an angle tan çè ÷ north of east. 3ø Average Speed and Average Velocity Suppose we wish to calculate the average speed and average velocity of the insect (in section (i)) between t = t1 and t = t2. From the path (shown in fig.) we see that at t = t1, the position of the insect is A(x1, y1, z1) and at t = t2, the position of the insect is B(x2, y2, z2). Y
C A rA
B rB X
Z
The average speed is defined as total distance travelled by a body in a particular time interval divided by the time interval. Thus, the average speed of the insect is The length of curve ACB v av = t 2 - t1 The average velocity is defined as total displacement of the body in a particular time interval divided by the time interval. Thus, the average velocity of the insect in the time interval t2 – t1 is AB v av = t - t 2 1 rB - rA v av = t 2 - t1
( x - x1 ) ˆi + ( y2 - y1 ) ˆj+ ( z 2 - z1 ) kˆ v av = 2 t 2 - t1 Example 2. In one second a particle goes from point A to point B moving in a semicircle (fig.). Find the magnitude of average velocity. A AB | vav |= Sol. Dt 1.0m 2.0 | vav |= m/s 1.0 B | vav |= 2 m / s Example 3. A particle goes from A to B with a speed of 40 km/h and B to C with a speed of 60 km/h. If AB = 6BC the average speed in km/h between A and C is _______ Sol. AB = 40t1 ...(1) BC = 60t2 ...(2)
www.ashoksirphysics.com
2
KINEMATICS
total distance travelled time taken AB + BC Vav = t1 + t 2 From eqn. (1) and (2) A 40t1 + 60t 2 Vav = ...(3) t1 + t 2 According to question AB = 6BC 40t1 = 6 × 60t2 From eqn (1) and (2) t1 = 9t2 40 9t 2 60t 2 Vav From eqn (3) from eqn (3) 9t 2 t 2 420t 2 Vav = Vav = 42 km / h 10t 2 Average speed =
B
C
Instantaneous Velocity Instantaneous velocity is defined as the average velocity over smaller and smaller interval of time. Suppose position of a particle at t is r and at t + t is r + Dr . The average velocity of the particle for time Dr interval t is v av = . Dt From our definition of instantaneous velocity, t should be smaller and smaller. Thus, instantaneous velocity is Dr dr v = lim = Dt ® 0 Dt dt Example 4. Let at any time t, the position vector of a particle is r = x iˆ + y ˆj+ z kˆ . Find the velocity of the particle.
Sol. x-component of velocity, v x =
dx dt
y-component of velocity, v y =
dy dt
vz =
dz dt
z-component of velocity,
Thus, velocity of particle v = v x ˆi + v y ˆj+ v 2 kˆ dx ˆi + dy ˆj+ dz kˆ v= dt dt dt
Average and Instantaneous Acceleration In general, when a body is moving, its velocity is not always the same. A body whose velocity is increasing is said to be accelerated. Average acceleration is defined as change in velocity divided by the time interval. Let us consider the motion of a particle. Suppose that the particle has velocity v1 at t = t1 and at a later time
www.ashoksirphysics.com
3
KINEMATICS t = t2 it has velocity v 2 . Thus, the average acceleration during time interval t = t2 – t1 is v 2 - v1 Dv a av = = t 2 - t1 Dt
If the time interval approaches to zero, average acceleration is known as instantaneous acceleration. Mathematically, Dv dv a = lim = Dt ® 0 Dt dt Example 5. The velocity of a point depends on time t, as v = c + t b where c and b are constant vectors. Find the instantaneous acceleration at any time t. Sol. Acceleration at any time t, dv d a= = (c + t b ) dt dt a = 0+ b = b
IMPORTANT FEATURES 1. If a body is moving continuously in a given direction on a straight line, then the magnitude of displacement is equal to distance. 2. Generally, the magnitude of displacement is less or equal to distance. 3. Many paths are possible between two points. For different paths between two points, distances are different but magnitudes of displacement are same. 4. The slope of distance-time graph is always greater or equal to zero. 5. The slope of displacement-time graph may be negative. 6. If a particle travels equal distances at speeds v1, v2, v3, ...... etc. respectively, then the average speed is harmonic mean of individual speeds. 2v1 v 2 7. If a particle moves a distance at speed v1 and comes back with speed v2, then v av = v1 + v 2 but v av = 0 8. If a particle moves in two equal intervals of time at different speeds v1 and v2 respectively, then v + v2 v av = 1 2 9. The average velocity between two points in a time interval can be obtained from a position versus time graph by calculating the slope of the straight line joining the co-ordinates of the two points. x2
x2
x1
x1
t1
t2 (a)
B A
( x2 – x1)
( t 2 – t1)
t1
t2 (b)
The graph [shown in fig.], describes the motion of a particle moving along x-axis (along a straight line). Suppose we wish to calculate the average velocity between t = t1 and t = t2. the slope of chord AB [shown in fig.(b)] gives the average velocity.
www.ashoksirphysics.com
4
KINEMATICS x 2 - x1 t 2 - t1 10. If a body moves with constant velocity, the instantaneous velocity is equal to average velocity. The instantaneous speed is equal to modulus of instantaneous velocity.
Mathematically,
v av = tan q =
11. x-component of displacement is y-component of displacement is z-component of displacement is
ò v dt Dy = ò v dt Dz = ò v dt Dx =
x
y
z
Thus, displacement of particle is Dr = Dx ˆi + Dy ˆj+ Dz kˆ 12. If particle moves on a straight line, (along x-axis), then v = 13. 14. 15. 16. 17.
dx . dt
The area of velocity-time graph gives displacement. The area of speed-time graph gives distance. The slope of tangent at position-time graph at a particular instant gives instantaneous velocity at that instant. The slope of velocity-time graph gives acceleration. The area of acceleration-time graph in a particular time interval gives change in velocity in that time interval.
ONE, TWO AND THREE DIMENSIONAL MOTION One Dimensional Motion If only one of the three co-ordinates is required to specify the position of an object in space changing w.r.t. time, then the motion of the object is called one dimensional motion. Motion of a particle in a straight line can be described by only one component of its velocity and acceleration. For example, motion of a block in a straight line, motion of a train along a straight track, a man walking on a level and narrow road, an object falling under gravity, etc. Two Dimensional Motion If two of the three co-ordinates are required to spacify the position of an object on space changing w.r.t. time, then the motion of the object is called two dimensional motion. The motion of a particle through its vertical plane at some angle with horizontal. (¹ 90º) is an example of two dimensional (2–D) motion. This is a projectile motion. Similarly, a circular motion is an example of 2–D motion. A 2–D motion takes place in a plane and its velocity (or acceleration) can be described by two components in any two mutually perpendicular directions (vx and vy). Three Dimensional Motion If all the three co-ordinates are required to specify the position of an object in space changing w.r.t. time, then the motion of an object is called three dimensional motion. Such a motion is not restricted to a straight line or plane but takes place in space. In a 3–D motion velocity and acceleration of a particle can be resolved in three components (vx, vy, vz, ax, ay, az). A few examples of 3–D motion are a flying bird, a flying kite, a flying aeroplane, the random motion of gas molecules, etc. UNIFORMLY ACCELERATED MOTION A motion, in which change in velocity in each unit of time is constant, is called uniformly accelerated motion. So, for uniformly accelerated motion, acceleration is constant or approximately. So for uniformly accelerated motion r ( a = constant) , equations of motion are as under,, r r r ...(i) v= u+ a t
www.ashoksirphysics.com
5
KINEMATICS r r 1r s = u t + a t2 ...(ii) 2 r r r r r r and ...(iii) v . v = u . u + 2a . s r where u = initial velocity of particle r v = velocity at time t r s = displacement of particle at time t If motion is described in one dimension, so vector sign () need not to be used. Normally, vertical upward motion is taken negative and vertical downward motion is taken positive. Similarly, for horizontal rightward motion is taken positive and leftward motion is taken negative. Sign convention : Sign convention for (a) motion in vertical (b) motion in horizontal, is shown in Fig.
+ve
–ve (a)
+ve (b)
–ve
Example 6. At a distance L = 400m from the traffic light brakes are applied to a locomotive moving at a velocity v = 54 km/hr. Determine the position of the locomotive relative to the traffic light 1 min after the application of the brakes if its acceleration is –0.3 m/sec2. 5 Sol. u 54 15 m / s 18 a = –0.3 m/s2 v = u + at 0 = 15 – 0.3 t0 15 t0 = = 50 sec 0.3 After 50 second, locomotive comes in rest permanently. v2 = u2 + 2as O2 = 152 – 2 × 0.3 S0
S0 =
225 2250 = = 375m 0.6 6
the distance of the locomotive from traffic light = 400 – 375 = 25 metre
Example 7. A car moves in the x–y plane with acceleration (3iˆ + 4 ˆj) m / s 2 . (a) Assuming that the car is at rest at the origin at t = 0, derive expression for the velocity as function of time. (b) Find the equation of path of car and find the position vector as function of time. Sol. Here, ux = 0, uy = 0, uz = 0 2 2 ax = 3 m/s , ay = 4 m/s (a) vx = ux + axt or vx = 3t and vy = uy + ayt
www.ashoksirphysics.com
6
KINEMATICS or
vy = 4t r v = v x ˆi + v y ˆj r v = (3tiˆ + 4tjˆ) 1 2 a t 2 x 1 3 ´ 3t 2 = t 2 2 2 1 2 uyt + a yt 2 1 (4)t 2 = 2t 2 2 2 4 2´ x = x 3 3
x = uxt +
(b) or
x=
and
y=
or
y=
y=
æ 3 2ö çQ x= t ÷ è 2 ø
4 x 3 Hence, the path is straight line. The position of car is r 3 r = x iˆ + y ˆj = t 2 iˆ + 2t 2 ˆj 2
y=
Motion Under Gravity The most familiar example of motion with costant acceleration on a straight line is motion in a vertical direction near the surface of earth. If air resistance is neglected, the acceleration of such type of particle is gravitational acceleration which is nearly constant for a height negligible with respect to the radius of earth. The magnitude of gravitational acceleration near surface of earth is g = 9.8 m/s2 = 32 ft/s2. Discussion : Case-I : If particle is moving upwards : In this case applicable kinematics relations are : g v = u – gt ...(i) 1 h = ut - gt 2 ...(ii) u 2 v2 = u2 – 2gh ...(iii) Here, h is the vertical height of the particle in upward direction. NOTE : For maximum height attained by projectile h = hmax, v=0 2 2 i.e., (0) = u – 2ghmax
hmax =
u2 2g
Case-II : If particle is moving vertically downwards : In this case : v = u + gt ...(i) v2 = u2 + 2gh ...(ii)
www.ashoksirphysics.com
u g
7
KINEMATICS 1 2 gt ...(iii) 2 Here, h is the vertical height of the particle in downward direction. h = ut +
Example 8. A particle is projected vertically upwards with velocity 40 m/s. Find the displacement and distance travelled by the particle in (a) 2s (b) 4s (c) 6s [Take g = 10 m/s2] Sol. Here, u is positive (upwards) and a is negative (downwards). So, first we will find t0, the time when velocity becomes zero.
t0 =
u 40 = = 4s a 10
(a) t < t0. Therefore, distance and displacement are equal. d = s = ut +
1 2 at 2
1 ´ 10´ 4 = 60 m 2 (b) t = t0. So, again distance and displacement are equal. 1 d = s = ut + at 2 2 1 d = 40´ 4- ´ 10´ 16 = 80m 2 (c) t > t0. Hence, d > s 1 s = 40 ´ 6 - ´ 10´ 36 = 60 m 2 d = 40 ´ 2 -
While
d=
u2 1 2 + a (t - t 0 ) 2a 2
d=
(40)2 1 + ´ 10´ (6 - 4)2 = 100 m 2 ´ 10 2
Example 9. A ball is thrown upwards from the ground with an initial speed of u. The ball is at a height of 80 m at two times, the time interval being 6s. Find u. Take g = 10 m/s2. Sol. Here, u = u m/s, a = g = – 10 m/s2 and s = 80 m. 1 Substituting the value in s = ut + at 2 , we have 2 2 80 = ut – 5t or 5t2 – ut + 80 = 0 or and
t= t=
u+ u-
u 2 - 1600 10 u 2 - 1600 10
Now, it is given that
www.ashoksirphysics.com
+ve
–ve
s = 80 m u
8
KINEMATICS
u+
u 2 - 1600 u 10
u 2 - 1600 =6 10
u 2 - 1600 or =6 or u 2 - 1600 = 30 5 or u2 – 1600 = 900 u2 = 2500 or u = ± 50 m/s Ignoring the negative sign, we have u = 50 m/s A
Example 10. A disc arranged in a vertical plane has two groves of same length directed 60º along the vertical chord AB and CD as shown in the fig. The same particles slide D down along AB and CD. The ratio of the time tAB/t CD is : (A) 1 : 2 (B) 1 : 2 (C) 2 : 1 (D) 2 : 1 B 1 2 SAB = g t AB Sol. 2 1 2 SCD = g cos 60º t CD 2 A C But SAB = SCD 60 60º º 60º 1 2 gcos g t g AB SAB 2 D = g SCD 1 g cos 60º t 2 CD 2 B t AB t 2AB = 1 : 2 1 = 2 or t CD t 2CD
C
Example 11. A stone is dropped from a height h. Simultaneously another stone is thrown up from the ground with such a velocity that it can reach a height of 4h. Find the time when two stones cross each other. Sol. For second stone, v 2 = v02 - 2g (4h) 0 2 = v 20 - 8gh
v 0 8gh
but they meth at height H in time t0. Displacement of 1st stone is 1 h – H gt 20 ...(1) 2 and that of second stone is 1 H = v0 t 0 - gt 02 ...(2) 2 After solving eqn (1) and (2) 1 1 h – v 0 t 0 g t 02 g t 02 or 2 2 h = v0t0
www.ashoksirphysics.com
v=0
(1) (2)
4h
h v0
9
KINEMATICS
t0 =
h h = v0 8gh
t0 =
h 8g
Example 12. A rocket is launched at an angle 53º to the horizontal with an initial speed of 100 ms–1. It moves along its initial line of motion with an acceleration of 30 ms–2 for 3 seconds. At this time its engine fails & the rocket proceeds like a free body. Find : (i) the maximum altitude reached by the rocket (ii) total time of flight. Sol. S0 = ut + ½ at 2 = 100 × 3 + ½ × 30 × 9 = 300 + 135 = 435 m In OAB h sin 53º = S0 4 h S0 sin 53º 435 87 4 348 m 5 v0 = u + at = 100 + 30 × 3 = 190 m/s (velocity at the time of switch off) After engin switch off 3 v0x v0 sin 37º 190 114 m / s 5 4 v0y v0 cos37º 190 152 m / s 5 ay = – 10 m/s2, ax = 0 (i) At maximum altitudes vy = 0 v 2y = v 20y + 2a y h 0
0 = v 20y + 2a y h 0 h0 –
A S0
v20y
u 53º
2a y
152 152 h0 2 10 11552 h0 = h 0 = 1155.2 m 10 The maximum altitude reached by the rocket is
O
37º v0
37º h
Engin fail x
B
= h0 + h = (1155.2 + 348) m
1503.2 m
(ii)Total time of flight. 1 y = v0 y t + a y t 2 2 1 - h = v 0y t 0 + a y t 02 2 1155.2 152t 0
1 10 t 02 2
www.ashoksirphysics.com
10
KINEMATICS - 1155.2 = 152t 0 - 5t 02 5 t 20 - 152 t 0 - 1155.2 = 0 t 02 - 30.4 t 0 - 231.04 = 0
t 0 = 35.54 sec.
NON-UNIFORMLY ACCELERATED MOTION When motion of a particle is not uniform i.e., acceleration of particle is not constant or acceleration is a function of time, then following relations hold for one dimensional motion : (i)
v=
ds dt
dv dv = v dt ds (iii) ds = vdt and (iv) dv = adt or vdv = ads Such problems can be solved either by differentiation or integration applying some boundary conditions.
(ii)
a=
Example 13. A particle is moving with a velocity of v = (3 + 6t + 9t2) cm/s. Find out : (a) the acceleration of the particle at t = 3 s. (b) the displacement of the particle in the interval t = 5 s to t = 8 s. Sol. (a) Acceleration of particle a=
dv = ( 6 + 18t ) cm / s 2 dt
At t = 3 s, a = (6 + 18 × 3) cm/s2 a = 60 cm/s2 (b) Given, v = (3 + 6t + 9t2) cm/s
or
ds = (3+ 6t + 9t 2 ) dt ds = (3 + 6t + 9t2)dt
ò
s = ëé 3t + 3t 2 + 3t 3 ûù5 or s = 1287 cm
or
8
5
ds =
8
ò (3+ 6t + 9t ) dt 2
5
8
Example 14 : The motion of a particle along a straight line is described by the function x = (2t – 3)2 where x is in metres and t is in seconds. (a) Find the position, velocity and acceleration at t = 2 s. (b) Find the velocity of the particle at origin. Sol. (a) Position, x = (2t – 3)2 Velocity,
v
dx 4 2t 3 m / s dt
and acceleration, a =
dv = 8 m / s2 dt
www.ashoksirphysics.com
11
KINEMATICS At t = 2 s, x = (2 × 2 – 3)2 = 1.0 m v = 4(2 × 2 – 3) = 4 m/s and a = 8 m/s2 (b) At origin, x = 0 or (2t – 3) = 0 v=4×0=0 EQUATIONS OF MOTION FOR VARIABLE ACCELERATION Case-I : When acceleration a of the particle is a function of time : Since, acceleration of a particle is a function of time, i.e., a = f(t) dv = f (t) dt dv = f(t)dt Integrating within the proper limits, we get
v= u+
t
ò0 f (t)dt
Case-II : When acceleration a of the particle is a function of distance : Since, acceleration of a particle is a function of distance, i.e., a = f(x)
dv = f (x) dt
dv dx . = f (x) dx dt vdv = f(x)dx Integrating with in proper limits, we get
x
v 2 = u 2 + 2ò f (x)dx x0
Case-III : When acceleration a of the particle is a function of velocity : Since, acceleration of a particle is a function of velocity, i.e., a = f(v) dv = f (v) dt dv dt = f (v) Integrating within proper limits, we get
t=
v
dv
òu f (v)
Therefore, we get v as a function of t i.e., v(t). Again
dv = f (v) dt dv dx . = f (v) dx dt dv v = f (v) dx vdv dx = f (v)
www.ashoksirphysics.com
12
KINEMATICS x
òx
v
vdv
v
vdv
v
vdv
dx =
òu f (v)
x - x0 =
òu f (v)
x = x0 +
òu f (v)
0
Therefore, we get x as a function of v i.e., x(v). GRAPHS IN ONE DIMENSIONAL MOTION The tabular forms of s–t and v–t graphs are given for one-dimensional motion with uniform velocity or with constant acceleration. Table-1 : Displacement-Time Graph S.No. Different Cases
s–t Graph
The main Features of Graph
s
1.
At rest
Slope = v = 0 t s
2.
s = ut
Uniform motion
s = 0 at t = 0 t
s
3.
1 s = 2 at 2
Uniformly accelerated motion with u = 0, s = 0 at t = 0
t
u = 0 i.e., slope of s–t graph at t = 0, should be zero.
s
4.
1 s = ut + 2 at2
Uniformly accelerated motion with u ¹ 0 but s = 0 at t = 0
t
Slope of s–t graph gradually goes on increasing.
s
5.
Uniformly accelerated motion with u ¹ 0 but s = s0 at t = 0
s0
2 s = s0 + ut + 1 2 at
s = s0 at t = 0
t
s
6.
Uniformly retarded motion t0
t
At t = t0, slope of s–t graph becomes zero.
Table-2 : Velocity-Time Graph S.No. Different Cases
v–t Graph v
1.
The main Features of Graph
v = constant a=0
Uniform motion t
www.ashoksirphysics.com
(i) Slope of v–t graph = a = 0 (ii) v = constant 13
KINEMATICS v
2.
v = at
Uniformly accelerated motion with u = 0, s = 0 at t = 0
t
(i) u = 0 i.e., v = 0 at t = 0 (ii) a or slope of v–t graph is constant.
t
At t = 0, v¹ 0 and slope of v–t graph is not zero.
v v = u + at
u
3.
Uniformly accelerated motion with u ¹ 0 but s = 0 at t = 0 v v = u + at
u
4.
Uniformly accelerated motion with u ¹ 0 but s = s0 at t = 0
v = u at t = 0 t
v u v = u – at
5.
Uniformly decelerated motion
Slope of v–t graph = –a (retardation) t
t0 v
6.
Non-uniformly accelerated motion
Slope of v–t graph increases with time
t
Acceleration-Time Graph (i) When a–t graph is a straight line parallel to time-axis, then acceleration = a = constant. a
Slope = 0
t (a)
(ii) When a–t graph is a straight line passing through origin, then acceleration of particle is increasing uniformly. a op Sl
e=
e +v
t (b)
(iii) When a–t graph is a straight line of negative slope, then acceleration is decreasing uniformly. a
Sl op e=
–v e
t (c)
www.ashoksirphysics.com
14
KINEMATICS v 20
Example 15. The fig. shows the v–t graph of a particle moving in straight line. Find the time when particle returns to the starting point. 10 Sol. When the particle comes at initial position, total displacement is zero. Since, the area of v – t graph gives displacement. In this case area of v – t graph should be zero. 1 1 S 20 25 t 25 v 0 2 2 v0 or 0 250 – t 25 2 500 or t – 25 v ...(1) 0 v 20 0 Also tan from figure 25 20 t 25 v0 or 4 t – 25 v 0 4 t – 25 ...(2) From eqn (1) and (2) 500 125 t – 25 4 t – 25 t – 25
10 20
25 t
v 20
20
25
t
20
t(s) 40
v0
t – 25 2 125 t – 25 125 5 5
t = (25 + 5 5 ) sec.
t = 36.2 sec
Example 16. From the velocity-time plot shown in figure, find the distance travelled by the particle during the first 40 seconds. Also find the average velocity during this period. Sol. The distance fravelled by the particle during the first 20 second. 1 S1 v t 2 1 S1 5 20 S1 = 50 m 2 The distance travelled by the partile during next 20 second is 1 S2 5 20 2 S2 = 50 m Since distance is a sclar quantity therefore total distance = S1 + S2 = 50 + 50
V 5m/s
–5m/s
S = 100 m
Example 17. A ball is dropped from a height of 80m on a floor. At each collision the ball losses half of its speed. Plot the speed-time graph and velocity-time graph of its motion till two collisions with the floor, [Take g = 10 ms–2].
www.ashoksirphysics.com
15
KINEMATICS Sol. The time in first collision 1 2 gt (during downward motion) 2 1 80 = 0 + ´ 10 ´ t 2 (Q u = 0) or 2 2´ 80 t= = 4s or 10 Final speed just before first collision v = 0 + 10 × 4 = 40 m/s h = ut +
æ 40 ö ÷m / s. It now loses half of initial speed after the collision i.e., when it first bounces its initial speed is 20çè = 2ø So, the time is loosing half of its speed. 0 = 20 – 10 × t´ (during upward motion) 20 t´= = 2s (final speed = 0) 10 In 2 s, it attains height 1 h´= 20´ 2 - ´ 10´ (2) 2 2 h´ = 40 – 20 = 20 m Now, it is dropped again from 20m with zero initial speed. Time taken in reaching the ground 1 20 = 0 + ´ 10(t) 2 2 t=2s Also final speed v´2 = 0 + 2 × 10 × 20 (from v2 = u2 + 2gh) v´ = 20 m/s Thus, with the above data, we can draw the speed-time graph. Speed (m/s) 40 20 4
6
Time(s)
8
Since, velocity is a vector quantity so from the above graph, we can now draw the velocity-time graph. [Take downward motion positive and upward motion negative in case of v–t graph] Velocity (m/s) 40 20 4
6
Time(s)
8
–20
Example 18. The velocity-time graph of an object moving along a straight line is as shown in the fig. Calculate the distance covered by the object : 20 ms–1
A
B
A´ 2 t (s)
5
v O
0
www.ashoksirphysics.com
B´
C 10
16
KINEMATICS (a) between t = 0 to t = 5 s and (b) between t = 0 to t = 10 s. Sol. (a) Let x1 be the distance covered in the time interval between t = 0 to t = 5 s. Then, x1 = area of the trapezium OABB´ æ AB + OB´ ö 3+ 5 =ç ´ 20 = 80 m ÷´ AA´= è ø 2 2 (b) Let x2 be the distance covered in the time interval between t = 0 to t = 10 s. Then, x2 = area of the trapezium OABC. AB + OC 3 + 10 = ´ AA´= ´ 20 = 130 m 2 2 IMPORTANT FEATURES 1. For a particle having zero initial velocity if v t, s t2 and v2 s then acceleration of particle must be constant i.e., particle is moving rectilinearly with uniform acceleration. 2. For a particle having zero initial velocity if s t, where > 2, then particle’s acceleration increases with time. 3. For a particle having zero initial velocity if s t, where < 0, then particle’s acceleration decreases with time. 4. When a body is non-uniformly accelerated, then problem can be solved either be differentiation or integration (applying some boundary conditions). Differentiation : s – t v – t a – t Integration : a–tv–ts–t By boundary condition we mean that velocity or displacement at some time (usually at t = 0) should be known to us. Otherwise we cannot find constant of integration. dv 5. Equation a = v or vdv = ads is useful when acceleration displacement equation is known and velocity ds displacement equation is required. 6. When either u = 0 or u a , motion is only accelerated. 7. It can be observed when either u = 0, u a or u ¯ a . 8. When u ¯ a motion is first retarded (till the velocity becomes zero) and then accelerated in opposite direction. 9. Following are few points we may conclude in case of a one dimensional motion : æ ds ö (a) slope of displacement-time graph gives velocityçè as v = ÷ . dt ø æ dv ö ÷. (b) slope of velocity-time graph gives accelerationçè as a = dt ø (c) area under velocity-time graph gives displacement as (ds = vdt). (d) area under acceleration-time graph gives change in velocity (as dv = adt). (e) displacement-time graph in uniform motion is a straight line passing through origin, if displacement is zero at time t = 0 (as s = vt). (f) velocity-time graph is a straight line passing through origin in a uniformly accelerated motionif initial velocity u = 0 and a straight line not passing through origin if initial velocity u¹ 0 (as v = u + at). æ 1 2ö (g) displacement-time graph in uniformly accelerated or retarded motion is a parabolaçè as s = ut ± at ÷ . 2 ø 10. Slopes of v–t or s–t graphs can never be infinite at any point, because infinite slope of v–t graph means infinite acceleration. Similarly, infinite slope of s–t graph means infinite velocity. Hence, the following graphs are not possible :
www.ashoksirphysics.com
17
KINEMATICS v
s
t
t
11. At one time, two values of velocity or displacement are not possible. Hence, the following graphs are not acceptable : v
s
v1 v2
s1 s2 t0
t
t0
t
RELATIVE MOTION If the velocities of two bodies are known w.r.t a common frame of reference, then the velocity of a body can be measured w.r.t. the second body. Therefore, if the velocities of two bodies A and B w.r.t. the ground are v A and v B then the relative velocity of A w.r.t. B is v AB = v A - v B Similarly, we see that v AB = - vBA Also, relative acceleration of A w.r.t. B is and a AB = a A - a B a AB = - a BA Example 19. Car A has an acceleration of 6 m/s2 due east and car B, 8 m/s2 due north. What is the acceleration of car B w.r.t. to car A ? Sol. It is a two dimensional motion. aBA aB = 8 m/s 2 Therefore, a BA = acceleration of car B w.r.t. car A N = aB - aA W E a BA = (6) 2 - (8)2 = 10 m / s 2 S and Thus, a BA
æ8 ö æ4 ö –aA = 6 m/s 2 a = tan- 1ç ÷= tan- 1ç ÷ è 6ø è 3ø -1æ 4 ö is 10 m/s2 at an angle of α = tan çè ÷ from west towards north. 3ø
Discussion (a) If a satellite is moving in equatorial plane with velocity v and a point on the surface of earth with velocity u relative to the centre of earth, the velocity of satellite relative to the surface of earth vSE = v - u (b) If a car is moving at equator on the earth’s surface with a velocity v CE relative to earth’s surface and a point on the surface of earth with velocity vE relative to its centre, then v CE = vC - v E (c) If the car moves from west to east (the direction of motion of earth)
www.ashoksirphysics.com
18
KINEMATICS vC = vCE + vE and if the car moves from east to west (opposite to the motion to earth) vC = vCE – vE (d) For crossing the river in shortest time, the boat should sail perpendicular to the flow. If the width of river is d and v the velocity of boat in still water, then, d t= v The position of boat at the other bank is C (not B). The displacement of the boat = OC = OB + BC
B
vr
C
vr v
O
(OB) 2 + (BC) 2
OC =
æ d ö2 OC = d + (v r t) = d + ç v r ÷ è vø (e) For crossing the river in shortest distance, the boat moves as such its horizontal component of velocity balances the speed of flow. A vr B OB = the shortest path = d vr v v sinθ = r vr = v sin v 2
2
2
cos θ = 1 sin 2θ
O
é æ v ö2 ù æ v 2 - v 2 ö r ÷ cos θ = ê 1ç r ÷ ú = ç è ø v v ø ë û è d d t= = v cos q v v 2 - v 2 r
v
(f)
t=
d 2
v - v 2r In this case, the magnitude of displacement = d. If boat crosses the river along the shortest path, then time is not least.
Example 20. A police van moving on a highway with a speed of 30 km/h fires a bullet at a thief’s car speeding away in the same direction with a speed of 192 km/h. If the muzzle speed of the bullet is 150 m/s, with what speed does the bullet hits the thief’s car ? Sol. Let v p , v b and v t represent velocity of the police van, muzzle velocity of bullet and the velocity of the thief’s ’s car respectively. This is a one dimensional motion. 25 v p = 30km / h = m/s 3 v b = 150 m / s 160 v t = 192 km / h = m/s and 3 Since, bullet is fired from the moving police van, the effective velocity of the bullet will be 25 475 v´t = v b + v p = 150 + = m/s 3 3
www.ashoksirphysics.com
19
KINEMATICS if the relative velocity of the bullet w.r.t the thief’s car is v bt , then v bt = v´b - v t 475 160 315 v bt = = = 105 m / s 3 3 3
Example 21. A, B & C are three objects each moving wit constant velocity. A’s speed is 10m/sec in a uur direction PQ . The velocity of B relative to A is 6 m/sec at an angle of cos–1(15/24) to PQ. The velocity uur of C relative to B is 12 m/sec in a direction QP , then find the magnitude of the velocity of C. r Sol. v A = 10 ˆi r v B 6 cos iˆ 6sin ˆj 15 351 Here cos , sin 24 24 r And v CB = - 12 ˆi r r r v BA = v B - vA r or 6cos i 6sin j v B 10 ˆi r v B cos iˆ 6sin ˆj 10 ˆi 6
=
15 ˆ 351 ˆ i6 j 10 ˆi 24 24 351 ˆ 15 ˆ 4 10 i 4 j
55 ˆ 351 ˆ i j 4 4 r r r v CB = vC - v B r r r 55 351 ˆ v C = vCB + v B = - 12 iˆ + ˆi + j 4 4 7 351 ˆ = ˆi + j 4 4
But
2 7 351 vC 4 4
=
49 + 351 = 4
2
400 20 = 4 4
Ans.
5 m/s
Example 22. A man crosses a river in a boat. If he crosses the river in minimum time he takes 10 min with a drift 120 m. If he crosses the river taking shortest path, he takes 12.5 min, find : (a) width of the river B (b) velocity of the boat with respect to water, (c) speed of the current. d v Sol. Let vr = velocity of river v = velocity of river in still water and A d = width of river For minimum time
www.ashoksirphysics.com
20
KINEMATICS Given,
tmin = 10 min
d = 10 v Drift in this case will be, x = vrt 120 = 10vr Shortest path is taken when vb is along AB. In this case,
or
Now,
vb =
v 2 - v r2
125 =
d = vb
...(i)
...(ii)
vr
d v 2 - v 2r
Solving these three equations we get, v = 20 m/min, vr = 12 m/min and d = 200 m.
B
...(iii) v
vb
A Shortest path
Example 23. A man with some passengers in his boat, starts perpendicular to flow of river 200m wide and flowing with 2m/s. boat speed in still water is 4m/s. When he reaches half the width of river the passengers asked him they want to reach the just opposite end from where they have started. (a) Find the direction due to which he must row to reach the required end. (b) How many times more total time, it would take to that if he would have denied the passengers. Sol. (a) Event (1) — From A to B, Time taken by boat to recent from A to B is 100 100 t= = = 25sec. vy 4 Also, AD = vx t = 2t = 50 m Event (2) — From B to C, Actual velocity of boat should be along BC. This actual velocity is found by resultant of vrel and vr. r r r v rel = v b - vr x–comput of actual velocity is y vx = vr – vrel sin = 2 – 4sin C E vb and y – comput of actual velocity is B 200m vrel vy = vrel cos vrel vr The time taken to go from B to C is D A BE t0 = vy 100 100 v rel cos 4 cos 25 cos EC = –vx t 0 = –(2 – 4 sin ) t 0 25 4 sin 2 cos EC = AD = 50 m
Also,
But
www.ashoksirphysics.com
21
KINEMATICS
(b)
25 cos 25 50 4 sin 2 cos 50 cos = 100 sin – 50 cos = 2 sin – 1 1 + cos = 2 sin 2cos 2 4sin cos 2 2 2 a 1 tan = 2 2 1 tan 1 2 2 EC 4 sin 2
1 2 tan 1 2 If boat crosses the river with initial condition 200 200 t1 = = vy vrel
200 = 50sec. 4 If boat cross the river with final condition, t 2 = t AB + t BC t2 = t + t0 25 t 2 25 cos 1 tan Q 2 2 2 cos 2 5 cos 2 cos 2 1 2 4 2 1 5 8 3 = - 1= 5 5 25 125 200 t 2 = 25 + = 25 + = 3 3 3 5 t2 4 t2 200 4 = t1 3 t1 3 50 3 t1 =
Example 24. To a person going west wards with a speed of 6 km/h rain appears to fall vertically downwards with a speed of 8 km/h. Find the actual direction of rain. Sol. Let v M = velocity of man = 6 km/h v = relative velocity of rain w.r.t. man = 8 km/h
www.ashoksirphysics.com
22
KINEMATICS Vertical v R = actual velocity of rain N In this case v = v R + (- v M ) vM O W E A v = vR - vM v vR B or C vR = vM + v S S v R = (6) 2 + (8)2 = 10 km / h The velocity of rain ( v R ) is given by the vector OC , the resultant of vectors OA and OB as shown in figure. If is the angle that v R makes with the vertical, then BC | v M | 6 tan 0.75 OB | v | 8 or = 36º 52´ (east of vertical) Example 25. Rain is falling vertically with a speed of 20 ms–1 relative to air. A person is running in the rain with a velocity of 5 ms–1 and a wind is also blowing with a speed of 15 ms–1 (both towards east). Find the angle with the vertical at which the person should hold his umbrella so that he may not get drenched. r Sol. v ra = - 20 kˆ z N r v m = 5 ˆi r E v a = 15 ˆi x W r r r v ra = v r - v a r S v r = - 20 kˆ + 15 ˆi r r r v rm = v r - v m = - 20kˆ +15iˆ - 5iˆ = - 20 kˆ + 10 ˆi 10 1 tan 20 2 1 tan1 2
vrm
vertically
Example 26. An aircraft flies at 400 km/h in still air. A wind of 200 2 km / h is blowing from the south. The pilot wishes to travel from A to a point B north east of A. Find the direction he must steer and time of his journey if AB = 1000 km. Sol. Given that vw = 200 2 km / h . vaw = 400 km/h and v a should be along AB or in north-east direction. Thus, the direction of v aw should be such as the resultant of v w and v aw is along AB or in north-east direction. N B va A
45º
www.ashoksirphysics.com
45º vw = 200 2 km/h C vaw = 400 km/h E
23
KINEMATICS Let v aw makes an angle with AB as shown in fig. Applying sine law in triangle ABC, we get AC BC sin 45º sin BC sin sin 45º or AC 200 2 1 1 sin 400 2 2 = 30º therefore, the pilot should steer in a direction at an angle of (45º + ) or 75º from north towards east. | va | 400 Further, sin(180º 45º 30º ) sin 45º sin105º km | v a | (400) or sin 45º h km 0.9659 km cos15º | va | (400) (400) sin 45º 0.707 h h km | va | 546.47 h The time of journey from A to B is AB 1000 t h t = 1.83 h | va | 546.67
Example 27. A glass wind screen whose inclination with the vertical can be changed, is mounted on a cart as shown in figure. The cart moves uniformly along the horizontal path with a speed of 6 m/s. At what maximum angle to the vertical can the wind screen be placed so that the rain drops falling vertically downwards with velocity 2 m/s, do not enter the cart ? r Sol. v c = 6 ˆi r v r = 2 ˆj r r r v rc = v r - vc vrc v 6 tan c 3 vr 2 AE l AE l cos ED l sin tan BE l l cos sin 3 2sin 2 / 2 2sin cos 2 2 cot 3 2 2sin 2 2
v=6m/s
vc
D
x
vr y
cos
www.ashoksirphysics.com
A cos sin
E
vr
D
B C
24
KINEMATICS or or or
3 2 1 tan 2 3 1 tan 1 2 3
cot
1 2 tan 1 3
MOTION IN TWO AND THREE DIMENSIONS INTRODUCTION A body is free to move in space. In this case, the initial position of body is taken as origin. Any convenient co-ordinate system is chosen. Let us suppose that at an instant t, the body is at point P(x, y, z). The position vector of the body is r = x iˆ + y ˆj+ z kˆ . Thus, velocity dr dx ˆi + dy ˆj+ dz kˆ v= = dt dt dt dt dx The velocity along x-axis is, v x = dt dv x and acceleration along x-axis is a x = . dt dy The velocity along y-axis is v y = dt dv y and the acceleration along y-axis is a y = dt dz dv z Similarly, v z = and a z = dt dt The acceleration of the body a = a ˆi + a ˆj+ a kˆ . x
y
z
Discussion (a) If ax is constant, vx = ux + axt 1 x = uxt + axt2 2 v 2x = u x2 + 2a x x If ax is a variable,
ò v x dx ò dv x = ò a x dt x=
(b) If ay is constant 1 2 a t 2 y vy = uy + ayt y = u yt +
v 2y = u 2y + 2a y y
www.ashoksirphysics.com
25
KINEMATICS If ay is variable,
ò v ydt ò dv y = ò a y dt y=
(c) If az is constant, vz = uz + azt 1 z = uzt + azt2 2 2 2 v z = u z + 2a z z If az is variable,
ò vz dt ò dvz = ò a z dt z=
If the motion of the body takes place in x–y plane, then az = 0, vz = 0, uz = 0 Example 28. A bird flies in the x–y plane with a velocity v = t 2 ˆi + 3t ˆj . At t = 0, bird is at origin. Calculate position and acceleration of bird as function of time. Sol. We have given v = t 2 ˆi + 3t ˆj
Here, Since,
vx = t2, vy = 3t and vz = 0 vx = t2
or
dx t2 dt
or
0 dx 0 t dt
or Also,
x
t 2
t3 3 vy = 3t x
or
dy 3t dt
or
0 dy 0 3t dt
y
t
3t 2 2 Thus, position of bird is r x ˆi y ˆj t3 3t 2 ˆ r ˆi j 3 2 vx = t2 y
and
dv x d(t) 2 2t dt dt vy = 3t ax
www.ashoksirphysics.com
26
KINEMATICS
dv y
dt dt dt ay = 3 unit Thus, acceleration of bird is a a x ˆi a y ˆj a 2t iˆ 3 ˆj or
3
PROJECTILE MOTION We next consider a special case of two dimensional motion : A particle moves in a vertical plane with initial velocity v 0 but its acceleration is always the free fall acceleration g , which is downward. Such a particle is referred to as a projectile (meaning that is projected or launched) and its motion is called projectile motion. A projectile might be a baseball in flight or a golf ball, but it is not air plane or a duck in flight. Or goal here is to analyze projectile motion using the tools for two-dimensional motion and making the assumption that air has no effect on the projectile. Now let us consider a projectile launched so that its initial velocity v0 makes an angle with with the horizontal (shown in figure). For discussion of motion, we take origin at the point of projection. Horizontal direction as xaxis and vertical direction as y-axis is taken. y
g
v0
O
A
x
The initial velocity of projectile along x-axis is ux = u cos . The component of gravitational acceleration along x-axis is ax = g cos 90º = 0. The component of initial velocity along y-axis is uy = u sin . The acceleration along y-axis is ay = – g NOTE : In projectile motion, the horizontal and the vertical motions are independent of each other, that is, neither motion affects the other. Discussion (a) The instantaneous velocity of the projectile as function of time : Let projectile reaches at point (x, y) after time t [Fig.]. y vx = ux = u cos vy and vy = uy – gt = u sin – gt g u vx P(x,y) ˆ ˆ v vx i vy j O x A v u cos ˆi (u sin gt) ˆj The instantaneous speed | v | (u cos ) 2 (u sin gt) 2 Also,
x = uxt = (u cos ) t = ut cos
www.ashoksirphysics.com
27
KINEMATICS 1 y u y t gt 2 2 1 y u sin t gt 2 2 The position of the projectile is r x ˆi y ˆj
or
1 r ut cos ˆi ut sin gt 2 ˆj 2
(b) Trajectory of projectile : The y–x graph gives the path or trajectory of the projectile. From discussion of instantaneous velocity of projectile. x = ut cos ...(i) 1 y ut sin gt 2 and ...(ii) 2 x t ...(iii) u cos Putting the value of t from Eq. (iii) into Eq. (ii). x 1 x y u sin g u cos 2 u cos
2
gx 2 y x tan 2 or 2u cos 2 This is the required path or projectile.
Multiplying the eq. (iv) by
x2
...(iv)
2u 2 cos 2 to both sides, we get g
2u 2 cos 2 2u 2 sin cos x y g g 2
u 2 sin cos Adding to both sides, we get g 2
2u 2 cos 2 u 2 sin cos u 2 sin 2 x y g g 2g This is of the form, (x – a)2 = – c(y – b) ...(v) which is the equation of a parabola. Hence, the equation of the path of the projectile is a parabola. NOTE : The trajectory of a projectile will be parabolic when direction of velocity of projectile is different from direction of acceleration and its acceleration is constant both in magnitude and direction. (c) Time of flight : In Fig., the time taken by projectile to reach at point A from point O is known as time of flight. Here, OA = vxT, where T is time of flight. The total displacement along y-axis during motion of projectile from O to A is zero. So, y = 0
www.ashoksirphysics.com
28
KINEMATICS but
1 y u y T gT 2 2
or
1 0 u sin T gT 2 2
2u sin ...(vi) g (d) Range of projectile : Distance OA is known as range of projectile [Fig.] The time taken to reach point A from point O is T
T
2u sin g
2u sin The range R u x T u cos g
R u
2sin cos g
u 2 sin 2 ...(vii) g Caution : This equation does not give the horizontal distance travelled by a projectile when the final height is not the launch height. Regarding range of projectile, two cases are discussed below : Case-I : Range will be maximum if sin 2 = 1 or = 45º R
u2 (at = 45º) g Case-II : For given velue of v, the range of projectile will be same for angle and 90º – though their times of flight and maximum heights are different. R max.
R 90º
u 2 sin 2 90º g
R 90º
u 2 sin 180º 2 g
u 2 sin 2 R g Thus, for the case as shown in fig, R30º = R60º
y
R 90º
u 60º
O
u
30º
x
(e) Height attained by projectile : At the maximum height (at point B) the vertical component of velocity is zero.
or
v 2y u 2y 2gH 2
2
H
2
u sin 2g
www.ashoksirphysics.com
vy = 0 B
(0) = (u sin ) – 2gH 2
y
ux H
O A
x
29
KINEMATICS NOTE : • If air resists or opposes the projectile motion [Fig.], then y u
I II
O
0
0
>
x
0
I—Trajectory in vacuum II—Trajectory in presence of air resistance
• Time taken by projectile during upward motion < Time taken during downward motion. • The values of height attained and of range of a projectile decrease. • The projectile returns to the ground with less speed. At its trajectory its horizontal velocity also decreases. • Time of flight also decreases. • At which angle, the projectile returns to the ground, increases. Example 29. Prove that the maximum horizontal range is four times the maximum height attained by the projectile; when fired at an inclination so as to have maximum horizontal range. Sol. For = 45º, the horizontal range is maximum and is given by R max
u2 g
Maximum height attained
or
u 2 sin 2 45º u 2 R max H max 2g 4g 4 Rmax = 4 Hmax
Example 30. There are two angles of projection for which the horizontal range is the same. Show that the sum of the maximum heights for these two angles is independent of the angle of projection. Sol. There are two angles of projection and 90º – for which the horizontal range R is same. Now,
H1
u 2 sin 2 2g
u 2 sin 2 90º H2 and 2g 2 2 u cos H2 2g 2 u u2 2 2 H H sin cos Therefore, 1 2 2g 2g Clearly, the sum of the heights for the two angles of projection is independent of the angle of projection.
Example 31. A particle is projected upwards with a velocity of 100 m/sec at an angle of 60º with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g = 10 m/sec2. Sol. Here ax = 0 ay = –g ux = 100 sin60º = 50 3 uy = 100 cos60º = 50
www.ashoksirphysics.com
30
KINEMATICS
r u 0 = u x ˆi + u y ˆj = 50 3 ˆi + 50 ˆj r v y = u y + a y t = 50 – gt
y
v x = u x = 50 3 m / s r v = 50 3 ˆi + (50 - gt )ˆj r r But u and v are perpendicular.. r r u v0 or or
50
60º
x
3 ˆi 50 ˆj 50 3 ˆi 50 gt ˆj 0
7500 + 2500 – 500 t = 0 10000 t= 500
t 0 = 20 second
Example 32. Fig. shows a pirate ship 560 m from a fort defending the harbor entrance of an island. A defense cannon, located at sea level, fires balls at initial speed v0 = 82 m/s. (a) At what angle 0 from the horizontal must a ball be fired to hit the ship ? (b) How far should the pirate ship be from the cannon if it is to be beyond the maximum range of the cannonballs? y
6 3º 27º
x R = 560 m
Sol. (a) Because the cannon and the ship are at the same height, the horizontal displacement is the range. The horizontal range is R
v 20 sin 2 g
...(i)
which gives us gR 20 sin 1 2 v0
9.8 560 20 sin 1 (82)2 20 = sin–1(0.816) ...(ii) 1 0 (46.7º ) 23º 2 and = 90º – 0 = 90º – 23º = 67º The commandant of the fort can elevate the cannon to either of these two angles and (if only there were no intervening air!) hit the pirate ship. (b) We have seen that maximum range corresponds to an elevation angle 0 of 45º. Thus, from Eq. (i) with
www.ashoksirphysics.com
31
KINEMATICS 0 = 45º. v02 (82)2 sin 20 sin(2 45º ) g 9.8 R = 686 m 690 m As the pirate ship sails away, the two elevation angles at which the ship can be hit draw together, eventually merging at 0 = 45º when the ship is 690 m away. Beyond that distance the ship is safe. R
IMPORTANT FEATURES 1. As we have seen in the above derivations that ax = 0, i.e., motion of the projectile in horizontal direction is uniform. Hence, horizontal component of velocity u cos does not change during its motion. 2. Motion in vertical direction is first retarded then accelerated in opposite direction. Because uy is upwards and ay is downwards. Hence, vertical component of its velocity first decreases from O to A and then increases from A to B. This can be shown as in fig. y
uy O
3.
A
u
ux
B
x
The co-ordinates and velocity components of the projectile at time t are x = sx = uxt = (u cos ) t 1 1 y s y u y t a y t 2 u sin t gt 2 2 2 vx = ux = u cos and vy = uy + ayt = u sin – gt Therefore, speed of projectile at time ‘t’ is v v 2x v 2y and the angle made by its velocity vector with positive x-axis is
4.
vy tan 1 vx Equation of trajectory of projectile x = (u cos )t x t u cos Substituting this value of ‘t’ in, 1 y u sin t gt 2 , we get 2 gx 2 y x tan 2 2u cos 2 gx 2 y x tan 2 sec 2 2u gx 2 y x tan 2 1 tan 2 2u These are the standard equations of trajectory of a projectile. The equation is quadratic in x. This is why the path of a projectile is a parabola. The above equation can also
www.ashoksirphysics.com
32
KINEMATICS be written in terms of range (R) of projectile as x y x 1 tan R
5.
6.
Range R is given by
R
2 u cos u sin g
R
2 Horizontal component Vertical component g of initial velocity of initial velocity
There are two unique times at which the projectile is at the same height h(< H) and the sum of these two times 1 2 equals the time of flight T. Since, h u sin t gt is quadratic in time, so it has two unique roots ‘t1’ and 2 2u sin 2h ‘t2’ (say) such that sum of roots (t1 + t2) is and product (t1t2) is . The time lapse (t1 – t2) between g g these two events is (t1 – t2)2 = (t1 + t2)2 – 4t1 t2
7.
4u 2 sin 2 8h g g If K´ is the kinetic energy at the point of launch then kinetic energy at the highest point is
8.
1 1 mv 2x mu 2 cos 2 2 2 2 K´ = K cos For complementary angles and 90º – , if T and T90º – are the times of flight and R is the range, then
t1 t 2
K´
T T90º
e.g.,
T1º T89º
2R g
2R 90º g
2R g
2R1º 2R 89º g g
Example 33. A particle is projected in the X–Y plane. 2 sec after projection the velocity of the particle makes an angle 45º with the X-axis. 4 sec after projection, it moves horizontally. Find the velocity of projection. Sol. After 4 sec the particle reach at maximum hight. At maximum height it move horizontally. So that, T y =4 2 v0 2u sin 2u sin T8 Q T g g O x 80 u sin 40 ...(1) 2 u cos = v cos 45º v sin45 = 45 sin – 10 × 2
www.ashoksirphysics.com
33
KINEMATICS u sin 20 20 u cos u cos From eqn (1) and (2) u2 sin2 + u2 cos2 = 402 + 202 u2 = 1600 + 400 = 2000 1
ucos = 20
...(2)
u 20 100 10 20 20 5 m / s
PROJECTILE PROJECTED FROM SOME HEIGHT Projectile Projected in Horizontal Direction Let a projectile is projected with velocity u. Take observation point O at a height h from ground [Fig.]. y O
u r
x
x y(x, y)
a y = –g vx
–h vy P
v A
Here, ux = u, uy = 0 and ax = 0, ay = –g (a) Let at time ‘t’, the co-ordinates of position of projectile is (x, y), then 1 2 x = ut and y 0 gt 2 Therefore, at time ‘t’ position vector r x ˆi y ˆj 1 r (ut) ˆi gt 2 ˆj 2 2 2 2 2 1 2 | r | x y (ut) gt 2 y tan and x (b) Let at time ‘t’ the horizontal and vertical velocities of projectile be vx and vy, so vx = u and vy = 0 + (–g)t = – gt v v ˆi v ˆj u iˆ ( gt) ˆj x
and
y
v v 2x v 2y
v u 2 ( gt) 2 v tan x and vy (c) Let time taken by projectile from O to point A at ground is T, then
1 h 0 ( g)T 2 2
www.ashoksirphysics.com
34
KINEMATICS 2h g The horizontal distance in time T
T
2h g Therefore, the bomb dropped from an aeroplane moving with velocity u horizontally at height h, covers a PA uT u
horizontal distance u
2h on the ground. g
Projectile Projected Upwards at an Angle Let projectile is projected upward at angle with horizontal velocity u [Fig.].
u sin
u
vy = 0, vx = u x = u cos ay = –g A B u cos
O u cos –h
x
u sin
P
u D
C
ux = u cos and uy = u sin ax = 0 and ay = – g Now from 2nd equation of motion, 1 h u sin T g T 2 2 or gT2 – (2u sin )T – 2h = 0 Solving this equation, we get horizontal distance covered in time T PC = (u cos )T
2u sin g and horizontal distance covered in this time Time taken in covering path OAB
u 2 sin 2 OB g In such case for range PC to become maximum, ‘’ should be just less than 45º. NOTE : In such case on earth’s surface for maximum range, u sin α = . 2 2u + 2gh If h = 0, then α =
1 = 45º. 2
Projectile Projected Downward at an Angle Let projectile is projected downward at an angle with horizontal velocity u [Fig.].
www.ashoksirphysics.com
35
u y = –u sin
KINEMATICS y O
u x = u cos u
x a y = –g
–h P
A
ux = u cos and uy = –u sin ax = 0 and ay = –g From 2nd equation of motion, 1 g T 2 2 2 or gT + (2u sin )T – 2h = 0 To solve this euation, value of T can be evaluated. In this time the horizontal distance covered on the earth, PA = (u cos )T –h = –u sin T
Example 34. A projectile is fired horizontal with a velocity of 98 m/s from the top of a hill 490 m high. Find (a) the time taken by the projectile to reach the ground (b) the distance of the point where the particle hits the ground from foot of the hill and (c) the velocity with which the projectile hits the ground (g = 9.8 m/s2) Sol. Here, ux = 98 m/s, ax = 0, uy = 0 and ay = g (a) At A, sy = 490 m. So, applying 1 sy u y t a y t2 2 1 490 0 (9.8)t 2 2 t = 10 s 1 BA s x u x t a x t 2 (b) 2 or BA = (98) (10) + (0) or BA = 980 m (c) vx = ux = 98 m/s vy = uy + ayt = 0 + (9.8) (10) = 98 m/s
v v 2x v 2y (98)2 (98)2 98 2 m / s
and
tan
= 45º
vy vx
O
u = 98 m/s x y
B
A
vx
vy
98 1 98
Thus, the projectile hits the ground with a velocity 98 2 m / s at an angle of = 45º with horizontal. Example 35. A train starts from rest and moves with a constant acceleration of 2.0 m/s2 for half a minute. The brakes are then applied and the train comes to rest in one minute. Find
www.ashoksirphysics.com
36
KINEMATICS (a) the total distance moved by the train, (b) the maximum speed attained by the train and (c) the position(s) of the train at half the maximum speed. Sol. (a) S1 = The distance moved by the train in first half minute. 1 S1 = u1t1 + a1t12 2 1 1 S1 = 0 × t1 + × 2 × (30)2 { Q t1 = minute} 2 2 1 S1 = × 2 × 900 S1 = 900 m 2 The distance moved by the train after brakes applied. 1 S2 = u2t2 + a2t22 2 u2 = u1 + a1t1 u2 = 0 + 2 × 30 u2 = 60 m/s v2 = u2 – a2t2 0 = 60 – a2 × 60
a 2 = 1 m / sec2
v22 = u22 + 2 a2S2 0 = 60 × 60 – 2 × 1 × S2 S2 = 1800 m Total distance(s) = S1 + S2 S = (900 + 1800) m S = 2700 m
S = 2.7 km
(b) the maximum speed attained by the train is u 2 = 60 m / s (c) the position of the train at half of minimum speed. u22 = u12 + 2a1S’ (30)2 = 0 + 2 × 2 × S’ 900 = S' S' = 225 m 4
Example 36. A car is moving along a straight line. It is taken from rest to a velocity of 20 ms–1 by a constant acceleration of 5 ms–2. It maintains a constant velocity of 20 ms–2 for 5 seconds and then is brought to rest again by a constant acceleration of –2 ms–2. Draw a velocity-time graph and find the distance covered by the car. Sol. v22 = u2 + 2aS (20)2 = 0 + 2 × 5 × S 400 S= S = 40 m 10 Car maintains a constant velocity of 20 m/s for 5 Second. S’ = v × t S '' = 100 m S’’ = 20 × 5
www.ashoksirphysics.com
37
KINEMATICS The car comes to rest by a constant acceleration of –2 m/s2 (v’)2 = v2 + 2 × a’S’’ 0 = (20)2 – 2 × 2 S’’ 400 S '' = S'' = 100 m 4 The total distance covered by the car. = S + S’ + S’’ = 40 + 100 + 100 = 240 m Example 37. A ball rolls off the edge of a horizontal table top 4m high. If it strikes the floor at a point 5m horizontally away from the edge of the table, what was its speed at the instant it left the table ? Sol. Using h
or
1 2 gt we have, 2 1 h AB gt 2AC 2 2h AB t AC g
Further,
24 0.9 s 9.8 BC 5.0 v 5.55 m / s or t AC 0.9
BC = vtAC
v A 4m C
B 5m
Example 38. A ball is projected at an angle of 30º above with the horizontal from the top of a tower and strikes the ground in 5 sec at an angle of 45º with the horizontal. Find the height of the tower and the speed with which it was projected. Sol. ux = v0 cos30º uy = v0 sin30º Vx = uX = v0 cos30º y vy = u y + a y t v0 vy = v0 sin30 – gt 30º x v0 H v y 10 5 2 v0 ax=0, ay= –g – 50 vy 2 But – tan 45º v x v 0 cos 30 v v 0 cos 30 0 50 2 1 3 v0 50 2 2
v0 =
100 3 +1 100
=
3 –1
3 1
50
3 –1
3 –1
www.ashoksirphysics.com
38
KINEMATICS Also,
1 y = - H = u yt + a yt2 2 1 H v0 sin 30 5 10 5 2 2
H 125 2 – 3 m
Example 39. A ball is thrown horizontally from a cliff such that it strikes ground after 5 sec. The line of sight from the point of projection to the point of hitting makes an angle of 37º with the horizontal. What is the initial velocity of projection. Sol. vx = v0cos37º vy = v0sin37º y ax = gsin37º = 6 m/s2 v0 O 37º 2 ay = –gcos37º = –8 m/s From O to A, displacement along y-axis is zero. 37º y = uyt + ½ ayt 2 A 0 = uy × 5 + ½ × –8 × 25 { Q t = 5 sec.} 5uy = ½ × 8 × 25 100 uy = = 20 m / s 5 3 20 v0 5 100 v0 = m/s 3
37º
x
Example 40. A ball is projected from top of a tower with a velocity of 5 m/s at an angle of 53º to horizontal. Its speed when it is at a height of 0.45 m from the point of projection is : (A) 2 m/s (B) 3 m/s (C) 4 m/s (D) data insufficient Sol. According to conservation principle of machenic energy Ui + T i = Uf + T f 1 1 0 + mu 2 = mgh + mv 2 2 2 2 2 u = 2gh + v v = u 2 - 2gh
v 52 2 10 0.45 v 25 9
16
v 4 m/s u
Example 41. In the figure shown, the two projectiles are fired simultaneously. 20m/s What should be the initial speed of the left side projectile for the two 60º 45º projectile to hit in mid-air ? 10 m Sol. When two projectiles are projected from same height, then for collision, vertical component of velocities of both projectiles should be same
www.ashoksirphysics.com
39
KINEMATICS
u sin 60º = 20 sin45º 20sin 45º u= sin 60º 20 2 u 2 3
u = 20
2 m/s 3
Example 42. The speed of a particle when it is at its greatest height is 2 / 5 times of its speed when it is at its half the maximum height. the angle of projection is _____ and the velocity vector angle at half the maximum height is ______. Sol. uy = usin u u cos ux = ucos vx = ucos ...(1) 2 2 vy = uy +2ayS H v 2y u 2 sin 2 2g ...(2) 2 2 u cos v 2x v 2y 5 2 H u cos u 2 cos 2 u 2 sin 2 2g 5 2 u cos
2 5 2 u 5
u2 g
1
u 2 sin 2 2g
sin 2 2
2 sin 1 5 2 2 2 sin cos2 1 5 2 cos
2 sin 2 or , 5 5 or 5 5sin 2 sin 2 2 or 3 – 4 sin2 = 0 3 sin 60º 2 vy u 2 sin 2 gH tan and vx u cos cos 2
u 2 sin 2 2g u cos
1 sin 2
{from eqn. (1) and (2)}
sin 2 sin 2 tan cos
tan
u 2 sin 2 g tan
sin 2 2 tan cos
www.ashoksirphysics.com
sin 2 2 5 5
2
tan tan 60º 2 2 40
KINEMATICS
tan
3 2
tan 1
3 2
Example 43. A projectile is to be thrown horizontally from the top of a wall of height 1.7 m. Calculate the initial velocity of projection if its hits perpendicularly an incline of angle 37º which starts from the ground at the bottom of the wall. The line of greatest slope of incline lies in the plane of motion of projectile. Sol. ux = v0 uy = 0 ay = g = 10 m/s2 vx = v1 cos53º = 0.6 v1 vy = v1 sin53º = 0.8 v1 vx = ux = v0 or 0.6 v1 = v0 v 10v0 5v 0 v1 = 0 = = ...(1) v0 O x 0.6 6 3 1 2 vy = u y + a y t gt 2 C 0.8v1 = 10 t v1 h = 1.7 m A 37º 5v 0.8 0 10 t 3 53º 37º x 53º 6 60 grad B t= t v0 = ...(2) y v1 0.8 8 In BAC 1 1.7 - gt 2 1.7 - 5t 2 AB 3 2 tan 37º = = = AC 4 uxt v0 t 2 or 3v0t = 6.8 – 20t 8v 64 v02 3v 0 0 6.8 20 or 60 3600 2 72 64 2 24 2 64v 0 v0 + = 6.8 or v 0 6.8 180 60 180 6.8 180 v 20 9 v0 = 3 m / s or 136 Example 44. A hunter is riding an elephant of height 4m moving in straight line with uniform speed of 2 m/ sec. A dear running with a speed V in front at a distance of 4 5m moving perpendicular to the direction of motion of the elephant. If hunter can throw his spear with a speed of 10 m/sec, relative to the elephant, then at what angle to it’s direction of motion must he thrown his spear horizontally for a successful hit. Find also the speed ‘V’ of the dear. 1 2 Sol. h = gt 2 2h 24 8 t g 10 10
www.ashoksirphysics.com
41
KINEMATICS Assume horizontal plane at x–y–plane. r v rel 10 cos ˆi 10sin ˆj r r v rel u 2 ˆi r r u v rel 2 ˆi 10 cos 2 iˆ 10 sin ˆj The deer is moving along y–axis. So, displacement of deer and displacement of spear along y–axis will be same in time t. v t = u yt v = 10 sin ...(1) Also, along x–axis :
ux t = 4 5 8 4 5 10 10 4 5 10 10cos 2 4 5 or 2 8 10 cos = 8 8 4 cos = 37º 10 5 From eqn (1) or
10cos 2
v = 10 sin = 10 sin37º = 10
3 = 5
4m 2m/s x
6 m/s
y A Example 45. An object A is kept fixed at the point x = 3 m and y = 1.25 m on a P 1.25m plank P raised above the ground. At time t = 0 the plank starts moving along the + x direction with an acceleration 1.5 m/s2. At the same instant a stone is u x O 3.0m projected from the origin with a velocity u as shown. A stationary person on the ground observes the stone hitting the object during its downward motion at an angle of 45º to the horizontal. All the motions are in x – y plane. Find u and the time after which the stone hits the object. Take g = 10 m/s2. Sol. ux = u cos uy = u sin ax = 0 u u =ucos 45º ay = –g 1.25 If the stone hitt the object after time t. So that virtical displacement of stone is 1.25 m u cos 1 2 y = uy t + a yt 2 1 2 therefore 1.25 (u sin )t gt 2 1 1.25 (u sin )t 10 t 2 2 1.25 = (u sin) t – 5 t 2 (u sin)t = 1.25 + 5t 2 ...(1) Hotizontal displacement of stone is x = 3 + displacement of object A. Initial velocity of object is zero.
u sin
x
www.ashoksirphysics.com
42
KINEMATICS so displacement of object is 1 1 1.25 at 2 1.5 t 2 0.75 t 2 2 2 x = 3 + 0.75 t 2 (u cos)t = 3 + 0.75 t 2 Since velocity vector inclined at 45º with horizontals. u y u sin gt tan(–45º ) ux u cos u cos = – (u sin – gt) u cos = gt – u sin (u cos) t + (u sin) t = 10 t2 Add eqn (1) and (2) (u cos) t + (u sin) t = 4.25 + 5.75 t2 from eqn (3) and (4) 10 t2 = 4.25 + 5 .27 t2 4.25 t2 = 4.25 t2 = 1 From eqn (1) and (2) uy = u sin = 6.25 m/s uy = 6.25 m/s ux = 3.75 m/s
...(2)
...(3)
...(4)
t = 1 sec.
u = u 2x + u 2y u = (6.25) 2 + (3.75) 2
u = 7.29 m / s
IMPORTANT FEATURES Projectile motion is a two dimensional motion with constant acceleration (g). So, we can use v uat 1 a u t a t2 2 etc. in projectile motion as well. Here, u u cos ˆi u sin ˆj and a g ˆj y
Now, suppose we want to find velocity at time ‘t’. v u a t v u cos ˆi u sin ˆj gt ˆj or v u cos ˆi u sin gt ˆj Similarly, displacement at time ‘t’ will be, 1 S u t a t2 2 1 S u cos iˆ u sin ˆj t gt 2 ˆj 2
www.ashoksirphysics.com
u
O
g
x
43
KINEMATICS 1 S u cos iˆ ut sin gt 2 ˆj 2
PROJECTILE MOTION ON AN INCLINED PLANE One an inclined plane projectile is projected into two cases, one upwards and the other downwards. Up the Plane A projectile is projected up the inclined plane from the point O with an initial velocity u at an angle with horizontal. The angle of inclination of the plane with horizontal is [Fig.]. x A
u
9 o s( gc
y
y
90º
O x´
B y´
x´
0 º–
)
s =g
in
x
g sin(90º– ) = g cos
–
O y´
ux = u cos ( – ) and ax = – g sin uy = u sin ( – ) and ay = – g cos (i) Time of flight : During motion from point O to A, the displacement along y-axis is zero. sy = 0 at t = T
or
1 sy u y t a y t2 2 1 0 u sin T g cos T 2 2
T
2u sin g cos
NOTE : Substituting = 0, in the above expression, we get T =
2u sin α . g
Which is quite obvious because = 0 is the situation shown in Fig. y u
O
g
x
(ii) Range : As shown in Fig, OA is the range of proectile. Horizontal component of initial velocity uH = u cos OB = uHT (as aH = 0) =
u cos θ 2u sin 2u 2 sin cos g cos
www.ashoksirphysics.com
g cos
44
KINEMATICS 2u 2 sin cos OB R OA cos g cos 2 Using, 2 sin A cos B = sin (A + B) + sin (A – B) Range can also be written as, R
u2 sin 2 sin g cos 2
This range will be maximum when u2 R 2 1 sin or and max g cos 2 2 4 2
we see that for = 0, range will be maximum for R max
u2 1 sin 0º g cos 2 0º
R max
u2 g
or 45º. 4
Alternative method : For range, sx = R, t = T 1 sx u x t a x t 2 2 1 R u cos T g sin T 2 2 2u sin Substituting the value of T , in above equation for R. g cos
or
R
u2 sin 2 sin g cos 2
Down the Plane A projectile is projected down the plane from the point O with an initial velocity u at an angle with horizontal [Fig.]. The angle of inclination of plane with horizontal is . y
x
u O
( 90 os c g
90
º– º–
g )=
sin
g sin(90º– ) = g cos
A
ux = u cos ( + ), ax = g sin uy = u sin ( + ), ay = –g cos Proceeding in the similar manner, we get the following results : Therefore,
T
2u sin g cos
www.ashoksirphysics.com
45
KINEMATICS R
u2 sin 2 sin g cos 2
IMPORTANT FEATURES If two particles are projected at angles 1 and 2 respectively as shown in fig., then the relative motion of 1 with respect to 2 is a straight line at an angle. y
y
u2 u1
1
x
2
x
u12y tan 1 with positive x-axis. u12x where
u12x = u1x – u2x = u1 cos 1 – u2 cos 2 u12y = u1y – u2y = u1 sin 1 – u2 sin 2
Example 46. A particle is projected with a velocity of 20 m/s at an angle of 30º to an inclined plane of inclination 30º to the horizontal. The particle hits the inclined plane at an angle of 30º, during its journey. Find the (a) time of impact, (b) the height of the point of impact from the horizontal plane passing through the point of projection. Sol. The particle hits the plane at 30º (the angle of inclination of plane). It means particle hits the plane horizontally. (a)
t
u
T u sin 2 g
20sin 30º 30º 1.76 s 9.8 u 2 sin 2 H 2g t
(b)
30º 30º
2 20 sin 2 60º H 15.3 m
2 9.8
Example 47. A particle is projected up an inclined plane with initial speed v = 20 m/s at an angle = 30º with plane. Find the component of its velocity perpendicular to plane when it strikes the plane. Sol. Component of velocity perpendicular to plane remains the same (in opposite direction) i.e., u sin = 20 sin 30º = 10 m/s Example 48. A particle is thrown horizontally with relative velocity 10 m/s from an inclined plane, which is also moving with acceleration 10 m/s2 vertically upward. Find the time after which it lands on the plane (g = 10 m/s2). Sol.
10m/s2 30º
ux = 10 cos30º = 5 3 m/s uy = 10 sin30º = 5 m/s arel =20 m/s2
www.ashoksirphysics.com
46
y 10sin30º 10 m/s2 v0= 10m/s
KINEMATICS ax = arel sin30º = 10 m/s ay = – arel cos30º
3 10 3 m / s 2 2 when ball lands on inclined plane, y = 0 1 y = uyt + ay t 2 2 1 0 5 t 10 3 t 2 2 5 3 t =5 1 t= sec. 3 20
O
30º
2
A
x
0º o s3 c 10
30º
Example 49. A particle is projected from point P with velocity 5 2 m/s perpendicular to the surface of a hollow right angle cone whose axis is vertical. It collides at Q normally. Find the time of the flight of the particle. u0x = 5 2m / s u0y = 0 ax = –g sin45º ay = –g cos45º At point Q, vx = 0 { Q the particle collied normally at point Q} The time taken by particle to go from P to Q is t0. vx = u x + a x t 0 = 5 2 – g sin45º t 0
P
Q 45º
y
x
x P
Q 45º
Sol.
y
g 45º
45º
5 2 5 2 2 1 sec. g sin 45º 10 t 0 = 1 sec.
t0
Example 50. A ball is projected on smoot inclined plane in direction perpendicular to line of greatest slope with velocity of 8m/s. Find it’s speed after 1 sec. Sol. vy = uy + ayt vy = 0 + 10 sin37º × 1 3 vy = 10 1 7º 5 in3 gs vy = 6 m/s 37º vx = ux = 8 m/s x y
8 m/s
º 37
v = v 2x + v 2y = 82 + 6 2 Ans. 10 m / s
Example 51. Two inclined planes OA and OB having inclination (with horizontal) 30º and 60º respectively, intersect each other at O as shown in fig. A particle is projected from point P with velocity u = 10 3 ms - 1
www.ashoksirphysics.com
47
B
u
KINEMATICS
A
along a direction perpendicular to plane OA. If the particle strikes plane OB perpendicularly at Q, calculate (a) time of flight, (b) velocity with which particle strikes the plane OB, (c) vertical height h of P from O, (d) maximum height from O attained by the particle. Sol. (a) u x = 10 3 m / s
Q P
h 60º
30º O
uy = 0 ax = –g sin60º
B
u A
ay = –g cos60º
Q P
At point Q
h
vx = 0 The time taken by particle to go from P to Q is to vx = ux + axt
60º
30º O
0 = 10 3 - g sin 60º t 0 t0 = (b)
(c)
(d)
10 3 g sin 60º
t 0 = 2sec
vy = uy – ayt 0 vy = 0 – g cos60º × 2 1 v y 10 2 10 m / sec 2 h sin 30º = x 1 h = x = 2h m 2 x 1 –2h = u y t + a y t 2 2 1 –2h 0 g sin 30º (2) 2 2 1 1 2h 10 4 2 2 At maximum height vy = 0 v 2y = u 2y - 2gH 2
0 = (u cos30º) – 2gH 2
H=
h
x 60º
30º O
h= 5m uy = u cos30º X 30º Y h 60º
2
u cos 30º 2g
3 10 3 2 H 2g 100 3 3 4 H 2 10
X
u
Y
30º 2
2
www.ashoksirphysics.com
48
KINEMATICS 25 9 45 H= = 20 4 Maximum height from O attained by the particle = H+h = 11.25 + 5 Ans. 16.25 m
11.25 m
Example 52. A large heavy box is sliding without friction down a smooth plane of inclination . From a point P on the bottom of a box, a particle is projected. inside the box. The initial speed of the particle with respect to box is u and the direction of projection makes P an angle with the bottom as shown in figure. (a) Find PQ if particle lands on Q. (b) If horizontal displacement of particle with respect to ground is zero. Find the velocity of box. Sol. ux = u cos uy = usin ax = –g sin ay = –g cos When the particle hitt the inclined plane then displacement in y-direction is zero. y x u =usin 1 y = u yt + a yt2 Q 2 s o uc s 1 P u = –gco ax = –gsin 0 (u sin )t g cos t 2 – = 2 90 ay 1 g (u sin )t g cos t 2 2
Q
y
x
2u sin ...(1) g cos with respect to box ux = u cos ax = 0 1 x = uxt + axt2 2 x = u cost (2u sin ) x (u cos ) (from eqn 1) g cos u 2 sin 2 x g cos According to question ux = –U ax = –gsin 1 x = uxt + axt2 2 2 2 2u sin 1 2u sin u sin 2 U g sin g cos g cos 2 g cos t
www.ashoksirphysics.com
49
KINEMATICS u sin cos cos u sin cos u cos U cos u sin cos U u cos cos cos cos cos sin U u cos u cos( ) U cos
u cos U
THINKING PROBLEMS 1.
Can you use the equations of kinematics to find the height attained by a body projected upwards with any velocity ?
2.
Can the relative velocity of two bodies be greater than the absolute velocity of either ?
3.
A boy sitting in a car moving with a constant velocity throws a ball straight up into the air. Will the ball fall behind him, in front of him or into his hand? What would happen if the car accelerated forward or went round a curve while the ball was in the air ?
4.
v f + vi A student argues that the mean velocity during an interval of time can also be expressed as ávñ= 2 r f - ri and this should always be equal to áv f ñ= . Is he right . t 2 - t1
5.
Consider a collection of a large number of particles moving with the same speed v in random directions. Could you, by using simple logic, show that the magnitude of the relative velocity of a pair of particles averaged over all the pairs of the collection is greater than v ?
6.
The barrel of a gun and a target lie along the same horizontal. If the target is released and the gun is fired at the same time, the bullet will always hit the target whatever be the distance between the gun and the target. Is this true or false ?
7.
A black dot is made at the tip of an aerofoil of an aeroplane. What is the trajectory of the black dot as it appears to the pilot and to an observer on the ground?
8.
A body is dropped fron the window of a train. W ill the time of the free fall be equal if the train is stationary, moves with constant velocity, moves with constant acceleration?
9.
We can order events in time, such as past, present and future. Hence there is a sense of time. So is time a vector? If not, why not?
10. Average speed can mean the magnitude of the average velocity vector. Another meaning given to it is that the average speed is the total length of the path traversed divided by the elapsed time. Are these meanings different? if so, give an example. 11. Can a body have zero velocity and still be accelerating? can a body have a constant speed and still have a varying velocity? Can a body have a constant velocity and still have a varying speed? 12. Can an object have an eastward velocity while experiencing westward acceleration?
www.ashoksirphysics.com
50
KINEMATICS THINKING PROBLEMS SOLUTION 1. No, because the equations are applicable only so long as the acceleration is uniform. The acceleration due to gravity is uniform only near the surface of the earth. 2. Yes, e.g., when two bodies move in opposite directions, the relative velocity of each is greater than the individual velocity of either. 3. It will fall into his hand because of the ‘physical independence of vectors’ or the fact that the forward velocity of the ball in the air is the same as that of the car. But if the car accelerated, the ball would fall behind him. If the car went round a curve the ball would fall in front of him because it would move along the tangent to the curved path through a certain distance while the car moved along the curved path through the same distance. 4. No, he is not right. The correct definition of average velocity is the latter one. the first one can be used only when there is uniform acceleration. 5.
v rel =
v 2 + v 2 - 2v 2 cos q = 2v sin q / 2. In other words between 0 and 60º the relative velocity is less
than v, while between 60º and 180º it is greater than v. Hence the relative velocity averaged over all directions
v rel > v. 6. True, provided the target is within the range of the bullet, because the downward acceleration of both is the same. 7.
A circular path relative to the pilot and a helical path relative to the observer fixed to the earth.
8.
Same in all the three cases due to the physical independence of vectors.
9.
Time is not a vector though it may have a sense because in order to be a vector it must add and subtract by vector algebra rules and it does not.
10. The meanings are different. The point can be made clear by considering the motion of a particle along a circle. Let a particle describe half a circle of radius R in time .
r –R iˆ – R iˆ 2R ˆ – i = average velocity vector v r 2R R | v | v = average speed r Obviously, v | v | , that is, average speed as the modulus of the average velocity vector is not the same as the average speed defined as the total length of the path traversed divided by time elapsed. 11. Yes, for example a particle in SHM at the extreme position has zero velocity but its acceleration is maximum. Yes, a particle in circular motion has constant speed but varying velocity. No, a body cannot have a constant velocity while having a varying speed. 12. Yes, a body can have eastward velocity and westward acceleration, e.g., SHM.
www.ashoksirphysics.com
51
KINEMATICS
ASSERTION & REASON it of (A) (B) (C) (D) (E) 1.
THE NEXT QUESTIONS REFER TO THE FOLLOWING INSTRUCTIONS A statement of assertion (A) is given and a Corresponding statement of reason (R) is given just below the statements, mark the correct answer as – If both A and R are true and R is the correct explanation of A. If both A and R are true but R is not the correct explanation of A. If A is true but R is false. If both A and R are false. If A is false but R is true.
Assertion (A) : Reason (R) :
2.
Assertion (A) :
Horizontal component of the velocity of angular projectile remains unchange during its flight. At highest point the velocity reduces to zero. (A) (B) (C) (D) (E) Suppose a particle starts moving in a straight line with initial velocity +u and an acceleration –a, then velocity at displacement s comes out to be, v 2 u 2 2as . If we draw a graph between v 2 and s, it will be a straight line as shown in figure. 2
v
S0
Reason (R) :
S
v 2 versus s graph is a straight line passing through origin with positive intercept and negative slope. (A)
(B)
(C)
(D)
(E)
3.
Assertion (A) : Reason (R) :
A body can have acceleration even if its velocity is zero at a given instant of time. A body is momentarily at rest when it reverses its direction of motion. (A) (B) (C) (D) (E)
4.
Assertion (A) :
When a body is projected with an angle 45°, its range is maximum.
Reason (R) :
For maximisation of range sin 2 should be equal to one. (A) (B) (C) (D)
(E)
5.
Assertion (A) : Reason (R) :
Rocket in flight is not an illustration of projectile. Rocket takes flight due to combustion of fuel and does not move under the gravity effect alone. (A) (B) (C) (D) (E)
6.
Assertion (A) :
The slope of displacement-time graph of a body moving with high velocity is steeper than the slope of displacement-time graph of a body with low velocity. Slope of displacement-time graph = Velocity of the body. (A) (B) (C) (D) (E)
Reason (R) :
7.
Assertion (A) : Reason (R) :
Displacement of a body may be zero when distance travelled by it is not zero. The displacement is the longest distance between initial and final position. (A) (B) (C) (D) (E)
www.ashoksirphysics.com
52
KINEMATICS 8.
Assertion (A) : Reason (R) :
9.
The relative velocity between any two bodies moving in opposite direction is equal to sum of the velocities of two bodies. Sometimes relative velocity between two bodies is equal to difference in velocities of the two. (A) (B) (C) (D) (E)
Assertion (A) : Reason (R) :
The displacement-time graph of a body moving with uniform acceleration is a straight line. The displacement is proportional to time for uniformly accelerated motion. (A) (B) (C) (D) (E)
10. Assertion (A) : Reason (R) :
A body falling freely may do so with constant velocity. The body falls freely, when acceleration of a body is equal to acceleration due to gravity. (A) (B) (C) (D) (E)
11. Assertion (A) : Reason (R) :
The speedometer of an automobile measure the average speed of the automobile. Average velocity is equal to total displacement per total time taken. (A) (B) (C) (D) (E)
www.ashoksirphysics.com
53
KINEMATICS
Level # 1 1.
A train starting from rest travels the first part of its journey with constant acceleration a, second part with constant velocity v and third part with constant retardation a, being brought to rest. The average speed for 7v the whole journey is . The train travels with constant velocity for .... of the total time 8 (A)
2.
3 4
(B)
7 8
(C)
5 6
(D)
9 7
A particle moving in a straight line with uniform acceleration is observed to be at a distance a from a fixed point initially. It is at distances b, c, d from the same point after n, 2n, 3n second. The acceleration of the particle is (A)
c 2b a n2
(B)
cba 9 n2
(C)
c 2b a 4 n2
(D)
c ba n2
3.
If a, b and c be the distances travelled by the body during xth, yth and zth second from start, then which of the following relations is true ? (A) a(y – z) + b(z – x) + c(x – y) = 0 (B) a(x – y) + b(y – z) + c(z – x) = 0 (C) a(z – x) + b(x – y) + c(y – z) = 0 (D) ax + by + cz = 0
4.
A body of mass 3 kg falls from the multi-storeyed building 100 m high and buries itself 2m deep in the sand. The time of penetration will be (A) 0.09 s (B) 0.9 s (C) 9 s (D) 10 s
5.
Two cars A and B, each having a speed of 30 km/hr are heading towards each other along a straight path. A bird that can fly at 60 km/hr flies off car A when the distance between the cars is 60 km, heads directly towards car B, on reaching B, the bird directly flies back to A and so forth, then the total distance the bird travels till the cars meet is. (A) infinite (B) 30 km (C) 60 km (D) 120 km
6.
Two cars A and B, each having a speed of 30 km/hr are heading towards each other along a straight path. A bird that can fly at 60 km/hr flies off car A when the distance between the cars is 60 km, heads directly towards car B, on reaching B, the bird directly flies back to A and so forth, the total no. of trips which the bird makes till the cars meet is (A) Four (B) Eight (C) Sixteen (D) Infinite
7.
Three particles start from the origin at the same time, one with a velocity v 1 along x-axis the second along the y-axis with a velocity v 2 and the third along x = y line. The velocity of the third so that the three may always lie on the same line. (A)
8.
v1 v 2 v1 v 2
(B)
2 v1 v 2 v1 v 2
(C)
3 v1 v 2 v1 v 2
(D) Zero
Choose the correct statement (A) A body starts from rest and moving with constant acceleration travels a distance y 1 and the 3rd second y1 5 and y2 in 5th second. The ratio y 9 . 2 (B) A ball falls from the top of a tower in 8 second in 4 second, it till cover the first quarter of the distance starting from top. (C) The distance traveled by a freely falling stone released. With zero velocity in the last second of its motion to that traveled by it in the last second of its motion to that traveled by it in the last but one second is 7 : 5. The stone strike the ground with velocity 39.2 m/s. (D) None of these
www.ashoksirphysics.com
54
KINEMATICS 9.
A steam boat goes across a lake and comes back (i) on a quiet day when the water is still, and (ii) on a rough day when there is a uniform current so as to help the journey onward and to impede the journey back. If the speed of the launch, on both days, was same, the time required for the complete journey on the rough day, as compared to that on the quiet day, will be. (A) Less (B) Same (C) More (D) Cannot be predicted
10.
A car is moving along a straight road with a uniform acceleration. It passes through two points P and Q separated by a distance with velocity 30 km/hr and 40 km/hr respectively. The velocity of the car midway between P and Q is (A) 33.3 km/hr
(B) 20 3 km/hr
(C) 25 2 km/hr
(D) 35 km/hr
11.
Starting from rest a particle moves in a straight line with acceleration a = (25 – t 2)1/2 m/s2 for 0 t 5 s, 3 a= m/s2 for t > 5 s. The velocity of particle at t = 7 s is. 8 (A) 11 m/s (B) 22 m/s (C) 33 m/s (D) 44 m/s
12.
A body is released from a great height and falls freely towards the earth. Exactly one sec later another body is released. What is the distance between the two bodies 2 sec after the release of the second body. (A) 4.9 m (B) 9.8 m (C) 24.5 m (D) 50 m
13.
The distance moved by a freely falling body (starting from rest) during 1st, 2nd, 3rd, .... nth s of its motion are proportional to. (A) Even numbers (B) Odd numbers (C) All integral numbers (D) Square of integral numbers
14.
A ball is thrown vertically upwards with a speed of 10 m/s from the top of a tower 200 m high and another is thrown vertically downwards with the same speed simultaneously. the time difference between them in reaching the ground in s(g = 10 m/s 2) is (A) 12 (B) 6 (C) 2 (D) 1
15.
Between two stations a train first accelerates uniformly, then moves with uniform speed and finally, retards uniformly. If the ratios of the time taken for acceleration, uniform speed and retarded motions are 1 : 8 : 1 and the maximum speed of the train is 60 km/h, the average speed of the train over the whole journey is. (A) 25 km/h (B) 54 km/h (C) 40 km/h (D) 50 km/h
16.
A ball is thrown vertically upwards. It was observed at a height h twice with a time interval t. The initial velocity of the ball is. (A)
2
8 gh g ( t )
2
(B)
g t 8 gh 2
2
(C)
1 2 2 2 8 gh g ( t )
(D)
8 gh 4g2 ( t )2
17.
A target is made of two plates, one of wood and the other of iron. The thickness of the wooden plate is 4 cm and that of iron plate is 2 cm. A bullet fired goes through the wood first and then penetrates 1 cm into iron. a similar bullet fired with the same velocity from opposite direction goes through iron first and then penetrates 2 cm into wood. If a1 and a2 be the retardation offered to the bullet by wood and iron plates respectively then (A) a1 = 2a2 (B) a2 = 2a1 (C) a1 = a2 (D) Data insufficient
18.
The motion of a body falling from rest in a resisting medium is described by the equation a and b are constants. The velocity at any time t is a b (A) v t = b (1 – e–bt) (B) v t = e–bt a
19.
a (C) v t = b (1 + e–bt)
(D) v t =
dv = a – bv where dt
b bt e a
P . To o Mv increase the velocity of the vehicle from v 1 to v 2, the distance travelled by it (assuming no friction) is
A self-propelled vehicle of mass M whose engine delivers constant power P has an acceleration a =
(A) s =
3P 2 (v 2 v 12 ) M
(B) s =
M 2 ( v 2 v 12 ) 3P
www.ashoksirphysics.com
(C) s =
M 3 ( v 2 v 13 ) 3P
(D) s =
3P 3 ( v 2 v 13 ) M
55
KINEMATICS For an airplane to take-off it accelerates according to the graph shown and takes 12 s to take-off from the rest position. The distance travelled by the airplane is. (A) 21 m (B) 210 m (C) 2100 m (D) 120 m
A
5
B
m/s2
20.
6
t (in s)
12
21. A river is flowing from west to east at a speed of 5 meters per minute. A man on the south bank of the river, capable of swimming at 10 meters per minute in still water, wants to swim across the river in the shortest time. He should swim in a direction. (A) due north (B) 30째 east or north (C) 30째 west of north (D) 60째 east of north 22. A boat which has a speed of 5 km/hr in still water crosses a river of width 1 km along the shortest possible path in 15 minutes. The velocity of the river water in km/hr is A (A) 1
(B) 3
(C) 4
(D)
41
23. In 1, 0, s, a particle goes from point A to point B, moving in a semicircle of radius 1.0 m (see Figure). The magnitude of the average velocity (A) 3.14 m/s (B) 2.0 m/s (C) 1.0 m/s (D) Zero
1.0 m
B
24. A ball is dropped vertically from a height d above the ground. It hits the ground and bounces up vertically to a height
d 2 . Neglecting subsequent motion and air resistance, its velocity v varies
with the height h above the ground as v
v d
(A)
h
(B)
v
d
v d
h
d
h
(C)
(D)
h
Acceleration 2 (m/s ) 10
25. A body starts from rest at time t = 0, the acceleration time graph is shown in the figure. The maximum velocity attained by the body will be (A) 110 m/s (B) 55 m/s (C) 650 m/s (D) 550 m/s
Time 11 (Sec.)
26. The velocity is displacement graph of a particle moving along a straight line is shown v v0
x0 The most suitable acceleration-displacement graph will be
a
a
a
x
(A)
x
a x
(B)
(C)
x
(D)
x
www.ashoksirphysics.com
56
KINEMATICS
Multiple Choice Question 27. A particle is moving eastwards with a velocity of 5 m/s. In 10s the velocity changes to 5 m/s northwards. The average acceleration in this time is (A) Zero (C) 1
(B) 1
2 m s 2 towards north-east
(D)
2 m s 2 towards north-west
1 m s 2 towards north-west 2
28. A particle of mass m moves on the x-axis as follows : it starts from rest at t = 0 from the point x = 0, and comes to rest at t = 1 at the point x = 1. NO other information is available about its motion at intermediate times (0 < t < 1). If denotes the instantaneous acceleration of the particle, then: (A)
(B)
cannot exceed 2 at any point in its path.
cannot remain positive for all t in the interval
0 t 1.
must be 4 at some point or points in its path. (D) must change sign during the motion, but no other assertion can be made with the information given. (C)
29. The coordinates of a particle moving in a plane are given by x(t) = a cos(pt) and y(t) = b sin(pt) where a, b(<a) and p are positive constants of appropriate dimensions. Then (A) the path of the particle is an ellipse (B) the velocity and acceleration of the particle are normal to each other at t
2 p .
(C) the acceleration of the particle is always directed towards a focus (D) the distance travelled by the particle in time interval t = 0 to t
2 p is a
30. At t = 0, an arrow is fired vertically upwards with a speed of 98 ms–1 A second arrow is fired vertically upwards with the same speed at t = 5s. Then (A) The two arrows will be at the same height above the ground at t = 12.5 s. (B) The two arrows will reach back their starting points at t = 20 s and at t = 25 s. (C) The ratio of the speeds of the first and the second arrows at t = 20 s will be 2 : 1. (D) The maximum height attained by either arrow will be 980 m. 31. A projectile is fired with a constant speed at two different angles of projection, say, same range. Then,
(A) cosec = sec
and
and
that give it the
are such that
(B) tan ( + ) (C) sin2 – cos2 = sin2 – cos2
(D) cot = cos sec
Fill in the blanks 1.
A particle moves in a circle of radius R. In half the period of revolution its displacement is _________ and distance covered is ___________.
2.
Four persons K, L, M, N are initially at the four corners of a square of side d. Each person now moves with a uniform speed v in such a way that K always moves directly towards L, L directly towards M, M directly towards N, and N directly towards K. The four persons will meet at a time __________.
3.
Spotlight S rotates in a horizontal plane with constant angular velocity of 0.1 radian/second. The spot of light P moves along the wall at a distance of 3 m. The velocity of the spot P when 45 (see figure) is __________ ms/. S
4.
2
The trajectory of a projectile in a vertical plane is y = ax – bx , where a, b are constants, and x and y are respectively the horizontal and vertical distances of the projectile from the point of projection. The maximum height attained is ________ and the angle of projection from the horizontal is ________.
www.ashoksirphysics.com
3m P
57
KINEMATICS
True / False 5.
Two balls of different masses are thrown vertically upwards with the same speed. They pass through the point of projection in their downward motion with the same speed (Neglect air resistance).
6.
A projectile fired from the ground follows a parabolic path. The speed of the projectile is minimum at the top of its path.
7.
Two identical trains are moving on rails along the equator on the earth in opposite directions with the same speed. They will exert the same pressure on the rails.
8.
An electric line of forces in the x-y plane is given by the equation x 2 + y2 = 1. A particle with unit positive charge, initially at rest at the point x = 1, y = 0 in the x-y plane, will move along the circular line of force.
Table Match 9.
Match List I and List II and select the correct answer using the codes given below in the lists: Column-I Column-II
I. Deceleration decreasing
A.
time
II. Deceleration increasing
B.
time velocity
III.Acceleration decreasing
C. time velocity
IV.Uniform acceleration
D. time velocity
E. time (A) I—D, II—E, III—C, IV—A (C) I—C, II—E, III—B, IV—A
www.ashoksirphysics.com
(B) I—E, II—B, III—C, IV—D (D) I—D, II—B, III—A, IV—C
58
KINEMATICS
Passage Type Questions THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE When an airplane flies, its total velocity with respect to the ground is –
vtotal v plane vwind
Where v plane , denotes the plane’s velocity through motionless air, and vwind denotes the wind’s velocity.. Crucially, all the quantities in this equation are vectors. The magnitude of a velocity vector is often called the “speed”. Consider an airplane whose speed through motionless air is 100 m/s. To reach its destination, the plane must fly east. The “heading” of a plane is the direction in which the nose of the plane points. So, it is the direction in which the engines propel the plane. 1.
If the plane has an eastward heading, and a 20 m/s wind blows towards the southwest, then the plane’s speed is – (A) 80 m/s (B) more than 80 m/s but less than 100 m/s (C) 100 m/s (D) more than 100 m/s
2.
The pilot maintains an eastward heading while a 20 m/s wind blows northward. The plane’s velocity is deflected from due east by what angle? (A) sin 1
3.
1 5
(B) cos 1
1 5
(D) None of these
(B) 100 m s cos
(C)
100 m s
(D)
sin
100 m s cos
Because the 20 m/s northward wind persists, the pilot adjusts the heading so that the plane’s total velocity is eastward. By what angle does the new heading differ from due east? (A) sin
5.
(C) tan 1
Let denote the answer to previous question. The plane in question 2 has what speed with respect to the ground? (A) 100 m s sin
4.
1 5
1
1 5
(B) cos
1
1 5
(C) tan
1
1 5
(D) None of these
Let denote the answer to previous question. What is the total speed, with respect to the ground, of the plane in previous question? (A) 100 m s sin
(B) 100 m s cos
(C)
100 m s
(D)
sin
100 m s cos
THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE During a car crash, the more rapidly a person decelerates, the more likely she is to be injured. A large deceleration is dangerous, even if it lasts for a short time. Airbags are designed to decrease the magnitude of the deceleration. Before the airbag inflates, the driver continues forward at constant speed. But once the airbag inflates, the driver decelerates gradually, instead of getting thrown into the windshield or steering wheel. 20 v (m/s)
Airbag 1
.01 .02 .03 .04 .05 t(s)
www.ashoksirphysics.com
20 v (m/s)
Airbag 2
.01 .02 .03 .04 .05 t(s)
59
KINEMATICS Airbag 3
20 v (m/s)
.01 .02 .03 .04 .05 t(s) Three different models of airbags were tested using identical crash test dummies. Sensors measured the velocity of the crash test dummy as a function of time, when the car crashed at 20 m/s into a brick wall. The shown velocity vs, time graphs resulted. Time t = 0 is the moment the car crashes. 6.
All three airbags are the same size and shape. Which one inflates most quickly? (A) Airbag 1 (B) Airbag 2 (C) Airbag 3 (D) We cannot determine the answer from the given information.
7.
Let amax denote the largest instantaneous acceleration that the crash test dummy experiences during the crash. The best airbag is the one for which amax is as small as possible. Which airbag is best? (A) Airbag 1 (C) Airbag 3
For airbag 2, which of the following graphs best represents the position of the crash test dummy as a function of time? Let x = 0 m be the dummy’s position at time t = 0s.
.01 (A) A 9.
time(s)
time(s)
.01
(B) B
D
(C) C
Position
C
Position
B
Position
A
Position
8.
(B) Airbag 2 (D) We cannot determine the answer from the given information.
.01
time(s)
.01 time(s)
(D) D
For airbag 2, approximately how much distance does the dummy cover between the moment the car crashes and the moment the dummy first makes contact with the airbag? (A) 0.2 m (B) 0.4 m (C) 0.6 m (D) 1.0 m THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE Recently, college teams from all over the country sent tennis players to participate in a series of experiments conducted by the Physical Education Department of a major university. A variety of coaching methods was used to improve the players’ serves, described below. Experiment 1 Two groups of 50 tennis players worked on the speed of their basic serves for two weeks. one group consisted solely of right-handed players; the other consisted solely of left-handed players. Half of each group watched videos of a right-handed tennis coach. Each player was told to pattern his or her serve on that of the coach in the video. The players received no verbal or physical guidance. the average speed of each player’s serve was measured at the beginning and end of the two-week period, and changes were recorded in Table 1.
Table 1 Players' handedness Right Right Left Left
Coach's handedness Right Left Right Left
Average change in speed (mph) 5 2 -1 8
Experiment 2 For two weeks, a second group of 100 right-handed tennis players watched the same videos of the righthanded tennis coach. The coach also physically guided 50 of the those players through the motions of the serve. Again, no verbal instruction was given during the experiment. The average speed and accuracy of each player’s serves were recorded at the beginning and end of this two-week period. The results are recorded in Table - 2.
www.ashoksirphysics.com
60
KINEMATICS
Table 2 Guided No Yes
Average Change in speed (mph) 5 9
Average change in Accuracy 15% 25%
Experiment 3 For two weeks, a third group of 100 right-handed tennis players worked on their basis serves. 50 players received no verbal instruction; they watched the same video of the right handed tennis coach, who also physically guided them through the motions of the serve. The other 50 players did not observe the video but received verbal instruction from the coach, who then physically guided them thought the motions of the serve. The results are shown in Table 3.
Table 3 Guided Plus Video Verbal Coaching
Average Change in speed (mph) 7 10
10. Which of the following results would the expected if Experiment 3 were repeated using left-handed tennis players and a left-handed coach– (A) The average service accuracy of all the players would increases by at least 30%. (B) The average service speed of all the players would decrease slightly. (C) Verbal coaching would improve average service speed less than would watching the video. (D) The average service speed of the players who watched the video would increase by at least 8 mph. 11. Which of the following conclusions could NOT be supported by the results of Experiment– (A) Imitating someone whose handedness is the opposite of one’s own will cause one’s skills to deteriorate. (B) Left-handed people are better than right-handed people at imitating the movement of someone with similar handedness. (C) People learn more easily by observing someone with similar handedness than by observing someone with handedness opposite their own. (D) Right-handed people are better than left-handed people at imitating the movement of someone whose handedness is opposite their own. 12. Which of the following hypotheses is best supported by the results of Experiment 2– (A) Instructional videos are more helpful for right-handed tennis players than is verbal instruction. (B) Instructional videos are more helpful for left-handed tennis players than for right-handed tennis players. (C) Physical guidance by a coach improves both speed and accuracy of service for right-handed tennis players. (D) Physical guidance by a coach improves service accuracy for right-handed tennis players more than for left-handed players. 13. Suppose 50 left-handed tennis players watch a video of a left-handed coach and are also physically guided by that coach. The results of the experiments suggest that the player’s average change in service speed will most closely approximate– (A) –1 mph (B) +5 mph (C) +8 mph (D) +12 mph 14. Which of the following hypotheses is best supported by the results of Experiment 1 alone – (A) Tennis players improve less by observing coaches whose handedness is the opposite of their own than by observing those with similar handedness. (B) Right-handed tennis players are coached by left-handed coaches more frequently than left-handed players are coached by right-handed coaches. (C) Right-handed coaches are better models for all tennis players than are left-handed coaches. (D) People learn much better from physical contact plus a visual stimulus than from the visual stimulus alone.
www.ashoksirphysics.com
61
KINEMATICS THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE It is known that if a steel ball and a feather are dropped from the same height, the steel ball falls faster than the feather. The following scientists have two different views on falling bodies: Scientist 1 : The force of gravity makes things fall. The greater the gravitational force on an object, the faster it falls. The steel ball falls faster because it is more massive than the feather, and thus is attracted more strongly by the earth’s gravitational field. Scientist 2 : The mass of an object does not determine how fast an object falls, but shape does. Two identical pieces of paper will fall at different speeds, if one of them is crumpled into a small ball. That is because gravity is not the only force acting on a falling object. Air buoys up objects falling through it. Since the shape of the steel ball gives it less air resistance than a feather, it falls faster. 15. According to Scientist 1, the property that determines how fast an object will fall is its– (A) chemical composition (B) mass (C) shape (D) gravity 16. According to Scientist 1, the speed of the falling ball could be increased by– (A) dropping the ball from a greater height (B) making the ball out of aluminum (C) reshaping the ball (D) using a larger, steel ball 17. According to Scientist 2, a crumpled piece of paper falls at a different speed from a flat piece of paper because of its– (A) mass (B) air resistance (C) gravitational attraction (D) texture 18. Both scientists agree that the rate at which an object falls is affected by the– (A) force of gravity (B) mass of the object (C) object’s resistance of air (D) shape of object 19. Scientist 2 would predict that in a vacuum, two objects would fall at the same speed if they had the same– (A) shape and different masses (B) air resistance and shape (C) composition and air resistance (D) mass and different shapes 20. Suppose a small ball and a feather having the same weight are dropped from the same height. Which of the following would Scientist 1 predict? (A) the feather would fall faster than the ball (B) the ball and feather would fall at the same speed. (C) the ball would fall faster than the feather (D) none of the above. 21. Two balls of identical shape, one made of lead and one made of aluminum, are both dropped from the same height. Which scientist’s prediction and reasoning is incorrect based on the arguments presented? (A) Scientist 1 : the lead ball falls faster because the balls have different masses. (B) Scientist 1 : the lead ball falls faster because the balls have different gravitational attraction. (C) Scientist 2: The lead ball falls faster because the balls have the same air resistance. (D) Scientist 2: The balls fall at the same rate because they have the same air resistance. THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE A student performs an experiment to determine how the range of a ball depends on the velocity with which it is released. The “range” is the distance between where the ball lands and where it was released, assuming it lands at the same height from which it was released. In each trial, the student uses the same baseball, and launches it at the same angle. Table 1 shows the experimental results.
Table 1 Trial 1 2 3 4
Launch speed (m/s) 10 20 30 40
www.ashoksirphysics.com
Range (m) 8 31.8 70.7 122.5
62
KINEMATICS Based on this data, the student then hypothesizes that the range, R, depends on the initial speed,
0 ,
according to the following to the following equation: R Cv0n , where C is a constant, and n is another constant. 22. Based on this data, the best guess for the value of n is– (A)
1 2
(B) 1
(C) 2
(D) 3
23. The student speculates that he constant C depends on: I. The angle at which the ball was launch. II. The ball’s mass III. The ball’s diameter If we neglect air resistance, then C actually depends on– (A) I only (B) I and II (C) I and III
(D) I, II and III.
24. The student performs another trial in which the ball is launched at speed 5.0 m/s. Its range is approximately– (A) 1.0 meters (B) 2.0 meters (C) 3.0 meters (D) 4.0 meters
25. Let denote the angle of the ball’s initial velocity, as measured from the horizontal. Neglect air resistance. At the peak (highest point) of its trajectory, the ball’s speed is– (B) 0 sin
(A) 0
(C) 0 cos
(D) 0
26. For trial 2, which of the following graphs best represents the vertical component of the ball’s velocity as a function of time, assuming upward is positive? + vy (A)
0
+ vy time
(B)
0
+ vy time
(C)
0
+ vy time
(D)
0
time
THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE Aristotle developed a system of physics based on what he thought occurred in nature. For example, he thought that if a stone is released from rest, it instantaneously reaches a speed that remains constant as the stone falls. He also believed that the speed attained by a stone falling in air varies directly with the weight of the stone. A 5–pound stone, for example, falls with a constant speed 5 times as great as that of a 1-pound stone. Aristotle also noted that stones dropped into water continue to fall, but at a slower rate than stones falling through air. To account for this, he explained that the resistance of the medium through which an object falls also affects the speed. Therefore, he said, the speed of a falling object also varies inversely with the resistance of the medium, and this resistance is the same for all objects. Galielo disagreed with Aristotle’s explanation. He generated the following arguments to refute. Aristotle. Consider a stake partially driven into the ground and a heavy stone falling from various heights onto the stake. If the stone falls from a height of 4 cubits, the stake will be driven into the ground, say, 4 finger breadths. But if the stone falls from a height of 1 cubit, the stake will be driven in a much smaller amount. Certainly, Galileo argued, if the stone is raised above the stake by only the thickness of a leaf, then the effect of the stone’s falling on the stake will be altogether unnoticeable. On the basis of a careful set of experiments, Galielo argued that the speed of an object released from rest varies directly with the time of fall. Also, the distance the object falls varies directly with the square of the time of fall if the effect of air resistance on the object is negligible. Thus, according to Galileo, objects actually fall with constant acceleration, and if air resistance is negligible, all object exactly the same acceleration. 27. Which graph accurately represents Galileo’s theory of the relationship between speed and time for an object falling from rest under conditions of negligible air resistance –
www.ashoksirphysics.com
63
Time
Time
(D)
Speed
(C)
Speed
(B)
Speed
(A)
Speed
KINEMATICS
Time
Time
28. A book dropped from a height of 1 meter falls to the floor in t seconds. To be consistent with Aristotle’s views, from what height, in meters, should a book 3 times as heavy be dropped so that it will fall to the floor in the same amount of time– (A) 1
9
(B) 1
3
(C) 1
(D) 3
29. Suppose a heavy object falls to the ground in t seconds when dropped from shoulder height. According to Galileo, if air resistance were negligible, how many seconds would it take an object half as heavy to fall to the ground from the same height– (A) 0.5 t (B) 1.0 t (C) 1.5 t (D) 2.0 t 30. A piece of putty weighing 2 pounds is dropped down a shaft from the top of a tall building. 1 second later, a 3 pound piece of putty si dropped down the shaft. According to Aristotle, what happens to the 2 pieces of putty if they fall for a relatively long time– (A) The separation between the 2 piece constantly increases until they strike the ground. (B) The separation between the 2 pieces is constant until the strike the ground. (C) The heavier piece catches up to the smaller piece. and the 2 pieces travel together with the speed of the heavier piece. (D) The heavier piece catches up to the smaller piece, and the 2 piece travel together with a speed faster than the speed of either. THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE Abhishek and Sweta Bachhan are two friends Abhishek is Joint to IIT-Pawai and Sweta come to see off him at Indore railway station. Non Abhishek drops ball 1 from 1 mt height, just when the train starts to move. Abhishek observes that ball doesn’t hit the floor point exactly below the dropped point, it gets deviated by 20 cm. For 50 seconds train accelerates and attains the maximum speed and starts moving with this velocity.
50 m
50 m Initial
Final
Now Abhishek drops ball 2 at the same time (t = 50 sec.) Abhishek throw ball 3 such that it just reach Sweta. 31. Path of ball 1 seen by Sweta will be (A) Parabolic (B) Straight line
(C) Circle
(D) Can’t predict
32. Path of ball 1 seen by Abhishek will be – (A) Parabolic (B) Straight line
(C) Circle
(D) Can’t predict
33. The acceleration of train is (A) g/2 (B) g/4
(C) g/5
34. The path of ball 2 as seen by Sweta will be (A) Parabolic (B) Straight line
(C) Circle
(D) Can’t predict
35. The path of ball 2 as seen by Abhishek will be (A) Parabolic (B) Straight line
(C) Circle
(D) Can’t predict
36. The speed with which Abhishek throw ball 3 will be (A) 30 (B) 20
www.ashoksirphysics.com
(D) Can’t predict
(C) 15
(D) None of these
64
KINEMATICS THE NEXT QUESTIONS REFER TO THE FOLLOWING PASSAGE A circus wishes to develop a new clown act. Figure 1 shows a diagram of the proposed setup. A clown will be shot out of a cannon with velocity v0 at a trajectory that makes an angle
45
with the ground. At this
angle, the clown will travel a maximum horizontal distance. The cannon will accelerate the clown by applying a constant force of 10,000 N over a very short time of 0.24 sec. The height above he ground at which the clown begins his trajectory is 10 m. A large hoop is to be suspended from the ceiling by a massless cable at just the right place so that the clown will be able to dive through it when he reaches a maximum height above the ground. After passing through the hoop he will then continue on his trajectory until arriving at the safety net.
42
vy (m/s)
28 21 80
45°
60 kg
40 kg
kg
t(s)
2.1 2.8 4.2 Figure 2 Figure 2 shows a graph of the vertical component of the clown’s velocity as a function of time between the cannon and the hoop. Since the velocity depends on the mass of the particular clown performing the act, the graph shows data for several different masses. 37. If the angle the cannon makes with the horizontal is increased from 45°, the hoop will have to be: (A) moved farther away from the cannon and lowered. (B) moved farther away from the cannon and raised. (C) moved closer to the cannon and lowered. (D) moved closer to the cannon and raised.
Figure 1
38. If the clown’s mass is 80 kg, what initial velocity v0 will he have as he leaves the cannon? (A)
3m s
(B) 15 m
s
(C)
30 m s
(D)
300 m s
39. The slope of the line segments plotted in figure 2 is a constant. Which one of the following physical quantities does this slope represent? (A) g
(B) v0
(C) y y0
(D)
sin
40. From Figure 2, approximately how much time will it take for clown with a mass of 60 kg to reach the safety net located 10 m below the height of the cannon? (A) 4.3 s (B) 6.4 s (C) 5.9 s (D) 7.2 s 41. If the mass of a clown doubles, his initial kinetic energy, mv02 2 , will: (A) remain the same (C) double
(B) be reduced to half (D) four times
42. If a clown holds on to the hoop instead of passing through it, what is the expression for minimum length of the cable so that he doesn’t hit is head on the ceiling as he swings upward?
2v02 (A) g
v02 (B) g
v02 (C) 2g
www.ashoksirphysics.com
v02 (D) 4g
65
KINEMATICS
Level # 2 M O TI O N I N O N E D I M E N SI O N 1.
Two intersecting straight lines moves out parallel to themselves with the speed v 1 and v 2. Calculate the speed of the point of intersection of the lines if the angle between them is .
2.
A base ball player hits a pitched ball so that its velocity reverses direction and its speed changes from 30 m/s to 40 m/s. The bat moves at an average velocity of 30 m/s. and it is in contact with the ball for a distance of 0.05 m. (A) For how long are the bat and ball in contact ? (B) What is the average acceleration of the ball while it is in contact with the bat ?
3.
A car starts from rest with an acceleration of 6 m/s2 which decreases to zero linearly with time, in 10 sec., after which car continues at a constant speed. Find the time required for the car to travel 400 m from the start.
4.
The speed of a motor launch with respect to the water is v = 7 m/s, the speed of the stream u = 3 m/s. When the launch began traveling upstream, a float was dropped from it. The launch travelled 4.2 km upstream, turned about and caught up with the float. How long is it before the launch reaches the float again ? Assume that float is moving with the speed of stream.
5.
The speed of a train increases at a constant rate from zero to v and then remains constant for an interval and finally decreases to zero at a constant rate . If is the total distance described, prove that the total time taken is t = shortest time ?
v 1 1 . At what value of v is the time of travel the shortest ? What is the value of the v 2
6.
A passenger is running at his maximum velocity of 8 m/s to catch a train. When he is a distance d from the nearest entry to the train, the train starts from rest with constant acceleration a = 1 m/s 2 away from the passengers. (A) If d = 30 m and he keeps running, will he be able to jump onto the train. (B) Sketch the position function x(t) for the train, choosing x = 0 at t = 0. On the same graph sketch x (t) for the passenger for various value of initial separation distance d, including d = 30 m and the critical separation distance dc, such that he just catches the train, (C) For the critical separation distance d 0, what is the speed of the train when the passenger catches it ? What is its average speed for the time interval from t = 0 until he catches it ? What is the value of dc.
7.
The acceleration of a particle is given by a = 4t – 30, where a is in meters per second squared and t is in seconds. Determine the velocity and displacement as functions of time. The initial displacement at t = 0 is 2 s0 = –5m, and the initial velocity is v 0 = 3 m/s. a ,m/s x
8.
The acceleration of a particle that moves in the positive x-direction varies with its position as shown. If the velocity of the particle is 0.8 m/s when x = 0, determine the velocity v of the particle when x = 1.4 m.
0.4 0.2 0
9.
A particle starts from rest at x = –2 m and moves along the x-axis with the velocity history shown. Plot the corresponding acceleration and displacement histories for the two seconds. Find the time t when the particle crosses the origin.
www.ashoksirphysics.com
0
0.4
0.8
1.2
v, m/s 3
0 0
2.0 0.5
1.0
1.5
-1
66
KINEMATICS
MOTION UNDER GRAVITY 10.
One student throws a ball vertically upward with an initial speed of 9.8 m/s. Another student standing. 5m away starts running towards the ball on release and catches it at the same height. What was the students acceleration ? (Assume a uniform acceleration).
11.
An aluminium ball with a mass of 4 kg and an iron ball of the same size with a mass of 11.6 kg are dropped simultaneously from a height of 49 m. (A) Neglecting air resistance, how long does it take the aluminium ball to gall to the ground. (B) How much later does the heavier iron ball strike the ground ?
12.
Ball A is dropped from the top of a building at the same instant that ball B is thrown vertically upward from the ground. When balls collide, they are moving in opposite directions and the speed of A is twice the speed of B. At what fraction of the height of the building did the collision occur ?
13.
A ball is thrown vertically upward from the 12 m level in an elevator shaft with an initial velocity of 18 m/s. At the same instant an open platform elevator passes the 5m level, moving upward with a constant velocity of 2 m/s. Determine (A) When and where the ball will hit the elevator, (B) the relative velocity of the ball with respect to the elevator when the ball hits the elevator.
14.
An object is thrown upward with an initial velocity v 0. The drag on the object is assumed to be proportional to the velocity. What time will it take the object to move upward and what maximal altitude will it reach ?
15.
A nut comes loose from a bolt on the bottom of an elevator as the elevator is moving up the shaft at 3 m/s. The nut strikes the bottom of the shaft in 2 sec. (A) How far from the bottom of the shaft was the elevator when the nut fell off. (B) How far above the bottom was the nut 0.25 s after it fell off ?
16.
A helicopter is descending vertically downward with a uniform velocity. At a certain instant, a food packet is dropped from it which takes 5 seconds to reach the ground. As this packet strikes the ground, another food packet is dropped from it, which takes 4 seconds to reach the ground. Find the velocity with which the helicopter is descending and its height, when second packet is dropped. Also find the distance travelled by the helicopter during the interval of dropping the packets.
RELATIVE MOTION 17.
Two bodies moves in a straight line towards each other at initial velocities v 1 and v 2 and with constant accelerations a1 and a2 directed against the corresponding velocities at the initial instant. What must be the maximum initial separation ď Ź max. between the bodies for which they meet during the motion ?
18.
The velocity of a ship in still water is 20 km/h. What is the velocity of a motor boat approaching the ship at right angle to its course if it appears to people on board the ship, that the motor boat heads towards the ship at 60° ?
19.
A man running on a horizontal road at 8 km/h, find the rain falling vertically. He increase the speed to 13 km/ h and find the drops make angle 30° with the vertical. Find the speed and the direction of the rain with respect to the read.
20.
A person riding in the back of a pickup truck traveling at 60 km/h. On a straight, level road throws a ball with a speed of 20 km/h relative to the truck in the direction opposite to its motion. (A) What is the velocity of the ball relative to a stationary observer by the side of the read ? (B) What is the velocity of the ball relative to the driver of a car moving in the same direction as the truck at a speed of 90 km/h. ?
www.ashoksirphysics.com
67
KINEMATICS 21.
The speed of a boat in still water is v. The boat is to make a round trip in a river whose current travels at speed u. Derive a formula for a time needed to make a round trip of total distance D if the boat makes the round trip by moving (A) Upstream and back down stream. (B) Directly across the river and back. We must assume u < v ; Why ? O
22.
In the adjacent figure, a vertical cemented circular plane of radius R having frictionless slots along the chords OA, OB, OC, OD and DE is shown. OGC is the diameter of the circle. Five Boys starts sliding down from rest along the slots OA, OB, OC, OD and OE simultaneously. Find the time taken by each boy to reach at the point A, B, C, D and E.
G A
E D
C
B
PROJECTILE MOTION 23.
A body is dropped from a stationary balloon at a height h above the ground. At the same time a bullet is fired from a gun on the ground with a velocity u. If the angle of elevation of the balloon from the position of the gun is , in which direction should the bullet be fired so that it strikes the body before reaching the ground. Also find the minimum value of u required for this.
24.
Particles P and Q of mass 20 gms and 40 gms respectively are simultaneously projected from points A and B on the ground. The initial velocities of P and Q makes 45° and 135° angles respectively with the horizontal as shown in the figure. Each particle has in initial speed of 49 m/s. The separation AB is 245 m. Both particles travel in the same vertical plane and under go a collision. After the collision P retraces its 135° path. Determine the position of Q when it hits the 45° ground. How much time after the collision does the A B 245 m particle Q take to reach the ground. Take g = 9.8 m/s2.
25.
A boy throws a ball horizontally with a speed of v 0 = 12 m/s from the gandhi setu bridge c of patna in an effort to hit the top surface AB of a truck travelling directly underneath the boy on the bridge. If the truck maintains a constant speed u = 15 m/s, and the ball is projected at the instant B on the top of the truck appears at point C, determine the position s where the ball strikes the top of the truck.
v0=12 m/s
8m
s u
B
A C
10m
u 26.
Two inclined planes intersect in a horizontal plane. Their inclinations to the horizontal being and . If a particle is projected at right angle to the former from a point in it so as to strike the other at right angles, then find the velocity of projection.
P
a
O
www.ashoksirphysics.com
68
KINEMATICS 27.
A cannon fires from under a shelter inclined at an angle to the horizontal. The canon is located at a point at a distance from the base of the shelter. The initial velocity of the shell is v 0 and its trajectory lies in the plane of the figure. Determine the maximum range of the shell.
28.
A juggler manages to keep five balls in motion, throwing each sequentially up a distance of 3m. (a) Determine the time interval between successive throws. (B) Give the positions of the other balls at the instant when one reaches her hand. (Neglect the time taken to transfer balls from one hand to the other.)
29.
A ball is shot from the ground into the air. At a height of 9.1 m., its velocity is observed to be v 7.6 ˆi 6.1ˆj in water per second ( ˆi horizontal, ˆj upward). (A) (B) (C) (D)
To what maximum height does the ball rise ? What total horizontal distance does the ball travel ? What are The magnitude and The direction of the ball’s velocity just before it hits the ground ?
30.
A bomb bursts on contact with the ground and pieces fly off in all directions with speed up to 30 m/s. A girl is standing 40 m away. What is the time duration over which she can be hit by a piece ?
31.
A boy sitting at the rear end of a railway compartment of a train running at a constant acceleration a on horizontal rails fires a shot towards the fore end of the compartment with a muzzle velocity u = 20 m/s at an angle = 37° above the horizontal when the train’s velocity v = 10 m/s. If the boy catches the shot without moving from his seat at the same height as that of projection find (a) Speed of the train at the time when he catches the shot and (B) the acceleration of the train (g = 10 m/s2). y
32.
A projectile of mass m is fired into a liquid at an angle 0 with an initial velocity v 0 as shown. If the liquid develops a frictional or drag resistance on the projectile which is proportional to its velocity. i.e., F = – kv, where k is a positive constant, determine the x and y components of its velocity at any instant. Also, what is the maximum distance x max. that it travels ?
33.
34.
35.
v0 0
x
An elevator is going up with an upward acceleration of 1 m/s2. At the instant when its velocity is 2 m/s, a stone is projected upward from its floor with a speed of 2 m/s relative to the elevator, at an elevation of 30°. (A) Calculate the time taken by the stone to return the floor. (B) Sketch the path of the projectile as observed by an observer outside the elevator. (C) If the elevator was moving with a downward acceleration equal to g, how would the motion be altered ? A particle is moving in the plane with velocity given by, u u0 ˆi a cos t ˆj , where ˆi and ˆj are unit vectors along x and y axes respectively. If particle is at the origin at t = 0 ; 3 (A) Calculate the trajectory of the particle. (B) Find its distance from the origin at time . 200
A man is travelling on a flat car which is moving up a plane inclined at cos –1 (4/5) to the horizontal with a speed 5 m/s. He throws a ball towards a stationary hoop located perpendicular to the inclined in such a way that the ball moves parallel to the slope of the incline while going through the hoop. If the distance d of the hoop from the level of the man’s hand is such that its component perpendicular to the incline is 4 m, calculate the time taken by the ball to reach the hoop.
www.ashoksirphysics.com
69
KINEMATICS 36.
A rifleman on a train moving with a speed of 60 km/hr. fires at an object running away from the train at right angles with a speed of 45 km/hr. If the line connecting the man and the object makes an angle of 30° to the train at the instant of shooting to what angle to the train should be aim in order to hit the object if the muzzle velocity is 850 km/hr. ?
37.
Water is ejected from the water nozzle with a speed v 0 = 14 m/s. For what value of the angle will the water land closest to the wall after clearing the top ? Neglect the effects of wall thickness and air resistance. Where does the water land ?
38.
A bullet is projected so as to graze the tops of two walls each of height 20 m located at distances of 30 and 170 m in the same line from the point of projection as shown in figure. Find the angle and the speed of projection of the bullet. V0
P1
P2 O
20 m
30 m
20 m 170 m
B
30°
39.
Two guns are projected at each other, one upward at an angle of 30 ° and the other at the same angle of depression, the muzzles being 30 m apart as shown in figure problem. If the guns are shot with velocities of 350 m/s upward and 300 m/s downward respectively, find when and where the bullets may meet. A
30 m P y 30° x Bomber
40.
A bomber is flying horizontally at a speed of 500 km/h at an altitude of 3 km such that a ship lies in a vertical plane through the line of sight as shown in figure. Determine the angle of the line of sight of the bomber with the ship at the instant a bomb is released so as to hit the ship. Where would the bomber be at the instant the ship is wrecked ?
Line of Flight
Trajectory
3 km Line of Sight
ship
41.
Two guns are pointed at each other one upwards at an angle of elevation 30° and the other downwards at the same angle of depression, the muzzles being 42 m apart. If the charges leave the guns with velocities 400 m/s and 300 m/s respectively, find when and where they meet.
42.
A particle is projected up an inclined plane of inclination with an initial velocity u at an angle to the horizontal. Find the maximum distance of the projectile from the inclined plane.
43.
On a frictions horizontal surface, assumed to be the x-y plane, a small trolley A is moving along a straight line parallel to the y-axis (see figure) with a constant velocity of
y
3 1 m s . At a particular instant, when the
A
line OA makes an angle of 45° with the x-axis, a ball is thrown along the surface from the origin O. Its velocity makes an angle with the x-axis and it hits the trolley. (a) The motion of the ball is observed from the frame of the trolley. Calculate the angle made by the velocity vector of the ball
45° O
x
with the x-axis in this frame. (B) Find the speed of the ball with respect to the surface, if
www.ashoksirphysics.com
4 . 3
[IIT 2002]
70
KINEMATICS 44.
Two guns, situated on the top of a hill of height 10 m, fire one shot each with the same speed 5 3 ms–1 at some interval of time. One gun fires horizontally and other fires upwards at an angle of 60° with the horizontal. The shots collide in air at a point P. Find (a) the time-interval between the firings, and (b) the coordinates of the point P. [IIT 1996] Take origin of the coordinate system at the foot of the hill right below the muzzle and trajectories in x-y plane.
45.
Two towers AB and CD are situated a distance d apart as shown in figure AB is 20 m high and CD is 30 m high from the ground. An object of mass m is thrown from the top of AB horizontally with a velocity of
2m
C
60°
10 m s towards CD.
Simultaneously another object of mass 2 m is thrown from the top of CD at an angle of 60° to the horizontal towards AB with the same magnitude of initial velocity as that of the first object. The two objects move in the same vertical plane, collide in mid-air and stick to each other. (i) Calculate the distance ‘d’ between the towers and, (ii) Find the position where the objects hit the ground.
A
d
B
D
Answer Key Assertion & Reason
Q. A n s. Q. A n s. Q. A n s. Q. A n s.
1 A 10 C 19 C 28 AD
Que.
1
2
3
4
5
6
7
8
9
10
11
Ans.
C
D
A
A
A
A
C
B
D
E
E
2 A 11 B 20 B 29 ABC
3 A 12 C 21 A 30 ABC
L ev e l – 1 4 5 A C 13 14 B C 22 23 B B 31 ABD
6 D 15 B 24 A
7 B 16 C 25 B
8 AB 17 B 26 A
9 C 18 A 27 B
Fill in the Blanks / True–False / Match Table 1. 2 r , 5. T
r
2.
d v
6. T
www.ashoksirphysics.com
3. 0.6 7. F
4. a 2 4b , 8. F
tan 1 a 9. A
71
KINEMATICS
Passage Type Que. Ans. Que. Ans. Que. Ans. Que. Ans.
1 B 12 C 23 A 34 A
2 C 13 D 24 B 35 B
3 D 14 A 25 C 36 D
4 A 15 B 26 A 37 D
5 A 16 D 27 A 38 C
6 A 17 B 28 D 39 A
7 B 18 A 29 B 40 C
8 C 19 B 30 D 41 B
9 A 20 B 31 B 42 D
10 D 21 C 32 B
11 A 22 C 33 C
Level # 2 M O TI O N I N O N E D I M E N SI O N 1. V v 12 v 22 2 v 1v 0 cos . cos ec
4.
( ) 2
2 5. t min. = ( v u) 15 t 2 + 2/3 t 3
8. V = 1.166 m/s
2. (a) 0.001667 s (b) 42000 m/s2
3. 16.678
6. (a) yes (b) 8 m/s, 4 m/s 32 m
7. v = 3 – 30t + 2t 2, S = –5 + 3t –
9. t = 0.917 s
MOTION UNDER GRAVITY 10. 2.5 m/s2
11. 3.2 s, O
1 r v0 14. t m = r n 1 g
v2 , h = 0 2g
12. 2/3
13. (a) 3.65 s, 12.30 m (b) – 19.81 m/s
15. (a) 13.6 m (b) 14 m
16. 11.04 m/s downward at 177.8 k height, 55.2 m downward.
RELATIVE MOTION 17. max
( v 1 v 2 )2 2 (a1 a 2 )
20. (a) 40 km/h (b) – 50 km/h 22. t = 2
18. 34.6 km/h 21. (a)
DV (b) v u2 2
19. 4 7 km/h
D 2
v u2
R g , every boy will take same time
PROJECTILE MOTION 23. , v min. =
gh .cosec 2
2 ag 26. u sin sin sin cos( )
24. 122.5 m, 3.53 sec.
25. 3.84 m
v 02 1 g sin 2 27. g sin 2 sin v0
www.ashoksirphysics.com
72
KINEMATICS 28. (a) 310 m/s (b) 1.9 m and 2.9 m above the hands 29. (a) 11m (b) 23 m (c) 17 m/s (d) 63 below horizontal.
30. 4.6 sec
m k k / m t g , x = mv 0 cos 0 32. v x = v 0 cos . e(– km) t, v y = k m v 0 sin g e k m x 34. (a) y = a sin u (b) 0
9 2 u02 a 2 4 2
37. = 50.7°, 0.835 to the right of B
31. 42 m/s 33. (a) 0.18 sec
35. 1 sec.
36. 34°.5'
38. 48 m/s
39. 0.0462 s, (14 m, 8.07 m)
40. 48.9°, over the ship. 41. In 0.068 s, at a point whose horizontal of vertical dist. from the 1st gun are 20.78 and 11.98 m
42. s
u 2 sin 2 ( ) 2g cos
43. (a) 45°
(b) 2 m/s.
44. (a) t 1 sec ond
(b) 5 3 m, 5m
45. 17.32, 11.547 m from B
—X—X—X—X—
www.ashoksirphysics.com
73