Hard x ray photoelectron spectroscopy haxpes 1st edition joseph c woicik eds

Visit to download the full and correct content document: https://textbookfull.com/product/hard-x-ray-photoelectron-spectroscopy-haxpes-1st-ed ition-joseph-c-woicik-eds/

More products digital (pdf, epub, mobi) instant download maybe you interests ...

Handbook of Mineral Spectroscopy: X-Ray Photoelectron Spectra: Volume 1: X-ray Photoelectron Spectra 1st Edition J. Theo Kloprogge

https://textbookfull.com/product/handbook-of-mineralspectroscopy-x-ray-photoelectron-spectra-volume-1-x-rayphotoelectron-spectra-1st-edition-j-theo-kloprogge/

X Ray Spectroscopy with Synchrotron Radiation Fundamentals and Applications Stephen P. Cramer

https://textbookfull.com/product/x-ray-spectroscopy-withsynchrotron-radiation-fundamentals-and-applications-stephen-pcramer/

X-Ray Lasers 2014: Proceedings of the 14th International Conference on X-Ray Lasers 1st Edition Jorge Rocca

https://textbookfull.com/product/x-ray-lasers-2014-proceedingsof-the-14th-international-conference-on-x-ray-lasers-1st-editionjorge-rocca/

X Ray Lasers 2016 Proceedings of the 15th International Conference on X Ray Lasers 1st Edition Tetsuya Kawachi

https://textbookfull.com/product/x-ray-lasers-2016-proceedingsof-the-15th-international-conference-on-x-ray-lasers-1st-editiontetsuya-kawachi/

Handbook of X-ray and Gamma-ray Astrophysics 1st Edition Cosimo Bambi

https://textbookfull.com/product/handbook-of-x-ray-and-gamma-rayastrophysics-1st-edition-cosimo-bambi/

X Ray Lasers 2012 Proceedings of the 13th International Conference on X Ray Lasers 11 15 June 2012 Paris France 1st Edition E. Allaria

https://textbookfull.com/product/x-ray-lasers-2012-proceedingsof-the-13th-international-conference-on-x-raylasers-11-15-june-2012-paris-france-1st-edition-e-allaria/

Resonant X Ray Scattering in Correlated Systems 1st Edition Youichi Murakami

https://textbookfull.com/product/resonant-x-ray-scattering-incorrelated-systems-1st-edition-youichi-murakami/

Handbook of X-ray Imaging: Physics and Technology 1st Edition Paolo Russo

https://textbookfull.com/product/handbook-of-x-ray-imagingphysics-and-technology-1st-edition-paolo-russo/

Handbook of X-ray Imaging: Physics and Technology 1st Edition Paolo Russo (Editor)

https://textbookfull.com/product/handbook-of-x-ray-imagingphysics-and-technology-1st-edition-paolo-russo-editor/

Joseph C. Woicik Editor

Hard X-ray Photoelectron Spectroscopy (HAXPES)

SpringerSeriesinSurfaceSciences

Volume59

Serieseditors

RobertoCar,Princeton,USA

GerhardErtl,Berlin,Germany

Hans-JoachimFreund,Berlin,Germany

HansLüth,Jülich,Germany

MarioAgostinoRocca,Genova,Italy

Thisseriescoversthewholespectrumofsurfacesciences,includingstructureand dynamicsofcleanandadsorbate-coveredsurfaces,thin films,basicsurfaceeffects, analyticalmethodsandalsothephysicsandchemistryofinterfaces.Writtenby leadingresearchersinthe field,thebooksareintendedprimarilyforresearchersin academiaandindustryandforgraduatestudents.

Moreinformationaboutthisseriesathttp://www.springer.com/series/409

JosephC.Woicik Editor

HardX-rayPhotoelectron Spectroscopy(HAXPES)

Editor JosephC.Woicik

BrookhavenNationalLaboratory NationalInstituteofStandards andTechnology

Upton,NY USA

ISSN0931-5195ISSN2198-4743(electronic)

SpringerSeriesinSurfaceSciences

ISBN978-3-319-24041-1ISBN978-3-319-24043-5(eBook) DOI10.1007/978-3-319-24043-5

LibraryofCongressControlNumber:2015950038

SpringerChamHeidelbergNewYorkDordrechtLondon © SpringerInternationalPublishingSwitzerland2016

Thisworkissubjecttocopyright.AllrightsarereservedbythePublisher,whetherthewholeorpart ofthematerialisconcerned,specificallytherightsoftranslation,reprinting,reuseofillustrations, recitation,broadcasting,reproductiononmicrofilmsorinanyotherphysicalway,andtransmission orinformationstorageandretrieval,electronicadaptation,computersoftware,orbysimilarordissimilar methodologynowknownorhereafterdeveloped.

Theuseofgeneraldescriptivenames,registerednames,trademarks,servicemarks,etc.inthis publicationdoesnotimply,evenintheabsenceofaspecificstatement,thatsuchnamesareexemptfrom therelevantprotectivelawsandregulationsandthereforefreeforgeneraluse.

Thepublisher,theauthorsandtheeditorsaresafetoassumethattheadviceandinformationinthis bookarebelievedtobetrueandaccurateatthedateofpublication.Neitherthepublishernorthe authorsortheeditorsgiveawarranty,expressorimplied,withrespecttothematerialcontainedhereinor foranyerrorsoromissionsthatmayhavebeenmade.

Printedonacid-freepaper

SpringerInternationalPublishingAGSwitzerlandispartofSpringerScience+BusinessMedia (www.springer.com)

Preface

PhotoelectronspectroscopyhasitsrootsintheNobelPrize-winningworkofAlbert EinsteinandKaiSiegbahn.Itisthereforebothanhonorandahumblingexperience toproduceabookthatdocumentstheexcitementofthenewestdevelopmentsin this field.

AccordingtoEinstein’sdiscoveryofthelawofthephotoelectriceffect,consideredtobethedawnofthequantumage,theconservationofenergybetweenthe incomingphotonandtheoutgoingphotoelectroninthephotoemissionprocess allowsthetechniquetouniquelymeasurethechemicalandelectronicstructureof atoms,molecules,andsolids.However,despiteSeigbahn’soriginaldevelopment ofthetechniqueforchemicalanalysiswithhigh-energyX-rays,theuseof low-energyphotonswithenergiesuptoonlyabout1.5keVbymodernresearchers, atbothlaboratoryandsynchrotronsources,resultsinextremelyshortphotoelectron inelasticmean-freepaths.Asaresult,thislimitedinformationdepthhashistorically restrictedexperimentstothestudyofsurfacesandshallowinterfaces,orwhatis referredtointheliteratureastraditionalsurfacescience.

Itisthereforenosurprisethatrecentadvancesinbothphotonsourceand electron-spectrometerinstrumentationhavedrivenexperimentsintotheextended 2–10keVphotonenergyrangeresultinginwhatisnowcalled hard X-rayphotoelectronspectroscopy(HAXPES).Duetoitsrelativelyunlimitedelectronescape depths,HAXPEShasemergedasapowerfultoolthathasgeneralapplicationtothe studyofthetruebulkandburiedinterfacepropertiesofcomplexmaterialssystems. Itsareasofapplicationarethusgrowingexponentiallycomparedtomoretraditional measurementsatlowerphotonenergies.

Inadditiontothemanyadvantagesofbeingabletostudy “real” samplestaken directlyfromairwithouttheneedforionsputteringorothersurfacepreparation, HAXPEShasopenedupotherresearchareasthatareincludedinthisbooksuchas:

Preface

• Thestudyofhighlycorrelatedandspintronicelectronsystemswithsurfaceand interfacecompositionsandstructuresthataredifferentfromtheirbulk.

• Thecombinationofenergyandanglemeasurements(X-raystandingwave, photoelectrondiffraction,andangle-resolvedvalencephotoemission)toproduce elementally,chemically,andspatiallyspecifi celectronicstructureinformation.

• Thestudyofrealisticprototypicalmultilayerdevicestructuresunderboth ambient and operando conditions.

• Thetuningofthephotoelectroninelasticmean-freepathandtheX-raypenetrationdepthtostudyburiedlayers,interfaces,andnanoparticleswiththe speci ficnanometerandmesoscopiclengthscalesrelevanttomodernindustry, as,forexample,today’ssemiconductorhetero-structures.

Thebrightnessofthird-andhighergenerationX-raysourceshasalsoopenedthe possibilitiesofbothhigh-resolutiontwo-dimensionalchemicalimagingwithdepth resolution(photoelectronmicroscopy)inadditiontotime-resolvedphotoemission. Thisvolumeprovidesthe fi rstcomplete,up-to-datesummaryofthestateofthe artinHAXPES.Itisthereforeamust-readforscientistsinterestedinharnessingits powerfulcapabilitiesfortheirownresearch.Chapterswrittenbyexpertsinclude historicalwork,moderninstrumentation,theoreticaldevelopments,andreal-world applicationsthatcoverthe fieldsofphysics,chemistry,andmaterialsscienceand engineering.Inconsiderationoftherapiddevelopmentofthetechnique,several chaptersincludehighlightsthatillustratefutureopportunitiesaswell.

Upton,USAJosephC.Woicik

1HardX-rayPhotoemission:AnOverviewandFuture Perspective..........................................1 CharlesS.Fadley

2TheFirstDevelopmentofPhotoelectronSpectroscopy andItsRelationtoHAXPES ............................35 SvanteSvensson,EvelynSokolowskiandNilsMårtensson

3HAXPESattheDawnoftheSynchrotronRadiationAge .......43 PieroPianettaandIngolfLindau

4Hard-X-rayPhotoelectronSpectroscopyofAtoms andMolecules .......................................65 MarcSimon,MariaNovellaPiancastelliandDennisW.Lindle

5InelasticMeanFreePaths,MeanEscapeDepths, InformationDepths,andEffectiveAttenuationLengths forHardX-rayPhotoelectronSpectroscopy .................111 C.J.PowellandS.Tanuma

6HardX-rayAngle-ResolvedPhotoelectronSpectroscopy (HARPES) ..........................................141 AlexanderX.Gray

7OneStepModelDescriptionofHARPES:Inclusion ofDisorderandTemperatureEffects ......................159 JürgenBraun,JánMinárandHubertEbert

8RecoilEffectsinX-rayPhotoelectronSpectroscopy ............175 YosukeKayanuma

9Depth-DependenceofElectronScreening,ChargeCarriers andCorrelation:TheoryandExperiments ..................197 MunetakaTaguchiandGiancarloPanaccione

10TheInfluenceofFinal-StateEffectsonXPSSpectra fromFirst-RowTransition-Metals .........................217 AndrewP.Grosvenor,MarkC.Biesinger,RogerSt.C.Smart andAndreaR.Gerson

11OptimizingPolarizationDependentHardX-rayPhotoemission ExperimentsforSolids .................................263

J.Weinen,T.C.Koethe,S.Agrestini,D.Kasinathan,F.Strigari, T.Haupricht,Y.F.Liao,K.-D.TsueiandL.H.Tjeng

12PhotoelectronEmissionExcitedbyaHardX-ray StandingWave .......................................277

JörgZegenhagen,Tien-LinLeeandSebastianThiess

13DepthPro filingandInternalStructureDetermination ofLowDimensionalMaterialsUsingX-rayPhotoelectron Spectroscopy ........................................309 SumantaMukherjee,PralayK.SantraandD.D.Sarma

14ProbingPerovskiteInterfacesandSuperlatticeswithX-ray PhotoemissionSpectroscopy .............................341 ScottA.Chambers

15HAXPESMeasurementsofHeterojunctionBandAlignment .....381 ConanWeiland,AbdulK.RumaizandJosephC.Woicik

16HAXPESStudiesofAdvancedSemiconductors ...............407 PatrickS.LysaghtandJosephC.Woicik

17Liquid/SolidInterfacesStudiedbyAmbientPressure HAXPES ...........................................447 Z.LiuandH.Bluhm

18HAXPESApplicationstoAdvancedMaterials ................467 KeisukeKobayashi

19PhotoelectronMicroscopyandHAXPES ....................533 RaymondBrowning

20FemtosecondTime-ResolvedHAXPES .....................555 Lars-PhilipOloff,MasakiOura,AshishChainani andKaiRossnagel Index

Contributors

S.Agrestini MaxPlanckInstituteforChemicalPhysicsofSolids,Dresden, Germany

MarkC.Biesinger SurfaceScienceWestern,TheUniversityofWesternOntario, London,ON,Canada

H.Bluhm LawrenceBerkeleyNationalLaboratory,ChemicalSciencesDivision, Berkeley,CA,USA

JürgenBraun DepartmentofChemie,Ludwig-Maximilians-Universität München,Munich,Germany

RaymondBrowning Shoreham,NY,USA

AshishChainani RIKENSpring-8Center,Hyogo,Japan

ScottA.Chambers PhysicalSciencesDivision,Pacifi cNorthwestNational Laboratory,Richland,WA,USA

HubertEbert DepartmentofChemie,Ludwig-Maximilians-UniversitätMünchen, Munich,Germany

CharlesS.Fadley DepartmentofPhysics,UniversityofCaliforniaDavis,Davis, CA,USA;MaterialsSciencesDivision,LawrenceBerkeleyNationalLaboratory, Berkeley,CA,USA

AndreaR.Gerson MineralsandMaterialsScienceandTechnology(MMaST), MawsonInstitute,UniversityofSouthAustralia,Adelaide,SA,Australia;Blue MineralsConsultancy,Adelaide,SA,Australia

AlexanderX.Gray DepartmentofPhysics,TempleUniversity,Philadelphia,PA, USA

AndrewP.Grosvenor DepartmentofChemistry,UniversityofSaskatchewan, Saskatoon,Canada ix

T.Haupricht II.PhysikalischesInstitut,UniversitätzuKöln,Cologne,Germany

D.Kasinathan MaxPlanckInstituteforChemicalPhysicsofSolids,Dresden, Germany

YosukeKayanuma MaterialsandStructuresLaboratory,TokyoInstituteof Technology,Nagatsuta,Yokohama,Japan

KeisukeKobayashi HiroshimaSynchrotronRadiationCenter,Hiroshima University,Higashi-HiroshimaCity,Japan;QuantumBeamScienceDirectorate, JapanAtomicEnergyAgency,Sayo-Cho,Hyogo,Japan;ResearchInstituteof KUT,KochiUniversityofTechnology,KamiCity,Kochi,Japan

T.C.Koethe II.PhysikalischesInstitut,UniversitätzuKöln,Cologne,Germany

Tien-LinLee DiamondLightSourceLimited,DiamondHouse,Didcot, Oxfordshire,UK

Y.F.Liao NationalSynchrotronRadiationResearchCenter,Taiwan,China

IngolfLindau SLACNationalAcceleratorLaboratory,MenloPark,CA,USA

DennisW.Lindle DepartmentofChemistry,UniversityofNevada,LasVegas, NV,USA

Z.Liu AdvancedLightSource,LawrenceBerkeleyNationalLaboratory, Berkeley,CA,USA;StateKeyLaboratoryofFunctionalMaterialsforInformatics, ShanghaiInstituteofMicrosystemandInformationTechnology,ChineseAcademy ofSciences,Shanghai,China;CondensedMatterPhysicsandPhotonScience Division,SchoolofPhysicalScienceandTechnology,ShanghaiTechUniversity, Shanghai,China

PatrickS.Lysaght SEMATECH,Albany,NY,USA

NilsMårtensson DepartmentofPhysicsandAstronomy,Uppsala,Sweden

JánMinár DepartmentofChemie,Ludwig-Maximilians-UniversitätMünchen, Munich,Germany;NewTechnologies ResearchCenter,UniversityofWest Bohemia,Pilsen,CzechRepublic

SumantaMukherjee SolidStateandStructuralChemistryUnit,IndianInstitute ofScience,Bengaluru,India

Lars-PhilipOloff InstituteofExperimentalandAppliedPhysics,Universityof Kiel,Kiel,Germany;RIKENSpring-8Center,Hyogo,Japan

MasakiOura RIKENSpring-8Center,Hyogo,Japan

GiancarloPanaccione IstitutoOfficinaDeiMateriali(IOM)-CNR,Laboratorio TASC,Trieste,Italy

MariaNovellaPiancastelli LaboratoiredeChimiePhysique-Matièreet Rayonnement,CNRSandUPMC,Cedex05Paris,France;DepartmentofPhysics andAstronomy,UppsalaUniversity,Uppsala,Sweden

PieroPianetta SLACNationalAcceleratorLaboratory,MenloPark,CA,USA

C.J.Powell MaterialsMeasurementScienceDivision,NationalInstituteof StandardsandTechnology,Gaithersburg,MD,USA

KaiRossnagel InstituteofExperimentalandAppliedPhysics,UniversityofKiel, Kiel,Germany;RIKENSpring-8Center,Hyogo,Japan

AbdulK.Rumaiz NationalSynchrotronLightSourceII,BrookhavenNational Laboratory,Upton,NY,USA

PralayK.Santra SolidStateandStructuralChemistryUnit,IndianInstituteof Science,Bengaluru,India;DepartmentofChemicalEngineering,Stanford University,Stanford,USA

D.D.Sarma SolidStateandStructuralChemistryUnit,IndianInstituteofScience, Bengaluru,India;DepartmentofPhysicsandAstronomy,UppsalaUniversity, Uppsala,Sweden

MarcSimon LaboratoiredeChimiePhysique-MatièreetRayonnement,CNRS andUPMC,Cedex05Paris,France

RogerSt.C.Smart MineralsandMaterialsScienceandTechnology(MMaST), MawsonInstitute,UniversityofSouthAustralia,SouthAustralia,Australia

EvelynSokolowski Tystberga,Sweden

F.Strigari II.PhysikalischesInstitut,UniversitätzuKöln,Cologne,Germany

SvanteSvensson DepartmentofPhysicsandAstronomy,Uppsala,Sweden

MunetakaTaguchi RIKENSPring-8Center,Hyogo,Japan;NaraInstituteof ScienceandTechnology(NAIST),Ikoma,Nara,Japan

S.Tanuma NationalInstituteforMaterialsScience,Tsukuba,Ibaraki,Japan

SebastianThiess DeutschesElektronen-Synchrotron,Hamburg,Germany

L.H.Tjeng MaxPlanckInstituteforChemicalPhysicsofSolids,Dresden, Germany

K.-D.Tsuei NationalSynchrotronRadiationResearchCenter,Taiwan,China

ConanWeiland MaterialsMeasurementsLaboratory,NationalInstituteof StandardsandTechnology,Gaithersburg,MD,USA

J.Weinen MaxPlanckInstituteforChemicalPhysicsofSolids,Dresden, Germany

JosephC.Woicik MaterialsMeasurementLaboratory,NationalInstituteof StandardsandTechnology,Gaithersburg,MD,USA

JörgZegenhagen DiamondLightSourceLimited,DiamondHouse,Didcot, Oxfordshire,UK

Chapter1

HardX-rayPhotoemission:AnOverview andFuturePerspective

CharlesS.Fadley

Abstract ThevariousaspectsofhardX-rayphotoemissionarereviewed,including inparticularmorenewlydevelopeddirectionsofmeasurement,butalsowithreferencestootherchaptersinthisbookorpriorpublicationsinwhichadditional detailscanbefound.Anoverviewofthedifferentdimensionsofthetechnique, includingalookatpromisingfuturedirections,ispresented.

1.1Introduction

AlthoughhardX-rayphotoemission(HXPS,HAXPES,HX-PES, )infacthasa longhistory,asreviewedelsewhereinthisbookbySvensson,Sokolowski,and Martensson,byPianettaandLindau,whopioneereditwithsynchrotronradiation (SR)excitationatSSRL[1],andbyKobayashi,whodiscussesthe fi rst undulator-basedactivitiesatSPring-8,itisreallyonlyinthelast15yearsorsothat thedevelopmentofbeamlines,spectrometers,andevenlaboratorysources,hasled toitsrapidgrowth.Bynow,variousstatisticsindicatetherapidgrowthofthe technique.Thenumberofpapersappearingandthecitationstothemaregrowing exponentially,asshownfromtheWebofSciencestatisticsinFig. 1.1,which certainlyrepresentconservativenumbersduetothefactthatauthorsmaynot alwaysuseoursearchkeywordsinpublications,andinfactprobablydothisless withtimeasthetechniquebecomesmorecommonlyused.Someoverallnumbers

C.S.Fadley(&)

DepartmentofPhysics,UniversityofCaliforniaDavis,Davis,CA95616,USA e-mail:fadley@physics.ucdavis.edu

C.S.Fadley

MaterialsSciencesDivision,LawrenceBerkeleyNationalLaboratory,Berkeley CA94720,USA

© SpringerInternationalPublishingSwitzerland2016

J.C.Woicik(ed.), HardX-rayPhotoelectronSpectroscopy(HAXPES), SpringerSeriesinSurfaceSciences59,DOI10.1007/978-3-319-24043-5_1

Measures of the growth and impact of hard x-ray photoemission

Fig.1.1 AWebofScienceplotofthenumberofpublicationsandcitationsversustimeinvolving thekeywords “hardX-rayphotoelectronspectroscopy” or “hardX-rayphotoemission” or “high energyphotoelectronspectroscopy” or “highkineticenergyphotoelectrondiffraction” or “hard X-rayphotoelectronmicroscopy” or “HXPS” or “HAXPES” or “HX-PES” or “HAXPEEM”.This dataisfromJune,2015

fromthissearchinJune,2015areabout640publications,1000citationsperyear, 5600citationsintotal,9cites/paper,andanh-indexof38.Thesepublicationshave furthermoreappearedinleadinghigh-impactjournals.Therearealsocurrently approximately20synchrotronradiationbeamlinesrunningorinconstruction/ commissioningthatareatleastpartlydedicatedtoHXPS,inalphabeticalorderat: ALS,BESSYII,CLS,Diamond,PetraIII,NSRRC,NSLS-2,Soleil,andSPring-8, withbyfarthelargestnumberatSPring-8,stilltheleadingfacilityinthistechnique. Commercialsystemspermittingin-laboratorymonochromatizedHXPSarealso nowavailable.Finally,therehasbeenacontinuingseriesofinternationalworkshopsandbynowinternationalconferencesonHXPS,withprogramsandproceedingsoftenonline[2–10].

Beyondthis,andmoreimportantly,thetechniquehasbynowbeenappliedtothe fullrangeofforefrontmaterialsissuesinphysicsandchemistry,includingbulk, surface,andburiedinterfacestudies,asbeautifullydemonstratedinvariouschapters inthisbook,e.g.,byBrowning photoelectronmicroscopyofvariousmaterials types;Chambers-oxideheterostructures;Gray dilutemagneticsemiconductors; Kobayashi abroadrangeofadvancedmaterialsanddevicestructures;Liuand Bluhm ambientpressurephotoemissionstudiesofsurfacesandinterfaces, includingveryrecentuseofhardX-rayexcitation[11];TaguchiandPanaccionne, plusTjengetal. stronglycorrelatedmaterials;Mukherjee,SantraandSarma nanostructures;Weiland,Rumaiz,andWoicik,plusLysaghtandWoicik band alignmentsandsemiconductors,andZegenhagen,Lee,andThiess oxidesand superconductors.Inaddition,itisclearthatHXPScanbeveryfruitfullyappliedin atomicandmolecularphysics,asoverviewedbySimon,Piancastelli,andLindle. IherealsonotewithdeepsadnessourlosslastyearofDennisLindle,atruepioneer

inapplyinghardX-rayexcitationtoatomicandmolecularphysicswithhisworkat theAdvancedLightSource.

Iwillnotattemptheretorepeatwhatisalreadysowellreviewedandpresented intheabove-citedchaptersdevotedtoapplicationsofHXPS,butwilllimitthis overviewtodiscussingthebasicprinciplesofthetechnique,includingitsstrengths, weaknesses,somenewdirections,andchallengesforfutureexperimentaland theoreticaldevelopments.Thisdiscussionwillthusmoredirectlyrelatetoother chaptersonthefundamentalphysicsofphotoemissioninthehardX-rayregimeby Braun,Ebert,andMinar photoemissiontheory;Browning-photoelectronmicroscopy;Grosvenoretal. fi nal-stateeffects;Kayanuma-recoileffects;Powelland Tanuma inelasticmeanfreepaths;andRossnageletal. time-resolvedmeasurements.Someadditionalnewmeasurementmethodswillbepointedout,for example,involvingstanding-wave(SW)ornear-total-reflection(NTR)excitation frommultilayerheterostructures,whicharenotcoveredelsewhereinthisbook.

Finally,thereaderisdirectedtoseveralotheroverviewsandspecialjournal issuesinvolvingHXPSanditsrelationshiptoconventionalXPSatlessthan2keV thathavebeenpublished[12–14],includingsomefrommygroup[15–20],andto whichspeci ficreferenceswillsubsequentlybemade.

Inconcludingthisintroduction,itisworthnotingthevariousmeasuring modalitiesinphotoemissioningeneral,whichareillustratedinFig. 1.2,allof whichwillbediscussedindividuallyinthefollowingsections.

Fig.1.2 Schematicillustrationofthedifferentmeasurementmodalitiesinphotoemission,with inset examplesdiscussedlaterinthisandotherchaptersofthisbook.Thecoreschematicfrom whichthis figureisderivedisfromY.Takata

GaAs(100)

1.2BasicEffectsandConsiderations

Advantages, Disadvantages,andChallenges

1.2.1ProbingDepth

Ofcourse,theabilitytoprobemoredeeplyintoasampleandreducetheimportance ofsurfaceeffectsisaprimaryreasonforusinghardX-rayexcitationinphotoemission.HardX-rayisheredefinedas>2keV,sincethatistypicallytheenergy abovewhichcrystalmonochromators,insteadofgratingmonochromators,mustbe used.Someprefertocalltherangeofca.2–10keVthatismostcommonlyusedin HXPSmeasurements “tender” X-rays.

Theprobingdepthiscontrolledbytheinelasticmeanfreepath(IMFP),and Fig. 1.3 showsacompilationofvaluesfor41elementscalculatedfromopticaldata andleadingtothemuch-usedTPP-2Mformulaforestimatingthem,fromthework ofTanumaetal.[21].ThismethodanditsapplicationtoHXPSarediscussedin moredetailinthechapterbyTanumaandPowell.

Theconclusionsfromthisandotherrecentexperimentalwork[22, 23]arethat theonlyreliablewaytoincreasebulkorburiedlayerandinterfacesensitivityforall materialtypesistogotohigherphotonenergiesinthesoftX-ray(ca.0.5–2keV)or hardX-ray(ca.2–10keV)regime.Goingtoverylowphotonenergieswithlaser excitationisalsooftendiscussedasamethodforenhancingbulksensitivity[24], butitseemsclearthatthiswillnotbeauniversalbenefitforallmaterials,andmay onlybetrueforthosewithasignifi cantbandgap.Furtherexperimentalandtheoreticalstudyofthislastpointisneeded.

Fig.1.3 Energydependenceofelectroninelasticmeanfreepathsascalculatedfromoptical propertiesfor41elements,withvaluescloselyrelatedtotheTTP-2Mformula(From[21])

1.2.2EaseofSpectralAnalysis

Thereareseveralwaysinwhichspectralanalysisissimplerathigherphoton energies:

• Theinelasticbackgroundsunderspectraaresigni ficantlyreduced,thusmaking theallowanceforthemin fittingtoderivevariouspeakintensitieseasier.

• Augerspectraareingeneralfurtherapart,thuscreatinglessoverlapwith photoelectronpeakswhosedetailedanalysisisdesired.

• Peakintensityanalyses: Theanalysisofpeakintensitiesviastandardformulasforcorephotoelectron emission,suchasthatshownin(1.1)andFig. 1.4 foratypical n‘j levelinatom Q,withanincident fluxof Ihv ðx; y; z; ^ eÞ,radiationpolarizationof ^ e,anIMFPof Ke ðEkin Þ,andaspectrometeracceptancesolidangleoverthesurfaceof XðEkin ; x; yÞ,

andasusede.g.inangle-resolvedXPS(ARXPS)depthprofileanalyses,are simplerbecause:

– TheIMFPs Λe(Ekin)ofdifferentpeaks,althoughkineticenergydependent,can havemuchlessvariationthanwithlowerenergyexcitation,becausethehigher kineticenergiesofless-boundelectroniclevelsarecloserinrelativevalues.

– Theinstrumentresponsefunction,indicatedasthesolid-angleofacceptance Ω(Ekin, x, y)inFig. 1.4,willalsotendtobemorenearlyconstantoverasetof peakswithhigh,andthusverynearlyequal,kineticenergies.

–

–

Theeffectsofelasticscatteringinsmearingoutthephotoelectronintensity distribution,indicatedbythescatteringfactor f(θscatt)inFig. 1.4,willbeless pronounced,duetothegenerallyincreasingforwardfocusingeffectas energyisincreased.

Theeffectofrefractionincrossingtheinnerpotentialbarrier V0 willbeless asenergyisincreased.

– Theeffectsofsurface-associatedinelasticscatteringwillalsobereducedas thekineticenergyincreases[25].

– In valencephotoemission,itisalsowellknown[26, 27]thatthephotoelectric crosssectionbecomesmoreandmoredominatedbythecoreregionofeach atomasenergyisincreased,thuspermittinganapproximatedecompositionof avalencespectruminthehigh-energyXPSordensity-of-states(DOS)limit intoasumofpartialintensitiesbasedonorbital-projectedDOSsandatomic differentialcrosssections,asindicatedin(1.2)below:

Fig.1.4 Corephotoelectronemission,withageneralsampleandexperimentalconfiguration indicated,alongwiththestandardformulaforanalyzingintensities,alsoappearingin(1.1)

Itotal ðEkin Þ¼ X Qn‘j I ðEkin ; Qn‘jÞ ¼ X Qn‘j

with qQn‘j ðEb ; x; y; zÞ theprojecteddensityofstatesforthe Qn‘j orbitalatagiven bindingenergyandpositioninthesample.

Thus,thesimpleformulasshownin(1.1)orin(1.2)willbemorequantitativefor HXPSinmanysituations,permittingsimplerspectralanalysesofbothcoreand valencespectra.

Itisimportant fi nallytonotethatauser-friendlyprogramexistsforcalculating spectraforcore-levelemission,namelySimulationofElectronSpectraforSurface Analysis(SESSA),whoseinputdatabaseshaverecentlybeenextendedtocover hardX-rayexcitation[28, 29].Thisprogramincludesallofthephysicaleffects indicatedinFig. 1.4,withelasticscatteringassumedtobefromanarrayofrandomlypositionedatoms,althoughitdoesnotincluderefractioneffectsincrossing theinnerpotential.

1.2.3PhotoelectricCrossSections,IncludingPolarization

Effects

Beyondtheseconsiderationshowever,istheclearchallengethatphotoelectriccross sectionsdecreasedramaticallyasphotonenergy,orequivalentykineticenergy Ekin isincreased[30–32],varyinginahigh-energyasymptoticlimitroughlyas σQn‘j (Ekin) ∝ (Ekin) 7/2 =(Ekin) 3.5 forssubshellsand ∝(Ekin) 9/2 =(Ekin) 4.5 forp,d,and fsubshells,butwithmoreaccuratecalculationsforspeci ficcasesinthetworeferencesmentioned.Figure 1.5 illustratesthisdecreasewithcalculatedcrosssections [30]forthesubshellsofMnandOover1–10keVspanningthemostcommon HXPSrange.Thus,thedevelopmentofHXPShasrequiredthedesignofbeamlines, includingenhancedintensitywithundulatorexcitation[33],andlaboratorysources withthehighestpossibleintensities,aswellasspectrometerswiththehighestsolid anglesofacceptance,withthelatterforexamplenowgoingupto ±30° incommercialhemisphericalelectrostaticinstrumentsandupto ±45° incustom-designed systems[34].Thepossibilityoftime-of-flightanalysistofurtherincreaseintensities isalsobeingdiscussed[35, 36].However,withpresentSRHXPSfacilities,itisstill possibletosaturateanyexistingdetectorforintensecorelevels,andresearchand developmentthusneedstobedoneforhigher-throughputelectrondetectorscapable oftheGHz-regime[37],ascomparedtothecurrent *1MHzfor2Ddetection,and *10MHzfor1Ddetection.

Anotherimportantconsequenceofthisenergyvariationforvalence-levelstudies isthatsubshellswithlower ‘ foragiven n thatthusexhibitmoreoscillationsinthe coreregiondecayinintensitylessrapidlythanthosewithhigher ‘.Figure 1.5 illustratesthat,forexample,Mn3sdecayslessrapidlythanMn3p,andMn3pless

Mn 1s(1/2)

Mn 2s(1/2)

Mn 2p(1/2)

Mn 2p(3/2)

Mn 3s(1/2)

Mn 3p(1/2)

Mn 3p(3/2)

Mn 3d(3/2)

Mn 3d(5/2)

Mn 4s(1/2)

O 1s(1/2)

O 2s(1/2)

O 2p(1/2)

O 2p(3/2)

RelativisticsubshellcrosssectionsforMnandOasafunctionofphotonenergyoverthe region1–10keV(From[30])

Photoelectric cross sections for O and Mn (Scofield)

Subshell

Photon energy (eV)

Fig.1.5

rapidlythanMn3d.AsimilarthingisfoundforO2s,whichdecayslessrapidly thanO2p.Thesevariationsalreadymaketheasymptoticformulasabove,whichdo notdiscriminatep,d,andfcrosssections,lookinaccuratefortypicalHXPS energies.Infact,theexponentsderivedwithcrosssectionratiosfromFig. 1.5 at8 and10keV,byassumingthat(Crosssectionathν =10,000)/(Crosssectionat hν =8000)=(Ekin at10,000eV)m/(Ekin at8000eV)m yieldvaluesofm= 2.4to 2.6forMn1s,Mn2s,Mn3s,andMn4s,orabout5/2,withO1sandO2sbeing somewhathigheratm=3.0,orabout6/2.Mn2pandMn3pshowm= 3.3to 3.4,andO2plargervaluesofm= 3.9to 4.0,orabout7/2–8/2.Lastly,Mn3d hasthelargestvalueatm= 4.2to 4.3,approachingtheasymptoticlimitof9/2. Thus,thesevaluesonlyroughlyagreewith,andspanagreaterrangethan,the asymptoticnumbersabove,andofcourseclearlyshowthetrendswith ‘ foragiven n alreadymentioned.

Othermorecomplexbutimportantvariationsinrelativeintensityalsooccurif oneconsiderstheimportantcaseofvalence-electronspectra.Forexample,by 10keVthevalencespectrumofasamplewithbothMnandOinit(aswouldbe typicalforatransitionmetal(TM)oxide)isexpectedtobedominatedbyO2pand Mn4scharacter.

Anothercross-sectioneffectthatmustbeallowedforasenergyincreasesisthe increasingimportanceofnon-dipoleterms[31, 32, 38](seealsochapterbySimon, Piancastelli,andLindle).Thesecanbebrokenintotwotypes,dependingon whethercore-likeintensitiesareinvolvedormomentum-resolvedangle-resolved photoemission(ARPES)valenceintensitiesarebeinganalyzed.Inthe firstcase, standardcorrectionparameterstotheusualdipoleformulaareavailablefora numberofatomsandenergies[31, 32, 38],andthesecanbeinterpolatedand extrapolatedforagivencaseathand.ForARPES,ormoreappropriately,soft-and hard-X-rayARPES(SARPESandHARPES,respectively),acorrectionduetothe photonmomentumisneededinthemomentum-conservationequation,asdiscussed elsewhere[15, 18, 19, 39, 40],laterinthischapter,andinthechapterbyGrayin thisbook.Thisisasimplecorrectiontodoaswell,providedthattheexperimental geometryispreciselydefined.

AsanothermorerecentlyrealizedaspectofhardX-rayphotoelectriccross sections,ithasrecentlybeenshownbyDrubeetal.thatinterchannelcoupling effectsthatareessentiallyresonantphotoemissionwithdeepcorelevelscansignificantlyinfluencetherelativeintensitiesofshallowercorelevels.Forexample,the Ag3d3/2,5/2 intensitiesatbindingenergiesof *374and368eVareinfluencedbyas muchas30%inscanningthephotonenergyovertheAg2p1/2,3/2 resonancesat *3560and3250eV.Thus,itmaybenecessarytoavoidsuchresonancesbyas muchasafewhundredeVifasimplequantitativeanalysisaccordingtoequations suchasthosein(1.1)or(1.2)istobevalid.

AsmoreandmorehardX-raybeamlinesarepermittingthevariationofpolarizationthroughtheuseofdiamondphaseretarders[41, 42],itisimportanttonote thestrongeffectsthatthiscanhaveonthedifferentialphotoelectriccrosssections. Thisisparticularlyimportantforvalence-levelspectrainwhichthedifferentorbital contributionscanberesolvedinenergythroughtheprojecteddensitiesofstates,as

indicatedin(1.2).Asasimpleillustrationofthis,Figs. 1.6 and 1.7 showsome calculateddifferentialcrosssectionsfortheCu3dz2 andO2pz orbitals,attwo energiesof0.8and5keVandthreedifferentpolarizationorientationsalongx,y, andz.Thesehavebeencalculatedinanon-relativisticlimitusingequationspublishedsometimeago[43],withextrapolationsofradialmatrixelementsandphase shiftsforthe ‘ ± 1interferingchannelsto5keVusingthedatain[43],andanonline programduetoNemšáketal.thatpermitscalculatingthemforanarbitrary experimentalgeometry[44].Ofcourse,foranyssubshellinthedipolelimit,the crosssectionlookslikeapwaveorientedalongthepolarizationdirection,thatisof thefunctionalformgivenattheleftofFig. 1.7,sincethereisonlyonechannelin the finalstate,andsowillhaveanodeforemissionperpendiculartothepolarization vectorforallthreeorientationsinthis figure.

Fromthesecalculationsformorecomplexnon-ssubshells,itisclearthatvarying polarizationawayfromthespecialcaseofbeingparalleltotheelectronemission

Fig.1.6 Non-relativisticdipole-approximationcrosssectionsfortheCu3dz2 orbital,forthree differentpolarizationdirectionsandat800and5000eVphotonenergies.Themaximumvalueof eachcontourisindicatedinthe inset (From[44])

direction(aspecialcasewhichisknowntoyieldcrosssectionsofexactlythesame formastheorbitalshapeangularshape[43]),canyielddramaticchanges.For example,theCu3dz2 crosssectiontendstolooksomewhatliketheorbitalforz polarization,butiscompletelydifferentinxandypolarization.Therearealsosome signifi cantchangesastheenergyisincreasedfrom800to5000,particularlyforx andypolarization.SimilarthingsaretruefortheO2pz crosssection,againtending tolookliketheorbitalforzpolarization,butchangingdramaticallysoastohave nodesalongtheorbitaldirectionwithxandypolarization.Althoughmoreaccurate relativisticcalculationsallowingfornon-dipoleeffectswouldnodoubtbesomewhatdifferentfromtheseresults,thechangeswithpolarizationandenergyin Figs. 1.6 and 1.7 wouldbeexpectedtobesemi-quantitativelymaintained. Asonesimpleillustrationoftheutilityofpolarizationinvalence-bandstudies,it ispointedoutininrecentpublications[42, 45],andinthechapterbyTjengetal., thatitcanbeusefultoemitelectronsperpendiculartothepolarizationdirectionin ordertosuppressthestrongTM4scontributionsinTMoxidevalencespectra,soas tomoredirectlyseethetransitionmetal3dcontributions(cf.Fig. 1.5).Figures 1.6

Fig.1.7 AsFig. 1.6,butfortheO2pz crosssections(From[44])

and 1.7 makeitclearthatotherpolarization-emissiongeometriescouldbeusefulin enhancingorde-enhancingthecontributionsofdifferentorbitals.Makinguseof suchvariationsincrosssectionswithpolarizationisofcoursealsoatypeoflinear dichroism,andwillbeaveryusefultechniqueinfutureHXPSstudies.

Finally,itisimportanttonotethatelasticscattering,asindicatedschematically inFig. 1.4,willminimallytendtosmearoutthevariousfeaturesseeninpurely atomiccrosssectionssuchasthoseinFigs. 1.6 and 1.7,butalsoforanyatomically orderedsystemtoproducestrongmodulationsduetophotoelectrondiffraction,as discussedfurtherbelow.

1.2.4ChemicalShifts,MultipletSplittings,andSatellites

inCore-LevelSpectra

Itisobviousthatcore-spectrainHXPScanbeminedforthesamekindsof informationasinsoftX-rayexcitedspectra:chemicalstatefromchemicalshifts, orbitaloccupationsandspinfrommultipletstructure,andlocalbondinginformation fromsatellites,whethertheyaredescribedasshake-upor final-statescreeningin nature.ButitwasrealizedearlyonbyHoribaetal.inworkonacolossalmagnetoresistivemanganite[46],subsequentlyinworkonhightemperaturesuperconductors[47]andlaterondilutemagneticsemiconductors(DMSs)[48]that goingtohigherenergiespermitsobservingextremelysharplow-binding-energy satellitesontransition-metal2pspectra,andthatthesecanbeinterpretedintermsof bulkscreeningbyhighlydelocalizedvalenceelectronsneartheFermilevel[49]. AnexampleofthiskindofdatafortheDMSGa0.97Mn0.03AsisshowninFig. 1.8, forwhichtheMn2p3/2 peakshowsaverystrongscreeningsatelliteofthistype. These final-stateeffectsprovideyetanotherhandleonvalenceelectronicstructure andhavebeenfoundtobesensitiveforexampletothepresenceofferromagnetic order[46, 48].ThespectruminFig. 1.8 alsoexhibitsamultipletsplittingfortheMn 3sspectrumthatcanbeusedtoestimatethespinonthisatom.These finalstate effectsandtheirinterpretationarereviewedinthechapterbyGrosvenoretal.and alsodiscussedinthechapterbyTaguchiandPanaccione.

1.2.5RecoilEffects

RecoileffectsinHXPSwere fi rstexploredbyTakataetal.[50],andarediscussed indetailinthechapterbyKayanuma.Theyhavebeenfoundtoaffectbothcore level-andvalencelevel-spectra[50–52],andmustbeconsideredassourcesofboth peakshiftstoeffectivelyhigherbindingenergies,andpeakbroadening.Figure 1.9b providesasimplewaytoestimatethemaximummagnitudeofthepeakshift,inthe simplestassumptionthatitisasingle-atomphenomenon.However,itisalsoclear

Mn2p “bulk” screening satellite linked to magnetism: Seen only in hard x-ray spectra

Mn3s splitting implies Mn3+ 3d4 (screening?)

Fig.1.8 SurveyspectrumfromthedilutemagneticsemiconductorGa0.97Mn0.03As(001)with 3.2keVexcitation,andwith enlargedinsets fromscanninglongeronMn2pand3s.Mn2preveals asharp final-statescreeningpeakonlyseenwithhardX-rayexcitation.Mn3sshowadoubletdue tomultipletsplittingthatcanbeusedtoestimatethespinofMn.DatafromSPring-8(From[40])

Fig.1.9 CalculatedparametersforestimatingthefeasibilityofARPESathigherenergies, including(a)contoursforvariousphotonenergiestoyieldaphotoemissionDebye-Wallerfactor W(T)of0.5at20K,and b therecoilenergyforallatomsasafunctionofphotonenergy.Values fortwo firstdemonstrationcasesWandGaAsstudiedwithhardX-rays[39]arehighlighted(From [55])

fromworktodatethatitisthedetailedvibrationalcouplingofagivenatomtoits nearneighborsthatcontrolsthemagnitudeoftherecoilshift[52],suggestingwhat hasbeencalled “recoilspectroscopy” asalocalprobeofsuchlocalbondingeffects, includingthoseinvalencespectra[53].

Aparticularlyilluminatingrecentexampleoftheobservationofrecoileffectsin gas-phaseHXPSisforNe1semission[54],forwhichthep-wavenatureofthe

crosssectionmeansthattheemissionofthephotoelectronsisstronglybiased towardbeingeitherparalleloranti-paralleltothepolarizationvector,asshownin Fig. 1.10a.ButifthephotoelectronsandthesubsequentKLLAugerelectronsare detectedalongthepolarizationasshown,thentheAugerelectronswillbeDoppler shiftedinenergydependingonthedirectionofemissionofthephotoelectrons.The turningonofthiseffectisseenasphotonenergyisincreasedintothe10keV regimeinFig. 1.10b.Sucheffectsarepresumablyalsopresentinsolidsaswell,and willbeasourceofbroadeninginAugerpeakwidthsathigherenergies.Thisand otheraspectsofHXPSinatomicandmolecularphysicsarediscussedinthechapter bySimon,Piancastelli,andLindle.

Asa finalcommentonrecoil,theuseofaDebye-Waller(D-W)factorto estimatetherecoil-freefractionforagivenexcitationisdirectlyrelatedtothe analysisofMössbauerspectra[50],andalsotothedegreetowhichHXPSvalence spectracanbeexpectedtoexhibitmomentum-resolvedelectronicstructurevia directtransitionsinangle-resolvedphotoemission(ARPES)[55].Figure 1.9ainfact permitsestimatingthefractionofmomentum-resolvedtransitionsasafunctionof Debyetemperature,atomicmass,andphotonenergy.WhentheD-Wfactorisvery small,onespeaksofbeingintheXPSlimitormorepreciselythe matrix-element-weighteddensityofstates(MEWDOS)limit.Thiscontinuum betweentheARPESlimitatlowtemperatureand/orlowenergyandtheXPSlimit athightemperatureand/orhighenergyisdiscussedfurtherbelow,inpriorpublications[18, 39, 40, 55],andinthechapterbyGray.

Fig.1.10 DopplereffectonAugeremissionfromafreeatom. a Thetwobasicrecoildirectionsof Ne1semissionrelativetothepolarizationdirectionsoftheincidentX-ray,dependingonwhether thephotoelectronisemittedtowardthespectrometerorawayfromit.Theexpectationforthis effectonasubsequentAugeremissionspectrumisindicatedinthe inset. b ActualAugerspectra forthetransitionNe+1 1s 1 → Ne+2 2p 2 (1D2)+Augerelectron,asphotonenergyisincreased. DatafromSoleil(From[54])

1.2.6CircularandLinearDichroism

Makinguseoflinearpolarizationtoaccentuatedifferentorbitalcontributionshasbeen discussedaboveundercrosssections,butbeyondthisisthewell-knownmagnetic circulardichroism(MCD)inmagneticsystems, fi rstobservedinsoftX-rayphotoemissionfromFebySchneideretal.[56],and firstobservedinHXPSfromFe3O4 and Zn-dopedFe3O4 byUedaetal.[57].TodistinguishphotoemissionMCDfromthe morecommonlypracticedX-rayabsorptionMCD(XMCD),itseemsworthwhileto designatethephotoemissionvariantasPMCD.Althoughthemuch-usedsumrulesof XMCDhavenosimpleanaloguesinPMCD,PMCDdatanonethelesspermits assessingmagneticorder,includingatburiedinterfaces.Linearmagneticdichroismin photoemission(PMLD)hasalsobeenmeasured,andoftenisreferredtowiththesuffix ADtodenotethatitismeasuredinangulardistributions.NotetoEditor:Thereare termsinthe figurecaptionthatInoticedarenotdefinedinthetext.SomePMCD resultsfromthe fi rstHXPSstudyarepresentedinFig. 1.11 [57],andinFig. 1.12c–e fromamorerecentstudyofaburiedlayerofCo2FeAl0:5Si0.5 [58].

PMCDhasinfactbeenusedinconnectionwithsoftX-raystanding-wave excitation(tobeintroducedbelow)toprobethedepthdistributionofmagnetic orderthroughburiedinterfacesofFe/Cr[59]andFe/MgO[60],andsuchmeasurementsshouldbepossiblewithhardX-rayexcitation.

1.2.7Spin-ResolvedSpectra

AddingthespindimensiontoHXPSisanobviousnextstepthatwouldincreasethe abilitytoprobemagneticsystemsenormously,and firstmeasurementsof spin-resolvedspectrahavealreadybeenmadeonthesameburiedlayercontaining Fe[58],asshowninFig. 1.12a–c.

Novelimagingspindetectors[61, 62]andothermoreefficientspindetectorsthat shouldbesuitableforHXPS[63]arealsobeingdevelopedthatpromiseafactorof *100,ifnotmore,inspeed,andsomeofthesearebeingimplementedforHXPS

Fig.1.11 HardX-ray photoemissionMCD(PMCD) forFe2pcore-levelemission froma10nm-thickFe3O4 film.DatafromSPring-8 (From[57])

Fig.1.12a–c Spin-resolvedspectraand d–e photoemissionMCD(PMCD)andMLD(PMLD) fromFe2p3/2 inaburiedlayerofCo2FeAl0:5Si0.5 with5.9keVexcitation. a Countratesinspin detectorchannels, b spinpolarizationderivedfromthecurvesin(a),and c spin-resolvedspectra. d–f Comparisonofspin-resolvedFe2p3/2 spectrawith(c)PMCDand(d),(e)PMLDfromthe samesample.DatafromSPring-8(From[58])

facilitiesatpresent.Thus,anexcitingelementoffuturestudieswillnodoubt involvemoreuseofspinresolution.

1.2.8PhotoelectronDiffraction

X-rayphotoelectrondiffraction(XPD)incore-levelemissionisawell-developed techniquefordetermininglocalatomicstructureinanelement-resolvedway,with over50,000citationsinaWebofSciencesearchbasedon “photoelectron diffraction”.VariousreviewsofXPDmakinguseofsoftX-rayexcitationhave appearedintheliterature[64–66].InthechapterbyChambers,heillustratestheuse ofXPDforcharacterizingoxideheterostructures.TheliteratureonhardX-ray photoelectrondiffractionismuchmorelimited,butgrowing,verymuchdueto Kobayashietal.[67].

Aninitialtheoreticalstudy[68]ofHXPDpointedoutthatthetraditional multiple-scatteringclustermodelforcalculatingXPD,asforexample,usedinthe onlineElectronDiffractioninAtomicClusters(EDAC)program[69]maynotbe themostrapidlyconvergentforHXPD,inwhichalargerno.ofatomscontribute

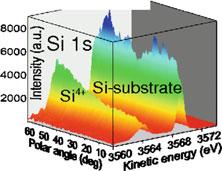
Fig.1.13 HardX-rayphotoelectrondiffractionfromSiwithvaryingthicknessesofSiO2 ontop andanexcitationenergyof5414.7eVfromamonochromatizedlaboratoryCrKα1 source.The spectrometerhereacceptedaverywideangleof *±40° a Si1sspectrafromaSi(001) single-crystalcoveredbyaSiO2 layerrecordedatacertainazimuth.Notethesingle-shotabilityto doARXPSfordepthprofiling. b–d Two-dimensionalHXPDpatternsofSi1satakineticenergy of3569eVfromaSicrystal b terminatedbyH, c coveredbya4.1-nm-thickSiO2 layerand d coveredbya7.0-nm-thickSiO2 layer.The dashedlines in b indicateKikuchibandsalongthe (110)and(111)planardirections. e Acalculatedpatternfromamultiple-scatteringcluster calculation.DatafromalaboratoryHXPSsystem(From[34])

duetothelargerIMFPs,andtheindividualelectron-atomscatteringeventsbecome muchmoreforwardpeaked,withthesecombinedeffectsleadingtodiffraction patternsmoreproperlyinterpretedasoverlappingKikuchibands[68].Thus,a dynamicaldiffractionapproachismoreappropriateinthehigh-energylimit.This priorstudypointedoutthepossiblesensitivityofHXPDtothesitetypeofanatom, e.g.asadopant,andthisisapromisingfuturedirectionforitsapplication.One preliminarystudyofthistypehasbeendone,forMninGaAs[70].

AsanexampleofHXPDresults,Fig. 1.13 showssomedatafromSiwithvarious thicknessesofSiO2 ontop,asobtainedfromalaboratorysystemusingCrKα excitationat5.4keV[71].Here,thedataarecomparedtoclustercalculations.Itis evidentthattheamorphousSiO2 overlayerattenuatesandsmearsouttheHXPD modulations,butthattheyarestillpresenttosomedegreeevenwith7nmof amorphousSiO2 ontop.AmuchmoredetailedsetofsuchdataforZnO,as comparedtobothclusteranddynamicaldiffractiontheory,ispresentedinFig. 18. 28 ofthechapterbyKobayashi.

H-Si(001)

4.1 nm SiO2 /Si(001)7.0 nmSiO2 /Si(001)Multiple scattering cluster simulation