Mathematics Teacher's Guide A K
About the Authors
Karren Schultz-Ferrell is a former elementary classroom teacher with teaching experiences ranging from 2-year-olds through Grade 5. Before retiring, she was an Elementary Integrated Instructional Specialist in Montgomery County Public Schools, MD, collaboratively aligning and writing the district’s mathematics curriculum with the Common Core State Standards in Kindergarten through Grade 5.
Karren is a mathematics consultant as well as co-author of Math Misconceptions, PreK-Grade 5: From Misunderstanding to Deep Understanding and Heinemann’s Math Process Standards series, Introduction to Reasoning and Proof (Grades PreK-2, Grades 3-5, and Grades 6-8).
She became involved in the PR1ME Kindergarten Math programme writing because she loves a challenge….and teaching math to young children! Karren is also part of the Problem Solving Programme authoring team.
Shara Sights Hammet has vast experience working in public, private and charter schools across the USA delivering professional development for superintendents, curriculum directors, principals, teachers and parents. Because of her work in such settings, potential schools and districts interested in Singapore content and pedagogy respect her direction and suggestions for their students and teachers.
Shara’s previous roles include Director of Singapore Math Professional Development, Product Manager of Math and Science Professional Development, Professional Development Trainer, contributing author, and classroom teacher.
Shara has traveled to Singapore to collaborate and create a series of quality professional development services for schools and districts adopting Singapore Math in the United States. Shara led the initiative to create a Professional Development Model that focuses on improving teacher effectiveness and student performance through the use of Singapore Math.
Shara is currently the Lower School Math Coach of Isidore Newman School in New Orleans, Louisiana. She works with teachers and administration each day to build consistency in best practices in mathematics instruction, oversees implementation of Singapore math curriculum, and leads professional development on key topics and trends. In addition, Shara serves in a consultancy and authoring role for Scholastic International, offering expertise on Singapore Math implementation in US classrooms.
Duriya Aziz S W PhD
Though a language specialist by training, math education found her and Dr Aziz has spent almost 20 years developing curriculum programs based on Singapore math pedagogical principles and practices for more than 20 countries, in different languages, and worked with ministries, schools and teachers on the implementation of these programs — particularly in the US, Australia, New Zealand, India, Latin America, and parts of Asia. In Singapore, she has worked on Ministry-led and developed projects for practically all subjects in the curriculum since the 1990s — in various capacities as editor, project director, and author. Dr Aziz has led the development of practically all the Singapore mathematics programs currently in use in the US. She has spent nearly twenty years working with teachers and districts in the implementation of the programs around the world, and observing the dynamic interplay between the Singapore mathematics pedagogy and the cultural and social contexts in which it has to operate. She is passionate about teachers’ continued professional learning and development and has led many seminars and workshops on diverse topics. Duriya’s focus has been on the development and implementation of programs that incorporate global best practices while remaining culturally and contextually appropriate, to drive sustainable change at a systemic level including development of teacher competence, knowledge, and independence.
About Mathematics
About Mathematics K
Welcome to Scholastic Mathematics K.
Scholastic Mathematics K is an innovative world-class mathematics program focused on the development of early numeracy and problem-solving skills, based on the effective teaching and learning practices of the global top-performers in Mathematics – Singapore, Republic of Korea and Hong Kong. The approach and instructional design are proven to be effective in developing conceptual mastery and procedural fluency, and are crafted to enable the teacher to continually evaluate learning and identify areas for remediation, if needed.
The content in Scholastic Mathematics K is presented under four strands of mathematics: Numbers and Operations, Measurement, Geometry and Data Analysis. There are two Teacher’s Guides, A and B, and accompanying Student Books, which are used consecutively. The Teacher’s Guide provides detailed lesson plans and embedded professional development to enhance teachers’ pedagogical knowledge, teaching and assessment. In addition, 20 Big Books and Readers, with accompanying Problem Solving Teacher’s Guide, have been developed to give teachers and students the opportunity to apply concepts learned to age-appropriate reallife scenarios, developing a problem-solving mindset.
Instructional Design
Scholastic Mathematics K is designed on a pedagogical model that ensures teaching and learning are effective, measurable and diagnostic.
Chapter
Each day’s teaching comprises four parts: Daily Warm-Up, Recall Prior Knowledge/ Quick Recall, Learn and Do and Daily Wrap-Up
Chapter Workout
Each Chapter ends with a Chapter Workout and Learning Center Activities that consolidate the concepts covered in the chapter.
The Problem Solving Big Books and Readers are intended to be used for a lesson after the Chapter Workout and Learning Center Activities, so students can see how the concepts they have learned can be applied in engaging characterled contexts.
Using the Teacher’s Guide
Scholastic Mathematics K Teacher’s Guides are designed to help you, the teacher, implement the program easily and effectively.
• Plan effectively with an overview of concepts and skills taught in each lesson, details of each day’s objectives and the materials and resources required, as well as detailed guidance for every day’s lesson.
• Deliver high quality lessons with the help of detailed lesson plans that explain the pedagogy and methodology for teaching each concept, thus deepening your conceptual understanding and equipping you to deliver lessons with confidence.
• Identify remediation needs via ongoing formative assessment during class activities and individual practice work. Reteach suggestions are included to help ensure all students achieve mastery.
This Teacher’s Guide includes
• Developmental Continuum showing how students progress from Kindergarten to Grades 1 and 2.
• detailed Scheme of Work
• scripted Lesson Plans
• answers for all tasks
• photocopiables for class activities
Plan
The Developmental Continuum appears at the beginning of the Teacher’s Guide. It offers the overall plan for learning outcomes from Kindergarten up to Grade 2. Teachers can refer to this to understand how the Kindergarten chapters provide the foundation for 1-6.
Strands are color coded to help teachers relate different topics.
Numbers and Operations Measurement Geometry Data Analysis
The Scheme of Work at the start of each chapter is designed to assist in planning the curriculum for the entire year and to prepare for teaching individual chapters.
Each day’s teaching represents 1 hour of instruction. Recommended pacing for each unit helps you manage your time effectively. Teachers can adjust the pacing based on their school calendar and the pace of individual classes.
The objectives list states what will be taught in each unit.
Materials shows what teachers will need to teach each lesson in the unit.
Resources refers teachers to the pages students will use in their Student Books as well as teaching resources found in the Teacher’s Guide.
New vocabulary
Each chapter begins with an Introduction which identifies the key mathematical ideas of the chapter, outlining what students will learn as they work through the chapter and how this lays the foundation for future mathematical concepts.
Teach
Daily Warm-Up
Each day’s teaching starts with a quick five minute routine designed to build mathematical fluency and confidence. Routines include:
• Rote Counting: includes counting from a given number other than 1, a foundational concept for counting on, and grows in difficulty throughout the school year.
• Data Collection: reinforces mathematics vocabulary, builds number sense and reinforces number concepts.
• Subitizing: strengthens students’ abilities to develop mental images of numbers which helps build fluency and strengthen their understanding of the part-whole concept.
• Comparison Jar: reinforces the comparison of two or three numerals to determine the numeral that is greatest or least and encourages use of objects and number tapes to support students’ thinking and reasoning.
• Number Jar: supports students’ abilities to make predictions and discuss results and begins to develop students’ understanding for magnitude of number.
Check for Readiness to Learn
Recall Prior Knowledge and Quick Recall require students to remember prerequisite knowledge. Each task is carefully crafted to check for readiness to learn new knowledge before new concepts are introduced.
Recall Prior Knowledge recaps teaching done in an earlier chapter in the program.
Quick Recall reminds students of concepts covered in the previous day’s lesson and assesses their readiness to move on.
Teacher’s Guide
Learn and Do: Developing Conceptual Understanding
Each chapter is taught over several lessons, with each lesson focusing on a concept or part of it. Some lessons may be taught in a single day, while others are broken down into several days’ instruction. Each day’s teaching follows a two-part structure of concept introduction in Let’s Learn and guided practice and formative assessment in Let’s Do
Concepts in Let’s Learn are taught using the three-stage Concrete-Pictorial-Abstract approach to develop deep conceptual understanding. Clear instructions are provided for directing students’ learning through each stage.
Teacher’s Guide
Purposeful questions are suggested in the Teacher’s Guide, and students are engaged in communicating, explaining and reflecting on their answers.
New concepts are frequently introduced with a hands-on activity. This is the concrete stage of the learning journey. Students work in groups or in pairs to participate in concrete mathematical experiences. The teacher guides enquiry – leading students to explore, investigate and find answers on their own.
Icons indicate the stages of the gradual release of responsibility model of instruction.
: whole class
: small group
: pair work
: independent work
In the pictorial stage, students are guided to understand mathematical ideas presented visually via the Student Book. This middle stage is a crucial link between the concrete experience and the abstract representation and serves to build a solid foundation.
Once conceptual understanding is developed, progress to the abstract stage where the concept or skill is represented using numbers and mathematical symbols.
Let’s Do reinforces students’ learning and builds mastery through guided, systematically-varied and child-friendly tasks. Tasks are crafted to allow for valuable and immediate feedback on whether students have progressed through the three-stage approach and mastered the concept or if re-teaching is required.
Teacher’s Guide
After a concept is taught in Let’s Learn, assign Let’s Do tasks for students to complete individually. Discuss students’ responses and remediate if necessary. The objective of each Let’s Do task is clearly stated, enabling teachers to check students’ understanding. Answers are provided for all tasks.
Reteach provides task-specific remediation to address gaps in understanding.
The Daily Wrap-Up helps students realize how much learning has taken place and solidify learning for future lessons. This is a crucial step to help students remember and apply the information they have learned.
Consolidate
The Chapter Workout and Learning Center Activities provide a one hour lesson at the end of each chapter and serve to consolidate concepts learned in the chapter. Teachers are provided with questions to guide the lesson and to encourage learners to talk about the concepts behind the Chapter Workout and assess understanding.
Each Chapter Workout consists of a picture for class discussion which is followed by three learning center tasks.
Activity 1 is a Think, turn and talk activity, intended to promote pair discussion, challenge common misconceptions and strengthen mathematical thinking.
Activity 2 is a game, providing a motivating context for students to practice what they have learnt and Activity 3 is an individual activity, ideal for formative assessment.
The Big Books and accompanying materials are designed to be used for a one-hour lesson after completing each chapter. More details are provided in the Problem Solving Teacher’s Guide.
Developmental Continuum
Year/Grade K
NUMBERS AND OPERATIONS
Whole Numbers / Place Value
Rote count within 100 by 1s and 10s.
Read and write 0 to 10 - the numeral and the corresponding number word.
Identify the last number counted as the number of objects in the group.
Count on and backwards within 10.
Count groups of up to 20 objects in different arrangements.
Compare the number of objects in two groups.
Compare and order numbers within 20.
Year/Grade 1
Year/Grade 2
Count within 100. Count within 1000.
Read and write a number from 0 to 100 — the numeral and the corresponding number word.
Count on and backwards within 100.
Use number notation and place values (tens, ones).
Estimate the number of objects in a group of fewer than 100 objects.
Compare the number of objects in two or more groups.
Compare and order numbers within 100.
Understand that the number that comes next is 1 more. Find the number which is 1 or 10 more than (or less than) a given number within 100.
Break apart 4 to 10 objects into two parts.
Make a number story to illustrate a number bond for 5 to 10.
Make 4 to 10 with two parts.Write a number bond for 5 to 10.
Write a number bond for 4 to 10.
Use ordinal numbers 1st to 10th to indicate position.
Estimate the number of objects in a group of fewer than 10 objects.
Subitize up to 6 objects. Compose and decompose numbers from 10 to 19 as 10 ones and some more ones.
Name a position using an ordinal number from 1st to 10th and position words.
Read and write a number from 0 to 1000 — the numeral and the corresponding number word.
Use number notation and place values (hundreds, tens, ones).
Compare and order numbers within 1000.
Use the symbols ‘>’ and ‘<’ for comparison of numbers.
Find the number which is 1, 10 or 100 more than (or less than) a given number within 1000.
Addition / Subtraction
Add or subtract within 10.Use picture cutouts (or other manipulatives) to illustrate the meanings of addition and subtraction.
Add or subtract within 1000.
Year/Grade K
Year/Grade 1
NUMBERS AND OPERATIONS (continued)
Addition / Subtraction (continued) Act out addition and subtraction stories to illustrate the meanings of addition and subtraction.
Make addition or subtraction stories with the given illustrations.
Count all to add two quantities within 10.
Illustrate addition and subtraction stories and problems with number bonds.
Use drawings to represent addition and subtraction stories.
Make a number story for a given addition or subtraction sentence.
Write a number sentence for a given situation involving addition or subtraction.
Observe the identity and commutative properties of addition.
Observe the answer when 0 is subtracted from a number.
Write a family of four addition and subtraction facts for a given number bond.
Write addition and subtraction facts within 5.
Count on to add or count backwards to subtract within 10.
Write a number sentence for an addition or subtraction problem.
Year/Grade 2
Use a part-whole bar model or a comparison bar model to represent an addition or subtraction situation.
Solve up to 2-step word problems involving addition and subtraction.
Find the missing part in an addition sentence.
Find the missing part or whole in a subtraction sentence.
Mentally add: - a 1-digit whole number to a 2-digit whole number with regrouping
- two 2-digit whole numbers without regrouping
- ones, tens or hundreds to a 3-digit whole number
- 98 or 99 to a number up to 3 digits
Identify a doubles fact.Mentally subtract:
- a 1-digit whole number from a 2-digit whole number with regrouping - a 2-digit whole number from another 2-digit whole number without regrouping
- ones, tens or hundreds from a 3-digit whole number
- 98 or 99 to a 3-digit whole number
Add or subtract within 100.
Solve a 1-step word problem involving addition or subtraction of numbers within 20.
Mentally add:
- two or three 1-digit whole numbers
- a 1-digit whole number to a 2-digit whole number
- tens to a 2-digit whole number
Mentally subtract: - a 1-digit whole number from another 1-digit whole number
- a 1-digit whole number from a 2-digit whole number
- tens from a 2-digit whole number
Year/Grade K
Year/Grade 1
NUMBERS AND OPERATIONS (continued)
Multiplication / Division
Year/Grade 2
Recognize equal groups and find the total number in the groups by repeated addition.
Use mathematical language such as ‘4 threes’ and ‘2 groups of 5’ to describe equal groups.
Use manipulatives to illustrate the meaning of multiplication and the sharing and grouping concepts of division.
Make a number story for a given multiplication sentence.
Write a number sentence for a given situation involving multiplication or division.
Work out a multiplication fact within 40 by repeated addition.
Solve a 1-step picture problem involving multiplication or division.
Write a family of four multiplication facts and division facts.
Count by twos, threes, fours, fives and tens.
Write an addition double as a multiplication fact.
Observe the commutative and distributive properties of multiplication.
Build up the multiplication tables of 2, 3, 4, 5 and 10 and commit the multiplication facts to memory.
Use a related multiplication fact to divide.
Multiply or divide numbers within the multiplication tables of 2, 3, 4, 5 and 10.
Use a part-whole bar model to represent a multiplication or division situation.
Solve a 1-step word problem involving multiplication or division using the multiplication tables of 2, 3, 4, 5 and 10.
Year/Grade K
Year/Grade 1
NUMBERS AND OPERATIONS (continued)
Fractions / Concepts
Recognize and name one half of a whole which is divided into 2 equal parts.
Recognize and name one quarter of a whole which is divided into 4 equal parts.
MEASUREMENT
Length
Describe an object using ‘long’, ‘tall’ and ‘short’.
Compare the lengths of two or more objects.
Compare up to 3 objects.Arrange objects in order according to their lengths.
Measure using up to 10 nonstandard units.
Estimate and measure the length of an object in nonstandard units.
Year/Grade 2
Recognize and name a unit fraction up to 1 12
Recognize and name a fraction of a whole which is divided into equal parts.
Find the fraction that must be added to a given fraction to make a whole.
Compare and order unit fractions.
Mass Describe an object using ‘heavy’ and ‘light’.
Compare the masses of two objects.
Compare up to 3 objects.Compare and order the masses of three objects.
Explore mass by hefting.Estimate and measure the mass of an object in nonstandard units.
Measure with a pan balance using up to 10 nonstandard units.
Explore estimation.
Understand a big object is not necessarily heavier than a smaller one.
Size Describe an object using ‘big’ and ‘small’.
Compare up to 3 objects.
Understand the need for standardized units of measure of length.
Understand that a meter is greater than a centimeter.
Estimate and measure length in meters or centimeters.
Compare lengths in meters or centimeters.
Choose an appropriate unit of measure when measuring lengths.
Measure the length of a line segment in centimeters.
Draw a line segment of a given length.
Solve up to 2-step word problems involving length.
Understand the need for standardized units of measure of mass.
Measure mass in kilograms or grams.
Compare masses in kilograms or grams.
Solve up to 2-step word problems involving mass.
Year/Grade K
MEASUREMENT (continued)
Volume Compare the volume of liquids in 2 identical containers.
Time: Calendar
Name the days of the week in sequence.
Link specific days to familiar events.
Name the months of the year in sequence.
Link familiar events to months.
Count the days in a month.
Sequence events in order of ‘morning’, ‘afternoon’ and ‘night’.
Measure and compare short periods of time in informal ways and using ‘longer ‘, ‘shorter’, ‘faster’ and ‘slower’.
Time: Clock
Money
Recognize and name the one-cent, five-cent, tencent, twenty-cent and fifthcent coins.
Count and tell the amount of money up to twentycents in one-cent coins.
Compare two amounts of money between one and ten cents.
Add or subtracts amounts of money: - in one-cents up to 10 cents and represent the result with drawings.
Year/Grade 1
Year/Grade 2
Read a calendar. Know the number of days in a month and in a year.
Name the days of the week.Understand the relationship between 1 hour, 1 day, 1 week, 1 month and 1 year.
Name the months of the year.
Read and write a date.
Tell time to the hour and half hour.
Relate time to events of a day.
Sequence events according to the time of the day.
Tell and write time to 5 minutes.
Use a.m. and p.m. in telling time.
Relate time to events of a day.
Describe estimated time relative to the hour and half past the hour. Find the duration of a time interval.
Compare durations using ‘longer’ or ‘shorter’.
Recognize and name the five-cent, ten-cent, fifty-cent and onedollar coins.
Recognize and name the five-dollar, ten-dollar and fifty-dollar notes.
Count and tell the amount of money in:
- a set of coins up to $1 - a set of notes up to $100
Develop a sense of the duration of daily activities.
Solve word problems involving time.
Count and tell the amount of money in a set of notes and coins.
Read and write an amount of money in decimal notation.
Change dollars and cents to cents, and vice versa.
Exchange money. Compare amounts of money in dollars and cents.
Year/Grade K
MEASUREMENT (continued)
Money (continued)
Year/Grade 1
Count and tell the amount of money in a set of notes and coins.
Make up an amount of money using a set of notes and coins.
Compare amounts of money in dollars or cents.
Add or subtract amounts of money: - in cents up to $1 - in dollars up to $100
GEOMETRY
Plane Shapes
Recognize and name five basic plane shapes: circle, triangle, rectangle, square and hexagon.
Count the sides and corners of a shape.
Build awareness of attributes: shape, size, and color.
Sort and group (and re-sort) according to attributes: shape, size and color.
Describe the location of plane shapes using positional and directional words.
Identify plane shapes on real-world objects.
Use plane shapes to create a new shape, and items commonly found in the environment.
Solid Shapes
Recognize and name basic solid shapes: sphere, cylinder, cone and cube.
Describe the attributes of solid shapes: slide, stack and roll.
Year/Grade 2
Exchange money.
Give change for a purchase paid with $1.
Solve a 1-step word problem involving money.
Recognize and name the four basic plane shapes: circle, triangle, rectangle and square.
Describe an object by its shape.
Count the sides and corners of a shape.
Classify a plane shape according to each of these attributes: shape, size and color.
Continue a pattern of plane shapes according to one or two of these attributes: shape, size and color.
Fit suitable pieces together to make a plane shape.
Identify a semicircle and a quarter circle.
Identify and name basic shapes that make up a new shape.
Fit cutouts of shapes together to make a new shape.
Copy a figure on a dot grid or square grid.
Continue a pattern of plane shapes according to one or two of these attributes: shape, size, color and orientation.
Recognize and name a cube, cuboid, cone, cylinder and sphere.
Classify solid shapes according to each of these attributes: shape, size and color.
Identify the flat and curved surfaces of a solid object in the shape of a cube, cuboid, cone, cylinder or sphere.
Identify the faces, edges and vertices of a solid object in the shape of a cube, cuboid, cone, cylinder or sphere.
Year/Grade K
GEOMETRY (continued)
Solid Shapes (continued)
Identify real-world objects as solids.
Describe the location of solid shapes using positional and directional words.
Identify plane shapes on solid shapes.
Use solids to build and compare two structures.
Patterns Identify visual patterns in the environment.
Describe, copy and extend AB, ABC and AAB shape, sound and action patterns.
Transfer patterns to a different format.
Line Segments
DATA ANALYSIS
Tally Charts
Year/Grade 1
Identify a solid shape that can slide, stack or roll.
Describe the relative position of a solid shape using position words.
Continue a pattern of solid shapes according to one or two of these attributes: shape, size and color.
Year/Grade 2
Use solid shapes to form different solid figures.
Continue a pattern of solid shapes according to one or two of these attributes: shape, size, color and orientation.
Graphs
Sort and group up to 10 objects by color or pattern.
Participate in a class survey to collect data.
Sort and count data to create a 3-column or 3-row picture graph of up to 10 objects per category.
Visually compare data.
Classify objects or people by a predetermined standard and count the numbers in each category.
Make and read a tally chart.
Make a simple picture graph using one-to-one representation.
Read and interpret a picture graph.
Identify a line segment and a curve.
Use a tally chart to gather data and represent data in a picture graph.
Use a tally chart to gather data and represent data in a picture graph.
Make a picture graph with scale.
Read and interpret a picture graph with scale.
Solve problems using data presented in a picture graph.
Counting Small Numbers
Scheme of Work
Lesson Objectives
Lesson 1: 1 and 2
Counting and Number Names for 1 and 2
Lesson 2: 1, 2 and 3
Counting and Number Names for 1, 2 and 3
Counting 1, 2 and 3
Lesson 3: 4 and 5
Counting and Number Names for 4 and 5
• Count groups of 1 and 2 objects
• Identify the last number counted as the number of objects in the group
• Read and write 1 and 2 in numeral form and recognize their number names
• Count groups of 1, 2 and 3 objects arranged in a line
• Identify the last number counted as the number of objects in the group
• Read and write 1, 2 and 3 in numeral form and recognize their number names
Count up to 5 with a 5-frame
Lesson 4: 1 to 5
Counting Objects Arranged in Different Ways
• Count groups of 4 and 5 objects arranged in a line
• Identify the last number counted as the number of objects in the group
• Read and write 4 and 5 in numeral form and recognize their number names
• Count up to 5 objects arranged in different ways (in a line, 5-frame, array, circular, scattered)
• State the number of objects in a counted set of up to 5 objects without recounting
Counting from a Set
• Count out a given number of up to 5 objects from a larger set of up to 8 objects
Total Duration:
10
Days
(1 day = 1 hour)
• Connecting cubes (1 or 2 in each bag), 6 bags
• Card paper, 1 sheet
• 10-cm strip of paper, 1 per pair
• Connecting cubes (1 or 2 in each bag), 1 bag per pair
• 10-cm strip of paper, 1 per pair
• Cups of 1, 2 or 3 counters, 1 cup per pair
• 3 paper clips, 1 envelope per pair
• Connecting cubes (3 red, 3 blue and 3 yellow), 1 set per pair
• Chart paper
• 10-cm strip of paper, 1 per pair
• Bags of 1–5 connecting cubes, 1 bag per pair
• SB pp. 1–4
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• SB p. 5
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• Number-Dot Card ‘3’ (TR 1.3)
• SB pp. 6–8
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• Number-Dot Card ‘3’ (TR 1.3)
• Number-Dot Cards ‘1–3’ (TR 1.1–1.3), 1 set per pair
• SB pp. 9–10
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• Number-Dot Card ‘3’ (TR 1.3)
• Number-Dot Card ‘4’ (TR 1.4)
• Number-Dot Card ‘5’ (TR 1.5)
one • two
2 days
three
• Sticky notes (each with a number 1, 2, 3, 4 or 5), 1 sticky note per pair
• Counters
• 5 connecting cubes, 1 cup per pair
• Counters
• Paper, a few sheets per student
• Pencils
• 8 counters, 5 sets
• Double-sided tape
• Paper, 5 sheets
2 days
• Number-Dot Cards ‘1–5’ (TR1.1–1.5), 1 set per pair • four • five
• SB pp. 11–13
• 5-frame (TR 1.6), 1 per pair
• Number-Dot Cards ‘1–5’ (TR 1.1–1.5), 1 set per pair
• SB pp. 14–15
• Number-Dot Cards ‘1–5’ (TR 1.1–1.5)
• 5-frame (TR 1.6)
• Picture Cards: 2 Objects in Different Arrangements (TR 1.7)
• Picture Cards: 3 Objects in Different Arrangements (TR 1.8)
• Picture Cards: 4 Objects in Different Arrangements (TR 1.9)
• Picture Cards: 5 Objects in Different Arrangements (TR 1.10)
• SB pp. 16–17
• Number Cards 2–5 (TR 1.11), 1 set per pair
• SB pp. 18–20
• 5-frame (TR 1.6), 5 copies
• Number Cards 1–5 (TR 1.11)
3 days
Lesson Objectives
Chapter Workout and Learning Center Activities
Chapter Workout
• Count up to 5 objects arranged in different ways (in a line, array, circular, scattered)
• Identify the last number counted as the number of objects in the group
• State the number of objects in a counted set of up to 5 objects without recounting
• Read and write 1 to 5 in numeral form and recognize their number names
• Counters (optional)
• Counters, 5 per pair
• Paper clips (different colors), 2 per pair
• SB p. 21
• 5-frame (TR 1.6), 1 per student (optional)
• SB p. 22
• SB p. 23
• Number Cards 1–5 (TR 1.11), 1 set per student
• SB p. 24
• Problem Solving Big Book 1 and Reader 1
• Problem Solving Teacher's Guide
1 day
1 day
Counting Small Numbers
Chapter Overview
Lesson 1: 1 and 2
Lesson 2: 1, 2 and 3
Lesson 3: 4 and 5
Lesson 4: 1 to 5
Introduction
In this chapter, students will count sets of up to 5 objects arranged in different ways. They begin to develop understanding about cardinality, that is, the concept that the last number counted also represents the total number of objects in the set. Students will be able to read and write numbers 1 to 5, recognize each numeral’s word, and match the numerals with their quantities.
Students will learn rote-counting routines in their Daily Warm-Up activities, which continue throughout the year with varying counting benchmarks (counting orally by 1s from 1–5, 1–10, 1–15, 1–20, 1–31, 1–50, 1–100 and by 10s to 100). Fluent oral counting skills will help students successfully engage in other counting concepts, such as one-to-one correspondence, keeping track, cardinality and conservation of number. These counting concepts enable students to eventually count with meaning.
Counting lays the foundation for building students’ understanding about number sequence (cardinal and ordinal) and number comparison, and will prepare them for learning measurement, addition and subtraction, as well as place value and money concepts in later chapters.
Lesson 1: 1 and 2 DAY 1
Objectives:
• Count groups of 1 and 2 objects
• Identify the last number counted as the number of objects in the group
• Read and write 1 and 2 in numeral for m and recognize their number names
Materials:
• Connecting cubes (1 or 2 in each bag), 6 bags
• Card paper, 1 sheet
Resources:
• SB pp. 1–4
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
Vocabulary:
• one
• two
Daily Warm-Up
Objective: Rote count by 1s from 1 to 5
Have students stand in a circle. Count from 1 to 5 and have them repeat after you. Repeat 2–3 times until students are fairly familiar with the numbers 1 to 5.
Then, have students take turns in the circle and rote count by 1s from 1 to 5 (each student says one number). The student who says 5 sits down. The next student begins counting from 1 again. Repeat this several times.
Observe:
• Does a student skip or repeat any numbers? If so, which ones?
• Does a student count in an incorrect sequence, e.g. 1, 2, 4, 3, 5?
• Does a student use incorrect number names?
Learn and Do
Counting and Number Names for 1 and 2
Hold up 1 finger on your hand.
Say: I am holding up 1 finger. 1.
Ask: How many fingers am I holding up? (1)
Hold up 2 fingers. Point at each finger as you count.
Say: I am holding up 2 fingers. 1, 2.
Ask: How many fingers am I holding up? (2)
Say: Hold up 1 arm. Hold up 2 arms.
Repeat with other actions, e.g. clapping once or twice, to familiarize students with the number names ‘one’ and ‘two’, and with counting to 2.
Have students sit in a circle. Place the bags of connecting cubes and the sheet of card paper in the middle of the circle. Explain that each bag has 1 or 2 connecting cubes. Have a student choose a bag, take out the cube(s) and place the cube(s) on the card paper. As the student places the cube(s) on the card paper, have the rest of the class count aloud with you for each cube.
Say: This bag has 1 (or 2) cubes. Set the bag aside. Have another student choose another bag and repeat the procedure until all the bags are used.
Let’s Learn SB pp. 1–2
Show students Number-Dot Card ‘1’ (TR 1.1). Point to the dot.
Ask: How many dots are there? (1)
Point to the number 1 on the Number-Dot Card.
Say: We write ‘one’ like this. This is the number 1.
Trace the 1 on Number-Dot Card ‘1’. Have students trace the number 1 in the air.
Write ‘1’ and ‘one’ on the board and read them aloud. Then, draw an object to show the quantity represented by the number.
Say: Point to the number 1 anywhere in our classroom. (on the clock etc.)
Then, have students hold up one object they can find on their desks or in their bags, e.g. 1 book, 1 pencil etc. Repeat this procedure for the number 2 using Number-Dot Card ‘2’ (TR 1.2)
Have students name the number on SB p. 1. Then, name the objects around it, e.g. 1 butterfly, 1 flower.
Counting Small Numbers
Repeat this instructional procedure with the number 2 on SB p. 2.
Let’s Do SB pp. 3–4
Task 1 provides practice in counting and matching the number of objects to its corresponding numeral. An example is provided.
Task 2 provides practice in counting the number of objects and writing the numerals. Guidance is provided.
NOTE: Taking out the cubes one at a time models one-to-one correspondence while stating the total quantity counted develops students’ understanding of cardinality, that counting a quantity to its number name also refers to its total. As the quantities are small, students may also be able to subitize and quickly identify the number of cubes.
Daily Wrap-Up
Point to the numeral 1 on Number-Dot Card ‘1’.
Ask: What number is this? (1)
What do you know about this number? (I start counting with 1.)
Point to the numeral 2 on Number-Dot Card ‘2’.
Ask: What number is this? (2)
What do you know about this number? (I count 1 first and then I count 2.)
Objectives:
• Count groups of 1, 2 and 3 objects arranged in a line
• Identify the last number counted as the number of objects in the group
• Read and write 1, 2 and 3 in numeral for m and recognize their number names
Materials:
• 10-cm strip of paper, 1 per pair
• Connecting cubes (1 or 2 in each bag), 1 bag per pair
Resources:
• SB p. 5
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• Number-Dot Card ‘3’ (TR 1.3)
Vocabulary:
• three
Lesson 2: 1, 2 and 3
Daily Warm-Up
Objective: Rote count by 1s from 1 to 5
Have students take turns in a circle and rote count by 1s from 1 to 5 (each student says one number). The student who says 5 sits down. The next student begins counting from 1 again. Repeat the activity several times.
Quick Recall
Revisit Number-Dot Cards ‘1’ and ‘2’ (TR 1.1–TR 1.2). Have students trace each number in the air and clap the corresponding number of times.
Ask: I have 2 eyes. What do you have 2 of? (feet, arms, hands etc.)
Ask: Do you see 2 of something in our classroom? Have pairs identify 2 objects in the classroom and share their findings with the class.
Learn and Do
Counting and Number Names for 1, 2 and 3
Show students the bags of connecting cubes and the strip of paper Say: We can put our cubes on a strip of paper so that we can count them easily.
Model how to count the connecting cubes using the strip of paper. Take the cubes, one at a time, from a bag and place them onto the paper. Have students count with you and ask "How many cubes did we count?"
NOTE: The counting strip is a strip of plain paper students use to count. Placing the cubes on the strip of paper one at a time reinforces one-to-one correspondence. Having students count with you and asking them "How many?" reinforces the concept of cardinality.
Stick the Number-Dot Cards '1' and '2' (TR1.1–1.2) on the board. Distribute bags of 1 or 2 cubes and a strip of paper to each pair. Have students count out the number of cubes in their bag using the strip of paper. Ask "How many?". Then, have students stand in front of their respective Number-Dot Cards '1' and '2'. Redistribute the bags of cubes and repeat the activity.
Arrange 6 students in lines of 1, 2 and 3 students. Count the number of students in each line and state the total number. Point to each student in the line as you count aloud with the rest of the class.
NOTE: Pointing to each student models one-to-one correspondence. Stating the total and asking the ‘How many?’ question reinforces students’ understanding of cardinality.
Have another group of 6 students form additional lines of 1, 2 and 3. Ask: There is 1 friend in this line. How many friends are in this other line?
Point to the next line.
Ask: How many friends are in this line?
Then, have students form a line with 2 people. If there is an odd number of
students in the class have the remaining student form a line with you. Then, have each student in each line number themselves by counting 1 and 2.
Then, have students form a line with 3 people and repeat the procedure of counting from 1 to 3.
Let’s Learn SB p. 5
Show students Number-Dot Card '3' (TR 1.3)
Trace the number 3 on Number-Dot Card ‘3’. Have students trace the number 3 in the air.
Write ‘3’ and ‘three’ on the board and read them aloud. Then, draw 3 worms to show the quantity represented by the number.
Say: This is the number 3.
Ask: Do you see the number 3 anywhere in our classroom?
Provide time for students to look and share where they find the number 3. (the clock, a number tape, on a storage box etc.)
Refer students to SB p. 5. Have students look at the number 3 and name the objects around it, e.g. 3 stones, 3 twigs.
Lesson 2 1 , 2 and 3
Daily Wrap-Up
Point to the numeral 3 on Number-Dot Card ‘3’.
Ask: What number is this? (3) Hold up 3 fingers. Have students count the number of fingers you held up.
Objectives:
• Count groups of 1, 2 and 3 objects arranged in a line
• Identify the last number counted as the number of objects in the group
• Read and write 1, 2 and 3 in numeral for m and recognize their number names
Materials:
• 10-cm strip of paper, 1 per pair
• Cups of 1, 2 or 3 counters, 1 cup per pair
• 3 paper clips, 1 envelope per pair
• Connecting cubes (3 red, 3 blue and 3 yellow), 1 set per pair
• Chart paper
Resources:
• SB pp. 6–8
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• Number-Dot Card ‘3’ (TR 1.3)
• Number-Dot Cards ‘1–3’ (TR 1.1–1.3), 1 set per pair
Lesson 2: 1, 2 and 3
Daily Warm-Up
Objective: Rote count by 1s from 1 to 5
Have students take turns in a circle and rote count by 1s from 1 to 5 (each student says one number). The student who says 5 claps his/her hands once and steps out from the circle. Repeat the activity a few times.
Quick Recall
Hold up Number-Dot Card ‘1’ (TR 1.1)
Ask: What number is this? (1) How do you know it is 1? (There is 1 dot on the card.)
Say: Show me 1 arm. Show me 1 hand.
Repeat the activity with Number-Dot Cards ‘2’ and ‘3’ (TR 1.2 and TR 1.3) with similar questioning.
Learn and Do
Counting 1, 2 and 3
Have students work in pairs to count out and build a tower of 3 red connecting cubes.
Ask: How many cubes are there? (3) Say: Now build a tower with 2 colors. When students are done, ask them how many cubes they have. They should have 3 cubes.
Ask: Can you use 3 colors to build your tower? Remind students that their tower can only have 3 cubes.
Have a student help you model the next activity. Place the strip of paper and a set of Number-Dot Cards ‘1–3’ (TR 1.1–1.3) on a table. Have the student choose a cup (containing up to 3 counters) while you keep the envelope with 3 paper clips.
Explain that one partner will count out the number of counters in the cup using the counting strip. Then, he/she will choose the Number-Dot Card that matches the number of counters. The other partner will check that the correct Number-Dot Card was chosen before counting out the same number of paper clips from the envelope, placing them on the counting strip and saying the number of both items, (e.g. 2 counters. 2 paper clips). Partners will switch roles and repeat the procedure.
Have students work in pairs to continue the activity. Distribute a counter-cup, a paper clip-envelope, a counting strip and a set of Number-Dot Cards ‘1–3’ to each pair. Once the pair has completed the activity, have them exchange their cup of counters with another pair and repeat the activity. DAY 1
NOTE: Representing the same quantity with different manipulatives supports students’ understanding about conservation.
Observe:
• Do students use one-to-one correspondence to count out the correct number of objects?
• Do students use an incorrect counting sequence? If so, where is his/her error?
• Can students subitize the quantities?
• Can students state the total quantity counted without recounting?
• Do students choose the correct Number-Dot Card to match a quantity?
Let’s Do SB pp. 6–8
Task 1 provides practice in counting the number of objects in a set and matching it to its corresponding numeral.
Task 2 provides practice in counting the number of objects in a set and writing the corresponding numerals. Guidance is provided.
Task 3 provides practice in counting the number of objects in a set and writing the corresponding numerals. An example is provided.
1 3 3 2 2
Reteach
Have students count out 1, 2 and 3 counters using a counting strip and ask them the ‘How many?’ question. Refer them to the Let’s Do section on SB pp. 6–8 and have students point to each object in a given set as they count. After each set is counted, ask the ‘How many?’ question.
Daily Wrap-Up
Write the numbers 1, 2 and 3 on chart paper. Then, write the number names below each number. As you point to each number, have students read the numbers aloud.
Objectives:
• Count groups of 4 and 5 objects arranged in a line
• Identify the last number counted as the number of objects in the group
• Read and write 4 and 5 in numeral for m and recognize their number names
Materials:
• 10-cm strip of paper, 1 per pair
• Connecting cubes (1–5 cubes in each bag), 1 bag per pair
Resources:
• SB pp. 9–10
• Number-Dot Card ‘1’ (TR 1.1)
• Number-Dot Card ‘2’ (TR 1.2)
• Number-Dot Card ‘3’ (TR 1.3)
• Number-Dot Card ‘4’ (TR 1.4)
• Number-Dot Card ‘5’ (TR 1.5)
• Number-Dot Cards ‘1–5’ (TR 1.1–1.5), 1 set per pair
Vocabulary:
• four • five
Lesson 3: 4 and 5
Daily Warm-Up
Objective: Rote count by 1s from 1 to 5
Have students take turns in a circle and rote count by 1s from 1 to 5 (each student says one number). The student who says 5 claps once and steps out from the circle. Repeat the activity a few times.
Quick Recall
Revisit Number-Dot Cards ‘1’, ‘2’ and ‘3’ (TR 1.1–TR 1.3). Show students each card for 3 seconds and have them identify the number. Mix up the cards and show them a card again. Have students clap the quantity that is represented by the number on the card.
Learn and Do
Counting and Number Names for 4 and 5
Hold up 4 fingers on your hand.
Say: I am holding up 4 fingers. Count with me. 1, 2, 3, 4. 4 fingers. Point to each finger as you count.
Then, hold up 5 fingers.
Ask: How many fingers am I holding up now? Say: Let’s count together. 1, 2, 3, 4, 5. 5 fingers.
Show students Number-Dot Card ‘4’ (TR 1.4)
Say: Let’s count the number of dots on the card. 1, 2, 3.
Ask: What comes after 3? (4)
Write ‘4’ and ‘four’ on the board. Draw 4 objects to show the quantity represented by the number.
Have students trace the number 4 in the air.
Ask: Do you see the numbers 4 anywhere in our classroom? (on my watch, in a book etc.)
Repeat the procedure for the number 5 using Number-Dot Card ‘5’ (TR 1.5)
Give out a strip of paper and a set of Number-Dot Cards 1–5 (TR 1.1–1.5) and a bag of connecting cubes to each pair. Model the activity with a student volunteer. Explain that one partner will count the cubes in the bag using the strip of paper. Then, he/she will show his/her partner the Number-Dot Card that matches the number of cubes. The cubes are then returned to the bag and the other partner will do the same. Have pairs switch bags with other pairs and repeat the activity.
Observe:
• Do students use one-to-one correspondence to count out the correct number of objects?
• Do students use an incorrect counting sequence? If so, where is his/her error?
• Can students subitize the quantities?
• Can students state the total quantity counted without recounting?
• Do students choose the correct Number-Dot Card to match a quantity?
Let’s Learn SB pp. 9–10
Refer students to SB p. 9. Have students identify the number 4 and count the objects that are in groups of 4, e.g. 4 flowers, 4 trees.
Refer students to SB p. 10. Have students identify the number 5 and count the objects that are in groups of 5, e.g. 5 twigs, 5 bees.
Daily Wrap-Up
Ask: When you were counting the cubes, I saw many of you touching each cube as you were counting. How did that help you to count? (I count one number when I touch one cube.)
Have students discuss with a partner and share their ideas with the class.
Objectives:
• Count groups of 4 and 5 objects arranged in a line
• Identify the last number counted as the number of objects in the group
• Read and write 4 and 5 in numeral for m and recognize their number names
Materials:
• Sticky notes (each with a number 1, 2, 3, 4 or 5), 1 sticky note per pair
• Counters
• 5 connecting cubes, 1 cup per pair
Resources:
• SB pp. 11–13
• 5-frame (TR 1.6), 1 per pair
• Number-Dot Cards ‘1-5’ (TR 1.1–1.5), 1 set per pair
Lesson 3: 4 and 5
Daily Warm-Up
Objective: Rote count by 1s from 1 to 5
Have students take turns in a circle and rote count by 1s from 1 to 5 (each student says one number). Have them count 1 to 4 in a whisper. The student who says 5 shouts it out loud. Repeat the activity a few times.
Quick Recall
Give out a sticky note to each pair. Explain that each pair should find and get the number of objects shown on their sticky note (e.g. they should get 2 objects if their sticky note has the number 2). Give students a few minutes to gather their objects. Have pairs read the number on their sticky note, count the number of objects they gathered and describe the objects, e.g. 4 markers, 3 pencils, 5 books.
Learn and Do
Count up to 5 with a 5-frame
Show students a 5-frame (TR 1.6) (without identifying it as a 5-frame). Ask: What do you see?
Have students share their thoughts with the class. (E.g. I see 5 boxes. They are in a row. The boxes touch.)
Explain to students that the strip of boxes is called a 5-frame and is used for counting.
Model using a 5-frame to count by placing one counter on the left side of the 5-frame, followed by a second counter beside the first one.
Ask: How many counters do you see? (2)
Continue adding one counter per box, one at a time (from left to right) until the 5-frame is filled. Each time one counter is added, ask students how many counters there are in the 5-frame.
Model the activity with a student. Provide the student with a cup of 5 connecting cubes, and a 5-frame. Mix up the Number-Dot Cards ‘1–5’ (TR 1.1–1.5) and place them face down in a stack. Have the student choose a card and identify its number. Then, have him/her count out the same number of cubes from the cup and place them on the 5-frame.
Ask: How many cubes are there? Is the number of cubes the same as the number on the card?
Have pairs continue the activity. Give out a cup of 5 cubes, a 5-frame and a set of Number-Dot Cards ‘1–5’ to each pair. Explain that one student will choose a Number-Dot Card from the top of the stack, identify the number and count out that many cubes using the 5-frame. The partner will check the student’s counting before students switch roles.
Observe:
• Do students use one-to-one correspondence to count out the correct number of objects?
• Do students use an incorrect counting sequence? If so, where is his/her error?
• Do students skip cubes while counting?
• Can students state the total quantity counted without recounting?
• Can students accurately count out a quantity when given the total?
• Do students begin by placing cubes in the left box on a 5-frame?
Let’s Do SB pp. 11–13
Task 1 provides practice in counting the number of objects in a set and matching it to its corresponding numeral.
Task 2 provides practice in counting the number of objects in a set and writing the corresponding numerals. Guidance is provided.
Task 3 provides practice in counting a set of up to 5 objects and writing the corresponding numerals. An example is provided.
Reteach
Have students count out 4 and 5 counters using a 5-frame, and ask them the “How many?” question. Ensure students count as they place each counter.
Daily Wrap-Up
Ask: How can we use a 5-frame to count?
(We put one thing in each box.)
Have students discuss with a partner and share their ideas with the class.
Objectives:
• Count up to 5 objects arranged in different ways (in a line, 5-frame, array, circular, scattered)
• State the number of objects in a counted set of up to 5 objects without recounting
Materials:
• Counters
Resources:
• SB pp. 14-15
• Number-Dot Cards '1–5' (TR 1.1–1.5)
• 5-frame (TR 1.6)
• Picture Cards: 2 Objects in Different Arrangements (TR 1.7)
• Picture Cards: 3 Objects in Different Arrangements (TR 1.8)
• Picture Cards: 4 Objects in Different Arrangements (TR 1.9)
• Picture Cards: 5 Objects in Different Arrangements (TR 1.10)
Lesson 4: 1 to 5
Daily Warm-Up
Objective: Rote count by 1s from 1 to 10
Have students stand in a circle and rote count from 1 to 5. Count from 6 to 10 and have them repeat after you. Once students are familiar with the numbers 6 to 10, have them count from 1 to 10.
Have students take turns in the circle and rote count by 1s from 1 to 10 (each student says one number). The student who says 10 sits down and the next student begins counting from 1 again. Repeat the activity a few times.
Quick Recall
Revisit Number-Dot Cards ‘1’ to ‘5’ (TR 1.1–1.5). Show students each card for 3 seconds and have them identify the number. Mix up the cards and show them a card again. Have students clap the quantity that is represented by the number on the card.
Learn and Do
Counting Objects Arranged in Different Ways
Prepare the Picture Cards: 2 to 5 objects in Different Arrangements (TR 1.7–1.10) before the activity.
Have students gather around a table. Place 4 counters on a 5-frame (TR 1.6).
Ask: How many counters are there? (4) Rearrange the counters in a line on the desk. Ask: How many counters are there now? (4) Rearrange the counters into 2 rows of 2.
Ask: How about now? (4) Did the number of counters change? (no) Explain that you did not use more counters or take away any counters. Ask: What changed about the counters? (the way they are arranged)
Rearrange counters in a circular and then a scattered arrangement, and ask if there are still 4 counters.
Say: We can arrange objects in different ways. 4 counters can be arranged in a circle, in a line or scattered everywhere. But the number of counters will not change.
Repeat the procedure with other quantities, e.g. 3 or 5. Start by using the 5-frame. After students have identified the number of counters, change the arrangement. Have them note that the quantity does not change.
Mix up the Picture Cards. Place them face down in a stack. Choose a card that shows items in a scattered arrangement (e.g. 3 scattered cubes). Show the card to students.
NOTE: Having students think about why they chose the number 3 helps to improve metacognition and communication skills.
Ask: How many objects? (3) How do you know? After students explain their thinking, place the card picture up on the table. Repeat this procedure with other cards. Do not stack the cards.
Say: Find all the cards that have 3 objects on them. Have students place these cards in a group.
Ask: What else do you notice about each card? (Some objects are in a line and some are in a circle.)
Have students group cards with 5 objects and talk about how they are arranged on each picture card. Choose the card that shows 5 objects in a circular arrangement.
Ask: How can we count the objects on this card?
Have students discuss in pairs and share their thoughts with the class. (E.g. We could put a counter on each object as we count./We could draw a line over each object counted.)
If students do not suggest putting a counter on each object as it is counted, model this for students.
Let’s Learn SB pp. 14–15
Give 5 counters to each student. Refer students to SB p. 14, part (a). Have students look at the counter and the numeral 1.
Ask: How many counters are there in the thought bubble? (1) Can 1 counter be arranged in another way? (no)
NOTE: Placing counters on the pictures models how to keep track of what has been counted and what still needs to be counted.
If necessary, model for students how 1 counter only has 1 arrangement. Refer students to SB pp. 14–15, parts (b) to (e). Have students look at the different arrangements in each picture and count the number of counters in each set. Ask students if they think the different arrangements have the same number of counters. Ask them how they would use a 5-frame to arrange 1 to 5 counters.
Daily Wrap-Up
Draw 4 objects in a row. Then, draw another 4 objects in an array.
Ask: How many objects are there in each picture? (4)
Have students identify that the number of objects in each group is 4, although they are arranged differently.
Objectives:
• Count up to 5 objects arranged in different ways (in a line, 5-frame, array, circular, scattered)
• State the number of objects in a counted set of up to 5 objects without recounting
Materials:
• Paper, a few sheets per student
• Pencils
Resources:
• SB pp. 16–17
• Number Cards 2–5 (TR 1.11), 1 set per pair
Lesson 4: 1 to 5
Daily Warm-Up
Objective: Rote count by 1s from 1 to 10
Have students take turns in a circle and rote count by 1s from 1 to 10 (each student says one number). The student who says 10 sits down and the next student counts from 1 again. Repeat the activity a few times.
Quick Recall
Revisit counting a group of 3 objects (using students) in different arrangements. For example, place the same 3 students in a line and then in a scattered arrangement. Each time, have the rest of the class count the students in the group and answer the ‘How many?’ question.
Ask: How many students are standing here? (3) Did the number of students change? (no)
Have students explain their thinking.
Learn and Do
Counting Objects Arranged in Different Ways
Have students gather around a table. Invite a student to model the activity with you. Provide the student with a set of Number Cards 2–5 (TR 1.11), a sheet of paper and a pencil. Have the student choose a number card and draw the corresponding number of dots on the paper. Check if the number of dots matches the number chosen. Draw the same number of dots in a different arrangement on your sheet of paper. Show students both sheets of paper.
Ask: Do they have the same number of dots? (yes) What is different? (They are arranged differently.)
Have students work in pairs to continue the activity. Give a set of Number Cards 2–5 and a sheet of paper to each pair.
Observe:
• Do students count the dots on their paper using one-to-one correspondence?
• Can students state the total quantity counted without recounting?
After students have finished the activity, collect all the sheets of paper with students’ dot drawings. Select a set of drawings with 2 to 5 dots. Lay them out separately on the floor at the center of the classroom. Say: We will put all the drawings with the same number of dots together.
Mix up the students’ dot drawings and distribute them to the class.
Ask: Who has a drawing with 2 dots?
Tell students who have drawings with 2 dots to place them on the floor, next to the correct drawing you put in the center of the classroom. Do the same for the rest of the numbers.
After all the drawings have been sorted out, have students look at the various arrangements that they have come up with to show the numbers 2 to 5.
Let’s Do SB pp. 16–17
Task 1 provides practice in counting and matching groups of counters arranged in different ways.
Task 2 provides practice in counting the number of objects in different arrangements and writing the corresponding numerals.
Observe:
• Can students count in sequence using one-toone correspondence?
• Do students have difficulty with any particular arrangement when counting pictorial representations of quantities?
Reteach
Have students refer to SB pp. 14–15. As they count, point to each counter in the group. Especially for circular arrangements, you might want to get students to put one finger on the first counter before counting on.
4 3 1 3 2 5 5 4
Daily Wrap-Up
Ask: What is your favorite arrangement? Why?
(E.g. I like to count objects in a circle because it’s like a game./ I like to count objects in a line because it is easy.)
Have students discuss with a partner and share their ideas with the class.
Objective:
• Count out a given number of up to 5 objects from a larger set of up to 8 objects
Materials:
• 8 counters, 5 sets
• Double-sided tape
• Paper, 5 sheets
Resources:
• SB pp. 18–20
• 5-frame (TR 1.6), 5 copies
• Number Cards 1–5 (TR 1.11)
Lesson 4: 1 to 5
Daily Warm-Up
Objective: Rote count by 1s from 1 to 10
Have students take turns in a circle and rote count by 1s from 1 to 10 (each student says one number). As they count, have them hop the corresponding number of times. Repeat the activity a few times.
Quick Recall
Revisit counting groups of 4 objects (using students) in different arrangements. For example, place the same 4 students in an array, then in a line and finally, in a scattered arrangement. Each time, have the rest of the class count the students in the group and answer the ‘How many?’ question. Ask: How many students are standing here? (4) Did the number of students stay the same? (yes) Why is this so? (Nobody left, you just moved them.) Have students explain their thinking.
Learn and Do
Counting from a Set
Prior to the lesson, prepare 5 copies of the 5-frame (TR 1.6) by sticking double-sided tape on each box of the 5-frame.
Have students gather around a table. Invite 2 students to model the activity. Place a 5-frame, 8 counters and a set of Number Cards 1–5 (TR 1.11) face down on the table. Have Partner A choose a number card and show it to Partner B. Partner B then counts out that many counters from the pile of 8 counters and sticks them on the 5-frame. Partner A checks to see if the number of counters is correct.
Ask: What number is on your number card? Did your partner count out the correct number of counters? Move the 5-frame (with the counters on it) aside.
Prepare a few more sets of 8 counters. Invite more students to do the activity until all the number cards and 5-frames have been used. Put up all the 5-frames.
Ask students to count and say how many counters are in each 5-frame. Get 5 students to each write the corresponding number on separate sheets of paper and place them next to the respective 5-frames. Assign each student one 5-frame.
Let’s Learn SB p. 18
Refer students to SB p. 18. Have them look at the pictures to recall what they did for the earlier activity. Remind students that when they count out a smaller set of objects from a bigger set of objects, they can use a 5-frame to help them count.
Let’s Do SB pp. 19–20
Task 1 provides practice in counting the number of counters in different arrangements and drawing the corresponding 5-frame.
Task 2 provides practice in counting out a given number of objects from a larger set of objects.
Reteach
Have students count out 4 and 5 counters using a 5-frame, and ask them the ‘How many?’ question. Ensure students count as they place each counter.
Daily Wrap-Up
Draw 4 dots in a line on the board. Ask students what other ways they can arrange the 4 dots. Have students share their ideas with the class.
Objectives:
• Count up to 5 objects arranged in different ways (in a line, array, scattered)
• Identify the last number counted as the number of objects in the group
• State the number of objects in a counted set of up to 5 objects without recounting
Materials:
• Counters (optional)
Resources:
• SB p. 21
• 5-frame (TR 1.6), 1 per student (optional)
Chapter Workout and Learning Center Activities
Chapter Workout SB p. 21
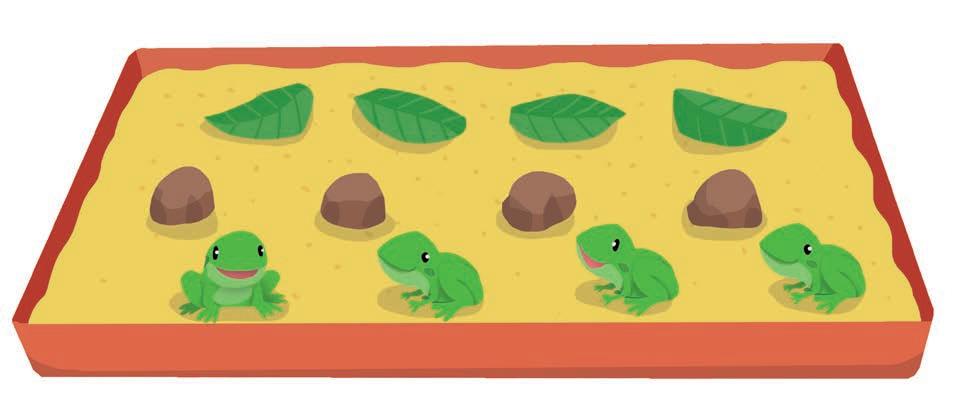
Have students look at the picture on SB p. 21.
Ask the following questions to encourage discussion.
Ask:
• What types of animals do you see? (birds, butterflies, bees, squirrels and a cat)
• What are the animals doing? (The birds are sitting on the branch. The butterflies are flying around. The squirrels are climbing up/down the tree. The bees are returning to their hive. The cat is standing under the tree.)
• Do you notice anything unusual about any of the animals? (One of the birds is wearing a hat. The squirrels are carrying strings of nuts. The cat is holding a broom.)
Have students look at the various objects and their arrangements on SB p. 21.
Ask:
• What is there 1 of? (1 beehive, 1 hat, 1 tree branch/tree, 1 cat, 1 broom, 1 worm)
• What are there 2 of? (2 squirrels)
• What are there 3 of? (3 birds, 3 bees) How are they arranged? (in a row, scattered)
NOTE: The questions assess students’ understanding of counting groups of up to 5 objects in various arrangements and whether they can identify the last number counted as the number of objects in the group.
• What are there 4 of? (4 leaves, 4 brown nuts, 4 yellow nuts) How are they arranged? (in a row, scattered)
• What are there 5 of? (5 butterflies)
For struggling students, have them place a counter on each object being counted, e.g. when counting the leaves, have students place a counter on each leaf. Students then transfer the counters onto the 5-frame (TR 1.6) to determine the number of counters, which is also the number of leaves.
Have students look at the butterflies.
Ask:
• Are they all the same color? (no)
• What can you say about the butterflies? (2 out of 5 are blue./3 out of 5 are red.)
NOTE: The questions assess students’ ability to count out a smaller number of objects from a larger set of objects.
Repeat the questioning for the leaves. (some are on the tree, some are not)
Objectives:
• Count up to 5 objects in a circular arrangement
• State the number of objects in a counted set of up to 5 objects without recounting
Materials:
• Counters, 5 per pair
Resources:
• SB p. 22
Activity 1 SB p. 22
Have students look at SB p. 22. Have them think about whether Belle or King is correct and why. Then, have them turn and talk to a partner to discuss their thinking. Provide time for pairs to share what they discussed.
Ask: How many counters are there? (5)
Ask: How can you keep track of your counting? (E.g. by putting my finger on the counter I start counting from.)
Distribute 5 counters to each pair. Have them verify the number of counters they see on the page by placing a counter on top of each picture. Have students count as they place their counters.
Observe:
• Do students keep track of where they start counting?
• Do students use different strategies to count objects in a circular arrangement?
Objectives:
• Identify the last number counted as the number of objects in the group
• Read 1 to 5 in numeral for m and recognize their number names
Materials:
• Paper clips (different colors), 2 per pair
Resources:
• SB p. 23
• Number Cards 1–5 (TR 1.11), 1 set per student
Activity 2 SB p. 23
Have students complete the counting game given on SB p. 23.
Distribute 2 paper clips (of different colors) and 2 sets of Number Cards 1–5 (TR 1.11) to each pair.
Have Partner A place his/her paper clip on the green box of Path A. Partner B should put his/her clip on Path B. Then, have each student mix up his/her Number Cards and place them face down in a stack. Partner A chooses the top card of his/her stack and tells Partner B the number. Partner A then moves his/her paper clip that many spaces up the path. Then, Partner A tells his/her partner how many steps were moved.
Partner B chooses the top card of his/her stack and repeats the procedure. Partners continue to take turns until the first of the pair reaches the red box on top. Partners then start over and repeat the activity if there is time.
Objectives:
• Count up to 5 objects arranged in different way (in a line, array, scattered)
• Identify the last number counted as the number of objects in the group
• Write 1 to 5 in numeral form
• State the number of objects in a counted set of up to 5 objects without recounting
Resources:
• SB p. 24
Activity 3 SB p. 24
Have students look at SB p. 24.
Explain that students are to count the number of objects in each group and write the corresponding number.
Have students check their partner’s work after the activity.
Chapter Wrap-Up
Have students discuss what they have learned in the chapter. Ask:
• What numbers can you count now? (1, 2, 3, 4 and 5)
• If I have 5 beads, can I arrange them in different ways? (yes)
• Can I arrange 1 bead in different ways? (no)
3 5 2 1 4
• Which number do you find easiest to write? The most difficult to write? (Answers will vary.)
Problem Solving (1 day)
Please refer to the Problem Solving Resources for teaching notes and materials.
Numbers 0 to 10
Scheme of Work
Lesson
Lesson 1: 6 and 7
Counting Groups of 6 and 7
Counting 6 and 7 with 10-Frames
Lesson 2: 8 and 9
Counting Groups of 8 and 9
Counting 8 and 9 with 10-Frames
Lesson 3: 10
Counting to 10
Lesson 4: 0
Zero
Objectives
• Count groups of 6 and 7 objects
• Identify the last number counted as the number of objects in the group
• Read and write 6 and 7 in numeral form and recognize their number names
• Count on from 5 to count groups of 6 and 7 objects arranged in a 10-frame
• Count groups of 6 and 7 objects
• Identify the last number counted as the number of objects in the group
• Read and write 6 and 7 in numeral form and recognize their number names
• Count groups of 8 and 9 objects
• Identify the last number counted as the number of objects in the group
• Read and write 8 and 9 in numeral form and recognize their number names
• Count on from 5 to count groups of 8 and 9 objects arranged in a 10-frame
• Count groups of 8 and 9 objects
• Identify the last number counted as the number of objects in the group
• Read and write 8 and 9 in numeral form and recognize their number names
• Count groups of 6 to 10 objects arranged in a line and in a 10-frame
• Count on from 5 to count groups of 6 to10 objects arranged in a 10-frame
• Identify the last number counted as the number of objects in the group
• Read and write 6 to 10 in numeral form and recognize their number names
• Awareness of 0
• Count groups of 1 to 10 objects
• Read and write 0 in numeral form and recognize its number name
Total Duration: 12 days (1 day = 1 hour)
• Chart paper
• Connecting cubes (7 green, 7 red and 7 yellow), 1 set per group of 3 students
• 10-cm strip of paper, 1 per group of 3 students
• Cups of connecting cubes (5, 6 and 7 cubes in each cup respectively), 3 cups
• Cups of 8 cubes, 1 cup per pair
• 10-cm strip of paper, 1 per pair
• Connecting cubes (9 green, 9 red and 9 yellow), 1 set per group of 3 students
• 10-cm strip of paper, 1 per group of 3 students
• Bags of connecting cubes (8 and 9 cubes in each bag respectively), 2 bags per pair
• 10-cm strip of paper, 1 per pair
• Cups of unconnected cubes (8 and 9 cubes in each cup respectively), 2 cups
• Cups of 10 cubes, 1 cup per pair
• 10-cm strip of paper, 1 per pair
• Cups of 10 unconnected cubes, 1 cup per pair
• Counters (2 different colors), 2 per pair
• Construction paper, 6 sheets
• Groups of 1 to 5 connecting cubes
• SB pp. 25–28
• Number-Dot Card ‘6’ (TR 2.2)
• Number-Dot Card ‘7’ (TR 2.3)
• SB pp. 29–30
• 5-frame (TR 1.6), 5 copies
• 10-frame (TR 2.1), 1 per pair
• Number-Dot Cards ‘1–7’ (TR 1.1–1.5, 2.2–2.3)
• SB pp. 31–33
• Number-Dot Card ‘8’ (TR 2.4)
• Number-Dot Card ‘9’ (TR 2.5)
• SB pp. 34–35
• 10-frame (TR 2.1), 1 per pair
• Number-Dot Cards ‘1–9’ (TR 1.1–1.5, 2.2–2.5)
• SB pp. 36–37
• 10-frame (TR 2.1), 1 per pair
• Number-Dot Card ‘10’ (TR 2.6)
• Number-Dot Cards ‘1–10’ (TR 1.1–1.5, 2.2–2.6), 1 set per pair
• Snaking Game Board (TR 2.8), 1 per pair
• SB pp. 38–39
• Number-Dot Card ‘0’ (TR 2.7)
• Number-Dot Cards ‘0–5’ (TR 1.1–1.5, 2.7)
• six • seven • group
• 10-frame
2 days
• eight • nine
• 10-frame
1 day
• ten 1 day
• zero
Lesson
Lesson 5: 0 to 10
Different Groups of 1 to 10 Objects
Comparing Groups
Objectives
• Count up to 10 objects (in a line, 10-frame, array, circular, scattered)
• Count out a group of up to 10 objects from a larger group of up to 15 objects
• State, without recounting, the number of objects in a group of up to 10 objects
• Write a numeral (0–10) to represent a number of objects (0–10)
• Compare 2 groups of up to 10 objects each
• Make comparisons using ‘the same as’, ‘more than’ and ‘fewer than’
Lesson 6: One More and One Fewer
One More and One Fewer
Lesson 7: Counting On
Counting to 10 Using a Number Tape
• Compare 2 groups of up to 10 objects each
• Make comparisons using ‘the same as’, ‘more than’ and ‘fewer than’
• Understanding that the number that comes next is 1 more
• Count groups of 1 to 10 objects
• Write a numeral (0–10) to represent a number of objects (0–10)
• Use ‘before’, ‘after’ and ‘in between’ to talk about number sequence
• Identify the number that comes before, after and in between other number(s)
Chapter Workout and Learning Center Activities
Chapter Workout
Activity 1
Activity 2
Activity 3
Problem Solving
• Count up to 10 objects (in a line, 10-frame, array, circular, scattered)
• Identify the last number counted as the number of objects in the group
• State how many objects are in a counted set of up to 10 objects without recounting
• Make comparisons using ‘the same as’, ‘more than’ and ‘fewer than’
• Read and write 0 to 10 in numeral form and recognize their number names
• Count out a group of up to 10 objects from a larger group of up to 15 objects
• Identify the numbers that come before, after and in between other number(s)
• Connecting cubes, 15 per pair
• Chart paper
• Connecting cubes (15 yellow and 15 blue)
• Construction paper
• 1 die
• 55 connecting cubes
• 1-m long white paper, 1 strip
• Masking tape
• Sticks of 1 to 10 connecting cubes
• SB pp. 40–41
• Number Cards 0–10 (TR 1.11 and 2.9), 1 set per pair
• SB pp. 42–44
• SB pp. 45–46
• SB pp. 47–48
• Number Cards 0–10 (TR 1.11 and 2.9)
2 days
• group/set
• 10-frame
• scattered
• group/set
• more than
• fewer than
• the same as
1 day
• one more
• one fewer
1 day
• number tape
• before
• after
• in between
1 day
• 10 counters per pair
• 15 counters, 1 cup per pair
• SB p. 49
• SB p. 50
• SB p. 51
• 10-frame (TR 2.1), 1 per pair
• Number Cards 0–10 (TR 1.11 and 2.9)
• SB p. 52
• Problem Solving Big Book 2 and Reader 2
• Problem Solving Teacher's Guide
1 day
TR 1.7 Picture Cards: 2 Objects in Different Arrangements
TR 1.8 Picture Cards: 3 Objects in Different Arrangements
TR 1.9 Picture Cards: 4 Objects in
TR 1.10 Picture Cards: 5 Objects in Different Arrangements
1.11 Number Cards 0 – 5
2 3 4 5
Mathematics Student Book A K
A world-class program based on top-performing Singapore, Republic of Korea and Hong Kong
About Mathematics K
Welcome to Scholastic Mathematics K
Scholastic Mathematics K is the foundation level of TM Mathematics, an innovative world-class mathematics program based on the effective teaching and learning practices of the global top-performers in Mathematics – Singapore, Republic of Korea and Hong Kong. K is focused on the development of early numeracy and problem-solving skills and supports the learning of all students at the highest possible level to build in students a strong foundation of meaningful learning with a problem-solving mindset. The content in Scholastic Mathematics K is presented under four strands of mathematics: Numbers and Operations, Measurement, Geometry and Data Analysis. There are two Student Books, A and B, which are used consecutively.
The instructional design of K integrates children’s learning, classroom practice, and teachers’ own requirements of content and pedagogical knowledge in a coherent, focused, and rigorous program. Each chapter in the program comprises concept lessons designed to provide enjoyable and effective learning experiences in readiness, engagement and mastery of mathematical concepts, skills and knowledge at an appropriate level.
The Teacher’s Guide provides a comprehensive plan for each lesson which begins with a daily warm-up followed by a lesson that introduces new concepts using engaging hands-on activities and ends with a wrap-up activity. The Student Book is designed for each child to work in when directed by the teacher and comprises four parts: Let’s Remember, Let’s Learn, Let’s Do and Chapter Workout and Activities.
Let’s Remember appears at the start of each chapter and provides an opportunity for systematic recall and assessment of prior knowledge in preparation for new learning.
Each Let’s Remember activity is carefully crafted to check for readiness to learn new concepts.
Each chapter comprises several lessons with each focusing on a concept. In each lesson Let’s Learn introduces the concept using the concrete-pictorial-abstract approach to develop deep conceptual understanding.
Concepts are taught with a hands-on activity. This is the concrete stage of the learning journey. Students work in groups or in pairs to participate in concrete mathematical experiences. The teacher guides enquiry – leading students to explore, investigate and find answers on their own.
In the pictorial stage, students are guided to understand mathematical ideas presented visually via the Student Book. This middle stage is a crucial link between the concrete experience and the abstract representation and serves to build a solid foundation.
The concept or skill is finally represented at the abstract level using numbers and mathematical symbols.
After a concept has been taught Let’s Do provides guided practice and immediate formative assessment.
Systematic variation of tasks reinforces students’ understanding and enables teachers to check learning and identify remediation needs.
The Chapter Workout and Activities serve to consolidate learning through discussions, games and individual activities.
Teachers guide a class discussion around the Big Picture to consolidate the concepts and skills taught in the chapter.
Activity 1 is a Think, Turn and Talk activity, intended to promote pair discussion, challenge common misconceptions and strengthen mathematical thinking.
Activity 2 is a game, providing a motivating context for students to practice what they have learned.
Activity 3 is an individual activity, ideal for formative assessment.
Chapter 1 Counting Small N umbers
Chapter
Chapter
Counting Small Numbers
Lesson 1 1 and 2
Count and say.
2
1. Count and match.
Count, trace and write.
2 1 , 2 and 3
1. Count and match.
2. Count, trace and write. 1
(a) (b)
(c) (d)
(e) (f)
Lesson 3 4 and Count and say.
1. Count and match.
e and write.
. Count and write.
Lesson 4 1 to Count and say.
1. Count and match.
. Count and write.
(e)
(f)
(g) (h)
Count and say.
1
. Count and draw .
circle.
(a) Circle 2 frogs.
(b) Circle 3 leaves.
(c) Circle 1 flower.
Chapter Workout
Look and talk.
Activity 1
Think, turn and talk.
There are 5 counters. No, 4 counters.
Who is right?
How do you know?
Belle
King
Activity 2
Choose a card. Count your steps. Get to the top first and win! PATH A
Count and write the number.
.
