SL Maths - Analysis & Approaches

SL Maths - Analysis & Approaches

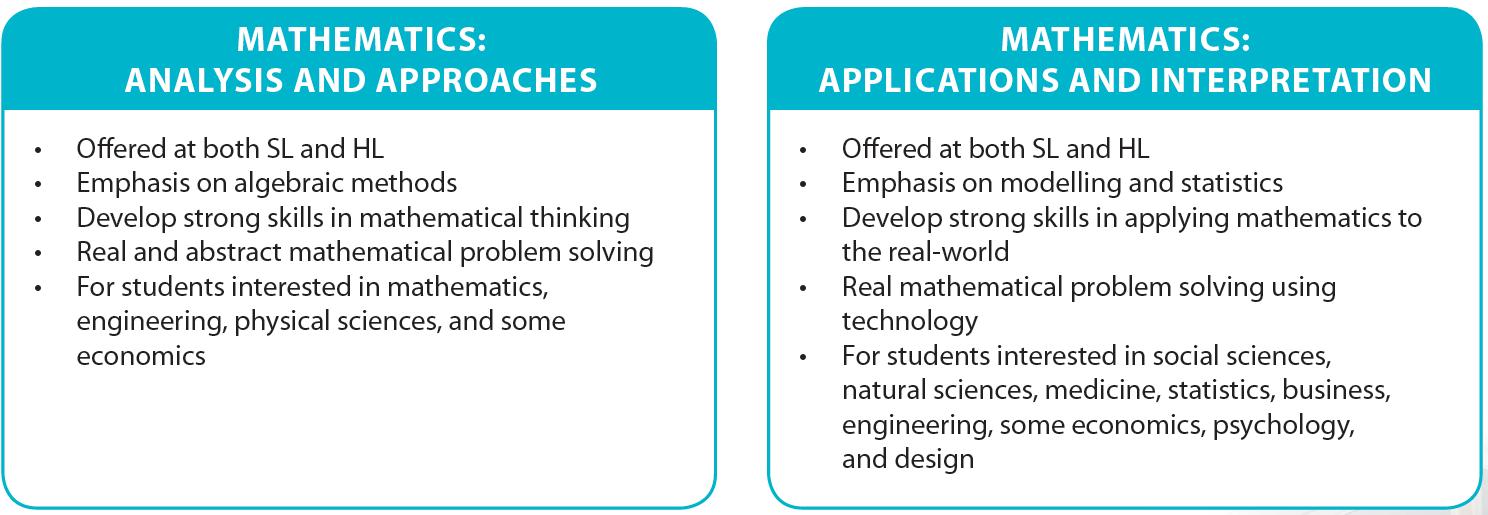
Comparison between the two courses:

This is a course which had its first examination in 2021 and is appropriate for students who intend studying a course at university with some mathematical content. For example, physical sciences or economics.

Aims: The mathematics courses aim to contribute to students' personal attributes, subject

understanding and global awareness by enabling them to:

1. develop a curiosity and enjoyment of mathematics and appreciate its elegance and power 2. develop an understanding of the concepts, principles and nature of mathematics 3. communicate mathematics clearly, concisely and confidently in a variety of contexts 4. develop logical and creative thinking, and patience and persistence in problem solving to instil confidence in using mathematics 5. employ and refine their powers of abstraction and generalization 6. take action to apply and transfer skills to alternative situations, to other areas of knowledge and to future developments in their local and global communities 7. appreciate how developments in technology and mathematics influence each other 8. appreciate the moral, social and ethical questions arising from the work of mathematicians and its applications 9. appreciate the universality of mathematics and its multicultural, international and historical perspectives 10. appreciate the contribution of mathematics to other disciplines, and as a particular

“area of knowledge” in the TOK course 11. develop the ability to reflect critically upon their own work and the work of others 12. independently and collaboratively extend their understanding of mathematics

Course Description:

This course caters for students with a good background in Mathematics who are competent in a range of analytical and technical skills. The majority of these students will be expecting to follow a university course where Mathematics is used to support the subject area, for example, courses such as medicine, biology, chemistry, business, psychology, or ICT. Others may take this subject because they have a strong interest in Mathematics and enjoy meeting its challenges and engaging with its problems.

The course covers five broad areas of Mathematics: number and algebra, geometry and trigonometry, functions, statistics and probability, and calculus. The students will need a graphical display calculator (GDC) which will be used throughout the course. Students will complete an internal assessment worth 20% of their final grade.

Students will learn to become fluent in the construction of mathematical arguments and proofs. They will enjoy mathematics in its more abstract form such as calculus and functions.

Graphic Display Calculator (GDC)

Students are required to use a GDC throughout the course and during their exams. In Tanglin we use the TI n Spire CX, and all students are expected to buy this particular model. It is available for sale in the school shop.

Requirements:

To follow the SL Analysis course. they need to be motivated and interested in mathematics and need to be particularly careful about choosing between Maths Applications SL and Maths Analysis SL. These two courses are viewed differently by different universities around the world.

Career Path:s

The study of mathematics is excellent for developing critical thinking, problem solving and being analytical in one’s response to a number of diverse problems. It requires application and dedication and helps students develop independence. As such, it is invaluable in any career but particularly important in careers requiring Mathematics as a major component. These careers include engineering, the actuarial profession and other financial sectors, economics, IT, computer programming and systems administration, research and development and statistical analysis. Intelligence agencies require top class mathematicians, as do many other careers. Analysis particularly suits courses with a high Mathematical content e.g. Maths, Physics, Economics.

Comparison between this course and A-Level

The main difference is in the assessment. A Level questions can be more structured. IB questions vary in style and bring many topics into one problem. Sometimes there are aspects that a student may not have seen before in order to test problem solving skills. Another difference is the internal assessment that is required for IB. No coursework is required for A Level. Also, A Level does not require confident use of a graphical calculator, whereas IB does.

Assessment:

The course is assessed with two papers, one non-calculator and one calculator worth 80% and an "exploration" worth 20%. The exploration is a piece of written work undertaken in Term 3 of Year 12 which investigates an area of mathematics of their choosing.

Contact for further information:

Mr Chris Hollingworth: chris.hollingworth@tts.edu.sg Mrs Sarah Aldous: sarah.aldous@tts.edu.sg