New Shapes

Isabel Hardwig

Bad Habits Editor

I have not always loved tile patterns, but I do love jumping on the tile patterns bandwagon whenever something interesting is going on there. A couple weeks ago, the most tile patterns thing happened since 1974, and it was a doozy! In case this is the first you’re hearing about it, here’s a rundown:

• Some tiles can make patterns that are infinite (fit into each other without gaps or overlaps, and could continue doing so forever).

• Some tiles can make patterns that are non-repeating (you can’t just take one segment of tile pattern and insert it into another segment of tile pattern elsewhere in the tile pattern–it has no translational symmetry).

• Although mathematicians have found sets of multiple tiles that meet both criteria, they have long been searching for the “Einstein” of tile patterns: a single tile that can make a pattern that is both infinite and non-repeating.

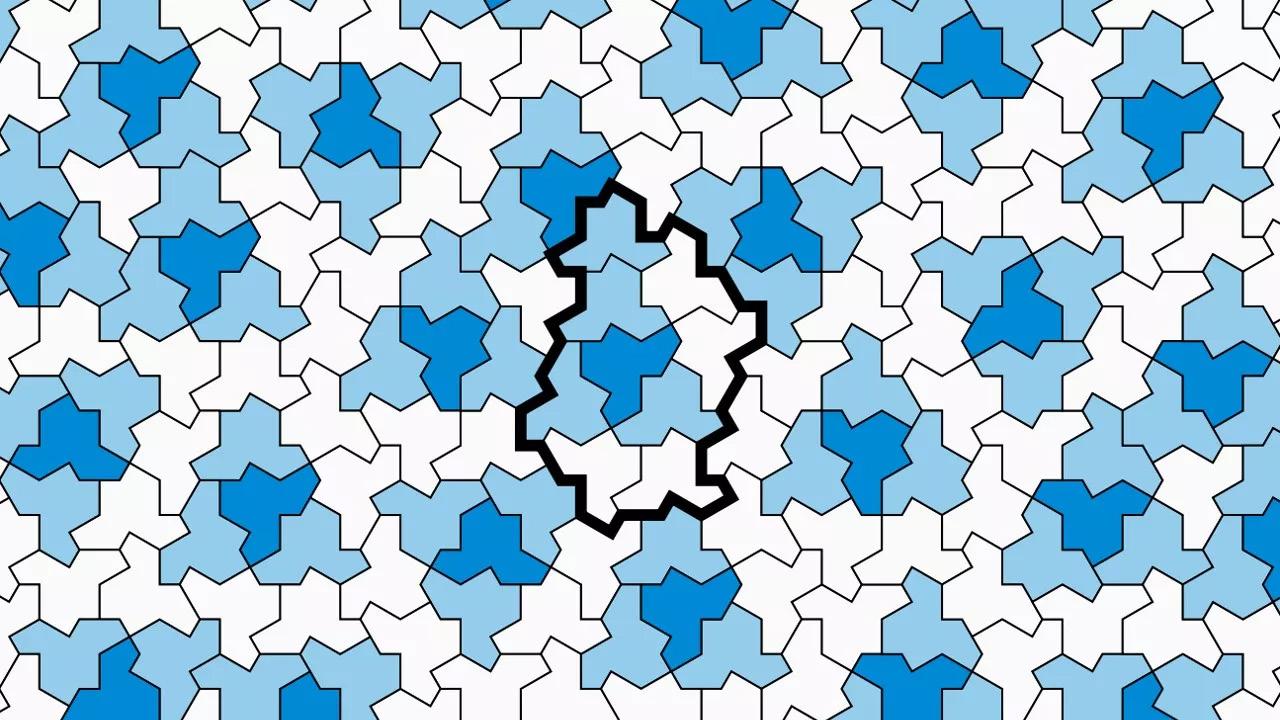
• A guy called David Smith, who is “always messing around with shapes,” recently discovered a shape that fits the bill, solving a math problem that has been vexing people since the ‘60s. It has 13 sides, it’s called “the hat,” and it rocks more than anything else in the world.

The Hat

I can admit that the whole concept of “hat tile” has already been done pretty well, but what can I say? I stand on the shoulders of giants. Here is a tile that probably would not fit together infinitely without repeating patterns, but which does look like a hat, and that’s one third of the battle.

Tree That Grew Around A Bike

This tile is in the shape of a bike that was abandoned near a seedling, and the seed grew into a big, strong tree that kind of made the bike into a part of it. I think it’s a really good tile, because every time you’re making eggs or taking a shower, you could look down and be imparted with an important message about nature and resilience. I didn’t really have time to check if it was infinite or nonrepeating, but if you tried it out I bet it would be.

Downstairs Bathroom

The world’s first shape with the vague contours of a downstairs bathroom, this tile would be perfect for a downstairs bathroom. You could also put it in an upstairs bathroom, but it might confuse people. As a bonus, I have a strong hunch that it’s mathematically significant somehow.

Though I’m no “mathematician,” I do love shapes, and I love shapes that are also somehow Einstein, so it seemed like a no-brainer to give this one a try. Here are some of my proposals for new tile shapes, which I hope will be just as, if not more, revolutionary.

Square

This one I’m thinking of calling a “square.”

Ivory Tower

This shape is one of the most important ones, in my opinion. A striking form of protest against all the hoity-toity academics who claim that a shape needs to “be infinite” and “can’t repeat” in order to have “mathematical significance.”

SCREENPLAY FOR “MAZE RUNNER 2: THE SCORCH TRIALS”

If you wrote one letter on each tile, and maybe did a couple pictures, you could easily make a pattern that doesn’t repeat and basically goes on forever (2 hr 13 min runtime).

Bigggg Tile

This tile is infinite and non-repeating because there’s only one of them in the whole pattern, because it’s soooo big. I don’t think anyone’s thought of this yet, but it could be a gamechanger.