Electrical Fields

ELECTRICAL FIELDS

Any type of contact force, such as between a tennis racquet and ball, can be explained through interactions of forces of atoms and molecules that are in close proximity to one another. The force that explains this is Coulomb force. This must be contact force because the action of charges between molecules occurs over extremely close distances. All objects are surrounded by a force field, however, small, that has the ability to act on another object a short distance away. This is how charged objects can act on other objects over a distance.

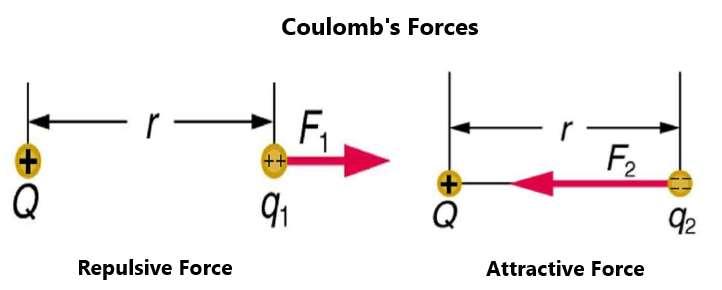
In this way, the Coulomb force field is the force field around any charged object in space. Coulomb’s law can be rewritten to be the force being proportional to a point charge, indicated by the capital letter Q, acting on a test charge, indicated by the small letter q, over the distance between them squared. Figure 108 describes this:

Figure 108.

Coulomb’s force fields will still involve repulsive forces with like charges and attractive forces on unlike charges. The magnitude of the force will be greater if the charge on either one of the objects is greater. What this means is that the magnitude will be proportional to the degree of charge on either side. The force field is not unique to any point in space but instead depends on the charge magnitude of either object.

If the test charge is changed and the point charge is held the same, the electric field is the total force (the Coulomb force) divided by the test charge q. The electric field E will

be a vector in the same direction as the force, which is held to be positive if there is attraction and negative if there is repulsion. The assumption is that the test charge is small compared to the total force. The SI units for electric field are Newtons per Coulomb. Because the test charge is small, the electric field will be a constant multiplied by the point charge divided by the radius squared.

As mentioned, the electric field is a vector, represented by a magnitude and a direction. In identifying a force field, multiple forces are described over a space rather than single force vector. Again, it is assumed that the test charge is so small so that the force field is mainly determined by the point charge or capital Q. Figure 109 describes the force field of a point charge with a test charge responding to the point charge anywhere it is placed in the electric field:

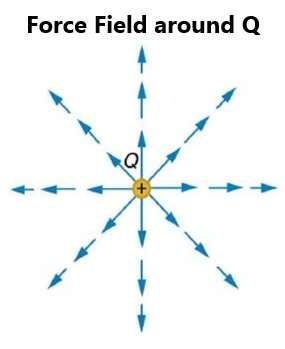
Figure 109.

According to convention, the path of the field lines points away from a positive charge and toward a negative charge. Its magnitude is directly proportional to the number of field lines per unit area, because the area is proportional to the radius squared. This means the magnitude is inversely proportional to the square area involved. The closer the field lines are in an electric field, the stronger is the field. This measure of strength applies to all types of fields, including electrostatic, gravitational, and magnetic fields.

The total electric field from multiple charges is the vector sum of the individual fields created by each charge. Figure 110 shows what an electric field would look like when multiple charges are involved in determining the electric field:

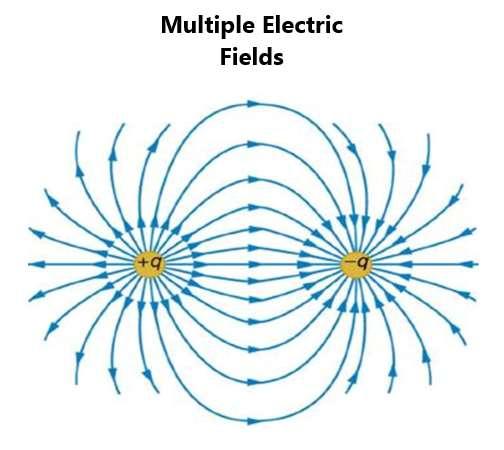
Figure 110.

The above situation is what an electric field looks like when a positive charge and a negative charge add their vectors to make a total electric charge. The field would be weaker between like charges as shown by lines being further apart, while when the field is greater, the lines would be closer together. The greater the distance from two like charges, the field becomes identical to what it would look like in a single, larger charge alone. At very large distances, there is no addition of the vectors because the distance is too far away.

To summarize field lines, these things about field lines are true:

• Field lines begin on positive charges and end on negative charges or at infinity for isolated charges (hypothetically).

• The density of field lines (or how close they are together) depends on the magnitude of the charge.

• The strength of the field is proportional to the number of lines per unit square area perpendicular to the lines.

• The direction of the electric field is tangent to the field line at any point in space.

• Field lines can never cross one another.

A conductor is something that contains free charges that will easily move. When there is an excess charge on a conductor in a static electric field, the conductor will quickly respond to reach a steady state known as electrostatic equilibrium. The free charges will move until the field is perpendicular to the surface of the conductor. Any force parallel to the surface will not indicate the presence of an electrostatic equilibrium. Again, the direction of the vector will be from positive to negative and will be perpendicular to the charged conductor.

Conductors in an electric field will be polarized so that the charges will shift. The negative charge will go toward the arrow of the vector, while the positive charge in the conductor will go away from the arrow of the vector. The field will become stronger near the conductor but is not present within the conductor itself. This is seen clearly in figure 111:

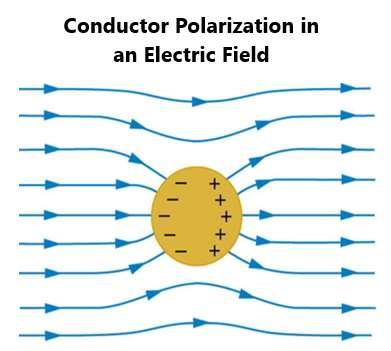
Figure 111.

According to the figure, the electric field is zero inside the conductor and the electrical field lines are perpendicular to its surface, ending or beginning on the charges on the surface of the conductor. Excess charge will reside entirely on the surface of the conductor. A uniform electric field can be created by having a field between two plates that are uniform in strength and direction. The excess charge will distribute themselves uniformly, producing field lines that are spaced uniformly and that will be perpendicular to the surfaces of the conductors.

There is a uniform electric field of about 150 Newtons per Coulomb directed toward the earth and surrounding the earth. This electric field is caused by the ionosphere, which is a layer of charged particles about 100 kilometers above the Earth’s surface. The ionosphere will be positive when the weather is good. When storm clouds gather, it creates an increase in the local electric field because the air is ionized, getting discharged in the form of lightning during a storm.

On uneven surfaces, there will be a clustering of charges on the sharpest points of the surface. Excess charges will tend to move off or on the conductor at the sharpest points. Figure 112 shows the concentration of charge on an uneven surface with an increase in the charge at the sharpest point:

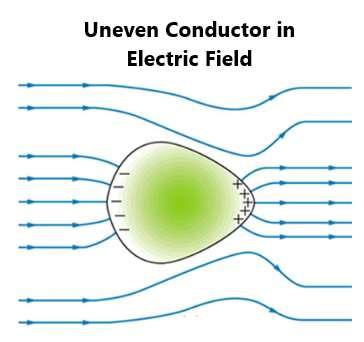
Figure 112.

This brings us to the issue of a very pointy conductor, such as a lightning rod. They work best when they are pointed because they can produce an opposite charge to the large charge in a storm cloud, attracting the charge and bleeding the charge away from a building rather than allowing that charge to be picked up by. On the other hand, the conductor that is smooth with a large radius will prevent the leakage of charge into the air.

A Faraday cage is a metal shield that will enclose a volume. All electrical charge will reside outside the cage and not in the cage. It is used to prevent stray electric fields from the outside of the cage causing interference with sensitive instruments within the cage. During an electrical storm, it is better to stay inside a car than outside because the car body acts like a Faraday cage so that there is zero electrical field inside the cage. The same is true if an electrical wire falls on a car during driving.