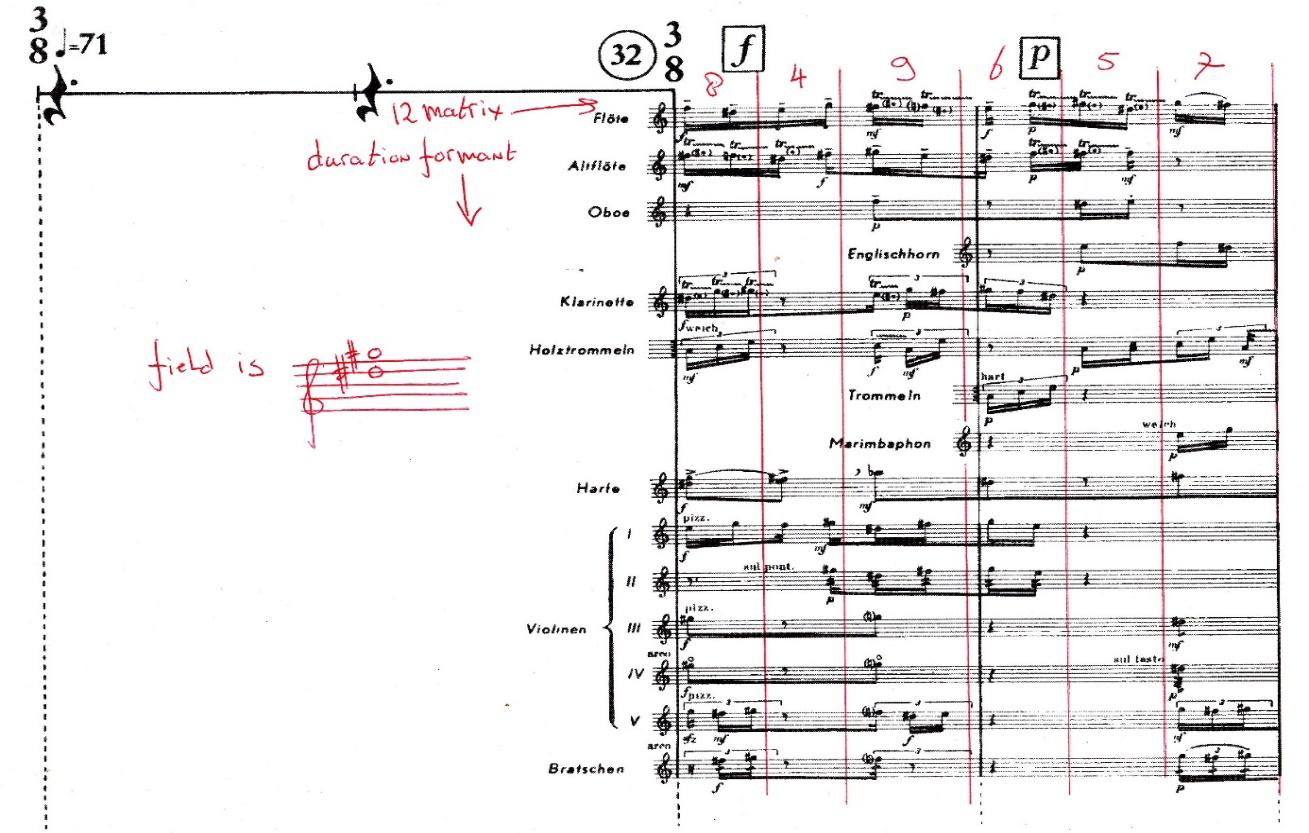
5.3.5.2 The pitch matrix

10 4 14 5 12 2 1 3 9 7 8 11 6 8 2 12 3 10 14 13 1 7 5 6 9 4 9 3 13 4 11 1 14 2 8 6 7 10 5 12 6 2 7 CHAPTER V - YOUNG MUSIC, MODERNISM AFTER WORLD WAR II14 4 3 5 11 9 10 13 8 7 1 11 2 9 13 12 14 6 4 5 8 3

Repetition number 12 (orchestra 2) now has the distribution of the duBlz 247rational formant: 12 8 1 10 9 11 5 3 4 7 2 6 12 12 12 12 12 12 12 12 11 11 11 11 11 10 10 10 10 10 10 10 10 10 9 9 9 9 9 9 9 9 9 9 9 8 7 7 6 6 6 6 6 6 6 6 6 6 6 6 5 5 5 4 4 4 4 4 4 4 3 3 3 3 2 2 2 2 2 2 1 1 1 1 1 1 1 1 1 1

Likewise, the second 12 matrix durational formant (rep number 32) forms the sequence: Likewise, the second 12 matrix durational formant (rehearsal number 32) forms the sequence: 8 4 9 6 5 7 1 11 12 3 10 2

Just as in the following pitch material the eventual rhythmic content of the lines that form the durational formant is relatively free.

This analysis has shown that the durational formants did not arise from drawing the contours of the mountains surrounding Davos, as Stockhausen was fond of telling (see example on page 241). At most, these sketches were helpful in making the final choice of the values he would use to define the density of the formants. The densities themselves, however, were arrived at serially.

5.3.5.2 The pitch matrix In order to properly understand the pitch structure of Gruppen we have to go back to the last part of …wie die Zeit vergeht… Here Stockhausen introduces the term Feldkomposition, by which he makes a connection between clearly observable pitches and the not exactly audible ones, the moment at which the punctual listening changes into statistic listening. In the case of the duration ordering this would be a durational formant that is observed as so complex that it can no longer be reduced to its components. In analogue terms this also applies to tones: in a complex sound the field sizes of that sound dominate and can no longer be reduced to the relation between the different tones. Seen as such, Gruppen is a Feldkomposition.

The durational formants consist of a sum of many anti-metric figures, with often also two or more tempi sounding simultaneously. Logically, Stockhausen regards the pitches also as statistic field ranges and that is why he assumes that the pitches per group must first be assigned a field or field range within which the pitches can move. He developed these fields from the Allintervallreihe: first, he wrote all 144 notes of the overall structure (see Ex. 38, colour page XIV) in retrograde, one octave higher.145 These octavised pitches always mark the upper limit of the range. The 144th octavised tone defines the upper limit of the range of the first group, the octavised 143rd tone that of the second group, and so on.

Then the interval relation between tones 144 and 143 (13:9) defines the lowest tone from the size of the first group. This becomes d-g . De g becomes the upper tone of the range of the second group. This tone, number 143, has an interval ratio of 2/9 with tone 142. This makes the note f , a none and an octave lower, and so on. Ex. 40: The tone fields of the first 14 groups.

The horizontal lines that eventually end up in the score have much in common with Klavierstück 1, in the sense that they are written freeœ ly and quickly fill in the chromatic total of the given range of a group. There’s also a second type of notation, which does not appear in Klavierstück 1. It is the one in which the instrument quickly goes back and forth between a limited number of tones, thus contributing more to the emergence of a sound field.

Stockhausen does follow the general structure of this range per group but is not very strict about it. We frequently find tones that fall outside of this structure. I was actually surprised to find that the pitches of the work were seen as part of a field and had only a derivative relation with the basic row of the work.

1 2 3 4 5 6 V 9 10 11 12 12 a b 13 14 15 √

œ œ# œ# #œ

œ œ œb œb œ œ# œ œœ œ œ# √ œ œ# œ œ # # œ œ# œ œb

œ# œ œ

2 2 2 2 2 2 1 1 1 1 1 1 1 bœ œ 2

9 2 11 13 7 5 4 8 6 12 3 10 10 9 _ _ _ _ _ _ _ _ _ _ _ _ _ _ 13 9 3 4 10 6 12 7 11 5 2 8 4 12

Pf & œ œb œ œb œ œb œ œ œn œb œ œ œnœ# œ œ œb œœ# œb œ œbœ# œn œb œn 1 2 1 2 3 2 1 3 4 2 3 1 4 5 1 4 2 3 5 6 1 2 5 4 6 1 7

145The 145th group was conceived as a final group from the beginning and therefore stands apart from the overall structure.

Ex. 41: The first and second group from the 12 matrix.