Particle Accelerators

BY OLIVIA DANNER

One of the fundamental questions of physics, and science in general, boils down to understanding the nature of the universe in its entirety. One facet of answering this question is studying the origins of the universe, back when everything was high-energy atomic soup full of particles colliding to make different, bigger particles. The key to being able to study this was the particle accelerator, which first allowed us to finally understand the structure of the atom, and later to create new particles.

As a straightforward first definition, particle accelerators are exactly what their name implies: “electrical devices that accelerate charged atomic or subatomic particles to high energies” (1). There are two ways to classify particle accelerators: by structure – linear accelerators (commonly known as linacs) or circular accelerators – or the method of acceleration – electrostatic or electrodynamic (2). Electrostatic means that unchanging electric fields are used to accelerate particles, while electrodynamic refers to the use of electromagnetic fields of changing direction and flux. The former are much easier to generate, so this was the method used for early accelerators (3). The very early x-ray machine is considered the first particle accelerator: an evacuated glass tube with a negatively charged cathode at one end and a positively charged anode at the other (4). Heating up the cathode excites the electrons in the metal enough for them to be released into the tube, while the potential difference between the cathode and anode causes the newly-freed electrons to be accelerated towards the positively-charged anode. Upon striking it, the electron is rapidly decelerated and releases an x-ray (4). Unfortunately, there is a limit to the amount of voltage that can be used to accelerate the electrons – too much and the system gets “spurious discharges” (4) which then short out the system. This led to the development of linacs, which accelerate the particle over a series of potential differences rather than attempting to use one massive potential difference (4). To better explain, imagine that an accelerator is a wind tunnel, the particles are paper airplanes, and the wind is our potential difference. Take two scenarios. In one, a single fan at the beginning of the tunnel is the only propulsion the planes receive to reach the end of the tunnel. In the other, a tunnel of equal length is split into sections and a fan is placed at the beginning of each section. The fan in the first scenario would have to be incredibly strong in order to get the planes to the end. The second scenario requires more fans, but they do not need to be very high-power – the boost to the planes is renewed each time they enter a new section.

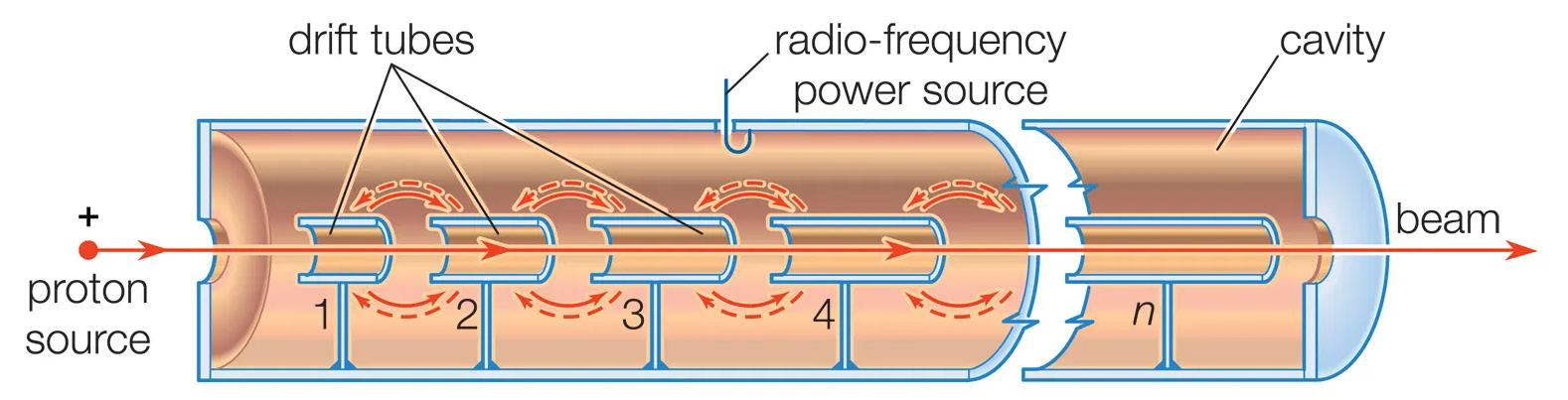
Now suppose each fan gives the planes a single boost at the beginning of each tunnel section, then turns off. This is the basic principle for a linac (4). Within a series of tubes, particles can drift through at a constant velocity until they hit the next field and receive a boost in speed. If they start off too slow, the entire accelerator will have to be incredibly long to get them to reach the desired energy, so an electrostatic accelerator is used to give the particles a jumpstart before entering the linear accelerator (4).

The first linac used a Wideroe structure, which has the electric fields flip direction while the particle is drifting, then flip again once the particle reaches the end of the tube. This is equivalent to changing the direction of the fans in our wind tunnel example. If the wind is blowing opposite the direction of the planes’ flight, it slows them down and we can consider this the ‘wrong’ direction. This is counter to the goal of acceleration but a product of the way the fields are generated, so it is important that the flip occurs while the particles are inside the tube, where they are unaffected by the fields. This also means that the particles get accelerated in bunches, resulting in a pulsed beam (5). Although G. Ising was the one to conceive the idea in 1925, it was Wideroe who executed it in 1928 (6). Around twenty years later, in the 1940’s, Luis Alvarez developed a new structure which was named after him (6). It operates similarly to the Wideroe structure, but it has the electric fields pointing in the same direction between every tube, allowing the particles to all be accelerated simultaneously (5). This was made possible by his use of a radio frequency (rf) generator, which can flip the direction of the electric fields at every tube rather than every other, like in the Wideroe structure (6). Both of these structures tend to be used for heavier particles, such as protons (3). Turning away from oscillating fields and into the realm of waves, two other types of linac structures enter the discussion: travelling and standing wave structures. If you take a piece of string and shake it, that motion will propagate through the length of the string. Now pick a point on the string: for a travelling wave, that point will appear to move along the string. For a standing wave, the point will simply appear to move up and down, like a bouncing ball. Waves can be used to accelerate particles that travel near the speed of light in a vacuum, so long as their phase velocity does not exceed the speed of light. This is akin to a traffic-heavy highway: groups of cars moving at similar speeds will be bunched up together. The speed of the individual cars (phase velocity) can be faster than that of the total group (group velocity). The speed limit ensures the phase velocity stays below a certain value, which is the role a waveguide plays in the accelerator.

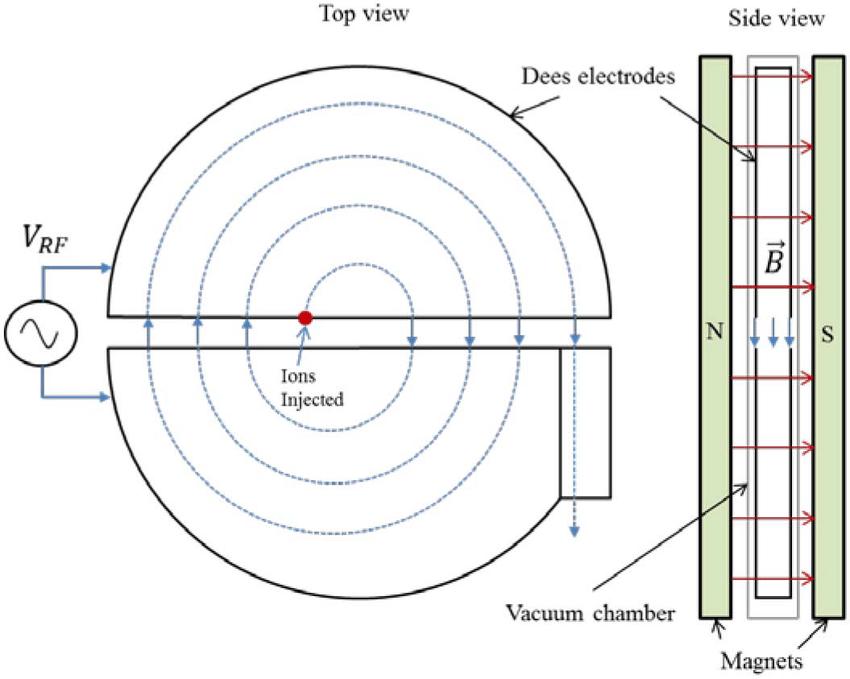
So, using a waveguide to reduce the phase velocity to one in which the particles can stay together, a travelling wave structure essentially carries particles with it and accelerates them that way. The standing wave structure accelerates particles in a similar way to the linacs previously discussed: when the peak of the wave is at a maximum, the electric field points in the direction of travel and gives the particle a boost (5). These wave-based structures tend to be used for electrons (3). The travelling wave structure is the basis for the Stanford linear accelerator at SLAC which, at two miles long, is the longest accelerator in the world (6). A fifth type of linac is the induction linac, which allows for much more beam current, energy, and pulse length than conventional linacs offer (7). This is achieved through a series of doughnut-shaped magnets with the beam passing through their center. Each magnet pulses successively, which exerts a force on the particles and causes them to accelerate (5). While linacs fix the issue of the system shorting out due to high voltage, they do pose new problems. Each tube in the accelerator has to be successively longer to ensure that the particle hits the electric fields at the right time, so linacs either have to be very, very long – which leads to the obvious issue of space – or the rate at which the electric fields switches direction has to be very high, which has a technological limitation. These issues mean that linacs are limited to energies of around 200 MeV, though there are ones that reach much higher energies; these reach thousands of feet in length (3). The alternative is a circular accelerator, which uses a magnetic field to bend particles’ paths into an ever-expanding spiral. The first and most commonly known of the circular accelerators is known as the cyclotron. The general setup of a cyclotron is an ion source placed between a pair of D-shaped electrodes placed so their flat sides are aligned (5). The particles that are to be accelerated are also between the two poles of an H-shaped magnet, which provides a uniform magnetic field that bends the particles’ paths into a circle so the particles drift through the D electrodes at constant speed (5). An electric field between the two electrode flats means the particles accelerate through the gap and the field reverses direction while the particles are drifting through the electrodes, which shield them so they are not slowed down. (3). The shape of the cyclotron means that particles cannot be injected into it like with linacs; this would lead to the particles simply smashing into the nearest wall before the magnetic field can cause them to start bending. Placing a vacuum chamber filled with gas in the center of the setup, then ionizing the gas, allows the particles to exit the chamber already under the influence of the magnetic field, giving them that initial circular motion (4). Extracting the particles poses a similar question of how to get particles engaged in circular motion to exit linearly. The solution is to have an electrode bending the particle beam so it leaves the cyclotron tangential to its curvature (4). This does run into the issue of some particles colliding with the electrode before they can be bent, so the beam that leaves the cyclotron is weaker than within the accelerator (4). Along with the weaker exiting beam, cyclotrons also have an upper limit on the speed the particles can reach due to two factors: the strength of the magnetic field has to decrease with radius so the particles maintain a stable orbit, and the voltage that generates the electric field has to be high lest the particles get out of phase with the cycle (7).

Following down an alternative route from the cyclotron leads to the betatron which, rather than direct particles out of the machine, smashes electrons into a target to generate x-rays (4). Unlike the cyclotron, which uses an electric field between the two D-electrodes to accelerate particles, the betatron uses magnetic fields to accelerate particles – two, in fact (3). One keeps the electrons in orbit (the guide field), while the other induces an electric field in the same direction as the electrons’ motion (3). Used in medical imaging and for their x-ray generations, betatrons are not in particularly high use at this point because linacs are lighter (some betatrons reach over 300 tons), reach higher energies, and are in general more easily controlled (7).

Another circular accelerator is the synchrotron, which combines the cyclotron’s fluctuating electric field with the betatron’s guide field (3). It matches the magnetic guide field to the momentum of the particles and the accelerating electric field to their frequency (7). The electric field sorts out the particles that are moving at the right speed to be accelerated (8). There are two ways to inject particles into a synchrotron. The first, used only when accelerating electrons, is to run the synchrotron like a betatron (without the fluctuating electric field) until the electrons reach the desired speed, then causing the electric field to fluctuate, as in regular synchrotron operation (3). The other, more common way is to use an electrostatic generator or a linac to pre-accelerate the particles, which means that the difference in frequency of the particles entering the machine and ex- iting is smaller than if the particles were accelerated from scratch (3). Extracting particles is different from a cyclotron or betatron and, like injection methods, there are two extraction methods. One uses magnets to guide exiting particles (3), while the second uses a pair of targets – a ‘jump target’ and the main target –and a magnet to guide the particle beam out. On their first pass, the particles lose enough energy to contract their orbit so their second pass leads them through the main target, which causes another drop in energy that decreases their orbital radius (3). On their third lap, the particles pass through the magnet, which then directs them out of the accelerator (3).

The main benefit of the synchrotron is cost reduction – the magnets used in cyclotrons are large and expensive, and the synchrotron only needs a magnetic field in the region of the orbit (8). The main drawback is synchrotron radiation which, despite its name, is not unique to the synchrotron; rather, it plays a role in all high-energy colliders that use a curved path. Energized particles under the influence of the magnetic field are constantly decelerating perpendicular to their curved path, which causes radiation (8). This is less of a problem with lower-energy particles because of the relationship synchrotron radiation has with energy (8). The energy lost to radiation is restored by the electric field, which is constantly accelerating the particles in a circle, meaning synchrotron radiation is less of a problem for lower-energy systems (8). At high energies, the acceleration is not enough to balance out the energy lost (8).

Particle accelerators are still being developed and refined to achieve even higher energies, to be smaller, to be cheaper. There are many more, old and new and obsolete-ish, that fall under the umbrella of linear and circular accelerators, and discussing all of them would take more space than is available for this paper. In terms of new accelerators, attention largely seems to be directed towards circular accelerators as the machines that have the potential to achieve higher energy in relatively less space. Developments will continue to be made, accelerators will become higher and higher energy (and hopefully lower in cost and size), and maybe one day we’ll be one step closer to replicating some of the conditions of the beginning of the universe.