Muestra del libro Mathematics 2º ESO Proyecto 5 etapas. Bruño

José María Arias Cabezas Ildefonso Maza Sáez

A guide to your book

With the book you are holding you will:

➜ Learn Maths using the 5-stage methodology: Engage, Explore, Explain, Elaborate and Evaluate, the same method used in the Spanish book.

➜ Consolidate and improve your English proficiency through activities where you will practise both written and oral comprehension and expression.

The following information is given:

1. The content of the block and the 5 stages:

➜ Engage: a stimulating GeoGebra applet related to the core concepts covered in the block.

➜ Explore: an interactive and dynamic activity based on the applet that lets you explore your current knowledge and relate it to the concepts you will be studying.

➜ Elaborate: a section to investigate and apply the concepts.

2. A learning situation with two phases:

➜ Explore: searching for information on a concept to be worked on.

➜ Elaborate: a short writing activity to present the information obtained.

Main content

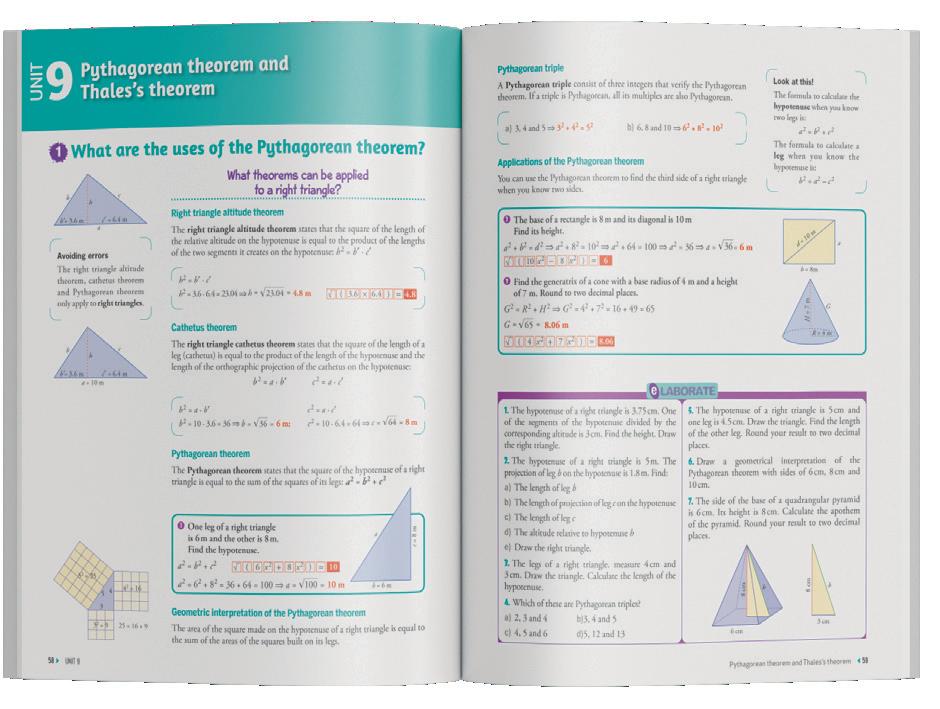
Each unit’s double page contains the Explain stage where the core concepts are presented. This is supported by a solved exercise or problem to illustrate the mathematical concept. The exercise is solved with straightforward operations so the students can focus on the new content being covered. The final Elaborate section has exercises and problems to enhance and consolidate the acquired knowledge.

Learning experience

A comprehensive learning experience will enable you to develop all the key competences and core concepts you have acquired, as well as enhance your teamwork skills. Additionally, you will be able to communicate the results in a creative manner, using different formats.

Self-evaluation

The last step in the 5-stage methodology is Evaluate, which has two parts:

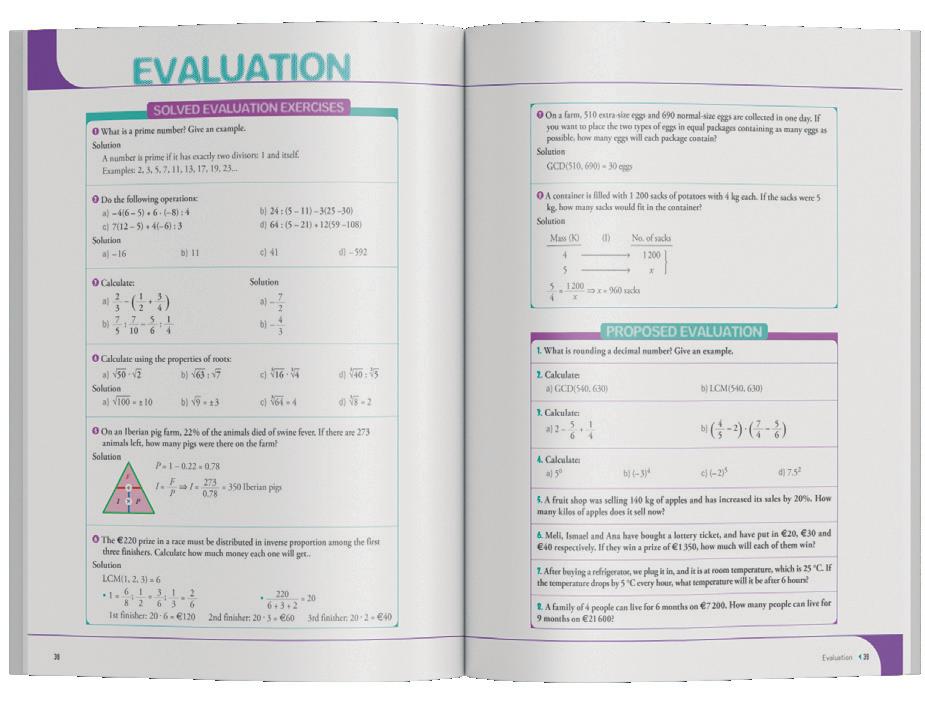
➜ A solved evaluation that serves as a review of the content covered.

➜ A proposed evaluation covering the block’s content.

Learning experience

At the end of this module, you will find a global learning situation that will allow you to work in teams. You will need to apply all the core concepts you have acquired. In addition, you will be able to communicate the results in a creative manner, using different formats.

e e e e

1 What is an equilateral cylinder? Search for information.

2 Write a few sentences with the information you have obtained.

UNIT 8 Systems of linear equations

What is a linear system? 1

Linear equations

Note that linear equations are first-degree equations.

• With one unknown: 2x = 5

• With two unknowns: 3x + 2y = 7

• With three unknowns:

How do we solve a system by graphing?

Linear equations with two unknowns

A linear equation with two unknowns is an algebraic expression in this form: ax + by = c

x and y are the unknowns, a and b are the coefficients of the unknowns, and c is the independent term.

The solution to a linear equation is a pair of values (x, y) that verifies the equation.

Linear equations with two unknowns have infinite solutions.

The graphic representation of the solutions for linear equations with two unknowns is the set of all points on a line.

Find a third point to verify that they are in a line.

B(– 1, 5)

2x – 3y + 5z = 4 Table

2x + y = 3 X Y

A(2, –1)

Every point on the line is one of the infinite number of solutions to the equation.

Procedure for plotting the line:

a) Solve for the unknowns that are easiest to isolate.

b) Create a table with the values.

c) Mark the two points on coordinate axes.

d) Connect the points with a line.

Graph the solutions to the equation: 2x + y = 3 ⇒ y = 3 – 2x x

Linear systems

A linear system of two equations with two unknowns is an algebraic expression in this form:

ax + byc = 3

a ′ x + b ′ y = c ′

The solution to the system is a pair of values ( x , y ) that confirms the two equations.

1 Check if x = 3, y = 2 is a solution to the equation:

Two linear systems are equivalent if they have the same solution.

Graphic solutions to linear equations

a) Plot the line representing the first equation on the graph.

b) Plot the line representing the second equation on the graph.

c) The solution is the point where the two lines intersect.

2 Solve the system graphically: –21 x xy 2 4 + = y = 3 x

Table of values:

Table of values:

Classification of linear systems

a) Independent consistent system. The system has one solution. The two lines intersect at one point.

b) Dependent consistent system. The system has infinite solutions. The two lines are the same.

c) Inconsistent. The system has no solution. The two lines are parallel.

3 Solve the system graphically. Classify it:

Table of values:

Table of values:

1. Graph the solutions to the equation:

2. Graph the solutions to the equation: x – 2y = –3

3. The difference of x and y is 1. Write an equation for this. Find five pairs of numbers that verify the equation. Graph the set of solutions.

Organising your notebook

Create the table for the first equation on the left. Plot your results in the centre. Create the table for the second equation on the right. Graph your results in the centre.

Graphic interpretation

The two lines are parallel. There are no intersection points.

4. Solve the systems graphically:

Are they consistent or inconsistent systems?

How do we use substitution, reduction, and equalisation methods to solve systems? 2

Substitution method

When one of the unknowns in a system is already found, you can solve it easily with the substitution method.

a) Put the value of the solved unknown into the other equation.

b) Solve the resulting equation.

c) Put the value you obtain back into the first equation.

4 Solve the system by substitution:

––yx xy 82 54 7 = = 3

a) Put the value of y from the first equation into the second equation. 5x – 4(8 – 2x) = 7

b) Solve the resulting equation. 5x – 32 + 8x = 7 13

c) Put the value you obtain for x back into the first equation.

Equalisation method

When the same unknown is solved in both equations, you can solve the equations easily with the equalisation method.

a) Put the values of the solved unknown into an equation.

b) Solve the resulting equation.

c) Put the value obtained into the simpler equation that is solved for one of the unknowns and solve.

5 Solve the system by equalisation:

a) Put the values of y into an equation.

b) Solve the resulting equation.

––5 4 x y y x 3 9 = = 3

c) Put the value you obtain back into the simpler of the two equations where the other variable is solved.

Solution: x = 2, y = –1 P

5 – 3x = 4x – 9

– 3x – 4 x = – 9 – 5

– 7x = –14

7x = 14 ⇒ x = 2

Reduction method

a) Using multiplication, make a new system in which one of the unknowns has opposite coefficients in the two equations.

b) Add the two equations together.

c) Solve the resulting equation.

d) Put the value obtained into the simpler equation and solve to find the value of the second unknown.

What kind of coefficients are easy to solve with the reduction method?

a) Equal coefficients. Subtract one equation from the other.

b) Opposite coefficients. Add the two equations.

c) One coefficient is a multiple of the other. Multiply the equation with the smaller coefficient by a number that will make the two coefficients opposites.

6 Solve by reduction: –– xy xy 76 11 53 14 += += 3

a) Change the sign of the first equation and multiply the second equation by 2. –– – xy xy 176 11 10 628 = += 3

b) Add the two equations.

c) Solve the resulting equation.

d) Put the value obtained into the simpler equation and solve to find the second unknown. x

Solution: x = 1, y = 3

5. Solve the system with the best method:

6. Solve the system with the best method:

7. Solve the system with the best method:

– 3 xy xy 11 +=24 = 3

8. Solve the system with the best method:

y 6= ––xy x 23 4 517 += 3

x = 1

Which method should I use?

a) When one of the unknowns in a system is already cleared, you can solve it with the substitutions method.

b) When the same unknown is solved in both equations, you can solve the system with equalisation method.

c) When there is no cleared unknown in any equation, you can solve the system with the reduction method.

Note

The coefficients of unknown y are multiples of each other. To obtain opposite coefficients change the sign of the first equation and multiply the second equation by 2.

9. Solve the system with the best method:

– –xy xy 23 11 54 30 = = 3

10. Solve the system with the best method:

y 7= –––xy x 35 12 425 += 3

11. Three kilos of apples and two kilos of oranges cost

€9. Two kilos of apples and two kilos of oranges cost

€7. How much does a kilo of apples and a kilo of oranges cost?

12. Two pairs of trousers and three shirts cost €120. Three pairs of trousers and two shirts cost €130. How much does each item of clothing cost?

How do we solve problems using systems? 3

Names of unknowns

If the names of the two objects start with different letters, use their first letters as unknowns. Or you can use x and y

If you know which value is smaller and which is larger, make the value of the smaller unknown x and the larger unknown y

What is the procedure to solve problems with systems?

To solve a problem, read the statement carefully until you know the unknown, the data, the relationships and the questions. Always make a drawing for geometric problems. Always make a diagram for numerical problems. Then follow the steps below.

1. Unknown: write down the data and the unknowns.

2. Questions: write down the questions.

3. Approach and working out: write the relations or conditions into a system of equations to be solved. Solve the system.

4. Solution and verification: write the answers to the questions, verify that they make sense and comply with the given relations.

Geometric problems

7 A tennis court is a rectangle. The perimeter is 64 m. The base is three times the height. Find the dimensions of the tennis court.

Unknowns

h = length of height

b = length of base

1. Unknown:

h = height

3. Aproach and working out:

Height + base = 32 ⇒ h + b = 32

base = 3 · height ⇒ b = 3h

h + b = 32 3 Equations System

Their sum is 32 h+b = 32 b = 3h

The base is three times the height.

b = base h b

2. Questions:

• The sum of the height and base is 32 m

• The base is three times the height.

• What are the lengths of the height and the base?

Equations:

h + b = 32 }⇒

b = 3h

Solve by substitution:

h + 3h = 32 ⇒ 4h = 32 ⇒ h = 8 Substitute

h = 8 in b = 3h ⇒ b = 3 · 8 = 24

b = 3h

3. Solution and verification

The height measures: 8 m The base measures: 24 m

Sum of height and base: 8 + 24 = 32 m

The base is three times the height: 3 · 8 m = 24 m

Numerical problem

8 Ana purchases 4 shirts and 3 pairs of trousers for €100. Oscar buys 2 shirts and 3 pairs of trousers in the same shop for €80. How much does each item of clothing cost?

1. Unknowns:

s = price of a shirt

t = price of a pair of trousers

How much does each item of clothing cost?

2. Question:

What is the price of each shirt and each pair of trousers?

3. Aproach and working out:

4 shirts + 3 pairs of trousers = 100

4s + 3t = 100

2 shirts + 3 pairs of trousers = 80

2s + 3t = 80

System:

Unknowns

h = length of height

b = length of base Equations

4s + 3t = 100 }

2s + 3t = 80

It is solved by reduction because there is no known unknown:

Subtract the second equation from the first equation.

2s = 20

Dividing by 2 we obtain: s = 10

Substitute s = 10 in the 2nd equation:

2s + 3t = 80 ⇒ 2 · 10 + 3t = 80

20 + 3t = 80

3t = 60

Dividing by 3 we obtain: t = 20

4. Solution and verification:

A shirt costs €10 A pair of trousers costs €20

4 shirts and 3 pairs of trousers cost: 4 · 10 + 3 · 20 = 40 + 60 = €100

2 shirts and 3 pairs of trousers cost: 2 · 10 + 3 · 20 = 20 + 60 = €80

13. The sum of two numbers is 20. Two times the first number plus three times the second number is 45. Find the value of both numbers.

14. There is a mixture of chickens and rabbits in a pen. The total number of animals is 80. The total number of legs is 220. How many chickens and how many rabbits are in the pen?

15. A mixture contains olive oil that costs €3 per litre and sunflower oil that costs €1 per litre. The price paid for 20 litres of the mixture is €2.50 per litre. How many litres of each oil are in the mixture?

4 shirts and 3 pairs of trousers cost €100

2 shirts and 3 pairs of trousers cost €80

4s + 3t = 100 2s + 3t = 80 2s

16. Ana’s age is three times the age of her daughter. Five years earlier Ana was five times older than the daughter. How old are Ana and her daughter now?

17. The longer side of a rectangle is three times the shorter side. Its perimeter is 40 m. Find the lengths of the sides of the rectangle.

18. In a shop, 5 ham sandwiches and 2 colas cost €17 and 3 ham sandwiches and 7 colas cost €16. How much does a ham sandwich cost? And a cola?

UNIT 11 Areas and volumes

How do we calculate the area and volume of orthohedrons, prisms and cylinders?

What are the formulas for finding the area and volume?

Area and volume of an orthohedron

• You can see the area of an orthohedron in its 2-dimensional net, which is made of 6 rectangles (3 pairs of identical rectangles).

A = 2(ab + ac + bc)

• To find the volume of an orthohedron, multiply its length by its width by its height.

V = abc

1 Calculate the area and volume of an orthohedron with 5 cm, 3 cm and 2 cm sides.

A

• You can see the area of a prism in its 2-dimensional net. It is made of two identical regular polygon bases and a number of identical rectangles equal to the number of sides of the base.

AT = 2AB + AL

• To find the volume of a prism, multiply the area of its base by its height.

V = ABH

2 Find the area and volume of the pentagonal prism.

b) AL = 5 ℓ H ⇒ AL = 5 · 4 · 8 = 160 m2

Therefore: AT = 2 · 27.5 + 160 = 55 + 160 = 215 m2 Volume:

• You can see the area of a cylinder in its 2-dimensional net. It is made of two equal circular bases and a rectangle.

AB = π · R 2 AL = 2πRHAT = 2AB + AL

• To find the volume of a cylinder, multiply the area of its base by its height.

V = AB · H

3 Calculate the area and volume of the cylinder shown in the figure. Use π = 3.14

Total area: AT = 2AB + AL

a) AB = πR 2 ⇒ AB = 3.14 · 32 = 28.26 m2

b) AL = 2πRH ⇒ AL = 2 · 3.14 · 3 · 7 = 131.88 m2

Therefore: AT = 2 · 28.26 + 131.88 = 188.40 m2

Volume: V = AB · H ⇒ V = 28.26 · 7 = 197.82 m3

1. Draw an orthohedron with dimensions of 5 m, 3.5 m and 4 m. Find its area and volume.

2. Draw a quadrangular prism with a base side of 3 cm and a height of 8 cm. Find its area and volume.

3. Draw a straight cylinder with a base radius of 3 cm and a height of 6 cm. Find its area and volume. Use π = 3.14. Round your result to two decimal places.

Volume of prisms and cylinders

Since all prisms and cylinders have two equal bases, you can calculate their volume using the same formula.

V = AB · H

4. Calculate the area and volume of the hexagonal prism below. Round your result to two decimal places.

6 m

2 m

5. A milk container is an orthohedron with dimensions of 8 cm, 5 cm and 25 cm. Draw it. Calculate its volume in litres.

How do we calculate the area and volume of pyramids, cones and spheres? 2

What are the formulas for finding the area and volume?

Volume of pyramids and cones

Since all pyramids and cones have a base and a vertex, you can calculate their volume using the same formula.

Nets of a sphere cannot be made because it does not have any flat surfaces.

Area and volume of a pyramid

• You can see the area of a pyramid in its 2-dimensional net. It is made of a regular polygon and a number of isosceles triangles equal to the number of sides of the base.

AT = AB + AL

• To find the volume of a pyramid, multiply the area of its base by its height by one-third.

V = 3 1 AB · H

4 Calculate the area and volume of a quadrangular pyramid with a base side of 6 m and a height of 8 m. Round your result to two decimal places.

Total area: AT = AB + AL Calculate the area of the base and sides:

Calculate the apothem of the pyramid; h = 38 73 22+= = 8.54 m A

ℓ

Area and volume of a cone

• You can see the area of a cone in its 2-dimensional net. It is made of a circular base and a circular sector.

AB = πR 2

AL = πRGAT = AB + AL

• To find the volume of a cone, multiply the area of its base by its height by one-third.

V = 3 1 AB · H

5 Find the area and volume of a right cone with a radius of 5 m and a height of 12 m. Round your result to two decimal places. Use π = 3.14

Total area: AT = AB + AL. Calculate the area of the base and sides:

AB = πR 2 ⇒ AB = 3.14 · 52 = 78.5 m2 AL = πRG

Calculate the generatrix. G = 512 169 22+= = 13 m

AL = 3.14 · 5 · 13 = 204.1 m2

Therefore: AT = 78.5 + 204.1 = 282.6 m2

Spheres do not have 2-dimensional nets.

• The area of a sphere is equal to four great circles:

A = 4πR 2

• To find the volume of a sphere, multiply π by the radius cubed by four-thirds.

V = 3 4 πR 3

6 Find the area and volume of a sphere with a radius of 5 m. Round your result to two decimal places. Use π = 3.14

A = 4πR 2 ⇒ A = 4 · 3.14 · 52 = 314 m2

V = 3 4 πR 3 ⇒ V = 3 4 · 3.14 · 53 = 523.33 m3

6. Draw a quadrangular pyramid with a base side of 10 cm and a height of 12 cm. Calculate its area and volume.

7. Draw a right cone with a radius of 6 m and a height of 8 m. Calculate its area and volume. Round your result to two decimal places. Use π = 3.14

5 m

8. Draw a sphere with a radius of 6 cm. Find its area and volume. Round your result to two decimal places. Use π = 3.14

9. You make a methacrylate decoration in the shape of a hexagonal pyramid. Its base is 4 cm wide and its height is 12 cm. The methacrylate costs €28.50/m2. Draw the object and calculate the price of the materials. Round your result to two decimal places.

What are trucated pyramids and truncated cones? 3

What are the formulas for finding the area and volume?

Area and volume of a truncated pyramid

• You can see the area of a truncated pyramid in its 2-dimensional net. It is made of two unequal regular polygonal bases and a number of isosceles trapezoids equal to the number of sides of the base.

AAAA TBBL 12 =+ +

• To find the volume of a truncated pyramid, multiply the sum of the areas of the bases plus the square root of the product of their areas by one-third, and then multiply everything by its height. V

7 Find the area and volume of a square truncated pyramid with a longer base side of 16 m, a shorter base side of 4 m and a height of 8 m

Total area:

AT = AB1 + AB2 + AL.

Calculate the area of the bases and sides:

• AB1 = ℓ12 ⇒ AB1 = 162 = 256 m2

• AB2 = ℓ22 ⇒ AB2 = 42 = 16 m2

• AL = 4 · ℓ1 + ℓ2 2 · h

Calculate the apothem of truncated pyramid h:

h = 100 6822+= = 10 m

AL = 4 · 2 16 4 + · 10 = 400 m2

Therefore: AT = 256 + 16 + 400 = 672 m2

Volume:

Area and volume of a truncated cone

• You can see the area of a truncated cone in its 2-dimensional net. It is made of two unequal circular bases and a circular trapezoid.

AT = AB1 + AB2 + ALAB1 = πR 2 AB2 = πr 2 AL = π(R + r)G

• To find the volume of a truncated cone, multiply the sum of the areas of the bases plus the square root of the product of their areas by one-third, and then multiply everything by its height.

V = AAAA 3 1

BBBB 12 12 ++ `j · · H

8 Find the area and volume of a truncated cone with a larger base radius of 15 m, a smaller base radius of 5 m and a height of 24 m. Round to two decimal places. Use π = 3.14

Total area: AT = AB1 + AB2 + AL

Calculate the area of the bases and sides:

• AB1 = πR 2 ⇒ AB1 = 3.14 · 152 = 706.5 m2

• AB2 = πr 2 ⇒ AB2 = 3.14 · 52 = 78.5 m2

• AL = π(R + r)G

Calculate the generatrix. G = 24 10 22 + = 26 m

AL = 3.14 · (15 + 5) · 26 = 1632.8 m2

Therefore: AT = 706.5 + 78.5 + 1632.8 = 2417.8 m2

Volume:

V = AAAA 3 1 BBBB 12 12 ++ `j · H V = 706.5 · 78.5 `j 706.5 + 78.5 + 3 1 · 24 = 8164 m3

10. Draw a square truncated pyramid with a longer base side of 18 m, a shorter base side of 8 m and a height of 12 m. Find its area and volume.

11. Draw a truncated cone with a height of 12 m, and base radii of 10 m and 4 m. Find its area and volume. Use π = 3.14

12. Calculate how much water fits in the bucket shown in the figure.

Use π = 3.14 20 cm

Packaging

You may have seen a variety of product packaging displayed in different shops. Perhaps the most widely used packaging shapes are prisms and cylinders.

Let’s take a look at cylinders.

To begin with, let’s read the following excerpt from the book Oranges and Penguins.

«[...] —Do you like this perfume bottle? It’s very elegant, isn’t it?

—Yes, stylish and very elegant.

—It’s a violet perfume. Don’t you think it’s too small? I’ve spent all my money...

—No, it’s not small! Besides, it’s designed precisely to look big.

—I don’t understand, grandfather, is it small or big?

—It is small in terms of volume and large in terms of surface area. It is like all bottles that have to contain expensive liquids. They are narrow and tall. On the other hand, have you noticed bean jars or, better still, paint pots? What are they like? Narrow and tall?

—No. Those are wide and short.

—Exactly.It´s all a question of volume, Filo. Come on, give me a sheet of paper. I want to make up pose a riddle to you. Tell me: how should I roll it up to make a cylinder with more capacity, lengthwise or widthwise?

—I think it doesn’t matter; the sheet is the same.

—Are you sure? I don’t think so, I think it DOES matter. So, how do we roll it up?

—We could do a test. We could roll it both ways and fill them both with something. That way we could compare and see which cylinder has a greater capacity.

—That’s the attitude! That’s the scientific method. So, here’s what we do: we take two identical pieces of construction paper, roll one lengthwise and the other widthwise, and tape the two ends together. Now take the scale...

—Yes, yes, I’ve got it... I can go on. You know what I’m going to do? I’ll put the cylinders, first one and then the other, on the plate of the scale. I’ll fill them with... I don’t know... with sugar, and then I’ll see which one is heavier.

[…] —I’m smart, don’t you think so, grandfather?

—Very clever. I’m sure that sooner or later you’ll be able to prove it rigorously too, using some formula. Now come with me, let’s get that can of red paint we used to fix up your desk. You will discover something very interesting.

—Take the ruler and measure the height of the can.

—Let’s see... the can is... 12 centimeters high.

—I want to make a bet: I’m sure the diameter of the base is the same, 12 centimeters. Can you check it?

—Wait... This is the diameter... —You’re right, it’s exactly 12 centimeters! »

Mister Cuadrado. Un recorrido por el sorprendente mundo de la geometría

A nna Cerasoli: «Naranjas y Pingüinos», en Maeva Ediciones.

1 Filo wants to compare the volume of the two cylinders by weighing sugar. Is this a valid method? Is it correct to measure volume with a unit of mass?

2 Knowing that it may not be accurate, if you were to fill one of the two cylinders with popcorn, how would you compare the volume of the two cylinders?

3 Find the volume of the can.

4 Why do you think paint cans and similar materials usually have the same height and diameter? These cylinders are called equilateral. Express the area and volume of an equilateral cylinder as a function of its radius.

We invite you to do the same experiment that Filo wants to do with his grandfather. We are going to do this in six steps:

1. Ask a question.

If we roll two equal pieces of construction paper lengthwise and widthwise, which cylinder will have more capacity?

2. Investigate the topic.

We take two identical pieces of construction paper and build two cylinders. We fill one with sand, salt or sugar. Then, we pour the filling from this cylinder into the other.

3. Write a hypothesis. We write down what we think will happen. In this case, we have to decide whether the two cylinders will have the same capacity or not.

4. Test the hypothesis by doing the experiment. Take two identical pieces of construction paper, like the ones in the picture, for example. Carry out step 2.

30 cm

20 cm

30 cm

20 cm

5. Analyse the data and draw a conclusion. For the calculations:

• Consider π = 3.14 and round to two decimal places.

• Call the tall cylinder C1 and calculate its radius and volume.

• Call the short cylinder C2 and calculate its radius and volume.

• Find the ratio of both volumes and write the conclusion. Are the capacities equal or different?

6. Share your results. Make a short report of your experiment. Include the steps you have taken and the calculations from step 5.

We suggest a similar activity. If your teacher agrees, you can work in groups of 4 pupils. Take one sheet of A4 paper (21 cm by 29.7 cm) and cut out a rectangle and two circles to form a cylinder. Different models can be made. For example:

1 Which dimensions allow you to make a cylinder with the greatest volume?

1 What is the numerical value of a polynomial? Give an example.

Solution

The numerical value of a polynomial is the value obtained by substituting a number for the variable and carrying out the operations.

Example: Find the numerical value of P(x) = x3 + 5x2 – 7x – 4, for x = 2

P(2) = 23 + 5 · 22 – 7 · 2 – 4 = 8 + 20 – 14 – 4 = 28 – 18 = 10

2 Multiply the following polynomials

P(x) = 3x3 – 7x – 6 Q(x) = 5x2 – 9x + 1 Find the degree of the product

Solution

15x5 – 27x4 – 32x3 + 33x2 + 47x – 6 The degree of the product is: 3 + 2 = 5

3 Solve the following equations:

a) 2(3x – 5) – 4(x – 2) = 13 – x

Solution

a) x = 5

b) 3x2 –3x 4 –9 8 = 0

b) x1 = 3 4 , x2 = –1 2

4 Solve the following system of equations using the best possible method.

2x + 3y = 12

5x – 7y = 1

Solution

You solve it by the reduction method; multiply the 1st equation by 5 and the 2nd by – 2 and then you add them.

x = 3, y = 2

5 A plan of a house shows a 2.4 cm corridor. The actual corridor measures 6 m. Find the scale.

Solution

Scale: 2.4 cm : 6 m = 2.4 cm : 600 cm = 1:250

6 The dimensions of a shoebox are 40 cm, 20 cm and 15 cm. Calculate the length of its diagonal. Round the result to two decimal places.

Solution

15 cm

20 cm

40 cm

D = √2 225 = 47.17 cm ⎧ ⎨ ⎩

Using the Pythagorean theorem in 3D space

D 2 = 402 + 202 + 152 =

= 1 600 + 400 + + 225 = 2 225

7 A rectangular field is 25 m longer than it is wide. If the perimeter is 250 m, what is the area? a

b Solution

b = a + 25

2a + 2b = 250

⎧ ⎨ ⎩

a = 50 m

b = 75 m

Área = 50 ∙ 75 = 3 750 m2

8 How many 5-litre jugs will be filled with the water from the tank shown in the figure?

Take π = 3.14 Solution

V = V Cylinder: 2 = = 3.14 ∙ 12 ∙ 3 : 2 = = 4.71 m3 = 4 719 L 4 710 : 5 = 942 jugs. 3

1. What is a linear system of two equations with two unknowns? Give an example.

2. Calculate: a) (2x + 1/2)2 b) (2x + 3) (2x – 3) c) (x – 5)2

3. Solve the following equations:

4. Solve the system using the best possible method: y

5. Calculate the apothem of a square pyramid with a 5 cm base edge and 7 cm height. Round the result to two decimal places.

6. Calculate the area and volume of a hexagonal prism in which the edge of the base is 2 m and the height is 6 m. Round the result to two decimal places.

7. Find two numbers knowing that one is three times the other and that twice the first plus five times the second is 85.

8. A lighthouse casts a 55 m long shadow. On the same day, at the same time and in the same place, a 1.5 m high vertical pole casts a 1.75 m shadow. Calculate the height of the lighthouse. Round the result to two decimal places.