José María Arias Cabezas
Ildefonso Maza Sáez
A guide to your book
With the book you are holding you will:
➜ Learn Maths using the 5-stage methodology: Engage, Explore, Explain, Elaborate and Evaluate, the same method used in the Spanish book.
➜ Consolidate and improve your English proficiency through activities where you will practise both written and oral comprehension and expression.
The following information is given:
1. The content of the module and the 5 stages:
➜ Engage: a stimulating GeoGebra applet related to the core concepts covered in the block.
➜ Explore: an interactive and dynamic activity based on the applet that lets you explore your current knowledge and relate it to the concepts you will be studying.
➜ Elaborate: a section to investigate and apply the concepts.
2. A learning situation with two phases:
➜ Explore: searching for information on a concept to be worked on.
➜ Elaborate: a short writing activity to present the information obtained.
Main content
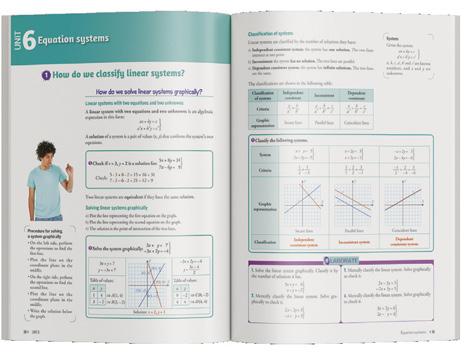
Each unit’s double page contains the Explain stage where the core concepts are presented. This is supported by a solved exercise or problem to illustrate the mathematical concept. The exercise is solved with straightforward operations so the students can focus on the new content being covered.
The final Elaborate section has exercises and problems to enhance and consolidate the acquired knowledge.
Learning experience
A comprehensive learning experience will enable you to develop all the key competences and core concepts you have acquired, as well as enhance your teamwork skills. Additionally, you will be able to communicate the results in a creative manner, using different formats.
Evaluation
The last step in the 5-stage methodology is Evaluate, which has two parts:
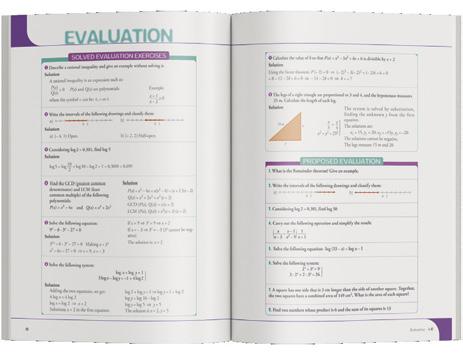
➜ A solved evaluation that serves as a review of the content covered.
➜ A proposed evaluation covering the module’s content.
II MODULE
7 Similarity
Open the applet using the QR.
1 Observe the solved exercise and work out the given exercise.
2 You can investigate further and draw conclusions.
Learning experience
At the end of this module, you will find a comprehensive learning activity that will allow you to work in teams. You will need to apply all the core concepts you have acquired. In addition, you will be able to communicate the results in a creative manner, using different formats.
e
e e e
1 Research the muqarnas and where they are used.
2 Find out how the muqarnas are used in the dome of the Hall of the Two Sisters in the Alhambra in Granada.
3 Write a few sentences with the information you have obtained.
How do we use Thales’s theorem? 1
What is Thales’s theorem?
Thales’s theorem
Thales’s theorem states that if two lines r and s intersect parallel lines a, b, c , etc., the segments on lines r and s are proportional. The ratio k is the similarity ratio.
A'B' AB = B'C' BC = k
1 Where AB = 4.2 cm, BC = 2.4 cm and B’C’ = 3.6 cm, find the length of segment A’B’ and similarity ratio k
2 Find the length of side B’C’ in the figure.
Two triangles are similar if they have two equal angles.
The similarity ratio is: k =
Triangles in Thales position
Two triangles are in Thales position if they have a common angle and the sides opposite the angle are parallel.
Two triangles in Thales position are similar. In other words:
a) The angles are equal.
b) The corresponding sides are proportional.
AB′ AB = AC ′ AC = B′C ′ BC
Similarity criteria for triangles
Two triangles are similar if they have an equal angle and the sides of the angle are proportional.
Two triangles are similar if they have three proportional sides.
3 Triangles ABC and A’B’C’ are similar, and a = 4.8 cm, b = 4 cm and a ′ = 6 cm
Find: a) the similarity ratio b) the length of side b′
Applying triangle similarity
4 A 1.5 m vertical pole casts a shadow of 2 m. How tall is a tree whose shadow is 9 m on the same day, at the same time and in the same place? Round your result to two decimal places.
2 9 = 1.5 x
Similar perimeters, areas and volumes
5 The volume of a tetrahedron is 12 m3. Find the area of another similar tetrahedron with similarity ratio k = 1.5
1. Find the length of segment A’B’ where AB = 7.5 cm, BC = 10 cm and B’C’ = 12 cm. What theorem did you use?
4. The two triangles in the following drawing are similar. Find the length of a ′ and c ′
2. In your notebook, draw a right-angled triangle with legs of 4 cm and 3 cm. Draw another rightangled triangle in Thales position where the longer leg is 8 cm. What is the length of the other leg?
3. Two angles of a triangle measure 45° and 60°. Two other angles of another triangle measure 75° and 60°. Are the two triangles similar?
5. Sue and her mother are in a photograph. Sue is 1.65 m tall. In the photo, Sue is 6.6 cm tall and her mother is 6.88 cm tall. How tall is Sue’s mother?
6. A tree of 1.6 m casts a shadow of 1.2 m. In the same place, on the same day and at the same time, the shadow of a building is 52 m. What is the height of the building?
7. The volume of a sphere is 7.5 cm3. Calculate the volume of another sphere with a radius twice as large.
How do we use the Pythagorean theorem? 2
Which theorems apply to right triangles?
Altitude theorem
Avoiding errors
The altitude theorem, the cath etus theorem and Pythagorean theorem only apply to triangles.
The riht-angled triangle altitude theorem states that the square of the length of the altitude relative to the hypotenuse is equal to the product of the lengths of the segments on the hypotenuse:
′ = 1.8 m c ′ = 3.2 m
Cathetus theorem
The right triangle cathetus theorem states that the square of the length of a cathetus (also called a leg) is equal to the product of the length of the hypotenuse times the length of the orthographic projection of the cathetus on the hypotenuse:
b′ = 1.8 m c ′ = 3.2 m
= 5 m
16
Pythagorean theorem
The Pythagorean theorem states that the square of the hypotenuse of a right-angled triangle is equal to the sum of the squares of its legs:
2 = b 2 + c 2
6 One leg of a triangle is 12 m and the other is 16 m. Calculate the hypotenuse.
Applications of the Pythagorean theorem
You can use the Pythagorean theorem to find the length of the third side of a triangle if you know the length of the other two sides.
7 Find the lateral area of a cone with a base radius of 4 m and a height of 7 m. Round to two decimal places. G 2 = R 2 + H 2 ⇒ G 2 = 42 + 72 = 16 + 49 = 65 ⇒ G = √ 65 = 8.06 m
Pythagorean triples
A Pythagorean triple is three integers that verify the Pythagorean theorem. If a triple is Pythagorean, all its multiples are also Pythagorean.
3, 4 and 5 ⇒ 32 + 42 = 52
The Pythagorean theorem in three-dimensional space
The Pythagorean theorem in three-dimensional space states that the square of the diagonal of an orthohedron is equal to the sum of the squares of its edges:
Classifying triangles by their sides
A triangle has three sides: a, b and c. The longest side is side a. What type of triangle is it?
• Right-angled if:
• Acute if:
• Obtuse
8. The hypotenuse of a triangle is 7.5 cm. One of the segments of the hypotenuse divided by the corresponding altitude is 6 cm. Draw the triangle and find its altitude.
9. The hypotenuse of a right triangle is 10 m. The projection of leg b on the hypotenuse is 3.6 m. Find:
a) The length of leg b
b) The length of projection of leg c on the hypotenuse.
c) The length of leg c
d) The altitude relative to hypotenuse h Then:
e) Draw the right triangle.
10. The legs of a right triangle measure 4 cm and 3 cm. Draw the triangle. Find the length of the hypotenuse and the area.
11. The hypotenuse of a right triangle is 4 cm and one leg is 3.5 cm. Draw the triangle. Find the length of the other leg. Round your result to two decimal places.
12. Draw a graphic interpretation of the Pythagorean
theorem with sides of 6 cm, 8 cm and 10 cm.
13. Which of these are Pythagorean triples?
a) 2, 3 and 4
b) 3, 4 and 5
c) 5, 12 and 13
d) 4, 5 and 6
14. The radius of the base of the cone below is 3 cm and the generatrix is 5 cm. Calculate the volume of the cone. Round your result to two decimal places.
15. Calculate the diagonal of an orthohedron with dimensions of 8 m, 4 m and 3 m
How do we use blueprints,
maps and models?
How do we use scales?
Scales
The scale of a drawing, map or model is the quotient of a length in the drawing, map or model and the corresponding real length of the object. In other words, it is a ratio of size. It is written as a quotient with a dividend of one, for example, 1:200. When you read it, you say ‘a scale of one to two hundred’.
If the scale of a blueprint is 1:200, 1 cm in the blueprint is equal to 200 cm in reality.
8 Find the scale of a blueprint in which 5 cm is equivalent to 12.5 m
5 cm : 1250 cm = 1:250 5 ab/c 1250 = 1 250
This means that 1 cm in the blueprint is equal to 250 cm = 2.5 m in reality.
Avoiding errors
Blueprints of buildings have larger scales than maps of regions. Note that scales are quotients. Normally, they are 1 divided by another number. When the denominator is greater, the quotient is smaller.
• The scale of a blueprint for an apartment is generally 1:200 = 0.005
• The scale of a map is generally 1:5000000 = 0.0000002
Blueprints
Blueprints (or architectural plans) are used to represent a house, an apartment, a piece of land, etc. Their scale is generally greater than 1:10 000
9 The blueprint for an apartment has a scale of 1:200. The length of a corridor in the blueprint is 6 cm. What is the real distance?
Maps
A map is a representation of all or part of the Earth. The scale is usually less than 1:10 000
10 The map in the margin has a scale of 1:25 000 000. Find the distance in a straight line from Sevilla to León.
If you measure with a ruler, the distance on the map is 2.4 cm
2.4 . 25000000 = 60000000 cm = 600 km
The distance from Sevilla to León in a straight line is 600 km
Models
A model is a three-dimensional representation of an object.
11 The model of the yacht below is built to a scale of 1:300. What is its real length?
Scale 1:300
If you measure the length of the model yacht with a ruler, it is 7.5 cm
7.5 . 300 = 2250 cm = 22.5 m
In reality, the yacht is 22.5 m
16. The blueprint of a house shows a 4 cm long corridor. Its real length is 10 m. Find the scale of the blueprint.
17. You have two scales, 1:200 and 1:20 000
a) Which is larger?
b) Which one would you use for a blueprint?
c) Which one would you use for a map?
18. A triangular piece of land has a side measuring 500 m. Draw a similar triangle with a corresponding side measuring 2.5 cm
a) Find the scale.
b) What would you use to represent the piece of land, a blueprint or a map?
19. The blueprint of a flat has a scale of 1:200. In the blueprint, the living room measures 3 cm × 2.5 cm. Calculate its real dimensions and area.
20. Use a ruler to measure the map in Solved Exercise 10. Calculate the distance in a straight line between:
a) A Coruña and Barcelona.
b) Bilbao and Cádiz.
c) Huelva and Oviedo.
d) Valencia and Madrid.
21. Find the height of the yacht in Solved Exercise 11
What are the characteristics of plane figures?
How do we find the perimeter and area of figures?
Perimeter and area of polygons
Length and area of circular figures Name Drawing
22. Calculate the area of a triangle with sides measuring 5, 9 and 10 m
23. Mentally calculate the area of a rhombus with diagonals of 6 and 16 cm
24. Mentally calculate the area of a rhomboid with a base of 15 m and a height of 4 m
25. Calculate the area of a trapezium with bases measuring 8.6 cm and 5.4 cm and a height of 3.8 cm
26. Calculate the area of a hexagon with sides measuring 8 m
27. Calculate the circumference of a circle with a radius of 7 cm
28. Calculate the area of a circle with a radius of 7.4 m
29. Calculate the length of an arc with a radius of 5.6 cm and an angle of 150°
30. Calculate the area of a circle sector with a radius of 15.3 m and an angle of 68.5°
31. Calculate the area of an annulus with R = 8.6 m and r = 5.4 m
and volumes What are the characteristics of geometric bodies?
Area and volume of a prism
• You can see the total area of a prism in its two-dimensional net. It is made of two equal bases, which are regular polygons, and a number of equal rectangles equal to the number of sides of the base.
• To find the volume of a prism, multiply the area of the base by the height.
1 Find the area of a quadrangular prism with a base edge of 3 cm and a height of 8 cm
• Total area: AT = 2AB + AL; AB = l 2 ⇒ AB = 32 = 9 m2 AL = 4lH ⇒ AL = 4 · 3 · 8 = 96 m2
• AT = 2 · 9 + 96 = 18 + 96 = 114 m2
• Volume: V = AB · H ⇒ V = 9 · 8 = 72 m3
Area and volume of a cylinder
• You can see the total area of a cylinder in its two-dimensional net. It is made of two equal circular bases and a rectangle.
• To find the volume of a cylinder , multiply the area of the base by the height.
2 Find the area and volume of a straight cylinder with a base radius of 4 cm and a height of 9 m. Use π = 3.14
• Total area: AT = 2AB + AL; AB
• AT = 2 · 50.24 + 226.08 = 326.56 m2
• Volume: V = AB · H ⇒ V = 50.24 · 9 = 452.16 m3
1. Mentally calculate the area and volume of a cube with an edge measuring 4 m
2. Calculate the area and volume of an orthohedron with sides measuring 7.5 cm, 6.4 cm and 3.2 cm
3. Calculate the area and volume of a quadrangular prism with a base edge of 7 cm and a height of 12 cm
4. Calculate the area and volume of a right cylinder with a base radius of 4.5 m and a height equal to double the base radius. Use π = 3.14
5. Calculate the area and volume of a hexagonal prism with a base edge of 10 m and a height of 15 m
Volume of prisms and cylinders
Since they all have two equal bases, you can calculate volume with the same formula:
6. A water container is an orthohedron with the following dimensions expressed in meters: 1.75 × 2.34 × 1.17. The tank is currently at 45% of its capacity. It took 180 days of water consumption for the tank to reach its current capacity. How much is consumed daily?
What
are the characteristics of pyramids and cones?
How do we find the area and volume of pyramids and cones?
Area and volume of a pyramid
• You can see the total area of a pyramid in its two-dimensional net. It has a regular polygon base and a number of isosceles triangles equal to the number of sides of the base: AT = AB + AL
• To find the volume of a pyramid, multiply the area of the base by the height and by one-third: V = 1 3 AB · H
3 Find the area and volume of a quadrangular pyramid with a base side of 12 m and a height of 20 m
• Total area:
Calculate the area of the base and the sides:
Calculate the apothem of the pyramid, h. Apply the Pythagorean theorem:
Area and volume of a cone
• You can see the total area of a cone in its two-dimensional net. It is made of a circular base and a circular sector:
B = π R 2 AL = π RGAT = AB + AL
• To find the volume of a cone, multiply the area of the base by the height and by one-third:
4 Find the area and volume of a right cone with a base radius of 5 m and a height of 12 m. Use π = 3.14
Volume of pyramids and cones
Since they all have a base and a vertex, you can calculate the volume with the same formula:
• Total area: AT = AB + AL
Calculate the area of the base and the sides:
B = π R 2 ⇒ AB = 3.14 · 52 = 78.5 m2 AL = π RG
Calculate generatrix
m2 • Volume: V = 1 3 AB · H ⇒ V = 1 3 · 78.5 · 12 = 314 m3 1 ÷ 3 × 78,5 × 12 = 314
7. Calculate the area and volume of a quadrangular pyramid with a base edge of 6 m and a height of 10 m
8. Calculate the area and volume of a right cone with a base radius of 2.5 m and a height equal to four times the base radius. Use π = 3.14 h r
9. Calculate the area and volume of a hexagonal pyramid with a base edge of 6 m and a height of 13 m
10. A fabric tent has the shape of a right cone with a base radius of 4 m and a height of 3 m. A square metre of the fabric costs €25. How much will the fabric to make the tent cost? Use π = 3.14
Volume of truncated pyramids and truncated cones
Since they have two unequal bases, you can calculate volume with the same formula.
What are the characteristics of truncated bodies and spheres?
How do we find the area and volume of truncated pyramids and cones?
Area and volume of truncated pyramids
• You can see the total area of a truncated pyramid in its two-dimensional net. It is made of two regular polygonal bases and a number of isosceles trapezoids equal to the number of sides of the base:
• To find the volume of a truncated pyramid, multiply one-third by the sum of the areas of the bases plus the square root of the product of their areas, and multiply everything by its height:
5 Find the area and volume of a rectangular truncated pyramid with a longer base edge of 30 cm, a shorter base side of 18 cm and a height of 8 cm
Total
Area of the bases and sides:
Calculate the apothem of truncated pyramid, h:
Area and volume of a truncated cone
• You can see the total area of a truncated cone in its two-dimensional net. It is made of two unequal circular bases and a circular trapezoid:
T = AB1 + AB2 + AL; AB1 = πR 2 ; AB2 = πr 2 ; AL = π(R + r)G
• To find the volume of a truncated cone, multiply one-third by the sum of the areas of the bases plus the square root of the product of their areas, then multiply by its height:
= 1 3 (AB1 + AB2 + √ AB1 · AB2 ) · H
6 Find the area and volume of a truncated cone with a larger base radius of 9 m, a smaller base radius of 4 m and a height of 12 m. Use π = 3.14
• Total area: AT = AB1 + AB2 + AL
Area of the bases and sides:
Calculate the generatrix: G = √ 52 + 122 = √ 169 = 13 m AL = π (R + r) G; AL = 3,14 · (9 + 4) · 13 = 530.66 m2 Therefore: AT = 254.34 + 50.24 + 530.66 = 835.24 m2
• Volume:
Area and volume of a sphere
Spheres do not have two-dimensional nets. The area of a sphere is equal to four times its cross-section (a circle of radius R) A = 4πR 2
To find the volume of a sphere, multiply four-thirds by π by the radius cubed: V = 4 3 πR 3
The area and volume of the sphere is:
11. Calculate the area and volume of a hexagonal truncated pyramid with:
• A larger base edge of 16 m
• A smaller base edge of 4 m
• A height of 8 m
12. Calculate the area and volume of a truncated cone with a larger base radius of 9 m, a smaller base radius of 5 m and a height of 10 m. Use π = 3.14
13. Calculate the area and volume of a sphere with a radius of 8.5 cm. Use π = 3.14
Cordovan Proportion
In the same way that the Golden Ratio, Phi (ϕ), the Fibonacci sequence, and the pentagon are related, the octagon has certain specific geometric relations. The regular octagon is not useful for covering a plane surface nor for generating regular polyhedra, but it has certain properties that make it special.
When a circle is divided into 8 equal parts using 4 lines, different polygons can be generated depending on how these lines are connected:
1 Connect all the vertices one by one. Which polygon is generated?
2 Connect the vertices alternating every two lines. Repeat the process from another free vertex. Which polygons are generated? Can the second polygon be generated by rotating the first one? How many degrees should it be rotated? The polygon formed is called a stellated octagon of order two.
3 Join the vertices alternating every three lines. Repeat the process from another free vertex until there are none left. This polygon is known as a stellated octagon of order three.
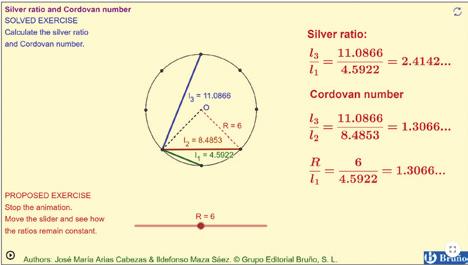
4 On the circle below, draw the segments l1, l2 and l3 and measure their lengths. Calculate the following quotients:
What can we observe from the second and third quotients?
The first quotient from the previous exercise is called the Silver Ratio. The result of the second and third quotients is called the Cordovan number. This name is a tribute to Rafael de la Hoz Arderius (1924-2000), who studied this ratio’s application to architecture and called it the Cordovan proportion.
1 Open the Applet Side Ratio. Modify the radius of the circumference and check if the same values are obtained.
2 With the segments l3 and l2, create an isosceles triangle with l2 as the unequal side. Find the angles in the triangle.
3 This triangle is called a Cordovan triangle. Draw another Cordovan triangle with the radius and l1
Many buildings use the octagon. The images below show examples of their use in the Alhambra de Granada:
1 Draw the octagonal shape that can be seen in the two vaults in these photographs above.
2 Draw a mosaic with octagons and squares.
3 Look for information on unique octagonal constructions and make a presentation with the ones you find most interesting.
Vault of the Hall of the Abencerrajes
Vault of the Hall of the Two Sisters
1 Write the statement of the Pythagorean theorem and give an example of a Pythagorean triple.
Solution
The Pythagorean theorem states that: in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the legs. a2 = b2 + c 2
Example of a Pythagorean triple: 3, 4 and 5 ⇒ 32 + 42 = 52
2 Calculate:
Draw a right triangle with legs measuring 4.5 cm and 6 cm.
Draw another smaller right triangle in the Thales position so that its shorter leg measures 3 cm.
Calculate the length of the other leg.
Solution
3 Find b, c, c′ and h in the right triangle shown below:
3,6
4 In a map, 5 cm corresponds to 1 200 km on the ground. Find what scale was used for the map.
Solution Scale: 5:120 000 000 = 1:24 000 000
5 Calculate the area and volume of a cube with a 7 m edge.
Solution A = 6 · 72 = 294 m 2 V = 73 = 343 m3
6 Calculate the surface area of a truncated square pyramid in which the edge of the larger base measures 10 m, the edge of the smaller base measures 7 m, and the height is 14 m. Round the result to two decimal places.
Solution
A B1 = 102 = 100 cm 2 ; A B 2 = 72 = 49 cm 2
Find the apothem of the side of the truncated pyramid: h = √ 142 + 1.52 = 14.08 m
AL = 4 (10 + 7) : 2 · 14.08 = 478.72 m2 AT = 100 + 49 + 478.72 = 627.72 m2
7 On a floor plan, a room measures 4 x 3 cm. If the scale of the plan is 1:125, find the area of the room. Round the result to two decimal places.
Solution
Dimensions: 4 · 125 = 500 cm = 5 m; 3 · 125 = 375 cm = 3.75 m Area = 5 · 3.75 = 18.75 m2
8 Calculate the height of a cylindrical hot water tank with a capacity of 50 L and a diameter of 30 cm. Consider π = 3.14. Round the result to two decimal places.
Solution
3.14 · 152 · H = 50 000 H = 70.77 cm
1. Write the formulas for the area and volume of an orthohedron. Draw a diagram, make up some measurements and calculate its area and volume.
2. A building casts a 20 m shadow. On the same day, at the same time, and in the same place, a 2 m pole casts a shadow of 1.75 m. Calculate the building height. Round the result to two decimal places.
3. Draw a right triangle in which the legs are in proportional to 3 and 4. How many triangles are there with this condition?
4. On a map, 5 cm corresponds to 1 000 km on the ground. Find the scale of the map.
5. Calculate the area of a hexagonal prism in which the edge of the base measures 8 m and the height 12 m. Round the result to two decimal places.
6. Calculate the volume of a square pyramid in which the edge of the base measures 9 m and the height is, 15 m
7. Calculate the area of an isosceles trapezoid in which the bases measure 10 cm and 4 cm, and the other two sides measure 5 cm each.
8. A quarter of a spherical construction is to be covered with solar panels costing €540 per square metre. If the radius of the sphere is 8 m, how much will it cost to install the panels? Consider π = 3.14. Round the result to one decimal place.
© GRUPO EDITORIAL BRUÑO, S. L., 2024 - C/ Valentín Beato, 21 - 28037 Madrid.
All rights reserved. No part of this publication may be reproduced, stored in a retrieval system, or transmitted, in any form or by any means, electronic, mechanical, photocopying, recording, or otherwise, without the prior permission of the publishers.