Muestra del libro Mathematics 4º B ESO Proyecto 5 etapas

José María Arias Cabezas

Ildefonso Maza Sáez

A guide to your book

With the book you are holding you will:

➜ Learn Maths using the 5-stage methodology: Engage, Explore, Explain, Elaborate and Evaluate, the same method used in the Spanish book.

➜ Consolidate and improve your English proficiency through activities where you will practise both written and oral comprehension and expression.

The following information is given:

1. The content of the module and the 5 stages:

➜ Engage: a stimulating GeoGebra applet related to the core concepts covered in the block.

➜ Explore: an interactive and dynamic activity based on the applet that lets you explore your current knowledge and relate it to the concepts you will be studying.

➜ Elaborate: a section to investigate and apply the concepts.

2. A learning situation with two phases:

➜ Explore: searching for information on a concept to be worked on.

➜ Elaborate: a short writing activity to present the information obtained.

Main content

Each unit’s double page contains the Explain stage where the core concepts are presented. This is supported by a solved exercise or problem to illustrate the mathematical concept. The exercise is solved with straightforward operations so the students can focus on the new content being covered.

The final Elaborate section has exercises and problems to enhance and consolidate the acquired knowledge.

Learning experience

A comprehensive learning experience will enable you to develop all the key competences and core concepts you have acquired, as well as enhance your teamwork skills. Additionally, you will be able to communicate the results in a creative manner, using different formats.

Evaluation

The last step in the 5-stage methodology is Evaluate, which has two parts:

➜ A solved evaluation that serves as a review of the content covered.

➜ A proposed evaluation covering the module’s content.

I MODULE

1 Observe the solved exercise and work out the proposed exercise. 2 You can investigate further and draw conclusions.

Learning experience

At the end of this module, you will find a comprehensive learning activity that will allow you to work in teams. You will need to apply all the core concepts you have acquired. In addition, you will be able to communicate the results in a creative manner, using different formats.

e

e e e

1 Find information on the Euribor, how it is calculated and how it relates to mortgages.

2 Write a few sentences with the information you have obtained.

UNIT 1

Real numbers

What are rational and irrational numbers? 1

ℚ

What are the characteristics of rational and irrational numbers?

Rational numbers

The set of the rational numbers includes the set of integers ℤ = {0, ±1, ±2, ±3...} and fractions.

The set of rational numbers is represented by the letter ℚ

Calculator

a) To write improper fractions directly:

MODE (Disp) 1 (d/c) 2

b) To use the decimal point in decimal notation:

(Disp) 1 ▶ (Comma) 2

Rational numbers can be expressed as decimals. They can be integers, or exact decimals if their decimal expression is finite. They can also be repeating decimals, if their decimal expression is infinite.

Density of rational numbers

The set of rational numbers is dense. In other words, there is always a rational number between any two rational numbers.

Between rational numbers 2.4 and 2.5 there is another rational number, for example, its arithmetic mean: 2.4 + 2.5 2 = 2.45

Between rational numbers 2.4 and 2.45 there is another rational number, for example, its arithmetic mean: 2.425

Between rational numbers 2.4 and 2.425 there is another rational number, for example, its arithmetic mean: 2.4125

Irrational numbers

Irrational numbers cannot be expressed as a quotient of two integers or as exact or periodic.

a) The length of the diagonal of a square with a 1 cm side: √ 2 = 1.414213562... √–2 = 1.414213562

b) The quotient of the length of a circle and its diameter: π = 3.141592653... π = 3.141592654

c) The quotient of the diagonal and the side of a regular pentagon (or golden number):

d) Euler’s number e = 2.718281828... ex 1 = 2.718281828

Graphic representation

The Pythagorean theorem is used to represent irrational numbers that are square roots. When you cannot find a geometric method to represent the numbers, you can use an approximation of the decimal expression.

Representation of sections a), b) and c).

1. Are the numbers below rational or irrational? a) 2 3 b) π c) √ 2 d) 1.455555...

2. Write three rational numbers.

3. Write three irrational numbers.

4. Write three rational numbers between 1/4 and 3/4

5. Graph these numbers exactly:

a) √ 8 b) √ 10

6. Graph using approximation:

a) √ 15

b) – π

c) √ 23

d) –√ 14

7. Find the exact side of a square with an area of 10 cm2. What type of number is this?

Calculate: 8. 4 5 + 3 – 7 15

Mathematical symbols ∈ Is an element of

Is not an element of

Implies ⇔ Is equivalent to

Union ∩ Intersection ∅ Null set

What are real numbers? 2

What are the characteristics of real numbers?

Real numbers

The set of real numbers consists of all rational and irrational numbers. It is represented with the letter ℝ Real numbers ℝ

Integers ℤ

Rational numbers

Natural numbers (ℕ): 0, 1, 2, 3… Negative numbers: …, – 3, – 2, – 1

Fractions: – 3 2 , –1 4 , 2 3 , 7 6 …

Irrational numbers: π, e, ϕ, √ 2 , 3 √ 5

The number line

The real number line is a line showing all the real numbers. Each point on the line is a real number and every real number corresponds to a point on the line.

Absolute value

The absolute value of a number represents its distance from zero on a number line, always resulting in a positive value or zero.

The absolute value of a positive number is the same number. The absolute value of a negative number is the same number without the negative sign. | a | =

Distance

The distance between any two real numbers is the absolute value of their difference.

Absolute value and distance The absolute value of a number is its distance to zero.

Intervals

a and b are real numbers, and a < b

Open interval (a, b) = {x ∈ ℝ; a < x < b}

Closed interval [a, b] = {x ∈ ℝ; a ≤ x ≤ b}

Half-open or half-closed interval

[a, b) = {x ∈ ℝ; a ≤ x < b}

(a, b] = {x ∈ ℝ; a < x ≤ b}

5) = {x ∈ ℝ; 1 < x < 5}

(a, + ∞) = {x ∈ ℝ; x > a}

[a, + ∞) = {x ∈ ℝ; x ≥ a} (– ∞, 4) = {x ∈ ℝ; x < 4} (– ∞, – 1] = {x ∈ ℝ; x

– 1} (2, + ∞) = {x ∈ ℝ; x > 2} [– 1, + ∞) = {x ∈ ℝ; x ≥ – 1}

Neighbourhoods

A neighbourhood with centre a and radius r is the set of real numbers with a distance to centre a that is less than radius r. E (a, r ) = {x ∈ ℝ; d (x, a) < r } = {x ∈ ℝ; | x – a | < r } = (

1 4

1 – 1

The reduced neighbourhood of centre a and radius r is a neighbourhood without the centre. It is expressed as: E *(a, r ) E *(a, r) = E(a, r ) – {a } = (a – r, a + r ) – {a } = (a – r, a)

13. Plot the pairs of numbers on the number line. Calculate the distance between them.

a) 2 and 5 b) – 3 and 2 c) – 4 and – 1 d) – 3 and 0

14. Write the intervals as an inequality. Write them and classify them.

a) (– 2, 4] b) [– 5, 1] c) [3, +∞) d) (– ∞, – 3)

15. Graph the neighbourhoods:

a) E(1, 4)

b) E *(– 1, 3)

16. Write the intervals in the figures and classify them. a)

b)

c)

d)

17. Graph the neighbourhoods: a)

b)

How do we solve problems? 3

Rule of three procedure

a) Identify the quantities involved and their units.

b) Organise the quantities and values, with the unknown quantity in the last place.

c) Determine if the proportion is direct or inverse.

• The proportion is direct if one quantity increases or decreases, the second variable also increases ( + and + ) or decreases (- and -).

• The proportion is inverse if one quantity increases, the other quantity must decrease (+ and -). Alternatively, if one quantity decreases, the other must increase (- and +)

Multiply across the vertical line (

Divide across the horizontal line (÷):

What is the procedure for solving problems?

Proportionality problems

• If the quantities are directly proportional:

Quantity A (units) (D)

Quantity B (units)

• If the quantities are inversely proportional:

Quantity A (units) (I)

Quantity B (units )

1 The price for three and a half kilograms of strawberries was €5. How much will four and a half kilos cost?

(kg) (D) Cost (€)

Percentage problems

• Percent decrease means subtracting a given percentage of a value from the original value.

• Index of percentage decrease: one minus the percentage expressed as a decimal.

• Percent increase means adding a given percentage of a value to the original value.

• Index of percentage increase: one plus the percent expressed as a decimal.

2 A printer with an original price of €247 is reduced by 20%. Find out the printer’s new price.

Index of percentage decrease: P = 1 – 0.2 = 0.8

3 Sonia’s salary has increased by 3%. If she now earns €1 545, how much was she earning before the increase?

Index of percentage increase: P = 1 + 0.03 = 1.03

Magic triangle method

Inverse relation

Successive percentage increases and decreases

To calculate successive percentage increases and decreases, multiply the percentage rate at each step.

4 A television’s original price was €300. It now has a 20% discount, and 21% VAT is added. What is the final price of the television?

Simple and compound interest problems

Simple interest is the money produced by capital deposited in a financial institution that does not accrue to the capital to earn further interest. Simple interest depends on the initial capital, the interest rate, and time.

Compound interest is interest that accrues on the capital to earn further interest. The final capital depends on the initial capital, interest rate, and time.

5 A deposit of €3 000 is made at a simple interest rate of 2% for 3 years. What will be the total interest earned at the end of this period?

6 What is the capital accumulated if a sum of €3 000 is deposited at 2% compound interest for three years, with interest paid annually?

Round all results to two decimal places.

18. A car consumes 36 litres of petrol in 450 km. How much will it consume on a 120 km trip?

19. A crew of 4 workers completes a job in 40 hours. How many hours will the job take with one more worker, working at the same pace?

20. Of the 40 technicians who have taken a training course, 34 have improved their performance. What is the percentage of technicians who have improved?

21. A car cost €15 000 last year and has now been sold for 40% less. How much money did the seller receive, considering 21% VAT had to be paid?

22. John paid €68 for a pair of trousers that had a 15% discount. What was the original price of the trousers?

Terms

C = Final capital

C = Initial capital

I = Interest

T = Time (expressed in years)

R = Rate or percentage

r = R/100

Investment periods

For simple interest, the formula for finding the total interest earned depends on the length of time of the deposit. See the formulas below.

• Quarters: I = crt 4

• Months: I = crt 12

• Days: I = crt 360

For compound interest, if interest is paid n times during one year, the final capital can be found using this formula:

C = c (1 + r n )nt

23. A locksmith charges €15 per hour for a standard service. If the work is carried out urgently, the price increases by 20%. How much will be charged for an urgent job that has taken 3 hours to complete?

24. A price of €15.75/m has been paid for cable trunking. If the same product was previously bought for €15/m, what percentage has been increased?

25. Find the final capital that will be obtained if a sum of €9 000 is deposited at 1.5% simple interest for the following periods:

a) 3 years

b) 18 months

26. A sum of €1 000 is deposited at 3% compound interest. Find the final capital to be received after 5 years if the interest is paid:

a) Annually

b) Quarterly

UNIT 5 Equation systems

How do we classify linear systems? 1

How do we solve linear systems graphically?

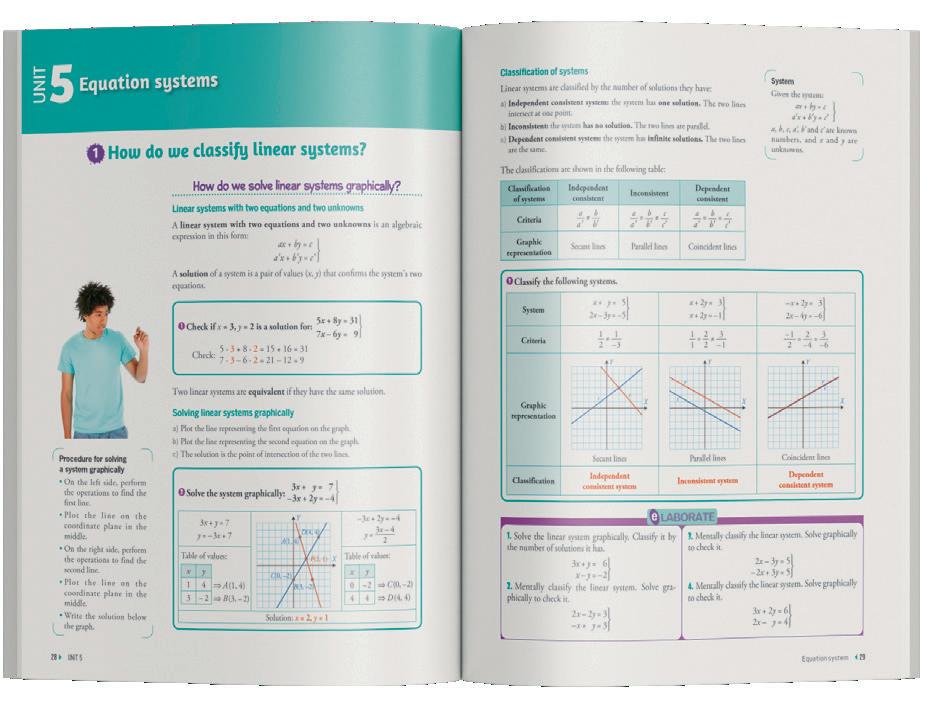
Linear systems with two equations and two unknowns

A linear system with two equations and two unknowns is an algebraic expression in this form: ax + by = c

Procedure for solving a system graphically

• On the left side, perform the operations to find the first line.

• Plot the line on the coordinate plane in the middle.

• On the right side, perform the operations to find the second line.

• Plot the line on the coordinate plane in the middle.

• Write the solution below the graph.

A solution of a system is a pair of values (x, y) that confirms the system’s two equations.

1 Check if x = 3, y = 2 is a solution for: 5x + 8y = 31 7x – 6y = 9

Two linear systems are equivalent if they have the same solution.

Solving linear systems graphically

a) Plot the line representing the first equation on the graph.

b) Plot the line representing the second equation on the graph.

c) The solution is the point of intersection of the two lines.

2 Solve the system graphically:

Solution: x = 2, y = 1

Classification of systems

Linear systems are classified by the number of solutions they have:

a) Independent consistent system: the system has one solution . The two lines intersect at one point.

b) Inconsistent: the system has no solution. The two lines are parallel.

c) Dependent consistent system: the system has infinite solutions. The two lines are the same.

The classifications are shown in the following table:

Graphic representation

Secant lines Parallel lines

3 Classify the following systems.

System

representation

Secant lines

Coincident lines

System

Given the system:

by

, b, c,

, b’ and c’ are known numbers, and x and y are unknowns.

Parallel lines

Coincident lines

Classification Independent consistent system Inconsistent system Dependent consistent system

1. Solve the linear system graphically. Classify it by the number of solutions it has. 3x + y =

2. Mentally classify the linear system. Solve graphically to check it.

2 x – 2y = 3 – x + y = 3 ⎫

3. Mentally classify the linear system. Solve graphically to check it.

4. Mentally classify the linear system. Solve graphically to check it. 3x + 2y = 6 2x – y = 4 ⎫

How do we solve linear systems algebraically?

How do we use the substitution, equalisation and reduction methods?

Substitution, equalisation and reduction methods

Substitution method

Isolating an unknown in one equation and putting it in the other equation.

Equalisation method Reduction method

Isolating the same unknown in the two equations and equalising the resulting expressions.

Which method should I use?

Preparing the equations so one unknown has opposite coefficients in the two equations.

You can solve any system with any of the three methods. For some systems, one of the methods will be easier than the others. To choose a method:

a) When only one unknown is isolated in a system, use the substitution method.

b) When the same unknown is isolated in all the equations in a system, use the equalisation method.

c) When no unknown is isolated in a system, use the reduction method.

4 Choose a method to solve the following system. Explain why you chose that method. Then solve the system:

Since only one unknown is isolated, use the substitution method:

a) Put the value of y from the first equation into the second equation.

b) Solve the resulting equation.

c) Put the value you obtain back into the equation with the isolated unknown.

Solution: x = 5, y = 2

Preliminary

operations

Sometimes systems have expressions that make it difficult to know which method to use. When this happens, you can perform operations to make solving them easier.

5 Solve the system:

a) To eliminate the denominators, multiply by the LCM of the denominators in each equation.

b) Eliminate brackets.

c) Reduce like terms.

d) Use the reduction method. Change the sign of the first equation. Add the two equations.

e) Put y = –1 in the second equation.

x =

6 Solve the system:

a) Eliminate the denominators by multiplying the first equation by the LCM of the denominators.

b) Write the system. Use the reduction method.

1. Add the equations.

2. Subtract the equations.

Solution: x = 4, y = 6

5. Solve the system with the best method. Explain why you chose that method:

7. Solve the system:

6. Solve the system with the best method. Explain why you chose that method:

8. Solve the system:

How do we solve systems of nonlinear equations?

What is a system of nonlinear equations?

Nonlinear systems

A system of nonlinear equations is a system with at least one equation that is nonlinear. These systems are usually solved by substitution. You isolate the easiest unknown in the simplest equation. You can also solve them with equalisation if the same unknown appears.

7 Solve the system. Plot the solution:

y = x 2 – 6x + 7 y = x – 3 ⎫ ⎬ ⎭

If you solve with equalisation, you have: x 2 – 6x + 7 = x – 3

Memorise the axis of symmetry formula

It is easy to remember: x = –b 2a

It is the formula for seconddegree equations

x = –b ± √ b 2 – 4 ac 2a

Eliminating ± √ b 2 – 4 ac

2 – 7x + 10 = 0

When you substitute the value of x with y = x – 3, you have:

• For x = 5 ⇒ y = 5 – 3 = 2

• For x = 2 ⇒ y = 2 – 3 = – 1

Solutions: x1 = 5, y1 = 2; x2 = 2, y2 = – 1

The graphic interpretation shows that parabola y = x 2 – 6x + 7 and line y = x – 3 intersect at points A (5, 2) and B (2, –1)

a) Graph parabola y = x 2 – 6x + 7

• Axis of symmetry: x = –b 2a ⇒ x =

• Vertex: x = 3 ⇒ y = 3 2 – 6

V (3, – 2)

Graphing a parabola from its vertex using odd numbers: 1, 3, 5, 7…

Mark the vertex: point V (3, –2)

From the vertex, count one to the right and one up, then another to the right and three up, then another to the right and five up, etc.

Draw the left branch using symmetry.

If the coefficient of x 2 is not 1, multiply the odd numbers by the coefficient.

2

Count odd numbers to draw the right branch of the parabola. Draw the left branch using symmetry.

b) Draw line y = x – 3

For x = 0, y = – 3, the line intersects point C (0, – 3) and has slope m = 1 Y X y = x 2 – 6x + 7 y = x – 3 B(2, – 1) A(5, 2) V(3, – 2)

The graph shows that the intersects are: A (5, 2) and B (2, –1)

Smart tips

When you have two second ­ degree equations and the coefficients of x 2 and y 2 are equal, subtract the two equations. The system will then have one second­degree and one first­degree equation.

Use this to find the intersects of two circles, since the standard equation for a circle is:

x 2 + y 2 + mx + ny + p = 0

8 Solve the system of two circles. Interpret the results:

x 2 + y 2 – 4x – 6y + 11 = 0

x 2 + y 2 – 6x – 8y + 21 = 0 ⎫ ⎬ ⎭

Subtract the second equation from the first equation.

2x + 2y – 10 = 0

Simplify by dividing by 2

x + y – 5 = 0 Solve for y

y = 5 – x

Put the value of y in the first equation:

x 2 + (5 – x)2 – 4x – 6(5 – x) + 11 = 0

x 2 + 25 – 10x + x 2 – 4x – 30 + 6x + 11 = 0

2x 2 – 8x + 6 = 0

x 2 – 4x + 3 = 0

Simplify by dividing by 2

x = 4 ± √ 16 – 12 2 = 4 ± 2 2 = 3 1

When you put the values of x in y = 5 – x, you have:

• For x = 3 ⇒ y = 5 – 3 = 2

• For x = 1 ⇒ y = 5 – 1 = 4

Solution: x1 = 3, y1 = 2; x2 = 1, y2 = 4

The graphic interpretation shows that the two circles intersect at points A (3, 2) and B (1, 4)

9. Solve the system. Graph the solution:

y = x 2 + 4x – 1 y = 2x + 2 ⎫ ⎬ ⎭

10. Solve the system of two circles. Interpret the result:

x 2 + y 2 = 18

x 2 + y 2 – 4x – 4y + 6 = 0 ⎫ ⎬ ⎭

11. Solve the system of a hyperbola and a line. Graph the solution: x y = 6 3x – 2y = 0 ⎫ ⎬ ⎭

12. Solve the system: x y = 3

x 2 + y 2 – 4x – 4y + 6 = 0

What are systems of exponential and logarithmic equations?

How

do

we solve a system of exponential equations?

Systems of exponential equations

To solve a system of exponential equations, you need to make two variable changes. This will convert the system into a linear system. Then you reverse the change of variable and find the value of the initial variables.

9 Solve the exponential system: 3x + 7y = 10

When you change the variables,

Logarithms

log p = x ⇔ 10 x = p

log 10 = 1

log 1 = 0

Properties:

log ( p ∙ q) = log p + log q

log q p = log p – log q

log pn = n ∙ log p

log √ p n = log n p

Solve by reduction. Multiply the first equation by 5 then add :

5u + 5v = 50 2u – 5v = 13 7u = 63 ⇒ u = 9

Replace the value in the first equation u + v = 10 ⇒ 9 + v = 10 ⇒ v = 1

Reverse the change of variables:

Systems of logarithmic equations

To solve systems of logarithmic equations, use the methods for solving linear systems and the properties of logarithms. This will make the system into a logarithmic system. Then you can solve it.

10 Solve the logarithmic system: 2 log x + log y = log 9 log x – log y = log 3 ⎫ ⎬ ⎭

a) Add the two equations: b) When you put x = 3 in the 2nd equation, you have: 3 log x = log 9 + log 3 log 3 – log y = log 3 log x 3 = log (9 ∙ 3) – log y = 0 ⇒ log y = 0 x 3 = 27 ⇒ x 3 = 33 ⇒ x = 3 y = 1

Solution: x = 3, y = 1

Solving problems with systems

To solve a problem with systems, use the same method as for equations, but now you have two equations and two unknowns.

Read the statement carefully until you identify the unknown, the data, the questions and the relationships. For geometric problems, you should always make a drawing. For numerical problems, you should make a diagram. Follow these four steps:

a) Unknown and data: write the unknown and the data.

b) Question: identify and write the question.

c) Approach and working out: write the unknown and the data. Formulate the relationship, transform it into an equation and sove it carefully.

d) Solution and verification: write the answers to the questions posed by the problem and check that they correctly fit the conditions.

Names of unknowns

If the names of the two objects start with different letters, use their first letters as unknowns. Or you can use x and y

If you know which value is smaller and which is larger, make the value of the smaller unknown x and the larger unknown y

11 Calculate the sides of a rectangle with a perimeter of 22 m and an area of 28 m2

1. Unknown

• lenght of base: b

• lenght of height: h b h

2. Question:

• What are the base and height of the rectangle?

3. Approach and working out:

Perimeter = 22 m. Semiperimeter = 11 m Base + height = 11 ⇒

+ h = 11

= 28

+ h = 11 b · h = 28 ⎫ ⎬ ⎭ Solve by substitution.

Solve for b en b + h = 11 ⇒ h = 11 – h

Substitute h in the 2nd equation: b · h = 28 (11 – h) h = 28 ⇒ 11h – h2 = 28 ⇒ – h2 + 11h – 28 = 0 ⇒ h2 – 11h

Substitute solutions in the equation:

Sides: b, h

Perimeter: 2b + 2h = 22 b + h = 11 Area: bh = 28

System: b + h = 11 bh = 28 ⎫ ⎬ ⎭

4. Solution:

The only solution is that one side measures 7 m and the other 4 m. Check your answer. Perimeter: 2 ∙ 7 + 2 ∙ 4 = 14 + 8 = 22 m Area: 7 ∙ 4 = 28 m2

13. Solve the exponential system: 3x + 5 y = 28

14. Find two numbers with a sum of 12 and a product of 35

15. Solve the logarithmic system: log (x – 1) – log (y + 3) = 0 2 log x + log (y + 1) = 4 log 2 ⎫ ⎬ ⎭

16. Find the sides of a right triangle with a hypotenuse of 10 m and legs with a proportion of 3 to 4

Household economics

For a family’s economy, it is essential to maintain a balance between income and expenses. To do this, it might be useful to draw up a budget with properly identified income and expenses. It is important to differentiate between different types of expenses:

• Required fixed expenses. These are required expenses that occur regularly; the amounts and payment dates are known. For example, housing, education, insurance, taxes, and loans are required fixed expenses.

• Necessary variable expenses. These are necessary expenses, but the amounts are not fixed. For example, grocery shopping, transportation, clothes, electricity, water, etc. All of these could be lowered if needed to cover the required fixed expenses.

• Superfluous or discretionary expenses. These are expenses that can be avoided. For example, a midmorning coffee, an impulse purchase, a subscription to a service that is rarely used, etc.

To manage the budget, you need to address what are the expenses, savings, debts, and investments in the family. Savings is a difficult category to control. A budget that correctly reflects expenses should also include savings as an additional expense, but how much should be allocated to this category? The 50-30-20 rule can be a good guide. It consists of dividing monthly income into the following percentages: 50% for required and necessary variable expenses, 30% for discretionary expenses, and 20% for savings.

The following table shows the monthly income and expenses for three families.

1 Does any family follow the 50-30-20 rule?

2 Does the case of family B make sense? To balance the budget, by what percentage should discretionary spending be reduced?

3 Open the Budget spreadsheet. Sheet 1 shows information for a family of 4: their monthly income and expenses, as well as their savings. Both the monthly income and expenses are taken from a pro rata of annual income and expenses. Analyse the budget in the example. Indicate whether it complies with the 50-30-20 rule and should there be an anomaly, state which items could be excluded.

SECTION 2

A mortgage is a financial product for the purchase of a house. It is a type of loan with a repayment period of up to 35 years. A mortgage is one of the required expenses that takes up the largest amount of money in a family’s annual budget. There are two types of mortgages:

• Variable mortgages: they have a variable interest rate that is usually Euribor plus a certain percentage. Thus, the rate varies according to changes in the value of Euribor.

• Fixed mortgages: the interest rate remains fixed throughout the repayment period.

1 A family was offered a variable mortgage for €140 000, to be repaid over 30 years at an interest rate of Euribor plus 2.5%

If they were to take out house insurance and life insurance and contribute €50 to a pension fund monthly, the interest rate would be Euribor plus 1%

At the beginning of the mortgage period, the Euribor was at 3.5% and they chose the option mentioned above, taking out insurance and contributing to the pension fund.

Open the Mortgage spreadsheet. Analyse the solved exercise on Sheet 1 and answer the following questions:

• What is their monthly mortgage payment?

• From the monthly payment, how much is capital and how much is interest?

• Are the monthly amounts of interest and capital paid constant?

• At the end of the first year, how much capital will they have repaid? What will be the outstanding capital?

• If the interest rate is maintained, how much interest will they have paid at the end of the 30 years?

• Analyse the amortisation graph and indicate in which period the interest paid is higher than the capital paid.

• If the family were to have money to make extra payments to reduce part of the loan, at what time would it be advisable to do so?

2 A family that is paying a mortgage has €40 000 of debt remaining to be paid over 5 years. The mortgage was taken out with a variable rate of Euribor + 0.75%. At this moment the Euribor is at 4%

From the Mortgage spreadsheet, open Sheet 2, edit the data to represent this situation, and answer the following questions:

• If the interest rate were to remain unchanged, how much interest would they pay over the 5 years?

• If the debt is reduced by €15 000, what would be the monthly installment, and how much would they save in interest by repaying the debt over the 5 years?

• Change the data in the spreadsheet to reduce the repayment period so that the monthly installment is similar to the initial one. How much interest would they pay in this case? Would they pay less interest by reducing the installment or by reducing the term?

• If they do not want to use their savings and choose to keep the €15 000 and invest it in a safe financial product over 5 years, what interest should they secure to obtain a return similar to what they would save by reducing the mortgage? Remember that 19% of the interest gained from an investment is paid in taxes.

If your teacher allows, you can work in a group. Open the Budget spreadsheet. On sheet 2, modify all the data needed to make a budget. You can work with monthly or annual income and expenses. There is income, such as bonuses, which can be earned in June and December. There are expenses, such as car or house insurance, which are paid on a certain month of the year.

1 Prepare a household budget and explain how much money a family can save if they have to pay rent.

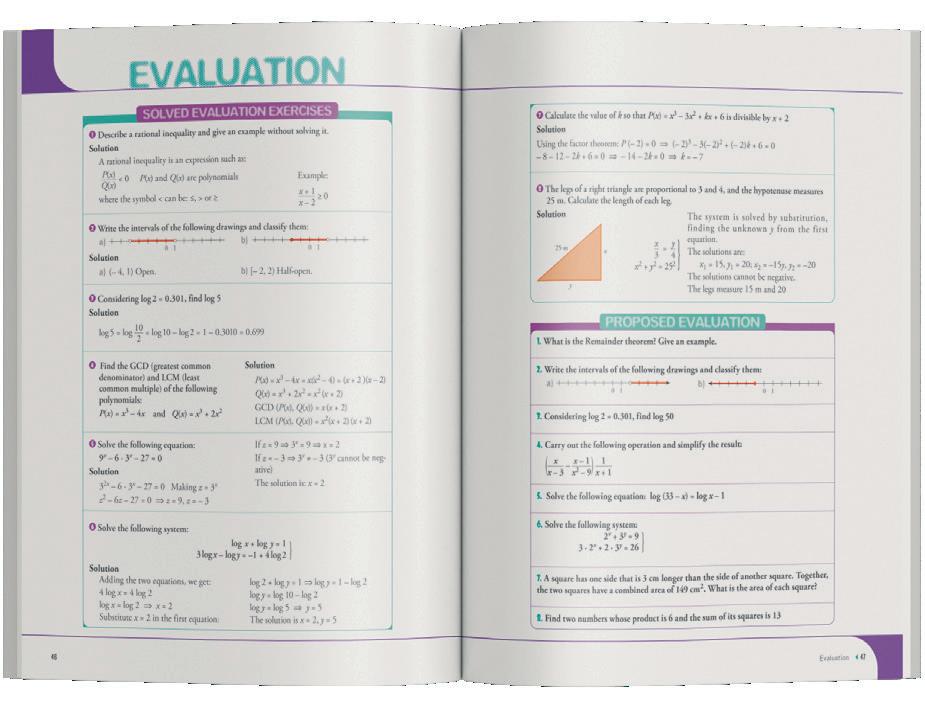
1 Describe a rational inequality and give an example without solving it.

Solution

A rational inequality is an expression such as: P(x) Q(x) < 0 P (x) and Q (x) are polynomials where the symbol < can be: ≤, > or ≥

Example: x + 1 x – 2 ≥ 0

2 Write the intervals of the following drawings and classify them: a) 0 1 b) 0 1

Solution a) (– 4, 1) Open. b) [– 2, 2) Half-open.

3 Considering log 2 = 0.301, find log 5

Solution

log 5 = log 10 2 = log 10 – log 2 = 1 – 0.3010 = 0.699

4 Find the GCD (greatest common denominator) and LCM (least common multiple) of the following polynomials:

P(x) = x3 – 4x and Q(x) = x3 + 2x2

5 Solve the following equation:

9x – 6 ∙ 3x – 27 = 0

Solution

32x – 6 ∙ 3x – 27 = 0 Making z = 3x z2 – 6z – 27 = 0 ⇒ z = 9, z = – 3

6 Solve the following system:

Solution

Solution

P (x) = x 3 – 4 x = x(x 2 – 4) = (x + 2 )(x – 2) Q (x) = x 3 + 2 x 2 = x 2 (x + 2)

GCD (P (x), Q (x)) = x (x + 2)

LCM (P (x), Q (x)) = x 2(x + 2) (x + 2)

If z = 9 ⇒ 3x = 9 ⇒ x = 2 If z = – 3 ⇒ 3x ≠ – 3 (3x cannot be negative)

The solution is: x = 2

log x + log y = 1 3 log x – log y = –1 + 4 log 2 }

Adding the two equations, we get:

4 log x = 4 log 2

log x = log 2 ⇒ x = 2

Substitute x = 2 in the first equation:

log 2 + log y = 1 ⇒ log y = 1 – log 2

log y = log 10 – log 2 log y = log 5 ⇒ y = 5

The solution is x = 2, y = 5

7 Calculate the value of k so that P(x) = x3 – 3x2 + kx + 6 is divisible by x + 2

Solution

Using the factor theorem: P (– 2) = 0 ⇒ (– 2)3 – 3(– 2)2 + (– 2)k + 6 = 0 – 8 – 12

8 The legs of a right triangle are proportional to 3 and 4, and the hypotenuse measures 25 m. Calculate the length of each leg.

Solution

The system is solved by substitution, finding the unknown y from the first equation.

The solutions are: x1 = 15, y1 = 20; x2 = –15, y2 = –20 The solutions cannot be negative. The legs measure 15 m and 20

1. What is the Remainder theorem? Give an example.

2. Write the intervals of the following drawings and classify them: a) 0 1

3. Considering log 2 = 0.301, find log 50

4. Carry out the following operation and simplify the result: ( x x – 3 –x – 1 x2 – 9) 1 x + 1

5. Solve the following equation: log (33 – x) = log x – 1

6. Solve the following system: 2x + 3y = 9 3 · 2x + 2 · 3y = 26 }

7. A square has one side that is 3 cm longer than the side of another square. Together, the two squares have a combined area of 149 cm 2 . What is the area of each square?

8. Find two numbers whose product is 6 and the sum of its squares is 13 b) 0 1

© GRUPO EDITORIAL BRUÑO, S. L., 2024 - C/ Valentín Beato, 21 - 28037 Madrid.

All rights reserved. No part of this publication may be reproduced, stored in a retrieval system, or transmitted, in any form or by any means, electronic, mechanical, photocopying, recording, or otherwise, without the prior permission of the publishers.