Harry O’Brien
250 mL 500 mL 750 mL 1 L NEW SOUTH WALES SYLLABUS 3
STAGE 2 MP_NSW_SB3_38329_TXT_4PP.indb 1 29-Aug-23 15:21:12
Greg Purcell
STUDENT BOOK
DRAFT


ii TERM 1 • Addition facts 2 • Skip counting 3 • Three-dimensional objects 4 • Centimetres 5 • Subtraction facts 6 • Writing and ordering numbers 7 • Symmetry 8 • Informal areas 9 • Changing addends to make 10 10 • Equal groups/multiplication 11 • Describing position 12 • Tally marks and column graphs 13 • Inverse operations of addition and subtraction 14 • Multiplication facts (2s) 15 • Prisms and cylinders 16 • Measuring in centimetres 17 • Odd and even numbers 18 • Connecting addition and subtraction 19 • Triangles 20 • Time in minutes 21 • Expanding 3-digit numbers 22 • Multiplication facts (5s) 23 • Grid maps 24 • Dot plots 25 • The division symbol 26 • Jump strategy for addition 27 • Parallel lines 28 • Informal capacity/litres 29 • Subtraction patterns/equal differences 30 • Halves, quarters and eighths 31 • Pyramids 32 • Calculating area 33 • Split strategy for addition 34 • Related multiplication facts (4s) 35 • Computer graph making 36 • Digital time 37 • Diagnostic review 1 38 TERM 2 • Using addition to solve subtraction 40 • Comparing and ordering fractions 41 • Angles 42 • The metre 43 • Counting by tens 44 • Thirds 45 • Patterns 46 • Informal mass 47 • Finding numbers on a number line 48 • Multiplication facts (10s) 49 • Symmetry 50 • Chance experiment/outcomes 51 • Subtraction jump strategy 52 • Fifths and tenths 53 • Angles 54 • Litres 55 • Bridging the decades/equality 56 • Dividing 57 • Modelling objects/nets 58 • Tables 59 • Rounding numbers 60 • 4-digit numbers 61 • Doubling and halving 62 • Quarter to and quarter past 63 • Addition patterns 64 • Revising multiplication facts 65 • Collecting data 66 • The kilogram 67 • Empty number lines 68 • Linking division and multiplication 69 • Following directions 70 • Measuring and recording perimeter 71 • Trading in addition 72 • Number patterns 73 • Describing prisms 74 • The square centimetre 75 • Diagnostic review 2 76 1 unit 2 3 4 5 6 7 8 9 10 unit 11 12 13 14 15 16 17 18 Contents MP_NSW_SB3_38329_TXT_4PP.indb 2 29-Aug-23 15:21:13 DRAFT


iii TERM 3 • Counting by tens or hundreds 78 • Multiplication facts (3s) 79 • Reflect, translate and rotate 80 • Chance—possible outcomes 81 • Trading in subtraction 82 • Division from multiplication 83 • Angles 84 • Millimetres 85 • 3-digit addition 86 • Calculating change 87 • Evaluating strategies 88 • Litres 89 • Trading in 3-digit subtraction 90 • Fractions of a quantity 91 • Turns 92 • Time intervals 93 • Multiplication facts/revision 94 • Levelling and constant difference 95 • Grids 96 • Collecting data 97 • Compensation strategy 98 • Division facts and strategies 99 • Testing predictions 100 • Scales 101 • Addition problems 102 • Fractions on a number line 103 • Polygons – trapeziums, rhombuses and kites 104 • The square metre 105 • 3-digit subtraction (trading) 106 • 4-digit numbers 107 • Octagons 108 • Cubic centimetres 109 • Multiplication mental strategies 110 • Division 111 • Interpreting maps/grid references 112 • Chance—likelihood 113 • Diagnostic review 3 114 TERM 4 • Checking work 116 • Commutative property 117 • Drawing objects 118 • Kilograms 119 • 3-digit addition (trading) 120 • Division problems 121 • Parallelograms 122 • Chance—likelihood 123 • Addition to 9999 124 • Complementary fractional parts 125 • Constant difference in subtraction/estimation 126 • Quadrilaterals 127 • Place value to tens of thousands 128 • Patterns on hundreds charts 129 • Nets 130 • The gram 131 • Number patterns 132 • Subtraction bar models 133 • Describing polygons 134 • Cubic centimetres 135 • Subtraction to 9999 136 • Associative property 137 • Non-standard partitioning 138 • Millilitres 139 • Introduction to decimals 140 • Multiplication strategies 141 • Combining and splitting shapes 142 • Length using decimal notation 143 • Problem solving 144 • Missing numbers 145 • Pentagons 146 • Square centimetres 147 • Diagnostic review 4 148 19 unit 20 21 22 23 24 25 26 27 28 unit 29 30 31 32 33 34 35 Dictionary 150 Answers 158 MP_NSW_SB3_38329_TXT_4PP.indb 3 29-Aug-23 15:21:15 DRAFT
The Maths Plus NSW Syllabus/Australian Curriculum series, Year K to Year 6, is based on the NSW Education Standards Authority 2023 Mathematics K–6 Syllabus for the Australian Curriculum Mathematics (ACARA). Each book after Year K builds upon prior knowledge and works towards an understanding of the achievement standards for the relevant year level and beyond. Maths Plus provides students with opportunities to sequentially develop their skills and knowledge in the strands of the Australian Curriculum Mathematics: Number, Algebra, Measurement, Space, Statistics and Probability

Series components
Student Books
Work towards achieving the relevant outcomes by developing skills and competency in understanding mathematical structures, fluency, reasoning and problem solving.
Mentals and Homework Books

Provide concise, essential revision and consolidation activities that correspond with the concepts and units of work presented in the Student Books.
Assessment Books
Include short post-tests with a simple marking system to assess students’ skills and understanding of the concepts in the Student Books.

Student Book features

• All pages are colour coded.


• Australian Curriculum Mathematics content descriptions, proficiency strand references and general capabilities appear on each page.
• The Dictionary (Years 2 to 6) features clear and simple explanations of mathematical terms and language.
Diagnostic term reviews
• Diagnostic term reviews (Years 1 to 6) assist in pinpointing students’ strengths and weaknesses, allowing intervention and re-teaching opportunities where required.
• The Find a topic page allows teachers the freedom to address particular topics and student needs as appropriate, providing essential revision and consolidation opportunities.
Teacher Book and Teacher Dashboard


Provide access to a wealth of resources and support material:
• curricula and planning documents
• interactive teaching tools
• potential difficulties videos
• learning activities
• support and extension activities
• reflection
• blackline masters and investigation pages
www.oxfordowl.com.au
is the home for Oxford
Primary professional resources.
• links to Advanced Primary Maths (Years 3 to 6)


• assessment tests
• answers for student resources
iv
Find a topic
Measurement
Dictionary
Number and Algebra
and Space
Statistics
and Probability
To the teacher
MP_NSW_SB3_38329_TXT_4PP.indb 4 29-Aug-23 15:21:19 DRAFT


v Find a topic NUMBER AND ALGEBRA Whole numbers 3-digit numeration 7, 22 4-digit & 5-digit numeration 61, 107, 128 Counting by 10s and 100s 44, 78 Rounding 60 Number lines 48 Non-standard partitioning 138 Decimal notation 140, 143 Odd and even numbers 18 Money 87 Number patterns 46, 73, 129, 132 Addition and subtraction Strategies 10, 14, 19, 27, 30, 34, 40, 52, 56, 64, 68, 88, 95, 98, 126, 144 Addition facts/subtraction facts 2, 6 Addition problems 102 Adding 2- and 3-digit numbers 72, 86, 102, 116, 120 Adding 4-digit numbers 124 Subtracting 2- and 3-digit numbers 82, 90, 106, 116 Subtracting 4-digit numbers 136 Inverse operations 14 Missing numbers 145 Empty number line 68 Levelling/constant difference 95, 126 Commutative property 117 Associative property 137 Subtraction bar models 133 Multiplication and division Strategies 3, 15, 23, 35, 49, 57, 65, 69, 79, 83 94, 99, 110, 141, 144 Multiplication 15, 23, 35, 49, 65, 69, 79, 83, 94 Division 26, 57, 69, 83, 99, 111, 121 Doubling and halving 62 Commutative property 117 Missing numbers 145 Fractions and decimals Common fractions 31, 41, 45, 53 Equivalent fractions 41 Fractions of a quantity 91 Fractions on a number line 103 Counting by fractions 125 Complementary fractional parts 125 MEASUREMENT AND SPACE Length Centimetres 5, 17 Metres 43 Perimeter 71 Decimal notation 143 Millimetres 85 Area Informal units 9 Calculating area 33 Square centimetres 75, 147 Square metres 105 Volume and capacity Informal units 29 Litres 29, 55, 89 Cubic centimetres 109, 135 Millilitres 139 Mass Informal units 47 Kilograms 67, 101, 119 Grams 131 Time Minutes 21 Digital time 37 Quarter to/quarter past 63 Analog time 93 STATISTICS AND PROBABILITY Chance Experiments 51, 81, 100 Describing likelihood 51, 81, 100, 113, 123 Data representation and interpretation Collecting data 13, 66, 97, 100 Column graphs 13, 25, 97, 100 Dot plots 25, 100 Computer graph making 36 Tables 59, 66, 97, 100 page page Three-dimensional space Three-dimensional objects 4 Prisms and cylinders 16, 74 Pyramids 32 Modelling objects/nets 58, 130 Drawing 3D objects 118 Two-dimensional space Symmetry 8, 50 Triangles 20 Parallel lines 28 Pentagons 146 Turn, reflect, translate and rotate 80, 92, 142 Octagons 108 Parallelograms 122 Quadrilaterals 127 Polygons 104, 134 Combine and split shapes 143 Angles Angles 42, 54, 84 Describing shapes 134 Position Describing position 12, 112 Grid maps 24, 96 Maps 70, 112 01_MP_NSW_SB3_38329_TXT_4pp.indd 5 31-Aug-23 13:35:05 DRAFT
NSW Syllabus Outcomes
MA2-RN-01 Representing numbers using place value
Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands
MA2-RN-02
Represents and compares decimals up to 2 decimal places using place value
MA2-AR-01 Additive relations
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers
MA2-AR-02
Completes number sentences involving addition and subtraction by finding missing values
MA2-MR-01 Multiplicative relations
Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems
MA2-MR-02
Completes number sentences involving multiplication and division by finding missing values
MA2-PF-01 Partitioned fractions


Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)
MEASUREMENT AND SPACE
MA2-GM-01
Geometric measure
Uses grid maps and directional language to locate positions and follow routes
MA2-GM-02
Measures and estimates lengths in metres, centimetres and millimetres
MA2-GM-03
Identifies angles and classifies them by comparing to a right angle
MA2-2DS-01
Two-dimensional spatial structure
Compares two-dimensional shapes and describes their features
MA2-2DS-02
Performs transformations by combining and splitting two-dimensional shapes
MA2-2DS-03
Estimates, measures and compares areas using square centimetres and square metres
MA2-3DS-01
Three-dimensional spatial structure
Makes and sketches models and nets of three-dimensional objects including prisms and pyramids
MA2-3DS-02
Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres
MA2-NSM-01
Non-spatial measure
Estimates, measures and compares the masses of objects using kilograms and grams
MA2-NSM-02
Represents and interprets analog and digital time in hours, minutes and seconds
MA2-DATA-01 Data
Collects discrete data and constructs graphs using a given scale
MA2-DATA-02
Interprets data in tables, dot plots and column graphs
MA2-CHAN-01 Chance
Records and compares the results of chance experiments
MAO-WM-01 Working mathematically
STATISTICS AND PROBABILITY
Develops understanding and fluency in mathematics through exploring and connecting mathematical concepts, choosing and applying mathematical techniques to solve problems, and communicating their thinking and reasoning coherently and clearly
vi
Units 1 2 3 4 NUMBER AND ALGEBRA
MP_NSW_SB3_38329_TXT_4PP.indb 6 29-Aug-23 15:21:19
DRAFT
MA2-RN-01 Representing numbers using place value
MA2-RN-02
MA2-AR-01 Additive relations
MA2-AR-02
MA2-MR-01 Multiplicative relations
MA2-MR-02
MA2-PF-01 Partitioned fractions


MA2-GM-01 Geometric measure
MA2-GM-02
MA2-GM-03
MA2-2DS-01
MA2-2DS-02
MA2-2DS-03
MA2-3DS-01
MA2-3DS-02
MA2-NSM-01
MA2-NSM-02
Two-dimensional spatial structure
MEASUREMENT AND SPACE
Three-dimensional spatial structure
Non-spatial measure
MA2-DATA-01 Data
MA3-DATA-02
MA2-CHAN-01 Chance
MAO-WM-01 Working mathematically
STATISTICS AND PROBABILITY
vii 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 NUMBER AND ALGEBRA
MP_NSW_SB3_38329_TXT_4PP.indb 7 29-Aug-23 15:21:19 DRAFT
What
Oxford University Press 2 1 unit CCT N Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
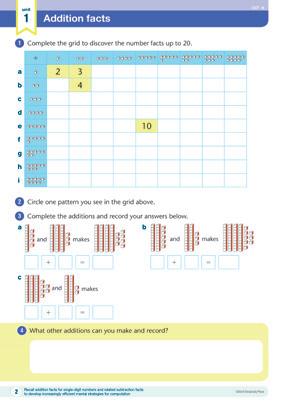
Complete the grid to discover the number facts up to 20. + a 2 3 b 4 c d e 10 f g h i Circle one pattern you see in the grid above. Complete the additions and record your answers below. a and makes b and makes + = + = c and makes + = 1 2 3
Addition facts
MP_NSW_SB3_38329_TXT_4PP.indb 2 29-Aug-23 15:21:20 DRAFT
other additions can you make and record? 4
Skip counting
Use skip counting to find the total number in each group. The first one has been started for you.
Use skip counting to find the total amount in each group, even though some are hidden.

Oxford University Press 3 1 unit Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems
a b c d e f
a b c
a 2 4 6 8 b 5 10 15 20 c 4 8 12 16 d 10 20 30 40 5 6 7 2 4 2, 4, 6, 8… MP_NSW_SB3_38329_TXT_4PP.indb 3 29-Aug-23 15:21:21
Complete these skip-counting patterns.
DRAFT
Three-dimensional objects
Colour all the prisms blue and tick all the cylinders to identify them from the rest of the objects.
MATCHES
Find and list some things in your classroom that are prisms and cylinders.
Draw a line matching each 3D object with its correct name.
DRAFT
Which objects above have curved surfaces?
Oxford University Press 4 unit 1 COMMUNICATING CCT L
Makes and sketches models and nets of three-dimensional objects including prisms and pyramids
sphere cylinder prism cone pyramid
8 9 10 11
Baked Beans
MP_NSW_SB3_38329_TXT_4PP.indb 4 29-Aug-23 15:21:23
and record the lengths of five pencils in your classroom.
Use the 1 cm dot paper to draw the following lines.

Length can be measured in centimetres. The short way of writing centimetres is cm. There are 100 centimetres in 1 metre. A Base 10 one is about 1 centimetre long.

Oxford University Press 5 unit 1 N Measures and estimates lengths in metres, centimetres and millimetres Centimetres d e f Measure
14
6 cm • • • • • • • • • • • • • b 9 cm • • • • • • • • • • • • • c 11 cm • • • • • • • • • • • • • d 8 cm • • • • • • • • • • • • • e 1 cm • • • • • • • • • • • • • f 10 cm • • • • • • • • • • • • • g 12 cm • • • • • • • • • • • • • 15
a
MP_NSW_SB3_38329_TXT_4PP.indb 5 29-Aug-23 15:21:24 DRAFT
Subtraction facts 2

Oxford University Press 6 unit CCT N Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Answer the questions. The number line may help you. 0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 a 10 take away 7 e 15 8 = i 17 minus 6 b 10 take away 4 f 16 7 = j 19 minus 9 c Difference between 13 and 6 g 18 8 = k Subtract 8 from 15 d Difference between 12 and 6 h 20 13 = l Subtract 5 from 13 Complete the subtraction targets. a 5 7 3 10 4 13 6 11 13 b 9 6 13 18 10 8 5 11 18 c 20 17 12 11 13 18 7 19 20 Supply the missing number to complete each fact. a 10 7 = f 18 5 = b 17 8 = g 18 13 = c 20 = 13 h 14 = 8 d 13 5 = i 14 = 6 e 14 = 7 j 21 7 =
from
2 1 2 3 4 ? MP_NSW_SB3_38329_TXT_4PP.indb 6 29-Aug-23 15:21:25 DRAFT
Discover the number sequence when 10 is subtracted
these numbers.
Writing and ordering numbers
Write the number represented by the Base 10 materials.
Hundreds Tens Ones Number
Use Base 10 materials to make more numbers, then record them.
Write each number in words. You may need to use some of the words in the box to help you with the spelling.
one, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen, fifteen, sixteen, seventeen, eighteen, nineteen, twenty, thirty, forty, fifty, sixty, seventy, eighty, ninety, hundred, thousand
a 321
b 737
c 295
d 574
Order the following numbers from smallest to largest.
a 256, 307, 291
b 999, 807, 364
c 259, 952, 529
d 742, 247, 472, 274
e 507, 607, 705, 605
Oxford University Press 7 unit COMMUNICATING, REASONING CCT Applies an understanding of place value and the role of zero to
numbers to at least tens of thousands 2
represent
a b c d 5
7
8
6
MP_NSW_SB3_38329_TXT_4PP.indb 7 29-Aug-23 15:21:26
DRAFT
A line of symmetry is a line that divides something exactly in half.

DRAFT
Oxford University Press 8 unit REASONING CCT Performs
2 Symmetry
transformations by combining and splitting two-dimensional shapes
d e f
MP_NSW_SB3_38329_TXT_4PP.indb 8 29-Aug-23 15:21:27
Jamal laid a plastic grid over the shapes. Use the grid to answer the questions.
a Which shape has the largest area?
b Which shape has the smallest area?
c Which two shapes have the same area?
d Which shape has an area of 16 square units?
Liam said that there was only one rectangle you could make with an area of 18 squares. He drew it below. Zoe thought that there was more than one.
See if you can draw more rectangles with an area of 18 squares to see who is correct.
Liam’s rectangle
18 squares
Emily covered her desk with round plates.
a Do you think these are good measuring units for area?
b Explain why or why not to a friend and then listen to what they have to say.
c Were their ideas the same as yours?
Oxford University Press 9 unit COMMUNICATING, PROBLEM SOLVING N Estimates, measures and compares areas using square centimetres and square metres 2 Informal areas
11
12 A B C D E F
13 MP_NSW_SB3_38329_TXT_4PP.indb 9 29-Aug-23 15:21:28 DRAFT
3 Changing addends to make 10
Look for pairs of numbers that make 10 when adding, e.g. 4 + 8 + 6 = 18. Add the 4 and 6 first.


By looking for numbers that add to make 10, the addition sum becomes easier for you to do.
How much did he save in the 3 months?
farm. How many animals did she see?
Mack and Myer Department Store
Calculate the cost of buying the following items.
a A baseball bat and a soccer ball $
b A teddy bear, a book and a car $
c A pen, a teddy and a car $
d A baseball bat, a teddy and a soccer ball $
On a piece of paper, write some of your own additions that have a 10 combination.

Oxford University Press 10 unit N CCT
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers
3
$4 $3 $15 $7 $6 $5
4
WORLD OF DOGS MP_NSW_SB3_38329_TXT_4PP.indb 10 29-Aug-23 15:21:30
DRAFT
Write number sentences to describe the groups.
2 groups of equals .
groups of equals .
groups of equals .
Draw groups to illustrate this number sentence: 3 groups of 6 equals 18
Oxford University Press 11 unit N CCT Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
Equal groups/multiplication 3
a
f
b 4 8 4 8 4 8 4 8
of
g
of equals . c 10 5 10 5 10 5 10 5 10 5
of equals . h
of equals . d
i
. e
j
groups of equals .
groups
equals .
groups
groups
groups
groups of equals .
groups of equals
5
MP_NSW_SB3_38329_TXT_4PP.indb 11 29-Aug-23 15:21:33
6
DRAFT
3 Describing position
Use the tray cupboard to answer the questions.
a Whose tray is on the top shelf on the right?
b Whose tray is in the middle of the shelf third from the bottom?
c Describe the position of Sienna’s tray.
d Write Harry’s name on his tray, second shelf from the top, on the left.
e Write Lauren’s name on her tray, second shelf from the bottom, in the middle.
f Write Zoe’s name on her tray, which is directly to the right of Brooke’s.
g Write Ethan’s name on his tray, which is in the bottom right corner.
h Describe the position of the tray with no name on it.
Find the secret message by placing letters on the shelves.
a Put a Y in the top left shelf.
b Put an I in the box in the 2nd top row and 2nd from the right.
c Put an S in the box in the 3rd bottom row, on the right.
d Put a V in the 2nd bottom row, on the left.
e Put a T in the 3rd row from the top and on the left.
f Put a D in the 2nd row from the top, on the right.
g Put an R in the 2nd row from the bottom and 2nd from the right.
Oxford University Press 12 unit N Uses grid maps and directional language to locate positions and follow routes
7 8 MP_NSW_SB3_38329_TXT_4PP.indb 12 29-Aug-23 15:21:33
DRAFT
Tally marks and column graphs
Tally the vehicles that are parked in or near your school.
Cars parked at my school
Manufacturer Tally
Ford
Holden
Toyota
Mitsubishi
Volkswagen
BMW
Subaru
Honda
Mazda
Hyundai
Other
a What was the most popular make of vehicle around your school?
b What was the least popular?
c Were any equally popular?
d What is your favourite make of car?
Mr Lee’s class did a survey and graphed the four most popular cars.
a Which was the most popular car?
b Which was the least popular car?
c Which car had a total of 6?
d Which car had a total of 5?
e How many more Holdens were there than Toyotas?
Oxford University Press 13 unit L CCT N Collects discrete data and constructs graphs using a given scale Interprets data in tables, dot plots and column graphs 3
9 10 Ford Holden Toyota Mitsubishi Number of
popular cars
of
1 2 3 4 5 6 7 MP_NSW_SB3_38329_TXT_4PP.indb 13 29-Aug-23 15:21:34
cars Most
Makes
cars
DRAFT
4 Inverse operations of addition and subtraction
Two subtraction number sentences can be written from 18 + 12 = 30. They are 30 – 12 = 18 and 30 – 18 = 12.

DRAFT

I had $12 but spent $7. Now I have $5. 12 – 7 = 5 7 + 5 = 12
On the board, the teacher wrote a number sentence that had an answer of 36. Write some addition number sentences that have a total of 36, then check your additions using subtraction.

Oxford University Press 14 unit PROBLEM SOLVING CCT N
and uses mental and written strategies for addition and subtraction
2- and 3-digit
number sentences
addition and subtraction
finding missing values
Selects
involving
numbers Completes
involving
by
12 9
3
14 5
9
d 18 4
14
e 13 11
2
20 12
8
b
=
+ = c
=
+ =
=
+ =
=
+ = f
=
+ =
3
36 Whole Part Part 30 18 12 MP_NSW_SB3_38329_TXT_4PP.indb 14 29-Aug-23 15:21:35
Use the arrays to solve the multiplication facts.
Use the arrays to complete the table of twos.
Solve the problems.
a Mrs Jones bought 7 books at $2 each. How much did she spend?
b Harry had 8 bags of 2 cakes. How many cakes did he have?

c Linh had 5 boxes with 2 books in each. How many books did she have?
d Austin bought 6 books at the shop for $2 each. How much change did he get from $20?
e Milla had 9 pairs of shoes. How many shoes did Milla have altogether?
Is there a relationship between the two times table and even numbers?
Learn your two times table over the next two weeks.
Oxford University Press 15 unit COMMUNICATING, PROBLEM SOLVING L N CCT WE Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
Multiplication facts (2s) 4
a b c d e 3 × 2 = 2 × 2 = 4 × 2 = 1 × 2 = 5 × 2 = f g h 6 × 2 = 8 × 2 = 7 × 2 = i j 10 × 2 = 9 × 2 =
4 6
1 × 2 = 2 × 2 = 3 × 2 = 4 × 2 = 5 × 2 = 6 × 2 = 7 × 2 = 8 × 2 = 9 × 2 = 10 × 2 = 5 2, 4, 6, 8…
8
7 MP_NSW_SB3_38329_TXT_4PP.indb 15 29-Aug-23 15:21:36 DRAFT
4 Prisms and cylinders
Study each set of faces and surfaces. Match each object to its set of faces or surfaces. Then, colour each 3D object and its set of matching faces or surfaces the same colour. The first one is done for you.
A vertex is where two or more lines meet to form an angle.
An edge is where two surfaces meet or intersect. Edges can be straight or curved.

unit COMMUNICATING, REASONING CCT L
9
MP_NSW_SB3_38329_TXT_4PP.indb 16 29-Aug-23 15:21:36
DRAFT
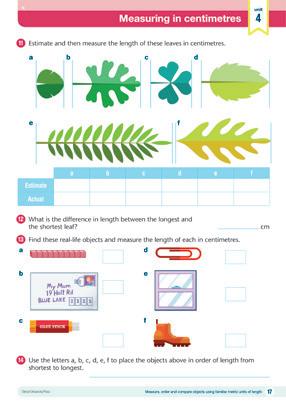
Estimate and then measure the length of these leaves in centimetres.
What is the difference in length between the longest and the shortest leaf? cm
Find these real-life objects and measure the length of each in centimetres.
Use the letters a, b,
to place the objects above in order of length from shortest to longest.
Oxford University Press 17 unit N Measures and estimates lengths in metres, centimetres and millimetres 4 Measuring in centimetres
a b c d e f
Estimate Actual
a d b e c f
c, d, e,
11 12 13 14 a b c d e f GLUE STICK GLUE STICK GLUE STICK GLUE STICK GLUE STICK GLUE STICK MP_NSW_SB3_38329_TXT_4PP.indb 17 29-Aug-23 15:21:37 DRAFT
f
5 Odd and even numbers

Colour the circles to model the numbers. Then tick the column to record if they are odd or even. The first one is done for you.
Odd Even a 12 ✔
b 13 c 18
d 25
e 28 f 31
Numbers that pair equally are even.
6
DRAFT
Shade all even numbers blue and odd numbers yellow.
All numbers that end in the digits 0, 2, 4, 6 and 8 are .
All numbers that end in the digits 1, 3, 5, 7 and 9 are .
Continue the skip counting patterns with odd and even numbers.
Oxford University Press 18 unit COMMUNICATING, REASONING N Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50
a 7 9 11 e 98 96 94 b 16 18 20 f 95 93 91 c 56 58 60 g 110 112 114 d 41 43 45 h 115 117 119 1 2 3 4 5
e.g.
MP_NSW_SB3_38329_TXT_4PP.indb 18 29-Aug-23 15:21:38
Connecting addition and subtraction
Use each addition fact to make two subtraction facts. The first one is done for you.
a 8 + 4 = 12 12 4 = 8 12 8 = 4
b 7 + 5 = 12 = =
c 6 + 9 = 15 = =
d 8 + 7 = 15 = =
e 9 + 8 = 17 = =
f 12 + 8 = 20 = =
g 13 + 6 = 19 = =
7
Write a number sentence for each problem, then solve it.
Problem
Number sentence
a Sally has 17 toys in a box. If she took 8 out, how many toys would be left in the box? =

b Kim needs $16 to buy a new game. If she has already saved $8, how much more does Kim need to save? =
c Harry had $20 in his bank account. He spent $14. How much money is left in the bank? =

=
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Oxford University Press 19 unit NUMERACY CCT
5
d Mrs Patel bought 2 dozen eggs. Unfortunately she tripped and smashed 11 of them. How many eggs are left? 6
MP_NSW_SB3_38329_TXT_4PP.indb 19 29-Aug-23 15:21:39 DRAFT
Write a story problem to suit this number sentence: 18 + 7 = 25. 8
Triangles
A triangle is made up of 3 straight sides and has 3 angles.



There are many different shaped triangles.
Cut straws to the lengths that are shown to see if they make triangles. Sketch the ones that do.






Is it possible for a triangle to contain a right angle?
Compares two-dimensional shapes and describes their features

20 unit Oxford University Press
REASONING N L
5
Shape Angles a b
a 3 cm 4 cm 5 cm b 5 cm 5 cm 5 cm c 4 cm 4 cm 9 cm
11 12 Shape Angles c d
MP_NSW_SB3_38329_TXT_4PP.indb 20 29-Aug-23 15:21:41 DRAFT
13
Time in minutes 5
A watch has:
a minute hand that shows minutes past the hour or minutes to the hour
an hour hand that shows the hour
a second hand that measures 60 seconds in each minute.
The minute hand counts off 60 minutes every hour. Each number on the clock represents a span of 5 minutes.
How many minutes does it take for the minute hand to move from:
a 12 to 1?
b 12 to 2?
c 12 to 3?
d 12 to 4?
e 12 to 6?
DRAFT
f 2 to 3?
g 4 to 6?
h 5 to 8?
i 6 to 9?
j 8 to 12?
The minute hand has fallen off Ben’s watch. Draw the minute hand on the watches below to show the times.
How many minutes does it take the hour hand to move from one number to the next?
Oxford University Press 21 unit L Represents and interprets analog and digital time in hours, minutes and seconds
a c e g b d f h
14 15 0 30 25 20 50 55 5 10 15 45 40 35 60 Counting minutes 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 5 past 12 10 past 12 20 past 12 1 2 past 12 15 past 12 12 o’clock 25 past 12 22 past 12 12 6 9 3 1 11 2 10 4 8 5 7
MP_NSW_SB3_38329_TXT_4PP.indb 21 29-Aug-23 15:21:42
Expanding 3-digit numbers 6
Use these number expanders to expand the numbers.
a 285 hundreds tens ones
b 743 hundreds tens ones
c 854 hundreds tens ones
d 999 hundreds tens ones
e 870 hundreds tens ones
f 809 hundreds tens ones
Expand each number. The first one has been done for you.
a 527 = 500 + 20 + 7
b 363 = + +
The symbols > (greater than) and < (less than) are used to compare numbers.

Use the greater than or less than symbol to make these number sentences true.
Forty-five is less than sixty-three. We write it like this:
45 < 63

unit L
g 347
= + +
h 296 =
+ +
i 390 = + +
694
j 470 = + +
856
k 508 = + +
797
l 609 = + + 1 2
c 725 = + +
d
= + +
e
= + +
f
= + +
a 45 63 h 153 298 o 864 67 b 72 51 i 504 376 p 67 325 c 86 49 j 900 899 q 63 9 d 37 80 k 401 921 r 504 405 e 8 81 l 569 385 s 327 723 f 21 45 m 216 621 t 528 347 g 89 53 n 308 925 u 999 100 3 MP_NSW_SB3_38329_TXT_4PP.indb 22 29-Aug-23 15:21:44 DRAFT
Write a multiplication fact to describe each array.
Use the arrays to complete the table of fives and other facts.
Revise your multiplication facts by solving the problems.
a Peyton saved $4 per week for 5 weeks. How much did she save?
b Stephen trains 5 days a week, running 5 km each day. How far does he run each week?

c Jarrah bought a ticket for $9 but Yindi’s ticket cost 5 times as much. How much did Yindi pay for her ticket?
Multiply by 10 then halve to solve these questions.
What happens when you multiply these numbers by zero in your calculator? 8
Learn your five times table over the next two weeks. 9
Oxford University Press 23 unit L N CCT WE Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values Multiplication facts (5s) 6
a b c d × = × = × = × =
a 1 × 5 = b 3 × 2 = 2 × 5 = 5 × 2 = 3 × 5 = 7 × 2 = 4 × 5 = 6 × 2 = 5 × 5 = 8 × 2 = 6 × 5 = 2 × 2 = 7 × 5 = 1 × 2 = 8 × 5 = 0 × 2 = 9 × 5 = 10 × 2 = 10 × 5 = 4 × 2 =
a 6 × 5 = c 10 × 5 = e 12 × 5 = b 5 × 5 = d 9 × 5 = f 16 × 5 = 4 5 6 7 2 5 10
8 x 5 = ? 8 x 10 = 80 half 80 = 40 MP_NSW_SB3_38329_TXT_4PP.indb 23 29-Aug-23 15:21:44
DRAFT
Grid maps
Grids help you locate places on maps. A grid uses lines to make rows and columns on a map. The rows go from side to side. The columns go from top to bottom. Letters are used to label the columns, and numbers label the rows.
DRAFT

What can be found in these grid references? a A1
F2
Are the shops north of Spin City School?
Is Lake Spin south of Jack’s house?
Give all the grid references for the shops.
Give all the grid references for Forest Park.
Tall Tales River passes through the following grid references. Answer true or false.
Is Tom’s house west of Forest Park?
Oxford University Press 24 unit
REASONING L Uses grid maps and directional language to locate positions and follow routes
6
e
f
g
d
D1 b F6
B5 c H1
G4
a
b
c
d
A4
C6
F3
B4
10 11 12 13 14 15 16 B A C D E F G H
MP_NSW_SB3_38329_TXT_4PP.indb 24 29-Aug-23 15:21:45
18 19
Dot plots
A dot plot is a number line that uses dots to record the frequency of events. For example, in this survey six people selected C.
on the picture graph?
The owners of the ten-pin bowling alley did a survey to find out who used their bowling alley on a Thursday afternoon. They recorded their data using tally marks.
DRAFT

Bowlers Tally Men
Women
Bowlers Tally Girls Boys
Create a dot plot to record the number of tenpin bowlers. Use one dot to represent each person. The faint grid might help you.
Answer the questions.
a How many men were at the bowling alley?
b How many girls were there?
c How many boys were there?
Bike Car
How we get to school Bus Walk
Thursday afternoon bowling
Men Women Girls Boys
Oxford University Press 25 unit 6 L CCT N Collects discrete data and constructs graphs using a given scale Interprets data in tables, dot plots and column graphs
A B C D E F
MP_NSW_SB3_38329_TXT_4PP.indb 25 29-Aug-23 15:21:45
The division symbol 7

Division can be expressed using the division symbol (÷) in number sentences.
The division symbol (÷) means: in groups of shared between divided by
Use the balls to help solve the divisions.
a 20 divided by 5 20 ÷
b 20 divided by 4 20 =
c 20 divided by 2 20 =
d 20 divided by 10 20 =
e 20 divided by 1 20 =
Use some counting materials to answer the questions, then check your answers with a calculator.
Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing
Oxford University Press 26 unit COMMUNICATING, REASONING N CCT WE
values
20 ÷ = 15 ÷ = 18 ÷ =
5 =
30 ÷ 5 = e 16 ÷ 4 = i 35 ÷ 5 =
32 ÷ 4 = f 18 ÷ 2 = j 28 ÷ 2 =
30 ÷ 2 = g 28 ÷ 4 = k 18 ÷ 3 =
25 ÷ 5 = h 24 ÷ 2 = l 24 ÷ 6 =
2 3 a
b
c
d
MP_NSW_SB3_38329_TXT_4PP.indb 26 29-Aug-23 15:21:49 DRAFT
Jump strategy for addition 7
Use jump strategies to solve the additions. The first one is done for you.


Use the jump strategy to add the numbers.
a 35 + 23 becomes 20 35 + + 3 =
b 42 + 25 becomes + + =
c 33 + 34 becomes + + =
d 46 + 24 becomes + + =
e 52 + 26 becomes + + =
32 + 25 becomes 32 + 20 + 5 = 57
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Oxford University Press 27 unit N CCT
a 27 + 14 = 41 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 b 37 + 20 = 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 c 28 + 13 = 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 d 39 + 23 = 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63
4 5 27
4.
+ 14? 27 add 10 then add
MP_NSW_SB3_38329_TXT_4PP.indb 27 29-Aug-23 15:21:50
DRAFT
Two or more lines that are exactly the same distance apart are called parallel lines. They do not have to be the same length and can be in any direction.
Shade all boxes that contain sets of parallel lines.
Some shapes have parallel sides.
a Is the broom parallel to the side of the door?
b Is the top of the door parallel to the leg of the table?
c Is the curtain rod parallel to the top of the window?
d Is the side of the door parallel to the leg of the table?
e Tick all horizontal lines that are found on the table.
Tick the shapes below that have parallel sides.

Oxford University Press 28 unit 7
Parallel lines
a d g b e h c f i
6 7
Study the sketch, then answer the questions.
REASONING
two-dimensional shapes and describes their features
Compares
Parallel
8
MP_NSW_SB3_38329_TXT_4PP.indb 28 29-Aug-23 15:21:51 DRAFT
Using three small containers like a mug, a tea cup and a glass, tally how many are needed to fill a 1 L bowl or container.
Tally
Informal capacity/litres
Containers needed
Use the grid to graph the results.
Draw lines to match the most suitable container to measure the capacity of the kettle, bath, medicine bottle and fruit-juice box.
Find three containers that have their capacity labelled and record them.
a Fruit juice 4 L c
Oxford University Press 29 unit REASONING L N Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres 7
12 11 10 9 8 7 6 5 4 3 2 1
11 45 mL 40 mL 35 mL 30 mL 20 mL
b d 12 300 L 100 mL Medicine
Mug Tea
Glass 9
Vessel
cup
10
1 L MILK MP_NSW_SB3_38329_TXT_4PP.indb 29 29-Aug-23 15:21:52
1 litre
DRAFT
8



Subtraction patterns/equal differences
We know that 7 4 = 3, so 70 40 must equal 30. Complete these examples to extend the basic number facts. The first one has been done for you.
You can add or subtract numbers from number sentences to make equal differences. Equal differences can make calculations easier for everyone.

If you add 2 to both sides of 66 – 28 you get 68 – 30.
72 – 39 becomes 73 – 40 Much easier!
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Oxford University Press 30 unit COMMUNICATING, REASONING N CCT
a 8 5 = 3 80 50 = 30 b 9 3 = 90 30 = c 8 6 = 80 60 = d 9 6 = 90 60 = e 9 7 = 90 70 = f 12 4 = 120 40 = g 13 4 = 130 40 = h 14 6 = 140 60 = 1
73 – 38 = 92 – 47 = 85 – 47 = 165 – 38 =
7 – 3 = 4 70 – 30 = 40 Cool! MP_NSW_SB3_38329_TXT_4PP.indb 30 29-Aug-23 15:21:54
DRAFT
Halves, quarters and eighths
Fractions can be written using a numerator and a denominator
1 4
The numerator shows us how many parts out of the whole (the fractional part).
g h
DRAFT
out of out of out of
Three children folded strips of paper.
Ravi Alisha Sam
Who folded their paper into quarters?
Who folded their paper into halves?
Who folded their paper into eighths?

Oxford University Press 31 unit Represents and compares
and
a
8
halves, quarters, thirds
fifths as lengths on
number line and their related fractions formed by halving (eighths, sixths and tenths)
i
5 6
7
The denominator shows us how many equal parts are in the whole. MP_NSW_SB3_38329_TXT_4PP.indb 31 29-Aug-23 15:21:55
8 Pyramids
Identify the faces, edges and vertices of the pyramids.

a Draw a coloured circle on all the vertices.
b Trace over all the edges with a coloured pencil.
edge face vertex
Draw a set of faces for each pyramid.
DRAFT
c Shade the three faces you can see in different colours.
What is the difference between a square pyramid and a cube?
Pyramids have only 1 base and all other faces are triangular.
32 unit COMMUNICATING L CCT
Makes and sketches models and nets of three-dimensional objects including prisms and pyramids
a b c
8 9 10
ICE
MP_NSW_SB3_38329_TXT_4PP.indb 32 29-Aug-23 15:21:56
BLOCK
Calculating area 8
Callum is setting the table for dinner. He has laid out a new tablecloth and some placemats. He has decided to measure the area of the tablecloth using the placemats. Placemat

It’s just like an array.
Tablecloth
Use the diagram to solve the problems.
a How many placemats has he used so far?
b How many placemats fit across the length of the table?
c How many placemats fit down the width of the table?
12
Explain the strategy you would use to work out how many placemats would fit on the table.
DRAFT
Jon and Ky were each given identical L shapes which they covered with square and rectangular grid overlays. Jon said his shape was bigger because he used more squares than Ky’s rectangles.
13
Explain why you agree or disagree with Jon.
Oxford University Press 33 unit COMMUNICATING, PROBLEM SOLVING N CCT Estimates, measures and compares areas using square centimetres and square metres
11
MP_NSW_SB3_38329_TXT_4PP.indb 33 29-Aug-23 15:21:57
Split strategy for addition 9
Add the following numbers by adding the tens part, then adding the ones part, then combining them. The first one has been done for you.
a 23 + 44 becomes 60 + 7 = 67
b 33 + 52 becomes + =
c 43 + 44 becomes + =
d 38 + 23 becomes +
e 27 + 45 becomes +
f 66 + 18 becomes +
g 49 + 44 becomes +
h 36 + 56 becomes + =
i 45 + 47 becomes + =
j 56 + 28 becomes + =
k 29 + 47 becomes + =
l 48 + 36 becomes +
Calculate the distance travelled to make the following journeys.
a Capital Hill to North Point via Light House.
b North Point to Cheetah Town via Leopard Hill.
c Cheetah Town to Capital Hill via Kangaroo Valley.
d Capital Hill to North Point via Possum Place.
e Light House to Leopard Hill via North Point and Possum Place.
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Use your head. 57 + 26 becomes 50 + 20 = 70 plus 7 + 6 = 13, so 70 + 13 = 83.

Oxford University Press 34 unit PROBLEM SOLVING CCT N
=
=
=
=
=
1 2 Kangaroo Valley Possum Place Cheetah Town Cheetah Island Capital Hill Light House North Point Leopard Hill 36 km 24 km 28 km 35 km 47 km 47 km 27 km 26 km 29 km
MP_NSW_SB3_38329_TXT_4PP.indb 34 29-Aug-23 15:21:58
DRAFT
Related multiplication facts (4s)

Complete the tables. The arrays will help you. a
Use the grid to answer the questions.
a Circle the table of 2s.
b Colour the table of 4s.
c What did you notice?
Use the “double then double again” strategy to multiply these numbers by 4.
Think double 6 = 12. Then double 12 = 24.
Learn your four times tables over the next two weeks.
Oxford University Press 35 unit PROBLEM SOLVING L N
and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values 9
Represents
b 3 1 × 2 = 2 × 2 = 3 × 2 = 4 × 2 = 5 × 2 = 6 × 2 = 7 × 2 = 8 × 2 = 9 × 2 = 10 × 2 = 1 × 4 = 2 × 4 = 3 × 4 = 4 × 4 = 5 × 4 = 6 × 4 = 7 × 4 = 8 × 4 = 9 × 4 = 10 × 4 =
4 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100
a 1 × 4 = f 7 × 4 = k 11 × 4 = b 3 × 4 = g 6 × 4 = l 12 × 4 = c 5 × 4 = h 8 × 4 = m 15 × 4 = d 2 × 4 = i 10 × 4 = n 20 × 4 = e 4 × 4 = j 9 × 4 = o 25 × 4 =
5 6 6 × 4 = ?
MP_NSW_SB3_38329_TXT_4PP.indb 35 29-Aug-23 15:21:59
DRAFT
9 Computer graph making
Mrs Tam’s class did a survey and made a tally of some very popular cars used by students’ parents to take them to school.
The class then made a dot plot of the data they collected.
How we get to school
Computer graph making
On a computer, make a column graph and a pie graph of the data presented in the dot plot above.
You may need to fill in a data grid that looks like this.
Examples of what the graphs may look like are supplied below.
Collects discrete data and constructs graphs using a given scale Interprets
Oxford University Press 36 unit N L CCT
data in tables, dot plots and column graphs
Ford Holden Toyota Mitsubishi
Ford Holden Toyota Mitsubishi
7 A B Series 1 C How we get to school Ford Holden Toyota Mitsubishi 6 7 5 4 8
7 6 5 4 3 2 1
Ford Holden Toyota Mitsubishi
Brands
Number of cars 0
Column graph
Pie graph
Ford Holden Toyota Mitsubishi MP_NSW_SB3_38329_TXT_4PP.indb 36 29-Aug-23 15:22:00
Series 1
DRAFT
Digital time is always read as minutes past the hour.
E.g. 12:35 is read as 35 minutes past 12 or twelve thirty-five. :
I eat lunch at
DRAFT
f Eleven twenty-six
Write digital times for the times given in words.

a 25 minutes past 3 : e six thirty-two :
b 35 minutes past 4 : f seven forty-three :
c 13 minutes past 12 : g nine forty-one :
d 56 minutes past 10 : h 39 minutes past 1 :
9 10
Mrs Wilson has four appointments today which she has listed in alphabetical order. Put them in the order that she will see her visitors throughout the morning.


Notes
Alana 11:45
Bree 10:30
Fran 9:45
Hilda 11:10
Name Time
My appointment is at 9:45.
Oxford University Press 37 unit L Represents and interprets analog and digital time in hours, minutes and seconds 9 Digital time
MP_NSW_SB3_38329_TXT_4PP.indb 37 29-Aug-23 15:22:02
d 3 + 8 + 7 = g 5 + 8 + 5 = e 6 + 8 + 4 = h 30 + 30 =
f 6 + 9 + 4 = i 40 + 50 =
Use the split strategy to add these numbers.
j 23 + 35 50 + =
k 37 + 52 + =
l 26 + 33 + =
m 23 + 76 + =
Answer the subtractions.
a – 6 18 20 12 24 16 4
b 8 5 = f 90 7 =
c 12 7 = g 80 50 =
d 15 6 = h 120 40 =
e 25 4 = i 130 60 =
Use skip counting to find the total number in each group.
multiplications.
divisions.
a Write 267 in the numeral expander. hundreds tens ones
Expand these numbers.
b 569 + +
c 267 + +
d 853 + +
4
Oxford University Press 38 Diagnostic review 1 PART Solve the additions. a b 9 + 7 7 10 8 6 2 4 3 15 + 20 11 7 3 4 1 5 10 c
+ =
1
Solve the
f 20 ÷ 2 = g 20 ÷ 5 = PART
PART
a b c Solve the
d × 2 e × 5 2 2 5 5 3 3 4 6 6 8 3
PART
2
MP_NSW_SB3_38329_TXT_4PP.indb 38 29-Aug-23 15:22:02
DRAFT
Label the cube’s parts with the words ‘face’, ‘edge’ and ‘vertex’.
a Who read the most books?
b Who read four books?
c Who read only two books?
Oxford University Press 39 Diagnostic review 1 PART Colour the larger shape. 8 PART Measure each line in centimetres. a cm b cm c cm d cm e cm PART Draw the minute hands on the clock faces. a 5 past 9 b 15 past 8 c 25 past 12 9 10 PART 11 PART Colour the boxes that have
lines. 6
Colour
to: 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10
parallel
d
the shape this set of faces belongs
PART
a b c 5 a b c d e f PART
the line of
of
shape. 7 12 10 8 6 4 2 0
Use
symmetry to help you draw the other half
this
MP_NSW_SB3_38329_TXT_4PP.indb 39 29-Aug-23 15:22:03 DRAFT
Mary
Tim Josh Sarah Effie Books read this year
10 Using addition to solve subtraction
Use known addition facts to answer the subtractions. The first one is done for you.
a 12 7 think 7 + 5 = 12
b 14 7 think + =
c 13 7 think + =
d 19 12 think + =
e 20 13 think + =
f 17 11 think + =
Demonstrate how addition can be used to solve subtractions. The first one is done for you.


a 38 32 think 32 + 6 = 38
b 47 42 think + =
c 58 51 think + =
d 69 61 think + =
e 22 18 think + =
f 33 27 think + =
g 142 138 think + =
h 154 148 think + =
Explain how you would solve 67 62 = . 3
13 minus 5? Think 5 + 8 = 13. The answer is 8. 39
Oxford University Press 40 unit PROBLEM SOLVING N CCT
1 2
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values MP_NSW_SB3_38329_TXT_4PP.indb 40 29-Aug-23 15:22:04
– 32? Think 32 + = 39.
DRAFT
Comparing and ordering fractions
Shade the fractions.
Order the fractions above from smallest to largest. 8 , 8 , 8 , 8
5 Order the fractions below from smallest to largest.
a 7 8 6 8 4 8 2 8
b 2 8 5 8 1 8 6 8
c 1 8 7 8 2 8 4 8
d 3 8 5 8 6 8 2 8
What other fraction could 4 8 be equal to?
Compare the fractions in the table above to find out whether the statements are true or false.
greater than < less than = equal to


Tell a friend what you noticed about fractions that have the same number for the denominator and numerator, e.g. 2
and 8 8

Oxford University Press unit
a 3 8 b 1 8 c 4 8 d 7 8
1 4 3 4 2 4 4 4
e
a 7 8 > 2 8 e 1 2 > 1 8 b 3 4 > 1 4 f 1 2 > 1 4 c 5 8 > 2 8 g 8 8 > 1 2 d 7 8 > 3 8 h 1 2 = 3 8 4 5 6 7 Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths) L 10
2
4 4
8 Eighths
,
>
1 2 2 2 1 4 2 4 3 4 4 4 1 8 2 8 3 8 4 8 5 8 6 8 7 8 8 8 1 whole Fraction wall MP_NSW_SB3_38329_TXT_4PP.indb 41 29-Aug-23 15:22:05 DRAFT
An angle is the amount of opening between two arms. Angles can be measured and named according to the size of their opening.
This common angle is called a right angle.

It is equal to a 1 4 turn of the hands on a clock face.
than a right angle?
e How many angles are larger than a right angle?
f How many right angles are there?
10 11
Name five angles larger than a right angle found in your classroom.
Construct some angles on geoboards, then draw them below.
Right angles
Angles smaller than a right angle
Angles larger than a right angle
Oxford University Press 42 unit COMMUNICATING, REASONING L Identifies angles and classifies them by comparing to a right angle Angles 10 ,
11 12 1 2 3 4 5 6 7 8 9 10 opening MP_NSW_SB3_38329_TXT_4PP.indb 42 29-Aug-23 15:22:06
DRAFT
Length can be measured in metres. The short way of writing metres is m. There are 100 centimetres in 1 metre.



Cut a streamer that is one metre long and use it to measure the objects to complete
Measure the length of these objects to the nearest metre using a metre rule or tape measure.



How many centimetres are there in:

Oxford University Press 43 unit COMMUNICATING, REASONING N Measures and estimates lengths in metres, centimetres and millimetres The metre 10
Length
b Length of a short
c Length of a gym mat About metres About metres About metres d Length of your classroom e Length of a netball court f Length of a hallway About metres About metres About metres
a
of a car
path
a 2 m? b 4 m? c 5 m? d 7 m? e 1 2 m? 13 14
MP_NSW_SB3_38329_TXT_4PP.indb 43 29-Aug-23 15:22:07 DRAFT
Counting by tens 11
Count forward by tens to complete each sequence.
Count backwards by tens to complete each sequence.
Use your counting by ten skills to quickly count the pencils.

Oxford University Press 44 unit N L
Completes number
subtraction
missing values
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers
sentences involving addition and
by finding
a 30 40 50 e 180 190 200 b 60 70 80 f 176 186 196 c 23 33 43 g 150 160 170 d 8 18 28 h 238 248 258
a 90 80 70 e 88 78 68 b 100 90 80 f 245 235 225 c 140 130 120 g 654 644 634 d 190 180 170 h 75 65 55 Supply the missing numbers to complete the sequences. a 87 97 107 127 157 b 65 85 105 115 c 727 747 777 807 d 364 404 434 444
1 2 3 4 MP_NSW_SB3_38329_TXT_4PP.indb 44 29-Aug-23 15:22:08 DRAFT
Adil folded three strips of paper then sketched dotted lines on their folds.
a Put a tick on the paper he folded into thirds.



b Draw a face on the paper he folded into halves.
c Draw a square on the paper he folded into quarters. Shade the shapes to match the thirds.
a 1 3 b 2 3 c 3 3 5 6 Oxford University Press 45 unit Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions
by halving (eighths, sixths and tenths) 11 Thirds 1 whole Fraction wall 1 2 2 2 Halves Thirds 1 4 1 3 2 3 3 3 2 4 3 4 4 4 Quarters
3 4 > 1 4 > greater than < less than MP_NSW_SB3_38329_TXT_4PP.indb 45 29-Aug-23 15:22:10 DRAFT
formed
A
netball court is divided into thirds.
Continue the number patterns modelled by the shapes, then write a rule for each one. a
3 6 9
4 8 12
6 12 18
5 10 15
8 16 24
Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
Oxford University Press 46 unit COMMUNICATING L CCT
11
Rule b
Rule
c
Rule d
Rule e
2 4 6
Rule f
Rule 8 Patterns MP_NSW_SB3_38329_TXT_4PP.indb 46 29-Aug-23 15:22:10
DRAFT
Use a pan balance to see how many Base 10 longs, marbles and pencils are needed to balance a calculator.
Why were the results different?

Which unit was the best for measuring the mass of the calculator?
Explain why.
Use Base 10 longs as a measuring unit to estimate and measure the mass of these objects. Lift each item to help with your estimates. Then measure and record the mass of each object.
Remember to do estimates and measure one item at a time.
47 unit Oxford University Press 11 Informal mass Estimates, measures and compares the masses of objects using kilograms and grams
9
Item Estimate Measurement a b Dictionary Dictionary c d 10 11 12 13 Base 10 longs marbles pencils 1 ON OFF 3 0 C 4 5 6 7 8 9 1 OFF 2 3 0 4 5 6 7 9 1 OFF 2 3 0 4 5 6 7 9
REASONING, COMMUNICATING, PROBLEM SOLVING N MP_NSW_SB3_38329_TXT_4PP.indb 47 29-Aug-23 15:22:11 DRAFT
Finding numbers on a number line
Put a cross to describe the position of each number on the number lines.
Put a cross on the number lines to estimate the numbers.
a 10 50 0 10 20 30 40 50 60 70 80 90 100 b 90 30 0 50 100 c 25 70 0 50 100 d 5 40 0 100 e 15 65 0 100
a 50 75 0 100 b 50 100 0 200 c 250 600 0 1000 d 1000 1500 0 2000 1 2 Oxford University Press 48 unit PROBLEM SOLVING, COMMUNICATING N CCT Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands
MP_NSW_SB3_38329_TXT_4PP.indb 48 29-Aug-23 15:22:12 DRAFT
12
Use the array to complete the tables.
The two machines magically multiply the amounts put in by 2 and by 10. Calculate the answers after the amounts have been through the machines.
What if …?
a If 4 friends each ate 10 cakes, they would have eaten cakes.

b If 10 friends each bought a pair of socks, there would be socks.
c If 6 friends each paid $10, they would have spent $ .
d If 4 friends each scored 10 points, they would have a total of points.
e If 7 friends each wrote 10 pages, they would have written pages. Learn your 10 times table over the next two weeks.
Oxford University Press 49 unit REASONING N CCT L Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values 12
Multiplication facts (10s)
a 1 × 10 = k 30 ÷ 10 = b 2 × 10 = l 20 ÷ 10 = c 3 × 10 = m 10 ÷ 10 = d 4 × 10 = n 90 ÷ 10 = e 5 × 10 = o 60 ÷ 10 = f 6 × 10 = p 70 ÷ 10 = g 7 × 10 = q 40 ÷ 10 = h 8 × 10 = r 50 ÷ 10 = i 9 × 10 = s 80 ÷ 10 = j 10 × 10 = t 100 ÷ 10 =
Times 10 Rule 5 6 8 4 2 Times 2 Rule f g h i j 6 3 7 9 5 a b c d e
3 4 5 6 MP_NSW_SB3_38329_TXT_4PP.indb 49 29-Aug-23 15:22:12 DRAFT
Discover the facts about these shapes.
a Trace over the following shapes, then cut them out.
b Carefully fold the shapes to discover the number of lines of symmetry for each shape.
c Draw the lines of symmetry on the shapes below.
d Does each line of symmetry break the shape into two identical shapes?
e Record the number of sides, angles and lines of symmetry for each shape.
DRAFT
Do these two quadrilaterals have lines of symmetry?
Compares two-dimensional shapes and describes their features
Oxford University Press 50 unit REASONING CCT
Symmetry 12
7 Shape Number of sides Number of angles Lines of symmetry
8 MP_NSW_SB3_38329_TXT_4PP.indb 50 29-Aug-23 15:22:13
Heads or tails?
a What are the two possible outcomes for flipping a coin?


b What do you think the result will be if a coin is tossed 30 times?

c In a group, toss a coin 30 times to discover which side comes up most. Use the table to tally your results. ( )
d Which side came up most in your experiment, heads or tails?
e Did the result of your experiment turn out as you thought it would, or was it different? Discuss this with your group and record your findings.
Colour the coins to create a picture graph of your result.
There are four possible ways that two coins can land when tossed into the air.

51 unit COMMUNICATING L N CCT 12 Chance experiment/outcomes
9 10 Tally
MP_NSW_SB3_38329_TXT_4PP.indb 51 29-Aug-23 15:22:15
DRAFT
Subtraction jump strategy 13
Use jump strategies to solve the subtractions. The first one is done for you.

Use the jump strategy to answer the questions.
a 40 16 think 40 10 6
b 50 24 think 50
c 60 33 think 60
d 34 13 think 34
e 35 21 think 35
f 46 23 think 46
g 57 32 think 57
Think! 57 – 20 = 37. Now take away 3, equals 34.
a 50 23 = 27 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 b 59 17 = 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 c 62 24 = 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 d 79 23 = 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80
=
=
=
=
=
=
= 1 2 57 – 23 = ?
Oxford University Press 52 unit N CCT Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
MP_NSW_SB3_38329_TXT_4PP.indb 52 29-Aug-23 15:22:16
DRAFT
Write fractions to represent the shaded part of each shape.
Shade the shapes to match the fractions.
Write the fractions in ascending order.
Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)

Halves, quarters and eighths Oxford University Press 53 unit
and tenths 13
Fifths
a 5 b c d e f
a 3 5 b 7 10 c 1 2 d 3 10 e 4 5 f 5 10 g 1 5 h 10 10
a 1 5 4 5 3 5 2 5 b 7 10 9 10 8 10 6 10 c 6 10 5 10 7 10 3 10 d 4 5 5 10 6 10 2 5 e 9 10 1 5 1 10 3 5 f 8 10 2 5 9 10 3 5
a 1 5 of 5 stars b 1 10 of 10 circles c 1 5 of 10 apples 3 4 5 6 Is 3 5 more than 6 10 ? MP_NSW_SB3_38329_TXT_4PP.indb 53 29-Aug-23 15:22:17 DRAFT
Shade the fractions.
Sometimes an angle is formed when only one arm of the angle is visible, such as a door opening or a car driving up a hill. In these cases you have to visualise where the other arm would be.
DRAFT
Answer the questions.
a Which door formed the smallest angle?
b Which door formed the largest angle?
c Is the angle formed by door 1 larger than the angle formed by door 3?
d Which door formed the closest to a right angle?
9 10

In your school, find two examples of angles with hidden arms.

Oxford University Press 54 unit COMMUNICATING L Identifies angles and classifies them by comparing to a right angle Angles 13
MP_NSW_SB3_38329_TXT_4PP.indb 54 29-Aug-23 15:22:19
The paint cupboard door forms an angle.
Capacity can be measured in litres.
The symbol for litre is a capital L. The most common example of a litre in everyday use is the milk carton.

Many everyday products such as milk, cordial, ice cream, soft drink and detergent are sold in containers measured in litres. Collect five containers that are marked in litres and list them below, along with the number of litres they each hold.
Container
Number of litres
DRAFT
Use a 1 L milk carton to estimate and measure the capacity of these containers.
Oxford University Press 55 unit REASONING L N Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres 13 Litres
1 L MILK
11 12 Container Estimate Litres a Ice cream L b Saucepan L c Bucket L d Watering can L e Sink L a b c d e 1 L MILK SHAMPOO 1 L Fizzy 1.25 L ICE CREAM MP_NSW_SB3_38329_TXT_4PP.indb 55 29-Aug-23 15:22:20
Bridging the decades/equality 14
Add these numbers by breaking up the second number and adding to a ten first.

a 9 + 6 becomes 9 + 1 + 5 = 15
b 8 + 7 becomes + + =
c 17 + 5 becomes + + =
d 16 + 5 becomes + + =
e 28 + 4 becomes + + =
f 47 + 5 becomes + + =
g 38 + 5 becomes + + =
h 54 + 17 becomes 10 + 6 + 1 = 54 +
i 39 + 13 becomes + + = +
j 46 + 26 becomes + + = +
k 67 + 27 becomes + + = +
Write the missing numbers to make the number sentences equivalent.
Write true or false. a 31 + 23 = 42 + 12
b 80 25 = 38 + 19
36
Oxford University Press 56 unit N CCT
+ 17 = ? Think! 36 + 10 = 46 Now add 4 and 3 more.
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
f 96 23 = 50 +
15
25 10 g 88 25 = 58 +
42
15 + 15 h 75 = 43 + 8
36
8 i 67 36 = 19 +
j 49 17 = + 16
a 20 5 = 9 +
b
=
c
=
d
13 = +
e 50 = 16 + 9
c 48 23 = 13 + 17
d 56 14 = 38 + 3 1 2 3 MP_NSW_SB3_38329_TXT_4PP.indb 56 29-Aug-23 15:22:21 DRAFT
Use the counters to solve the problems.
a Ten marbles were shared between two girls. How many did each girl receive? marbles
b Fifteen toys were shared among three children. How many did each child receive? toys
c Fourteen pencils were shared between two boys. How many did each boy receive? pencils
d Twelve stickers were shared among four children. How many did each child receive? stickers
Use the arrays to answer the division facts. a b c d 8 ÷ 4 = 12 ÷ 3 = 15 ÷ 3 = 18 ÷ 3 = e f g h 9 ÷ 3 = 16 ÷ 4 = 20 ÷ 5 = 24 ÷ 6 = i j k l 12 ÷ 4 = 20 ÷ 4 = 25 ÷ 5 = 30 ÷ 6 = Draw arrays to model the division number sentences. a 10 ÷ 2 = 5 b 15 ÷ 3 = 5 c 18 ÷ 3 = 6
4 5 6 Oxford University Press 57 unit Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values PROBLEM SOLVING, COMMUNICATING CCT N 14 Dividing MP_NSW_SB3_38329_TXT_4PP.indb 57 29-Aug-23 15:22:21 DRAFT
Modelling objects/nets 14
Construct the prisms below using centicubes or similar building cubes. After you have correctly modelled each prism, pull it apart and record how many blocks were used in its construction.
Use polydrons or paper nets copied onto 2 cm grid paper to discover which nets fold to form a cube. Put a cross on the ones that form a cube.
Describe to a friend how you folded one of the nets to form a cube.
Oxford University Press 58 unit Makes and sketches models and nets of three-dimensional objects including prisms and pyramids COMMUNICATING, PROBLEM SOLVING, REASONING
a b c d e f 7
a b c d 8
9 MP_NSW_SB3_38329_TXT_4PP.indb 58 29-Aug-23 15:22:22
DRAFT
Mr Riley’s class searched the playground for insects and recorded their findings in a table.
Ants Flies Butterflies Cicadas Cockroaches Bees
50 24 4 0 8 11
Interpret the table.
a Which was the most common insect?
b Which insect did they fail to find and why do you think this was so?
c What can you say about the number of ants found compared to the number of butterflies?
d Of which insect were there 6 times more than butterflies?
e Create your own table by recording the number of children with each hair colour in your class.
Blonde Brown Black Fair Red Other
Complete the table using tally marks ( ) and the information about the children’s favourite winter sports.
DRAFT
Soccer Rugby Netball Touch football
Mark Nadine Luca Lauren
Jenni Mohammed Sofia Trent
Sally Tom Lola Xavier
Bill Jack Maria Anita
John Emma Amber
Con Harry
12
Answer the questions.
Sport Boys Girls
Soccer Rugby Netball Touch football
a Which sport was the most popular for the boys?
b Which was the most popular sport for the girls?
c How many more boys played soccer than played rugby?
d How many more girls played netball than played touch football?
e If there were 25 children surveyed, how many didn’t have a favourite sport?

Oxford University Press 59 unit COMMUNICATING N L CCT Collects discrete data and constructs graphs using a given scale Interprets data in tables, dot plots and column graphs 14 Tables
10 11
f What is your favourite sport? MP_NSW_SB3_38329_TXT_4PP.indb 59 29-Aug-23 15:22:22
Draw a line to round each number to the nearest 10.

Use the pink number line below to assist you with the next question.
Round each number to the nearest 10. Numbers that end with a 5 are rounded up. a 19 to
to
to
Round these larger numbers to the nearest 10.
a 129 to c 637 to e
to d 251 to f 372 to
Round each number in the addition number sentences to estimate an answer.
a 8 + 21 is about
b 17 + 22 is about
c 36 + 23 is about
Write some numbers and round them to the nearest 10. 5
a 8 b 13 c 18 d 23 e 28 0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30
0 10 20 30 40 50 60 70 80 90 100
g 35
h 44
c 38
e 27 to
to i 89 to b 23 to d 52
f 73 to
to j 99 to
g
221 to
331 to i 789 to b 128
h 549 to j 135 to
1 2
3 4
EXAMPLE 18 + 33
50 Round up to 20 Round down to 30 Oxford University Press 60 unit Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands N WE Rounding
15 MP_NSW_SB3_38329_TXT_4PP.indb 60 29-Aug-23 15:22:23 DRAFT
is about
numbers
4-digit numbers
How many of each piece of Base 10 material would be needed to make the number? Use tally marks to model your answer, e.g. ( = 5).


3746 equals 3 thousands + 7 hundreds + 4 tens + 6 ones.
Thousands Hundreds Tens Ones
a 3764
b 7569
c 3570
d 6964
e 8507
f 2643
Write the number before and after the numbers given.
Expand these numbers on the number expanders. The first one is done for you.
a 3724 thousands hundreds tens ones 3 7 2 4
b 5694 thousands hundreds tens ones
c 8763 thousands hundreds tens ones
d 1144 thousands hundreds tens ones
e 1067 thousands hundreds tens ones
Write the largest number you can using the digits supplied.
Oxford University Press 61 unit Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands COMMUNICATING, REASONING L CCT
15
96 e 2648 b 347 f 7777 c 2641 g 6943
3547 h 8470
a
d
a 3, 5, 6 b 7, 0, 9 c 3, 4, 2 d 1, 3, 6, 5 6 7 8 9
MP_NSW_SB3_38329_TXT_4PP.indb 61 29-Aug-23 15:22:24
DRAFT
Continue the doubling patterns (multiplying by 2). Shoes Tricycles Horses
Bowling pins Continue the halving patterns (dividing by 2). You may need a calculator.

pins Complete the function machines.

Oxford University Press 62 unit Doubling and halving REASONING N L
the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
Represents and uses
15
Pentagons
Eyes Tripods Cows Fingers Bowling
10 11 12 a 2 4 8 b 3 6 c 4 8 d 5 10 20 e 10 20 40 a 128 64 32 b 192 96 48 c 256 128 64 d 320 160 80 e 640 320 160 2, 4, 8 multiply by 2 8, 4, 2, 1 divide by 2 3 a Double plus 1 Rule b +8 Rule 7 1 2 3 4 12 10 8 6 Half plus 1 MP_NSW_SB3_38329_TXT_4PP.indb 62 29-Aug-23 15:22:26 DRAFT
Quarter to and quarter past
15
When the minute hand reaches 3 it is a quarter of the way around the clock face. We call this quarter past (15 minutes past) the hour.
When the minute hand reaches 9 it only has one quarter of the way to go. We call this quarter to (15 minutes to) the hour.
Use the large clock face to help you write the times.
to Quarter past Quarter to past
Draw the times on the clock faces.
10
Each number the hour hand counts is equal to 1 hour (60 minutes).
How many minutes does it take for the hour hand to:
a move from one number to the next?
b move from 12 to 1?
c move from 12 to 2?
How many minutes would it take for the hour hand to move halfway between 12 and 1?


63 unit L
a 11 12 1 2 3 4 5 6 7 8 9 10 b 11 12 1 2 3 4 5 6 7 8 9 10 c 11 12 1 2 3 4 5 6 7 8 9 10 d 11 12 1 2 3 4 5 6 7 8 9 10 7 o’clock Half
Quarter to 8 Quarter past
14
past
4
13 The time is 20 to 2. Minutes to the hour Minutes past the hour 11 12 0 5 10 15 20 25 30 25 20 15 10 5 1 2 3 4 5 6 7 8 9 10 quarter past quarter to a 11 12 1 2 3 4 5 6 7 8 9 10 b 11 12 1 2 3 4 5 6 7 8 9 10 c 11 12 1 2 3 4 5 6 7 8 9 10 o’clock Half past past d 11 12 1 2 3 4 5 6 7 8 9 10 e 11 12 1 2 3 4 5 6 7 8 9 10 f 11 12 1 2 3 4 5 6 7 8 9 10 g 11 12 1 2 3 4 5 6 7 8 9 10
15 16 MP_NSW_SB3_38329_TXT_4PP.indb 63 29-Aug-23 15:22:28
DRAFT
Addition patterns
Adding the same single-digit numbers results in the same digit in the ones position. You can use this knowledge to add larger numbers, e.g.

Oxford University Press 64 unit N CCT 16
and uses mental and written strategies for addition and subtraction
2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Selects
involving
73 + 6 = 57 + 9 = 34 + 7 = 63 + 6 = 67 + 9 = 24 + 7 = 53 + 6 = 77 + 9 = 14 + 7 = Work backwards to find the input numbers on each function machine. 62 72 82 92 a +5 Rule b +8 Rule 25 45 65 85 Solve the addition number puzzles. The first one has been started for you. a 26 23 13 32 39 94 b 32 26 13 25 c 27 37 24 35 2 3
6 + 3 = 9 16 + 3 = 19 26 + 3 = 29 36 + 3 = 39 46 + 3
49 MP_NSW_SB3_38329_TXT_4PP.indb 64 29-Aug-23 15:22:28 DRAFT
=
Revising multiplication facts
Study the arrays, then complete the number sentences.
Draw arrays of your own to model the number sentences, then answer them.
Use the arrays to complete the tables.
Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
Oxford University Press 65 unit
L N 16
a b c d 5 × 2 = 3 × 5
4 × 5 = 5 × 5
e f g h 6 × 2 = 5 × 4 = 6 × 4 = 6 × 5 =
=
=
a 4 × 2 = b 3 × 2 = c 7 × 2 = d 7 × 5 =
a b c 4 5 6 × 2 1 3 5 2 4 7 6 8 9 × 5 1 3 5 2 4 7 6 8 9 × 10 1 3 5 2 4 7 6 8 9 MP_NSW_SB3_38329_TXT_4PP.indb 65 29-Aug-23 15:22:29 DRAFT
Collecting data
School snacks
Sai said that chocolate is the most popular school snack for children under 10.

Predict whether you think this is correct or incorrect.
Survey your class using tally marks to find out the most popular snacks for your class. Each student may have two choices.
a Which snack was most popular?
b Which snack was least popular?
c What is the difference in total between the most popular snack and the least popular snack?
d Were chips more popular than bananas?
e Were sultanas more popular than cupcakes?
Favourite cereals of our class
a What is your favourite cereal?
b What is the favourite cereal of the person sitting closest to you?
c How might you go about finding out the favourite cereal of the whole class?
d Discuss in a group how you might find out your class’s favourite cereal.
e What is your class’s favourite cereal?
Oxford University Press 66 unit COMMUNICATING, REASONING L CCT 16 Collects discrete data and constructs graphs using a given scale Interprets data in tables, dot plots and column graphs
Food Tally Total Food Tally Total
POTATO CHIPS SULTANAS SULTANAS ChocolateBarBAR Other
7 8
MP_NSW_SB3_38329_TXT_4PP.indb 66 29-Aug-23 15:22:30
9
DRAFT
The base unit for measuring mass is the kilogram. The symbol for kilogram is kg.
Many grocery items, such as sugar, are packaged in kilograms. Draw three items that you could buy that are packaged or purchased in kilograms.

Hold a 1 kg mass in one hand and the object, shown in the table below, in the other hand. Estimate whether each object has a mass of less than 1 kg, about 1 kg or more than 1 kg. Shade the appropriate box to record your answer.
Object Less than 1 kg About 1 kg More than 1 kg
Use a balance scale to check if your estimates above were correct.
Solve these problems based on kilograms.
a Jack had a mass of 34 kg but Joe’s mass was 14 kg more. What was Joe’s mass? kg

b Sanjay had a sack of potatoes that had a mass of 60 kg. If he took 25 kg out of the bag, how many kilograms of potatoes were left? kg
c Sally had 6 bags of potatoes. Each bag had a mass of 5 kg. How many kilograms of potatoes did she have? kg
Oxford University Press 67 unit Estimates, measures and compares the masses of objects using kilograms and grams REASONING, COMMUNICATING, PROBLEM SOLVING N
16
The kilogram
Fizzy 1.25 L A4 PAPER
10 11 12 13 MP_NSW_SB3_38329_TXT_4PP.indb 67 29-Aug-23 15:22:31
DRAFT
17 Empty number lines
Rory had to solve this number sentence using an empty number line. This is what he did.
Solve the number sentences by completing the empty number lines. Some help has been given.
had 22 marbles in one bag and 31 in another. How many marbles did she have in total? 22
had 192 pop sticks but gave 33 away. How many did he have left?
Explain another strategy you could use to solve 86 23 =
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

Oxford University Press 68 unit COMMUNICATING N CCT
2 46
69 66 56 46 67 68 69
+ 23 =
a 56 + 23 = 56 66 76 77 78 79 b 43 + 22 = 43 c 49 + 33 = 49 d 86 23 = 86 e There
were
91 f
g Amal
192 1 MP_NSW_SB3_38329_TXT_4PP.indb 68 29-Aug-23 15:22:32
were 91 children at a school, but 32 of them
away. How many children were present?
Kiara
DRAFT
4 rows of 3 = 12
3 columns of 4 = 12
12 shared into 4 rows = 3
12 shared into 3 columns = 4
4 × 3 = 12
3 × 4 = 12
12 ÷ 3 = 4
12 ÷ 4 = 3
DRAFT















4

















Use the pictures to help you write a number sentence to solve each problem.
a There are 16 fish in the rock pool. How many groups of 4 fish can be made?
b James has 12 marbles. If he shares them among himself and three other friends, how many would they each receive?















c 15 girls are shared among five teams. How many girls are in each team?















Oxford University Press 69 unit COMMUNICATING, REASONING CCT N L WE Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values 17
= × = ÷ = ÷ = × = ÷ = ÷
Linking division and multiplication
MP_NSW_SB3_38329_TXT_4PP.indb 69 29-Aug-23 15:22:35
Use the map to answer the questions.
a Start at George’s house and go down Shane St to the corner of South St. Turn left into South St and follow it until it reaches the intersection of Batman Ave. Turn right into Batman Ave and follow it until you are halfway between North Rd and Second Ave.
Whose house did you find?
b Colour the shortest route from the primary school to the swimming pool.
c Whose house is on the corner of Ship Rd and Batman Ave?
d What two streets form part of the boundary of the park?
e Colour a route from the swimming pool to George’s house.
Imagination Island
Interpret the map.
a Can you get to Happy City by road?
b Which town can only be reached by train?
c Which town can only be reached by boat and rail?
d List all the ways you can reach The Palms.
e Is Ban Bay south of Princeville?
Oxford University Press 70 unit Uses grid maps and directional language to locate positions and follow routes COMMUNICATING L
17 Following directions
CATHY HWY BILLY GOAT RD BATMAN AVE SHANE ST LAUREN RD JACK CL JILL CL LONG AVE JENNY RD SHORT ST PITT CR SECOND AVE FIRST AVE SOUTH ST NORTH RD SHIP RD PRIMARY SCHOOL SHOPPING CENTRE MASON TOM BART PARK CAR YARD GEORGE SWIMMING POOL MADONNA
5 Blue Lake Stillwater The Palms Happy City
6 MP_NSW_SB3_38329_TXT_4PP.indb 70 29-Aug-23 15:22:35
DRAFT
Measuring and recording perimeter
Perimeter is the total distance around the edge of a two-dimensional shape. Shapes with straight sides can be measured with a ruler or tape measure.

Measure the dimensions of each shape in centimetres then calculate the perimeter of each one. The first one has been done for you.
Find two shapes in your classroom that could have their perimeters measured in centimetres. Sketch each shape, then record its perimeter.
I found a small book to measure.

Oxford University Press 71 unit COMMUNICATING, REASONING N Measures and estimates lengths in metres, centimetres and millimetres 17
7
8 RED-HOT MATCHES a d P = cm b c e P = cm P = cm P = cm 7 cm 7 cm 2 cm 2 cm Perimeter is equal to 2 cm + 7 cm + 2 cm + 7 cm = 18 cm
BUG BANK 1234 5678 9101 2345 CHARLES PICKWICK STAN’S STAPLES 1000 CONTENTS Sunny SULTANAS a b MP_NSW_SB3_38329_TXT_4PP.indb 71 29-Aug-23 15:22:36 DRAFT
Learning to trade in an addition sum.
Complete the additions, then check them by doing them again.

Add these numbers. Look for combinations that make it easier.
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers

Oxford University Press 72 unit
PROBLEM SOLVING N CCT 18 Trading in addition
3 3 1 + 1 9 5 2 Tens Ones Tens Ones +
a Tens Ones c Tens Ones e Tens Ones g Tens Ones i Tens Ones 2 6 6 7 1 9 1 4 2 8 + 3 5 + 1 8 + 1 7 + 1 7 + 3 3 b Tens Ones d Tens Ones f Tens Ones h Tens Ones j Tens Ones 3 9 2 8 5 5 6 4 2 5 + 1 5 + 1 6 + 1 5 + 2 8 + 4 8
a 4 + 5 + 6 = f 7 + 15 + 5 = k 9 + 7 + 1 + 3 = b 7 + 8 + 2 = g 9 + 4 + 16 = l 8 + 5 + 7 + 2 = c 9 + 6 + 4 = h 17 + 3 + 8 = m 7 + 5 + 3 + 4 = d 7 + 3 + 6 = i 14 + 7 + 6 = n 19 + 6 + 4 + 1 = e 5 + 9 + 5 = j 18 + 9 + 2 = o 25 + 6 + 5 + 4 = 1 2 EXAMPLE
Trade 10 ones for 1 ten. Record 2 in the
1 ten + 3 tens
tens. Trade the 10 ones for 1
MP_NSW_SB3_38329_TXT_4PP.indb 72 29-Aug-23 15:22:38 DRAFT
9 ones plus 3 ones equals 12 ones.
ones column. Add
plus 1 ten equals 5
ten.
3
Complete each pattern.
a 0 2 4 6
b 0 3 6 9
c 0 5 10 15
4
Number patterns 18
d 0 4 8 12
e 0 6 12 18
f 0 7 14 21
Complete each pattern, then write a rule for it.
a 8 12 16 20
b 7 10 13 16
c 18 22 26 30
d 30 35 40 45
5
Make up your own number pattern, then ask a friend to guess its rule.
Use the + function on your calculator to make counting patterns.
a 7 + 4 = = = = = = =
b 8 + 6 = = = = = = =

c 12 + 5 = = = = = = = =
d 6 + 7 = = = = = = = =
e 3 + 9 = = = = = = = =
f 9 + 8 = = = = = = = =
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Oxford University Press 73 unit
PROBLEM SOLVING L N
MP_NSW_SB3_38329_TXT_4PP.indb 73 29-Aug-23 15:22:38
6
DRAFT
Hexagonal prism
Prisms have two parallel faces that are congruent. All other faces on a prism are rectangular if the faces are square to the ends.
Prisms are named by these congruent faces.
DRAFT
A vertex is where two or more lines meet to form an angle.
six other rectangular faces.


I am a prism that has two octagonal faces and eight other rectangular faces.
An edge is where two faces meet or intersect.
COMMUNICATING, REASONING L CCT unit 18 Describing prisms d
MP_NSW_SB3_38329_TXT_4PP.indb 74 29-Aug-23 15:22:39
Small areas are measured using square centimetres. The symbol for square centimetre is cm².

Calculate the area of each shape below by counting the square centimetres.
Draw as many shapes as you can that cover an area of 9 cm2.
Tanya said that there is only one rectangle with an area of 24 cm². Can you prove her wrong by drawing more? They may overlap.
FLUENCY CCT N Oxford University Press 75 Estimates, measures and compares areas using square centimetres and square metres
square centimetre unit 18 COMMUNICATING, PROBLEM SOLVING N
1234 5678 9101 2345 CHARLES PICKWICK
The
BUG BANK
10
9
11 1 cm = 1 cm2 1 cm a b Area = Area = cm² cm²
MP_NSW_SB3_38329_TXT_4PP.indb 75 29-Aug-23 15:22:40
DRAFT
Count forwards by ten.
a 50 60 70
b 32 42 52
Count backwards by ten.
c 80 70 60
d 97 87 77
Solve these subtractions.
a 12 6 = f 14 7 =
b 11 5 = g 47 43 =
c 14 6 = h 38 22 =
d 18 13 = i 57 43 =
e 20 8 = j 38 28 =
Use bridging to 10 to solve the additions.
a 9 + 5 becomes 9 + 1 + =
b 17 + 4 becomes + + =
c 26 + 7 becomes + + =
d 77 + 8 becomes + + =
Write a fraction for the shaded section of each shape.
a b c
Complete the multiplication facts.
a 8 × 3 = d 7 × 5 =
b 6 × 10 = e 6 × 4 =
c 8 × 4 = f 9 × 5 =
Complete the division facts. a
16 ÷ 4 = 18 ÷ 3 =
c Twenty cakes were shared among 5 children. How many did each receive?
Round these numbers to the nearest 10.
a 38 b 21
c 69 d 141
Round these numbers to the nearest 100.
e 396 f 301
g 417 h 579
Oxford University Press 76 76 Diagnostic review 2 PART
1 PART
2
PART
3
PART
4
PART
5
2
PART
d e
b
÷
6 PART
=
7 MP_NSW_SB3_38329_TXT_4PP.indb 76 29-Aug-23 15:22:41 DRAFT
Oxford University Press 77 Diagnostic review 2 PART Write the time each clock is showing. a b 8 PART Write the size of each shape on it, using square centimetres (cm2). 9 PART a Tick all prisms. b Circle all cylinders. 10 PART Draw the lines of symmetry on each shape. 11 PART Name three items that match each heading. Less than 1 kg About 1 kg More than 1 kg 14 PART Shade the word box that best describes the chance of a coin landing on heads. 15 PART Measure the sides and calculate the perimeter of these shapes. a b 13 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 HONEY SULTANAS SULTANAS a b c P = cm P = cm certain equally likely no way unlikely Quarter past a right angle a right angle less than a right angle less than a right angle larger than a right angle larger than a right angle Quarter to 6 Quarter to Half past 3 Draw the times on the clock faces. c d 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 A B PART Shade the word box that best describes each angle. a b 12 MP_NSW_SB3_38329_TXT_4PP.indb 77 29-Aug-23 15:22:41 DRAFT
19
Counting by tens or hundreds
Count forward by tens or hundreds to complete the sequences.
Supply the missing numbers to complete the sequences.
Count backwards by tens or hundreds to complete the sequences.
Write the largest and smallest number you can using the supplied digits.
Think of a number between 1 and 30, then count on by 100 each time.

Oxford University Press 78 N Applies an understanding of place value and the role of zero to
at least tens of thousands
represent numbers to
unit
a 100 110 120 e 230 330 430 b 120 220 320 f 301 401 501 c 205 305 405 g 99 199 299 d 440 450 460 h 58 158 258
a 100 200 500 600 b 85 105 115 145 c 3800 3810 3820 3850 d 3127 3227 3427 3627
a 800 790 780 e 550 450 350 b 920 820 720 f 999 899 799 c 850 750 650 g 4801 4701 4601 d 799 789 779 h 3635 3535 3435
1 2 3 4
5
3 9 5 5 6 1 2 8 3 4 8 6 7 9
MP_NSW_SB3_38329_TXT_4PP.indb 78 29-Aug-23 15:22:42 DRAFT
Digits
Largest Smallest
Solve the problems.
a Mrs Jones bought 7 books at $3 each. How much did she spend?
b Harry had 8 bags of 3 cakes. How many cakes did he have?
c Charlotte had 5 boxes with 3 books in each. How many books did she have?
d Arjun bought 6 books at the shop for $3 each. How much change did he get from $20?
What is the highest number before 50 you can reach if you skip count by 3s?
Oxford University Press 79 L N CCT WE Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values Multiplication facts (3s) unit 19 Write a problem that can be solved using the facts of 3. 10 Use the arrays to solve the multiplication facts. a b c d e 3 × 3 = 2 × 3 = 4 × 3 = 1 × 3 = 5 × 3 = f g h i j 6 × 3 = 8 × 3 = 7 × 3 = 0 × 3 = 9 × 3 = Use your knowledge of multiplication facts to solve the table. × 1 4 10 9 5 7 6 8 3 2 0 a 2 b 4 c 3 d 5
6 7 8
9 MP_NSW_SB3_38329_TXT_4PP.indb 79 29-Aug-23 15:22:42 DRAFT


Oxford University Press 80 unit REASONING L Performs transformations by combining and splitting two-dimensional shapes Reflect, translate and rotate 19 Use a book and demonstrate to a friend how it might be reflected, translated or rotated. 14 c f i Continue the translating pattern. Continue the reflecting pattern. 12 13 Re ect ( ip) Translate (slide) Rotate (turn) MP_NSW_SB3_38329_TXT_4PP.indb 80 29-Aug-23 15:22:44
DRAFT
Draw a line to match each label with a place on the scale from ‘Never’ to ‘Certain’.
The school swimming carnival will be on a Monday.
We will have homework tonight.
A tossed coin lands on heads.
I’ll get to school before my teacher.
A red bead is picked out of a bag of five different colours.
A baby is born a boy.
16
Throwing a double with two dice
a Predict how many times it will take you to throw a double.
b Roll two dice to find out how many times it does take.
c Did everyone get the same result?
Certain Likely
Equally likely
Unlikely
Never
d Colour the label that best describes the chance of throwing a double at first go.
CERTAIN NEVER UNLIKELY PROBABLE
17
The football team is about to get a new uniform. They have a choice of a blue top or a yellow top, and blue, red or yellow shorts.
Sketch how many different combinations of uniforms the team could have.
Oxford University Press 81 unit Records and compares the results of chance experiments 19 Chance—possible outcomes
Blue Yellow
Blue Yellow Red
15
MP_NSW_SB3_38329_TXT_4PP.indb 81 29-Aug-23 15:22:45
DRAFT
Trading in subtraction
Learning to trade in subtraction.
5 ones from 3 ones can’t be done. Trade 1 ten from the tens column to the ones column to make 13 ones. 5 ones from 13 ones equals 8 ones.
Subtract 1 ten from 3 tens to give 2 tens.
Complete
Trade 1 ten for 10 ones.
Tens Ones
Jack’s little sister spilt paint all over his homework. Help Jack to fix his homework.

Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

Oxford University Press 82 unit 20 PROBLEM SOLVING N CCT
−
the subtractions,
them
doing them
a Tens Ones b Tens Ones c Tens Ones d Tens Ones e Tens Ones f Tens Ones 9 4 5 4 4 3 8 3 9 2 5 5 3 8 2 6 3 6 3 5 2 6 3 4 g Tens Ones h Tens Ones i Tens Ones j Tens Ones k Tens Ones l Tens Ones 3 6 2 8 5 7 9 0 7 5 4 0 2 7 1 9 4 8 8 6 6 9 3 7
then check
by
again.
a 5 7 1 7 4 4 b 8 8 2 7 6 5 c 9 5 6 7 6 8 d 7 7 6 7 2 3 e 7 2 6 7 4 8 1 2 4 3 3 1 – 1 5 2 8 Tens Ones EXAMPLE
MP_NSW_SB3_38329_TXT_4PP.indb 82 29-Aug-23 15:22:47 DRAFT
Division from multiplication
Write a division fact from each multiplication fact. The first one has been done for you.

a 6 × 2 = 12 12 ÷ 2 = 6
b 9 × 2 = 18
c 5 × 4 = 20
d 4 × 4 = 16
e 5 × 3 = 15
f 7 × 5 = 35
g 6 × 4 = 24
6 × 5 = 30
=
Division and multiplication are related!
Use a calculator to write some harder multiplication number sentences. Then write a division fact for each, e.g. 9 × 7 = 63 and 63 ÷ 7 = 9.
=
Circle the winning bingo card. a 3 × 4 = i 32 ÷ 4 = b 5 × 4 = j 45 ÷ 5 = c 8 × 2 = k 12 ÷ 6 = d 8 × 4 =
Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
Oxford University Press 83 unit L N CCT 20
÷
÷
=
÷
=
÷
=
÷ =
÷ =
÷ = 3
h
e
m
f 12 ÷ 3
n 9
5
g 30 ÷ 5
o 7 × 6
h 14 ÷ 2
p 9 × 3
5 3 5 20 42 1 4 12 28 3 7 21 32 2 6 12 30 4 7 21 45 2 6 20 27
l 25 ÷ 5
12 ÷ 4 =
7 × 3 =
=
×
=
=
=
=
=
a b c d 4 ×
= ÷ × = = ÷ × = = ÷ × = = ÷ A B C
=
MP_NSW_SB3_38329_TXT_4PP.indb 83 29-Aug-23 15:22:48 DRAFT
An angle is the amount of opening between two arms.
Make an angle tester out of the right-angled corner of a piece of paper. Compare it to the angles above and answer the questions.
a Which angles are right angles?
b Which angles are smaller than right angles?
c Which angles are larger than right angles?
Use the angle tester to find and classify some more angles in your school environment.
Where the angle was found
Draw copies of these angles on the dot paper below. The bottom arm of each angle has been drawn for you.

Oxford University Press 84 unit COMMUNICATING L Angles 20 Identifies angles and classifies them by comparing to a right angle
than
Equal
Larger than
Less
a right angle
to a right angle
a right angle
6 7 8
A B C D E F MP_NSW_SB3_38329_TXT_4PP.indb 84 29-Aug-23 15:22:49 DRAFT
Angle tester
Length can be measured in millimetres. The short way of writing millimetres is mm. There are 10 millimetres in each centimetre.
Use the rulers to measure the length of the objects in millimetres. Remember that 1 centimetre is equal to 10 millimetres.
Draw lines of these lengths. a 50 mm b 80 mm c 120 mm
How many millimetres in:
How many centimetres in:

Oxford University Press 85 unit 20 N Measures and estimates lengths in metres, centimetres and millimetres
Millimetres
a mm b mm c mm d mm 9
b
c
d
cm? e 1
a 2 cm?
3 cm?
10 cm?
9
2 cm?
a
b
c
d
e
10 11 12 STICK STICK GLUE STICK GLUE STICK GLUE STICK STICK MP_NSW_SB3_38329_TXT_4PP.indb 85 29-Aug-23 15:22:50
1 m?
2 m?
8 m?
4 m?
1 2 m?
DRAFT
3-digit addition
Complete these 3-digit additions without trading.
Complete these 3-digit additions with trading in the ones.
Create different addends for each sum.
Write a problem to suit each number sentence.
237 + 123 =
209
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

Oxford University Press 86 unit PROBLEM SOLVING CCT N 21
3 4 2 4 1 2 + 2 5 a Tens Hund Ones 3 4 2 6 2 2 + 4 3 b Tens Hund Ones 4 3 2 1 5 2 + 2 4 c Tens Hund Ones 0 6 2 8 1 2 + 6 2 d Tens Hund Ones 0 7 2 2 3 2 + 4 2 e Tens Hund Ones
3 4 2 2 + 4 8 6 6 2 1 a Tens Hund Ones 0 8 2 7 2 2 + 3 4 b Tens Hund Ones 3 9 2 2 6 2 + 3 7 c Tens Hund Ones 3 5 2 3 7 2 + 2 6 d Tens Hund Ones 2 7 2 0 6 2 + 2 8 e Tens Hund Ones 0 7 2 0 7 2 + 1 7 f Tens Hund Ones 0 9 2 2 2 2 + 5 8 g Tens Hund Ones 3 6 2 3 3 2 + 3 6 h Tens Hund Ones 7 7 2 0 7 2 + 2 7 i Tens Hund Ones 4 8 2 4 3 2 + 3 7 j Tens Hund Ones
+ 3 8 4 a + 4 6 9 b + 6 3 8 c + 7 7 8 d + 6 3 9 e + 5 7 4 f + 8 6 3 g + 4 9 7 h 1 2 3
4
135 +
= a b MP_NSW_SB3_38329_TXT_4PP.indb 86 29-Aug-23 15:22:53
DRAFT
Salespeople often use ‘counting on’ when giving a customer their change. EXAMPLE What change did I get if I bought a pencil for 65 cents and I paid with one dollar?
Pencil 65c + = 70c + = 80c + = $1.00. I received 35c change.
Five people each handed $5 to the salesperson to buy things. Show how you would count out the change by drawing tally marks under each coin you would give the customer.









Total change
a Pencil $3.50 $ .
b Scissors $4.20 $ .
c Eraser $1.40 $ .
d Ruler $2.80 $ .
e Tape $1.75 $ .
DRAFT

Calculate the change that each person would receive.




a Aki handed over $5 when he bought a tin of baked beans costing $3.60.
b Kirsty handed over $4 when she bought a juice box costing $1.80.
c Max handed over $5 when he bought a carton of milk costing $2.70.
d Zeedan handed over $20 when he bought a book costing $18.50.
e Tara handed over $5 when she bought an apple for $0.80.
Strategy
Oxford University Press 87 unit 21 N WE PSC CCT Calculating
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values 65c
change
5 6
Baked Beans MILK BOOK OF BUGS JUICE $1.80 $2.70 80c $18.50 $3.60 $1.80 $2.70 80c $18.50 $3.60 $1.80 $2.70 80c $18.50 $3.60 $1.80 $2.70 80c $18.50 $3.60 MP_NSW_SB3_38329_TXT_4PP.indb 87 29-Aug-23 15:22:57
Evaluating strategies
There are many strategies that you can use to solve addition problems, such as levelling, compensation, bridging to decades and the split strategy.

g a guitar and a skateboard
h two guitars and a set of headphones
i two skateboards and two tennis racquets 125 + 24
A teacher wrote the sum 125 + 24 on the board and asked the children to solve it mentally.
a Write the mental strategy you used to solve it.

b Find another person in the class who solved it in a different way to you and describe their method.
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
Oxford University Press 88 unit
21 PROBLEM SOLVING, REASONING L
8
MP_NSW_SB3_38329_TXT_4PP.indb 88 29-Aug-23 15:22:58
DRAFT
Use a 1 L measuring jug or 1 L milk carton to measure the capacity of these items.
a A kettle
b A large bowl
c A tote tray litres litres litres
Solve the problems.
a Mum put 45 L of petrol in her car and 38 L of petrol in Dad’s car. How many litres was that in total?
b Some campers took an 80 L water container camping.
If they drank 62 L, how much water was left?
How many of each container could be filled from the 40 L oil drum?
DRAFT
Litre containers can be various shapes. Two litre containers have been supplied. Find and sketch some more.
89 Oxford University Press 89 unit Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres 21 REASONING N L CCT WE
Litres
9 10 11 XLT OIL 1 L XLT OIL 4 L XLT OIL 8 L 10 L XLT OIL a b c d
12 1 litre 1 litre MP_NSW_SB3_38329_TXT_4PP.indb 89 29-Aug-23 15:22:59
Trading in 3-digit subtraction
Solve the problems.
a What is the difference in price between Mall Madness and Crazy Car Chase?
c What is the difference in price between Create-a-Word and Amazing Art Drawing Software?
e How much would I pay for two copies of Amazing Art Drawing Software?
b What is the difference in price between Amazing Art Drawing Software and Sonic Penguin?
d How much would I pay for Mall Madness and Sonic Penguin?
f What change would I get from $150 if I bought Crazy Car Chase and Sonic Penguin?
Oxford University Press 90 unit PROBLEM SOLVING CCT N PSC WE 22 Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
7 3 5 5 6 1 2 4 1 3 9 a Tens Hund Ones 6 3 2 4 9 8 8 b Tens Hund Ones 5 4 2 2 8 6 9 c Tens Hund Ones 9 3 2 6 9 4 5 d Tens Hund Ones 6 3 2 4 5 2 7 e Tens Hund Ones 3 2 2 1 7 4 7 f Tens Hund Ones 9 3 2 6 4 2 7 g Tens Hund Ones 9 2 2 1 7 3 4 h Tens Hund Ones 8 3 2 3 9 7 6 i Tens Hund Ones 6 5 2 3 2 1 6 j Tens Hund Ones 7 3 2 4 8 3 5 k Tens Hund Ones 6 2 2 2 4 2 7 l Tens Hund Ones 7 5 2 5 8 6 m Tens Hund Ones 9 3 2 7 4 6 n Tens Hund Ones 6 4 2 2 6 8 o Tens Hund Ones CRAZY CAR PG CHASE $28 MALL ADNESS PG M $37 SONIC PENGUIN PG $49 AMAZING ART DRAWING SOFTWARE $36 CREATE-A-WORD A N U F T O Y S U B S $99 $28 $37 $49 $36 $99
Complete these 3-digit subtractions with trading in the ones.
1 2 MP_NSW_SB3_38329_TXT_4PP.indb 90 29-Aug-23 15:23:02
DRAFT
Fractions of a quantity
Find the fraction of each quantity.
a 1 2 of 8 carrots =
b d 1 2 of 12 apples = 1 5 of 10 squares =
Melinda baked eight cakes.
c 1 4 of 8 triangles =

a Her daughter Alisha ate half of all the cakes. How many was that?
b Her son Zak ate one-quarter of all the cakes. How many was that?
c How many were left for Melinda?
Use division facts to solve the problems.
a How many students went on the music camp if 1 5 of the 30 children in our class went?
Fractions can show part of a quantity. 1 3 of 6 boys = 2 boys 1 5 of 25?
Think 25 ÷ 5 = 5.
b How many points did Ashleigh score if she scored 1 4 of our team’s total of 24?
c When we did our traffic survey we found out that 1 2 of the children walk to school. How many walk if there were 40 children in our survey?







d How old is Elizabeth if she is 1 5 the age of her sister Rachael, who is 15 years old?
Answer the questions.
Oxford University Press unit 22 N CCT WE
Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)
a 1 5 of 10 = b 1 4 of 20 = c 1 3 of 15 = d 1 8 of 24 = 3 4 5 6
MP_NSW_SB3_38329_TXT_4PP.indb 91 29-Aug-23 15:23:05
DRAFT
Turns
A turn is the amount of rotation around a centre point. Some common turns are 1 4 turns, 1 2 turns, 3 4 turns and a full rotation of the compass (clockwise). This shape has been rotated around a full turn.
Write the amount of turn for each shape: 1 4
Start Turns
1 (full rotation).
Start Turns

Oxford University Press 92 unit 22 COMMUNICATING, REASONING N
Performs transformations by combining and splitting two-dimensional shapes Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)
, 1 2 , 3 4 ,
7 N S E W 1 4 1 2 3 4 start 1 4 turn 1 2 turn 3 4 turn 1 full rotation
or
a g b h c i d j e k f l MP_NSW_SB3_38329_TXT_4PP.indb 92 29-Aug-23 15:23:06
DRAFT
Time intervals
The hands on a clock tell us a lot of information.
The hour markers on the clock tell us the hour, but also count off lots of 5 minutes or 5 seconds. This clock shows: 3 the hour shown by the hour hand
5 minutes past the hour, shown by the minute hand
25 seconds counted off by the second hand The time on the clock is 5 minutes past 3. We do not usually include the seconds unless an extremely accurate time is needed.
On the watches below, draw a minute hand to complete the time.
DRAFT
On the watches below, add a second hand to show the seconds.
Twelve ten and twenty seconds
Twelve twenty-five and five seconds
Twelve twenty and fifteen seconds
Ten twenty and thirty-five seconds
Estimate a length of time for each activity (for example, 20 minutes).

Activity
a Play half a game of football
b Sneeze
c Lunchtime at school
d Read a small book
e Wash the car
f Brush my teeth
Time units
93 Oxford University Press 93 unit 22 L Represents and interprets analog and digital time in hours, minutes and seconds
8
9
10 0 30 25 20 50 55 5 10 15 45 40 35 60 a b c d 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 20 past 3 a quarter past 12 10 past 9 20 to 7 a b c d 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7 12 6 9 3 1 11 2 10 4 8 5 7
MP_NSW_SB3_38329_TXT_4PP.indb 93 29-Aug-23 15:23:07
Do you know your tables? Your teacher will give you 35 seconds to complete each table.
What happens when you multiply a number by 10?

a 5 books = $
b 6 pens = $
c 10 glue sticks = $
d 3 calculators = $
e 4 staplers = $
4 books and a stapler = $
6 glue sticks and a calculator = $
8 pens and a book = $
6 calculators and a stapler = $
2 books and 7 pens = $
Can you say your 2, 3, 4, 5 or 10 times tables in less than 30 seconds? Work with a partner to find out.
Oxford University Press 94 unit Multiplication facts/revision N CCT WE Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems 23
3
Table of fives 0 × 5 = 2 × 5 = 4 × 5 = 3 × 5 = 5 × 5 = 1 × 5 = 6 × 5 = 9 × 5 = 7 × 5 = 8 × 5 = 10 × 5 = b Table of twos 0 × 2 = 2 × 2 = 4 × 2 = 3 × 2 = 5 × 2 = 1 × 2 = 6 × 2 = 9 × 2 = 7 × 2 = 8 × 2 = 10 × 2 =
Table of tens 0 × 10 = 2 × 10 = 4 × 10 = 3 × 10 = 5 × 10 = 1 × 10 = 6 × 10 = 9 × 10 = 7 × 10 = 8 × 10 = 10 × 10 = d Table of noughts 0 × 0 = 2 × 0 = 4 × 0 = 3 × 0 = 5 × 0 = 1 × 0 = 6 × 0 = 9 × 0 = 7 × 0 = 8 × 0 = 10 × 0 = e Table of fours 0 × 4 = 2 × 4 = 4 × 4 = 3 × 4 = 5 × 4 = 1 × 4 = 6 × 4 = 9 × 4 = 7 × 4 = 8 × 4 = 10 × 4 =
0 × 1 = 2 × 1 = 4 × 1 = 3 × 1 = 5 × 1 = 1 × 1 = 6 × 1 = 9 × 1 = 7 × 1 = 8 × 1 = 10 × 1 = Find the cost.
a
c
f Table of ones
f
g
h
i
j
1 2 BOOK OF BUGS $4 1 ON OFF 2 3 0 + + =% C / 4 5 6 7 8 9 GLUESTICK GLUESTICK GLUESTICK GLUESTICK GLUESTICK GLUESTICK $10 $1 $2
MP_NSW_SB3_38329_TXT_4PP.indb 94 29-Aug-23 15:23:08
DRAFT
Sometimes it’s easier to solve additions if you do levelling. In other words, you balance both numbers to more manageable numbers. For example, 38 + 36 can be viewed as 40 + 34 = 74.
Add 2 Subtract 2
Solve these additions using levelling.
a 68 + 25 becomes 70 + 23 =
b 37 + 46 becomes + =
c 49 + 38 becomes + =
d 66 + 34 becomes + =
e 78 + 36 becomes + =
f 86 + 23 becomes + =
g 117 + 26 becomes + =
h 128 + 42 becomes + =
i 139 + 36 becomes + =
j 148 + 37 becomes + =
A subtraction method is to apply the constant difference strategy. Again, you need to balance both numbers.

For example, 84 – 27 can be viewed as 87 – 30 = 57
Add 3 Add 3
Solve the subtractions using the constant difference strategy.

a 83 – 28 becomes 85 – 30 =
b 76 – 37 becomes – =
c 93 – 47 becomes – =
d 146 – 38 becomes – =


e 173 – 57 becomes – =
f 127 – 38 becomes – =
136 + 38 becomes 140 + 34 = 174
Much easier!
184 – 26 becomes 188 – 30
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
95 unit Levelling and constant difference
N PSC WE 23
4
5
4 4
MP_NSW_SB3_38329_TXT_4PP.indb 95 29-Aug-23 15:23:10 DRAFT
× =
? Think 4 + 4 + 4 + 4 = 16
I found block 2 at grid reference E3. 2
Sam put all his letter, number and shape blocks into a stack. He then labelled them across from A to G and up from 1 to 5.
Find the blocks from the grid references. Remember to read ACROSS before UP. The first one is done for you.
Give grid references for these blocks.
Draw on the blocks on the grid at the top of the page.
a Draw a hand on the block with a grid reference of B4.
b Draw a face on the block G1.

c Put a cross on the block C5.
d Colour the block D4.
Find the secret word by writing the letters found at each grid reference.
Oxford University Press 96 unit COMMUNICATING L 23 Uses grid maps and directional language to locate positions and follow routes Grids 5 4 3 2 1 A B C D E F G
a B3 = c C3 = e E2 = g G4 = i A5
b B2 = d E5 = f F1 = h A3 = j D2 =
=
a
b
c = d
e
=
=
=
=
G2 C2 E5 G4 A4 F1 6 7 8 9
MP_NSW_SB3_38329_TXT_4PP.indb 96 29-Aug-23 15:23:11
DRAFT
Favourite T-shirt colours
Every student in Mrs Cook’s class drew a picture of their favourite coloured T-shirt. They drew the first letter of the colour on the drawing of their T-shirt before pinning their drawings up on the wall.
Represent each coloured T-shirt using tally marks. Red White Blue Pink
Answer the questions.
a Which was the most popular coloured T-shirt?
b Which T-shirt colour had a tally of 4?
c Which was the least popular colour of T-shirt?
d How many more children like blue than like pink?
e What coloured T-shirt do you like the most?
f Carrie said that all girls like blue T-shirts the best. If there are 15 girls in Mrs Cook’s class, do you think she was correct?
Use your tally to make a column graph of the T-shirt colours. Colour one square on the graph for each T-shirt.
Favourite T-shirts
97 Oxford University Press 97 unit 23 COMMUNICATING L CCT Collects discrete data and constructs graphs using a given scale Interprets data in tables, dot plots and column graphs
Collecting data
Yellow Orange
10
12
T-shirt
Number
1 2 3 4 5 6 7 8
colours
of T-shirts
11 MP_NSW_SB3_38329_TXT_4PP.indb 97 29-Aug-23 15:23:11
DRAFT
Compensation strategy
The compensation strategy involves rounding one of the numbers so that you can work out the answer in your head. Later you have to take off the amount you rounded, e.g. 54 + 38 =
Think 54 + 40 = 94, then take off the 2 that was rounded up. The answer is 92.

Use this strategy to complete these additions. The first one has been done for you.
a 26 + 38 becomes 26 + 40 2 = 64
b 34 + 27 becomes + =
c 28 + 39 becomes + =
d 46 + 28 becomes +
e 27 + 49 becomes +
f 35 + 48 becomes +
g 56 + 27 becomes +
h 63 + 28 becomes +
i 47 + 49 becomes +
j 76 + 37 becomes +
Mario has completed these operations on a calculator but has made some mistakes. Use your estimation skills to decide which ones he should do again.
Question Good Do again
Question Good
a 38 + 43 = 81 h 71 + 28 = 80
b 27 + 61 = 88 i 52 − 27 = 35
c 74 − 37 = 57 j 68 + 24 = 92

d 49 + 33 = 62
e 92 − 56 = 13
f 62 + 27 = 89
g 39 − 22 = 37
k 96 − 38 = 48
l 82 − 39 = 43
m 48 − 33 = 15
n 93 − 37 = 76
Oxford University Press 98 unit PROBLEM SOLVING, COMMUNICATING, REASONING N CCT 24 Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
=
=
=
=
=
=
=
1 2
MP_NSW_SB3_38329_TXT_4PP.indb 98 29-Aug-23 15:23:12
When adding 56 + 39, think 56 + 40 – 1 = 95.
DRAFT
Division facts and strategies
Use known facts or the arrays to answer the questions.
Use known facts to solve the problems.
a 10 marbles shared among 5 boys.
b 15 football cards shared among 3 girls.
c $40 shared among 10 sisters.

d 9 cows shared among 3 paddocks.
e $24 shared among 4 children.
f 32 eggs shared among 4 people.
g 40 chickens shared among 4 families.
Solve the secret code by exchanging numerals for letters.
Oxford University Press 99 unit 24 REASONING, COMMUNICATING N CCT WE Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems
a 24 ÷ 4 = d 20 ÷ 4 = g 20 ÷ 5 = b 24 ÷ 6 = e 20 ÷ 10 = h 30 ÷ 6 = c 20 ÷ 2 = f 30 ÷ 5 =
3
2 4 8 6 10 3 9 7 1 5 K A G W D T R O U E 1 16 ÷ 2 = 6 12 ÷ 2 = 11 5 ÷ 5 = 2 18 ÷ 2 = 7 14 ÷ 2 = 12 30 ÷ 3 = 3 15 ÷ 3 = 8 90 ÷ 10 = 13 25 ÷ 5 = 4 16 ÷ 4 = 9 8 ÷ 4 = 5 9 ÷ 3 = 10 40 ÷ 4 = 1 2 3 4 5 6 7 8 9 10 11 12 13 4 5
MP_NSW_SB3_38329_TXT_4PP.indb 99 29-Aug-23 15:23:13 DRAFT
Halve, then halve again to divide by 4.
Testing predictions
Predict answers to the following questions.
Prediction
What will the colour of the next car to pass our school be?
What will the most popular colour car to pass our school be?
Survey
With your teacher, conduct a survey of the colours of cars passing, or parked in or around, your school. Complete the survey details on the grid. Fifty cars will be enough. Use tally marks to record your survey information on this grid.

Silver Black White
Construct a dot plot to record your results. The vertical axis shows how many cars. The horizontal axis shows what colour car.
Silver Black White Other
What colour was the next car to pass the school?
What was the most popular colour car to pass the school?
Oxford University Press 100 unit COMMUNICATING, REASONING L CCT 24 Collects discrete data and constructs graphs using a given scale Interprets data in tables, dot plots and column graphs
6 7 8 9 10
MP_NSW_SB3_38329_TXT_4PP.indb 100 29-Aug-23 15:23:14
I think red is the most popular.
DRAFT
Fruit and vegetables are often sold in kilogram lots. Record the mass of each purchase.
Look carefully at the scales in the picture. What can be learnt from them?

101 Oxford University Press 101 unit 24 REASONING Estimates,
and
measures and compares the masses of objects using kilograms
grams Scales
a 0 4 1 7 2 6 3 5 Kilograms b 0 4 1 7 2 6 3 5 Kilograms c POTATOES 0 4 1 7 2 6 3 5 Kilograms d kg
to
0 to 1 kg 1 to 2
2 to 3
4 to 5
Dictionary Dictionary A4 PAPER
kg kg kg Use a pan balance
find the mass of these items.
kg
kg
kg
11 12 13 SUGAR RICE TOMATOES 0 4 1 7 2 6 3 5 Kilograms MP_NSW_SB3_38329_TXT_4PP.indb 101 29-Aug-23 15:23:16
DRAFT
Solve the problems.
a Julia travelled from The Ship to Roller Coaster. If she passed through Bumper Cars, how far did she travel?
b Marco travelled from The Ship to Ferris Wheel. If he passed through Gravitron, Carousel and The Tower, how far did he travel?
c Write another problem based on this map.
Use any strategy you wish to solve the problems. Problem
a Our hens laid 80 eggs on Saturday and 65 on Sunday. How many eggs did they lay over the weekend?
b Farmer Green sheared 43 sheep on Monday, 44 on Tuesday and 42 on Wednesday. How many sheep has he shorn?
c If 75 cows were milked this morning and 75 will be milked this afternoon, how many will be milked today?
d The water tank has 123 L of water. If a further 47 L of water are added, how much water will there be in the tank?
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
102 unit Oxford University Press 25
PROBLEM SOLVING N CCT
Amusement Island The Ship 7 km 7 km 4 km 4 km 8 km 8 km 6km 6km 9 km 6km 5km 5 km 5 km Bumper Cars Roller Coaster Circus The Tower Carousel Gravitron Water Ride Ferris Wheel Swing Ride
Addition problems
1
out
Working
2 MP_NSW_SB3_38329_TXT_4PP.indb 102 29-Aug-23 15:23:17
DRAFT
Fractions on a number line
Draw lines to place the fractions in the correct positions on the number line.
James and Jacinta were told to fold their paper strips into fifths.
a Explain why James has done it incorrectly.
b Jacinta said that 1 5 is a bigger fraction than 1 10 . Explain why.
Shade the fractions of each bar.
Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)
103 unit Oxford University Press
25
a 1 –2 1 –8 3 –8 6 –8 7 –8 1 –4 4 –8 3 –4 0 1 b 1 –2 1 –10 2 –5 6 –10 4 –5 4 –10 5 –10 3 –5 0 1
Jacinta James
a 3 4 0 1 b 3 8 0 1 c 1 3 0 1 d 7 10 0 1 e 1 5 0 1 3 4 5 MP_NSW_SB3_38329_TXT_4PP.indb 103 29-Aug-23 15:23:18 DRAFT
Polygons – trapeziums, rhombuses and kites
Polygons are two-dimensional shapes with three or more straight sides. Examples include triangles, quadrilaterals, pentagons and hexagons. A few trickier polygons are mentioned below.
A trapezium is a four-sided polygon with one set of parallel sides.

A rhombus is a polygon that has four equal sides with the opposite angles being equal.
A kite is a four-sided polygon that has two pairs of equal sides. None of the sides are parallel to other sides.
Classify the shapes below by writing R for rhombus, T for trapezium, or K for kite on them.
Compares two-dimensional shapes and describes their features
104 unit Oxford University Press
COMMUNICATING, REASONING CCT
25
6
a b c d e i f j g k l m n h MP_NSW_SB3_38329_TXT_4PP.indb 104 29-Aug-23 15:23:18 DRAFT
Square centimetres are not suitable for measuring large areas.
Large areas such as the area of a room are measured using square metres.
The symbol for square metre is m²
Make your own square metre.
Using newspaper, stick pieces together to make a square that is one metre wide and one metre long.

Use your square metre to measure the area of these places. Make an estimate first. Place Estimate Area
a The area of a large table square metres square metres

b The area of a handball court square metres square metres
c The area of a small room square metres square metres
d The area of a square drawn on the playground with 4 m sides square metres square metres
8 9 10
Look carefully at the shape above. It is 2 m long and 1 2 m wide. Does it take up a square metre of space?
Explain why.
105 unit Oxford University Press Estimates, measures and compares areas using square centimetres and square metres
COMMUNICATING, PROBLEM SOLVING N 25
The square metre
7
1
1
1 m = 1 m2 1 m
m
m
2 m 1 2 m
MP_NSW_SB3_38329_TXT_4PP.indb 105 29-Aug-23 15:23:19
DRAFT
3-digit subtraction (trading)
Complete the 3-digit subtraction algorithms with trading in the tens.


Find the missing digits in each subtraction.


DRAFT
Four children have each been saving to buy the Racer.
Special $469
Racer
Calculate how much more each person needs to save before they can buy the car.
Reuben has saved $137.
has saved $318.
has saved $267.
Erin has saved $375.
a Hund Tens Ones b Hund Tens Ones c Hund Tens Ones d Hund Tens Ones e Hund Tens Ones 8 9 1 2 7 6 1 6 8 4 7 4 2 1 5 3 3 5 3 3 4 4 2 6 5 4 3 4 0 2 5 1 3 9 4 f Hund Tens Ones g Hund Tens Ones h Hund Tens Ones i Hund Tens Ones j Hund Tens Ones 6 2 9 8 1 6 7 3 9 8 4 4 6 5 5 3 4 3 7 4 5 2 6 7 7 5 3 2 6 3
a Hund Tens Ones b Hund Tens Ones c Hund Tens Ones d Hund Tens Ones e Hund Tens Ones 9 6 7 6 7 8 8 7 5 2 9 7 2 4 2 2 5 3 2 4 1 5 6 2 3 2 2 8 6 3 9 4
1 2
b
c
3
a
Fran
Li
d
Full remote control Powerful motor The coolest model car around! 106 unit Oxford University Press 26 Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values PROBLEM SOLVING N CCT PSC WE
MP_NSW_SB3_38329_TXT_4PP.indb 106 29-Aug-23 15:23:21
4-digit numbers

The 4 in 4527 is worth a lot more than the 4 in 5436.
d 6740 = + + + e 8407 = + + +
f 9936 = + + +
6 7

Place each set of numbers in descending order (highest to lowest).
a 8507, 7503, 5073, 3057
b 2645, 3658, 1999, 2500
c 2907, 8436, 3541, 2657
d 3524, 5234, 2453, 4532
e 837, 238, 1438, 2745
107 unit Oxford University Press 26 COMMUNICATING, REASONING N L
these numbers
the numeral expanders. The first one is done for
a 2534 4 3 5 2 thousands hundreds tens ones ones thousands hundreds tens b 1367 4 3 5 2 thousands hundreds tens ones ones thousands hundreds tens c 2456 4 3 5 2 thousands hundreds tens ones ones thousands hundreds tens d 3721 4 3 5 2 thousands hundreds tens ones ones thousands hundreds tens e 4699 4 3 5 2 thousands hundreds tens ones ones thousands hundreds tens f 6391 4 3 5 2 thousands hundreds tens ones ones thousands hundreds tens
the place value of the numbers in bold. The first one is done for you. a 4678 tens d 3930 g 4544 b 3294 e 1597 h 2825 c 2128 f 2716 i 3089 4 5 Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands 63 hundreds and 91 ones is another way I could write 6391.
Expand
on
you.
Record
Expand each number. The first one has been done for you.
=
a 4527
4000 + 500 + 20 + 7
b 5436 = + + + c 6748 = + + +
MP_NSW_SB3_38329_TXT_4PP.indb 107 29-Aug-23 15:23:23
DRAFT
Octa means eight. An octagon is a shape with eight sides.


A regular octagon has all its sides equal in length and all its angles equal. Regular octagon Irregular octagon
Carefully count and then record the number of sides and angles on each shape. Write the name of each shape on the shape.
Join the dots to form the shapes, then write their names on them.
Answer the questions.
a How many sides on two pentagons and three octagons?
b How many sides on two hexagons and five triangles?
c How many sides on five squares and two octagons?
108 unit Oxford University Press
REASONING L N Octagons 26 Shape Sides Angles a b c Shape Sides Angles d e f
Compares two-dimensional shapes and describes their features
a 4 5 6 7 8 1 2 3 c e 1 2 3 4 5 6 7 8 b 4 5 6 1 2 3 d f 1 2 3 4 5 6
9 4 3 2 1 8 7 6 5 10
8 1 2 3 4 5
MP_NSW_SB3_38329_TXT_4PP.indb 108 29-Aug-23 15:23:24
DRAFT
Cubic centimetres

Jack cut the front off two identical boxes then filled one with centicubes and the other with marbles.
a Which unit do you think was better for measuring the volume of the boxes?
b Explain why.
A standard unit for measuring volume is the cubic centimetre The abbreviation for cubic centimetre is cm³. A cubic centimetre is a cube that has 1 cm sides.
Make these models from centicubes or Base 10 ones, then record their volumes by counting the number of cubes used in their construction.
Ava made this object with a volume of 8 cubic centimetres. Make another object with a volume of 8 cubic centimetres.
109 unit Oxford University Press Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres
COMMUNICATING, REASONING L CCT 26
11
cubic
cubic centimetres
cubic centimetres cubic centimetres
cubic
a b c d e f g h
cubic centimetres
centimetres
cubic centimetres
cubic centimetres
centimetres
12
1 cm3
13 MP_NSW_SB3_38329_TXT_4PP.indb 109 29-Aug-23 15:23:26
DRAFT
Multiplication mental strategies
When multiplying a one-digit number by a multiple of ten, we can use the strategy of repeated addition.
Use repeated addition to multiply the numbers. The number line above may assist you.
We can also use place value strategies to multiply by a multiple of 10. E.g. 4 × 20 becomes 4 × 2 tens = 8 tens = 80.
Use this strategy to multiply the numbers below.
Write as many multiplication number sentences as you can to create 120. 3


unit Represents and
the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
uses
N L CCT 27
a 3 × 20 = g 2 × 30 = b 5 × 20 = h 5 × 30 = c 6 × 20 = i 6 × 30 = d 8 × 20 = j 3 × 40 = e 3 × 30 = k 5 × 40 = f 4 × 30 = l 3 × 50 = 1
a 2 × 50 = e 9 × 20 = b 5 × 50 = f 6 × 50 = c 4 × 50 = g 4 × 60 = d 7 × 20 = h 5 × 60 = 2
4 × 20
0 30 50 70 90 10 20 40 60 80 100 110 0 30 50 70 90 110 130 150 170 190 10 20 40 60 80 100 120 140 160 180 200
E.g.
becomes 20 + 20 + 20 + 20 = 80
MP_NSW_SB3_38329_TXT_4PP.indb 110 29-Aug-23 15:23:27 DRAFT
a How many groups of 5?
b How many groups of 3?
a How many groups of 4?
b How many groups of 5?
c How many groups of 10?
d How many groups of 2?
Another method of solving division is to use known table facts, e.g. 20 ÷ 5. Think 4 × 5 = 20, so the answer is 4.
7

At the start of the year Mrs Miller put 32 children into 4 sports teams. How many children in each team?

111 unit Oxford University Press Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values 27 Division COMMUNICATING L Use the pictures to solve the problems. 4 5
Discuss in a group how you could solve this problem, then solve it.
MP_NSW_SB3_38329_TXT_4PP.indb 111 29-Aug-23 15:23:29 DRAFT
When giving grid references, always read the horizontal axis first (A to I) then the vertical axis (1 to 3). Arun’s house can be found at E1.
The closest bus stop to Jill’s house is on the intersection of Hope St and Collins St.
Use compass points to answer the questions.

a Is the school to the west of Green Park?
b What direction would Ben travel along Brown St to go to Sam’s house?
c What direction would Jill travel along Green St to go to Green Park?
112 unit Oxford University Press Uses grid maps and directional language to locate positions and follow routes 27 Interpreting maps/grid references COMMUNICATING L
10 N W E S Bill Ave Brown St Victoria St A 1 2 3 B C D E F G H I Yuk St Collins St Green St Phillip St SHOPPING CENTRE CAR PARK SMITH PARK GREEN PARK Milk Rd Jill St Hook Rd Hope St Hoot St Dolphin St Red St School Rd SCHOOL Jill Sam Sue Arun Ben Mia Joe Con Than MP_NSW_SB3_38329_TXT_4PP.indb 112 29-Aug-23 15:23:29
DRAFT
Jimmy has a bag of 20 marbles.
a How many of the marbles are red?
b How many of the marbles are blue?
c How many of the marbles are pink?
d How many of the marbles are green?
e How many of the marbles are yellow?
Use the data you have collected above to answer the questions.
a Is it more likely that a red marble will be pulled out of the bag than a green one?
b Is it less likely that a green marble will be pulled out of the bag than a yellow one?
c Is it more likely that a green marble will be pulled out of the bag than a pink one?
Mr Brown placed 12 coloured marbles in a bag. He asked each child to select one marble at a time and then to put it back into the bag. Colour the label which best describes the chance of pulling out each colour.
There are 10 counters in a box. They are made up of three different colours. The counter that is most likely to be drawn out of the box is green, the least likely colour is red and the colour which has a slightly better chance than red is blue.
Colour the counters to display these chances.
113 unit Oxford University Press Records and compares the results of chance experiments 27 Chance—likelihood COMMUNICATING CCT
11 12 13
14 a b c d Likely Unlikely Maybe Never Likely Unlikely Maybe Never Likely Unlikely Maybe Never Likely Unlikely Maybe Never Red Yellow Green Orange R R R R R R R R R R G G G G P P P B B Y MP_NSW_SB3_38329_TXT_4PP.indb 113 29-Aug-23 15:23:30 DRAFT
Solve the following.
Solve the additions using the compensation strategy or any other strategy.
e 46 + 28 =
f 34 + 39 =
g 27 + 49 =
h 54 + 29 =
i 27 + 37 =
Answer the multiplications.
k I bought 6 packets of nails. If there are 5 nails in each packet, how many nails did I buy?
Oxford University Press 114 114 Diagnostic review 3 PART
a Hund Tens Ones c Hund Tens Ones 2 3 4 3 4 8
1 2 5
Hund Tens Ones d Hund Tens Ones 7 5 9 7 8 2 3 4 7 5 1 5
+ 7 5 3 +
b
1 PART
a 3 × 5 = f 3 × 3 = b 7 × 5
g 6 × 5 = c 6 × 4 = h 9 × 3 = d 8 × 4 = i 7 × 10 =
j 6 × 3 =
=
e 9 × 5 =
2 PART
the
a 10 ÷ 2 = b 12 ÷ 3 = c 16 ÷ 4 = d 20 ÷ 5 = e 25 ÷ 5 = 3 PART
these numbers. a 6274 b 3505
the number before and the number
c 3624 d 2073 e 7988 4 PART
the counts. a 80 90 100 b 205 215 225 c 2315 2415 2515 d 3550 4550 5550 5 PART
the fractions. 6 a 1 2 of 24 = b 1 3 of 24 = c 1 4 of 24 = MP_NSW_SB3_38329_TXT_4PP.indb 114 29-Aug-23 15:23:31 DRAFT
Complete
division facts.
Expand
Write
after.
Complete
Find
Oxford University Press 115 Diagnostic review 3 PART a Draw this shape after making a 1 4 turn clockwise. 7 PART Colour the trapeziums red, the rhombuses blue, and the kites green. 9 PART Measure the length and width of this shape in millimetres. a Length = mm b Width = mm 10 PART Draw the shapes found at the grid references. a A2 b D1 c C3 d B4 8 4 3 2 1 A B C D PART Record the volume of each model. 11 PART Tick the box that best describes the chance of two sixes being thrown. 12 Likely Unlikely Maybe Never turn turn 1 4 b Draw this shape after it has made a 1 2 turn. 1 2 x x y y x x y y a b c Cubic centimetres Cubic centimetres Cubic centimetres MP_NSW_SB3_38329_TXT_4PP.indb 115 29-Aug-23 15:23:31 DRAFT
Check Kuljit’s subtractions by repeating them yourself.
2
What did Kuljit forget to do in question 1b above?
Amelia did four calculations on her calculator and has given us her answers. Use a calculator to check, then mark her work by giving her a tick or a cross in each box.
a 6 books cost $46
b A watch and a skateboard cost $60
c 7 calculators cost $105
d 5 watches cost $150
Jarrah has done five subtractions. His teacher asked him to check them by doing additions. He has done the first one, can you do the rest?
116 unit Oxford University Press
and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values 28 Checking
PROBLEM SOLVING, COMMUNICATING, REASONING N CCT
Selects
work
a 5 4 2 3 3 1 Tens Ones b 9 5 3 8 Tens Ones 6 7 1 c 8 7 4 9 3 8 Tens Ones 1 7 d 6 2 3 8 2 4 1 5 Tens Ones e 9 4 7 7 1 7 Tens Ones 1 8 5 4 2 3 Tens Ones 9 5 3 8 Tens Ones 8 7 4 9 Tens Ones 6 2 3 8 Tens Ones 9 4 7 7 Tens Ones 1
WORLD OF DOGS 1 ON OFF 2 3 0 + +% C / 4 5 6 7 8 9
a 7 5 2 3 5 2 ✓ Tens Ones b 9 7 3 3 6 4 Tens Ones c 8 8 3 5 5 3 Tens Ones d 6 3 2 6 5 7 1 6 Tens Ones e 8 4 4 7 3 7 1 7 Tens Ones 5 2 2 + 3 7 5 Tens Ones 6 4 3 + 3 Tens Ones 3 + 5 Tens Ones 2 + 6 Tens Ones 4 + 7 Tens Ones 3 4 12 10 7 5 1 11 10 12 3 8 7 5 9 2 10 $8 $25 $15 $35 MP_NSW_SB3_38329_TXT_4PP.indb 116 29-Aug-23 15:23:34
DRAFT
5
When numbers are added it doesn’t matter in which order they are added, e.g. 4 + 5 = 9, 5 + 4 = 9.
Add the following numbers to see if this is true.
a 6 + 4 = 4 + 6 =
b 7 + 6 = 6 + 7 =
c 9 + 7 = 7 + 9 =
d 10 + 12 = 12 + 10 =
e 16 + 4 = 4 + 16 =
f 18 + 5 = 5 + 18 =
g 16 + 8 = 8 + 16 =
6
Enter some large numbers on your calculator to see if the order they are added in makes a difference.
7 8 9

For each fact given, write another multiplication fact using the same numbers. The first one is done for you.


Can numbers be multiplied in any order?
What did you notice?
Solve the problem.
Mitchell hit six fours and then was bowled out. Georgia hit four sixes and then was caught out. Who scored more runs: Mitchell or Georgia?
117 unit Oxford University Press Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values 28 Commutative property COMMUNICATING, REASONING N
a 3 × 4
12 4 × 3 = 12 b 5 × 2 = × = 10 c 7 × 2 = × = 14 d 6 × 5 = × = 30 e 6 × 3 = × = 18
=
MP_NSW_SB3_38329_TXT_4PP.indb 117 29-Aug-23 15:23:35 DRAFT
Drawing objects 28
Sketch each 3D object. Try to show how long it is, how wide it is and how tall it is.
Below are two drawings of a square pyramid from different views. Sketch the pyramids with the help that has been given, then draw them by yourself.
Model object
With some help
My drawing
118 unit Oxford University Press
COMMUNICATING, REASONING CCT
Makes and sketches models and nets of three-dimensional objects including prisms and pyramids
a
b c d
10 11
A
Z H N
BAR
Baked Beans
B C E G U
ChocolateBar
MP_NSW_SB3_38329_TXT_4PP.indb 118 29-Aug-23 15:23:36
Perfume
DRAFT
Kilograms
Many items are packaged in half-kilogram packages. Half a kilogram is 500 grams.

Fruit and veg. Estimate Amount
Apples
Oranges
Bananas
Tomatoes
How many of each item could be packed in a bag that holds 24 kg?
119 unit Oxford University Press Estimates, measures and compares the masses of objects using kilograms and grams
COMMUNICATING, PROBLEM SOLVING, REASONING N CCT 28
14 Flour 2 kg STICKY TAPE 3 kg NAILS 4 kg a b c d e Dictionary
1KG MP_NSW_SB3_38329_TXT_4PP.indb 119 29-Aug-23 15:23:37
DRAFT
3-digit addition (trading) 29
Complete these additions with trading in the tens. The first one is done for you.
Complete these additions with trading in the tens and ones.
Calculate the cost of the following items.
120 unit Oxford University Press
numbers PROBLEM SOLVING N CCT PSC WE
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit
4 7 9 3 1 + 2 1 3 6 8 a Tens Hund Ones 3 7 2 8 6 + 1 2 b Tens Hund Ones 5 6 2 7 4 + 1 3 c Tens Hund Ones 4 4 2 6 3 + 2 4 d Tens Hund Ones 3 2 2 9 4 + 4 2 e Tens Hund Ones 4 3 2 7 3 + 2 1 f Tens Hund Ones 7 4 2 9 6 + 2 4 g Tens Hund Ones 3 3 2 9 2 + 3 3 h Tens Hund Ones 3 5 2 8 4 + 3 2 i Tens Hund Ones 7 4 2 8 2 + 3 2 j Tens Hund Ones
6 5 2 6 3 + 3 5 a Tens Hund Ones 3 8 2 9 4 + 2 8 b Tens Hund Ones 8 6 2 8 6 + 1 7 c Tens Hund Ones 3 4 2 9 2 + 4 7 d Tens Hund Ones 7 3 2 5 3 + 4 8 e Tens Hund Ones 3 9 2 9 1 + 2 7 f Tens Hund Ones 8 7 2 8 2 + 3 9 g Tens Hund Ones 5 7 2 8 3 + 9 h Tens Hund Ones 7 4 2 8 3 + 4 9 i Tens Hund Ones 7 8 2 8 + 3 4 j Tens Hund Ones
1 2 3 Kola POTATO CHIPS $1.50 $1.25 $4.65
a A lollipop and a soft drink $ 1 . 5 0 $ 1 . 2 5 + b A soft drink and a pen c A cap and a can of soft drink d Potato chips and a pen MP_NSW_SB3_38329_TXT_4PP.indb 120 29-Aug-23 15:23:40 DRAFT
$7.99 $2.49
A farmer has 24 sheep that will need to be separated into equal groups for shearing.
Write a number sentence to solve each problem.
Problem
a Farmer Bindi wants to put the sheep into three paddocks. How many will be in each paddock?
b Farmer Mills wants to put the sheep into six paddocks. How many sheep will he have in each paddock?
c Farmer Hassan wants to put the sheep into two paddocks only. How many sheep will he have in each paddock?
d Farmer Julie wants to put the sheep into four paddocks. How many sheep will she have in each paddock?
Solve these problems.
a Divide 18 people into 3 groups.
b Share 20 marbles among 5 children.
c Divide 24 plants into 4 groups.
d Share 25 cakes among 5 people.
e Share 30 teddies among 5 girls.
f Break 20 lollies into 4 groups.
g Share 30 marbles among 6 children.
h Share 30 fish into 5 fish bowls.
i Share 40 chocolates among 5 boys.
Number sentence
121 unit Oxford University Press Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values 29
PROBLEM SOLVING L N CCT WE
Division problems
5
÷ =
4 MP_NSW_SB3_38329_TXT_4PP.indb 121 29-Aug-23 15:23:41
DRAFT
This parallelogram’s opposite sides are of equal length and are parallel to each other.
A rectangle is a parallelogram because its opposite sides are of equal length and are parallel to each other.
Colour the shapes below that are parallelograms.
DRAFT

A square is also a parallelogram because its opposite sides are equal and parallel to each other.
A rhombus is a parallelogram because its opposite sides are equal and parallel.
Use the dot paper to complete these models of three parallelograms.
Answer the questions.
a Which shapes above are parallelograms?
b Which shapes have parallel sides but are not parallelograms?
c Which shapes have no parallel sides?
Parallelograms are four-sided shapes (quadrilaterals) where each pair of opposite sides are parallel and of equal length. Identifies angles and classifies them by comparing to a right angle
d Do all parallelograms contain at least one right angle?
Oxford University Press 122 unit
REASONING L N Parallelograms 29
Compares two-dimensional shapes and describes their features
A B C D E F G H
6 7 8 MP_NSW_SB3_38329_TXT_4PP.indb 122 29-Aug-23 15:23:42
Answer true or false.
a Blue is the most likely colour to be spun.
b The spinner is more likely to spin yellow than green.
c Red is the least likely colour to be spun.

d The spinner is more likely to spin yellow than blue.
e The spinner is less likely to spin red than blue.
f There is no chance of spinning purple.
g Green is more likely to be spun than all the other colours combined.

Mr Borg gave his class a spinning wheel divided into 10 sections. He asked his class to colour the sections to reflect the chances of the colours being spun, shown on the labels below.
a
Green has only one chance of being spun.
b
Red is twice as likely to be spun than green.
c
Pink has twice the chance that red has.

d
Yellow has more chance than red but less than pink.
11
Answer the questions.
a What are the possible outcomes when drawing a lolly out of this box?
LolliesSuper
b Are you more likely to pick out a black lolly than a blue lolly?
Oxford University Press 123 unit Records and compares the results of chance experiments
COMMUNICATING L 29
Chance—likelihood
9 10
Yellow Blue
Green Red
Lollies
c Explain why. MP_NSW_SB3_38329_TXT_4PP.indb 123 29-Aug-23 15:23:43
DRAFT
Trading Cards ALBUM
Angelo had 3133 trading cards in one album and 2119 in another. How many did he have altogether?
Add 3 thousands plus 2 thousands equals 5 thousands.
3 ones plus 9 ones equals 12 ones. Trade 10 ones for 1 ten. Record 2 in the ones column. Add 1 hundred plus 1 hundred equals 2 hundreds.
Add 1 ten plus 3 tens plus 1 ten equals 5 tens.
Jack worked out a way to add 500 + 650 easily. He added 500 + 500 to make 1000 and then added 150 more to make 1150.
Use any mental strategy you wish to add these numbers mentally.
300 + 425 =
500 + 617 =
600 + 780 =
800 + 965 =
600 + 837 = b 600 + 725 =

Oxford University Press 124 unit Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers PROBLEM SOLVING N CCT Addition to 9999 30 Write a problem to suit this number sentence. 2237 +
= 3 Add these numbers. a 4 2 7 + 6 3 3 1 5 Tens Hund Thou Ones b 5 6 3 + 3 4 2 3 6 Tens Hund Thou Ones c 6 2 6 + 4 0 3 3 4 Tens Hund Thou Ones d 4 7 7 + 5 2 3 3 Tens Hund Thou Ones e 5 8 6 + 2 2 3 4 3 Tens Hund Thou Ones f 0 9 4 + 2 7 4 2 5 Tens Hund Thou Ones g 5 7 9 + 0 2 3 0 3 Tens Hund Thou Ones h 4 8 8 + 3 3 2 5 3 Tens Hund Thou Ones i 4 6 7 + 2 7 3 5 2 Tens Hund Thou Ones j 5 7 7 + 2 8 6 6 2 Tens Hund Thou Ones k 8 8 6 + 5 6 7 2 Tens Hund Thou Ones l 8 9 8 + 3 8 6 3 Tens Hund Thou Ones
423
c
e
d
f
1 2
a
EXAMPLE 3 1 3 2 9 + 2 5 1 1 2 3 1 2 5 Tens Hund Thou Ones MP_NSW_SB3_38329_TXT_4PP.indb 124 29-Aug-23 15:23:45 DRAFT
Complementary fractional parts

The complement of a fraction is the number of parts that haven’t been counted. For example, if you look at 3 5 , then 2 5 is the complement because it takes both 3 5 and 2 5 to make the whole ( 5 5 ).
Write the shaded fraction and the complementary fractional part for each.
Write the complementary fraction of each strip below.
Extend each strip to one whole.
Oxford University Press 125 unit
Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths) 30
REASONING 3 4 4 and and
and and 4
a b c d 4
a b c d e
a 4 4 c 4 4 d 5 5 b 3 3 5 6 MP_NSW_SB3_38329_TXT_4PP.indb 125 29-Aug-23 15:23:46 DRAFT
8

30 Constant difference in subtraction/estimation
Sometimes it’s easier to solve subtraction if you balance both numbers to more manageable numbers. For example, 83 – 36 can be viewed as 87 – 40 = 47.
Add 4 Add 4
74 – 39 becomes
75 – 40 = 35 Much easier!
DRAFT
i 163 – 38 becomes – =
j 262 – 47 becomes – =
Example
Round up to 50 Round down to 30
48 – 33 is about 20
a 79 – 31 is about


b 63 – 22 is about
f 88 – 34 is about
g 99 – 51 is about
c 87 – 24 is about h 73 – 47 is about
d 83 – 58 is about i 172 – 37 is about
e 92 – 47 is about
j 264 – 46 is about
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
126 unit L N CCT WE
Round each number in the subtraction number sentences to estimate an answer. MP_NSW_SB3_38329_TXT_4PP.indb 126 29-Aug-23 15:23:47
Quadrilaterals are shapes that have 4 straight sides. There are many types of quadrilaterals and some have special names.
A B C
A square is a quadrilateral that has 4 equal sides and 4 right angles.

A rectangle is a quadrilateral that has 2 pairs of equal sides and 4 right angles.
A rhombus is a quadrilateral that has 4 equal sides and the opposite angles are equal.
D E F
A trapezium is a quadrilateral with one set of parallel sides.
A parallelogram is any quadrilateral with 2 sets of parallel sides.
Draw an example of each quadrilateral listed above.
A kite is a quadrilateral that has two pairs of equal sides. None of the sides are parallel to other sides.
Answer the questions.
a Do all quadrilaterals have parallel sides?
b Which quadrilaterals (A to F above) have all sides of equal length?
127 unit Oxford University Press REASONING L N Compares two-dimensional shapes and describes their features Quadrilaterals 30
a b c d e f
9 10 MP_NSW_SB3_38329_TXT_4PP.indb 127 29-Aug-23 15:23:47
DRAFT
Write the numbers on the place value chart. The first one has been done for you.

Write the place value of each bold digit.
Expand each number. The first one has been done for you.
The 4 in 4679 is worth a lot more than the 4 in 8046.
Order the numbers from least to greatest.
Oxford University Press 128 unit Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands 31 COMMUNICATING, REASONING N CCT
thousands
Place value to tens of
Number Ten thousands Thousands Hundreds Tens Ones a 435 4 3 5
3568
2079
35 674
56 759
25 807
b
c
d
e
f
a 523 e 35 765 b 2854 f 43 279 c 3863 g 56 423 d 4782 h 51 274
a 2347
2000 + 300 + 40 + 7
3578
+ +
6354
+ + +
35 672
+ + + +
74 263
+
=
b
=
+ c
=
d
=
e
=
+ + +
a 357 276 589 277 b 3546 2089 307 3544 c 32 579 32 501 32 525 32 999 d 33 506 34 506 35 506 60 533 e 27 963 9999 35 902 34 006 1 2 3 4 MP_NSW_SB3_38329_TXT_4PP.indb 128 29-Aug-23 15:23:48 DRAFT
Patterns on hundreds charts
5
Counting by 3 and 6
31
a Start at 3 and circle all numbers as you count by 3 to 100.
b Start at 6 and colour all numbers blue as you count by 6 to 100.
c What patterns do you see?
6
More patterns
a Start at 9 and circle all numbers as you count by 9 to 100.
b Put a cross on all numbers that are 5 less than any number ending in 9.
c What patterns do you see?
Can you see any other patterns on the hundreds chart? What are they? 7
Continuing a pattern
a What is the next number in this pattern?
b Continue the pattern.
c If the pattern was extended past 100, what would the next four numbers be?
Oxford University Press 129 unit Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
PROBLEM SOLVING, COMMUNICATING L CCT N
8 1 2 4 5 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 3 6 1 2 4 5 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 3 6 1 2 4 5 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 3 6 MP_NSW_SB3_38329_TXT_4PP.indb 129 29-Aug-23 15:23:49 DRAFT
Nets 31
Find a small cardboard box, such as a thumbtack box, sultana packet or staples box, and carefully tear along the edges until you can lay it out flat.
Study its net and sketch it in the space opposite.
Find a larger box, such as a cardboard fruit box, and pull it apart to reveal its net.
Study its net, then sketch it below.
Oxford University Press 130 unit
COMMUNICATING, PROBLEM SOLVING, REASONING L
Makes and sketches models and nets of three-dimensional objects including prisms and pyramids
9
10 MP_NSW_SB3_38329_TXT_4PP.indb 130 29-Aug-23 15:23:50
DRAFT
Grams are used to measure objects that are not very heavy. 1 kilogram = 1000 grams.
The packet of oats is 400 g.

Use a pan balance to see how many of these standard masses are needed to balance 1 kg.
What is the total mass of these masses?

Oxford University Press 131 unit Estimates, measures and compares the masses of objects using kilograms and grams 31 The gram REASONING N CCT L
a 500 g b 200 g c 100 g 500
=
200
1 kg 100 g ×
kg
g ×
1 kg
g × =
= 1
13 14 500 g 200 g 100 g 50 g
+ + +
Coffee SALT Mayo Coolah Iced Tea HONEY MP_NSW_SB3_38329_TXT_4PP.indb 131 29-Aug-23 15:23:51
DRAFT
Number patterns 32
Complete the pattern up to eight numbers, then state what the tenth number would be. a 40 36 32 28 24
8 16 24 32 40
What would the tenth number be? What would the tenth number be?
7 14 21 28 35
18 27 36 45
What would the tenth number be? What would the tenth number be? Complete the fraction patterns.

What did you learn?
Subtract 6 from this group of numbers.
What did you learn?
Make up a number pattern that adds 7 to a sequence of numbers.
Oxford University Press 132 unit Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths) COMMUNICATING L
c
d 9
b
a 1 8 2 8 3 8 4 8 c 1 4 2 4 3 4 4 4 b 1 10 2 10 3 10 4 10 d 1 5 2 5 3 5 4 5
6 to
of
+ 6 16 26 36 46 56 66 76 86 6
Add
this group
numbers.
– 19 29 39 49 59 69 79 89 99 6
1 2 3 4
+ 7 5
55, 50,
MP_NSW_SB3_38329_TXT_4PP.indb 132 29-Aug-23 15:23:53 DRAFT
My pattern is take away 5.
45, ...
Subtraction bar models
Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

Oxford University Press 133 unit
32
the bar models to help you write a subtraction number sentence. a 55 32 23 55 – 32 = 23 b 64 42 22 64 – = c 77 62 15 77 – = d 67 44 23 67 – = e 52 39 13 52 – = f 72 44 28 72 – = g 65 39 65 – = h 60 15 60 – = i 199 93 199 – = j 192 89 192 – = 6 You can write 58 – 32 = 26 or 58 – 26 = 32 58 32 26 MP_NSW_SB3_38329_TXT_4PP.indb 133 29-Aug-23 15:23:54
Use
DRAFT
I am a shape that has 4 right angles and 4 sides the same length. I am drawn with my base on a different orientation.
I am a shape that has 4 right angles. I have 2 shorter sides the same length and 2 longer sides the same length.
I am a 3-sided shape. I have 3 angles the same size and 3 sides the same length.
I am an irregular shape that has 5 sides. My angles and side lengths are not always the same size.
I am a regular shape with 6 sides. All my angles are the same size. All my sides are the same length.
I am a 3-sided shape. I have 2 angles the same size and 2 sides the same length.
I am a regular shape that has 5 sides. All my angles are the same size. All my sides are the same length.
I am a regular shape with 8 sides. All my angles are the same size. All my sides are the same length.
I am a quadrilateral. I have one pair of parallel sides only.
I am a quadrilateral. None of my angles are the same size, all my side lengths are different. None of my sides are parallel.
I am an irregular shape that has 8 sides. My angles and side lengths are not always the same size.
Oxford University Press 134 unit Compares two-dimensional shapes and describes their features Describing polygons 32 Draw a line to match each shape to its description. 7 a b c d e f g h i j k
MP_NSW_SB3_38329_TXT_4PP.indb 134 29-Aug-23 15:23:55
DRAFT
Cubic centimetres
Find a small box, such as a sultana packet or small chalk box, and fill it with cubic centimetre blocks, e.g. centicubes or Base 10 ones.
What was the volume of the box? cm3
Make these models from centicubes or Base 10 ones, then record their volumes by counting the number of cubes used in their construction.
Which model has the smallest volume?
Which model has the largest volume?
Which four models have the same volume?
SULTANAS SULTANAS
Oxford University Press 135 unit Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres
32
COMMUNICATING, REASONING L CCT N
8
9
10 11 12 a b c d e f g h cm3 cm3 cm3 cm3 cm3 cm3 cm3 cm3 MP_NSW_SB3_38329_TXT_4PP.indb 135 29-Aug-23 15:23:55 DRAFT
Samantha had $3476 in her bank account but spent $2232 on a new TV. How much did she have left?

Solve the problems. 2
a How much more expensive is the watch than the ring?
b How much more expensive is the ring than the bracelet?

Oxford University Press 136 unit Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values PROBLEM SOLVING N CCT PSC WE Subtraction
e f g h 7 7 7 7 6 7 6 6 7 6 7 8 8 8 7 6 3 0 4 7 3 7 5 6 4 4 4 3 3 0 6 5 i Thou Hund Tens Ones j Thou Hund Tens Ones k Thou Hund Tens Ones l Thou Hund Tens Ones 7 6 9 8 9 9 9 7 8 7 5 4 3 8 7 5 4 2 0 7 7 7 4 3 5 6 3 3 1 7 5 4
to 9999 33
Hund Tens Ones
Thou
Thou Hund Tens Ones 8 5 1 11 2 10 12 3 4 8 7 5 9 1 11 2 10 6 12 3 4 8 7 5 9 1 11 2 10 6
3 thousands take away 2 thousands leaves 1 thousand. 6 ones take away 2 ones leaves 4 ones. 4 hundreds take away 2 hundreds leaves 2 hundreds. 7 tens take away 3 tens leaves 4 tens. EXAMPLE 7 6 2 4 4 4 3 2 3 2 2 1 Tens Hund Thou Ones $2987 $2574 $1331 MP_NSW_SB3_38329_TXT_4PP.indb 136 29-Aug-23 15:23:57 DRAFT
When numbers are added, it doesn’t matter in which order they are added, e.g. 2 + 7 + 8 = 17 8 + 7 + 2 = 17 7 + 8 + 2 = 17
When numbers are multiplied, it doesn’t matter in which order they are multiplied,
Complete the sets of number sentences to see if the rule above is true.
a 6 + 5 + 4 =
b 7 + 5 + 6 =
d
e
f

=
Write your own multiplication number sentences.
Rewrite each number sentence to make it easier to solve.
a 6 + 8 + 4 = becomes + + =
b 17 + 9 + 3 = becomes + + =
c 18 + 7 + 2 = becomes + + =
d 9 + 15 + 5 = becomes + + =
e 17 + 4 + 6 = becomes + + =
f 5 × 7 × 2 = becomes × × =

Who baked the most cakes?
Dad baked enough cakes to fill 2 trays with 5 rows of 4 cakes on each tray. Grandma baked enough cakes to fill 4 plates with 2 rows of 5 cakes on each plate.
Oxford University Press 137 unit Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems 33 Associative property COMMUNICATING, REASONING N
e.g.
2
3
3 × 2 × 4 = 24 4 × 2 × 3 = 24
×
× 4 = 24
4
5
6
+
+
=
6 + 5 + 7 =
5 + 7 + 8 =
c 8 + 7 + 5 =
4 + 6 + 9 =
9 + 6 + 4 =
3
2
4
4 × 2 × 3 =
×
×
1
4
5
5 × 4 × 1 = 3
×
×
=
×
4
× = × × =
5 6 7 + 9 + 3 becomes 7 + 3 (to make 10)
9. MP_NSW_SB3_38329_TXT_4PP.indb 137 29-Aug-23 15:23:58
+
DRAFT
Non-standard partitioning
Complete the numeral expanders to show how the numbers can be partitioned in different ways.
How many tens in each number?
a 374
b 563
c 727
d 805
e 297
8 9
How many hundreds in each number?
a 3546
b 2780
c 3676
d 8504
e 3874
f 5679
What number is 354 tens and 6 ones? 3446, 3546, or 6453?

Oxford University Press 138 unit 33 Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands COMMUNICATING L
a ones tens hundreds ones tens 3 6
b ones tens hundreds ones tens 5 0 7 c ones tens hundreds ones tens 6 9 0 d ones tens hundreds ones tens ones 8 5 7 e ones tens hundreds ones tens ones tens hundreds thousands 3 5 7 6 ones f ones tens hundreds ones tens ones tens hundreds thousands 2 5 4 3 ones 7
7
MP_NSW_SB3_38329_TXT_4PP.indb 138 29-Aug-23 15:23:59
DRAFT
Millilitres are used to measure small quantities of liquid. The symbol for millilitres is mL. There are 1000 mL in one litre (1 L).

Use a 1 millilitre eye dropper to measure the capacity of a teaspoon. You may have to balance the teaspoon with a pencil.
Use a 50 mL medicine glass to measure the capacity of four caps that you can find around the school. For example, the cap from a bottle of water, or a glue stick.
Oxford University Press 139 unit Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres 33 Millilitres REASONING N Millilitres Cap mL 50 mL 45 mL 40 mL 35 mL 30 mL 25 mL 20 mL 15 mL 10 mL 5 mL
11
12
cap
STICK STICK GLUE STICK GLUE STICK GLUE STICK STICK MP_NSW_SB3_38329_TXT_4PP.indb 139 29-Aug-23 15:24:01
DRAFT
4
Fractions can be written as decimals.
E.g. 25 out of 100 can be written in the decimal form as 0.25


The decimal point separates the fractional part from the whole number.
Order the decimals you marked on the metre ruler above from smallest to largest. Smallest Largest
Oxford University Press 140 unit Represents and compares decimals up to 2 decimal places using place value 34 Introduction to decimals COMMUNICATING, REASONING CCT
25
0 10 20 30 40 50 60 70 80 90 100 rulex a 0.25 m b 0. m c 0. m d 0. m e 0. m f 0. m MP_NSW_SB3_38329_TXT_4PP.indb 140 29-Aug-23 15:24:02
100
DRAFT
Double and double again to multiply by four.
a 2 × 4 = f 7 × 4 =

b 3 × 4 = g 9 × 4 =
c 5 × 4 = h 8 × 4 =
d 4 × 4 = i 10 × 4 =
e 6 × 4 = j 1 × 4 =
Double a smaller times table to find the answer.
a 3 × 6 = e 10 × 6 =


b 5 × 6 = f 9 × 6 =
c 2 × 6 = g 7 × 6 =
d 4 × 6 = h 6 × 6 =
the skip counting patterns for 6, 7, 8 and 9.
Use the skip counting patterns above to complete the multiplication number sentences.
Oxford University Press 141 unit
REASONING N L Multiplication strategies 34
Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values
a 0 6 12 30 48 60 b 0 7 14 35 49 70 c 0 8 16 40 56 80 d 0 9 18 27 54 81 90
Complete
a 10 × 6
e 7 × 7 = b 8 × 6 = f 9 × 7 = c 3 × 7 = g 5 × 8 = d 5 × 7 = h 6 × 9 = 5 6 7 8 5
4
Double
5
8
...2, 4, 6, 8... MP_NSW_SB3_38329_TXT_4PP.indb 141 29-Aug-23 15:24:04 DRAFT
=
x
=
5 = 10 Double 10 = 20
x 4 = 20
x 6 = Think 8 x 3 = 24 Then double =48
Combining and splitting shapes
Reflect, rotate and translate the tangram shapes to construct the designs shown on the right. Use tangram sets, or trace over the tangram below and cut it out to complete the activity.
DRAFT
Use tangrams to construct these shapes. Help has been given by shading some tangram shapes.
Oxford University Press 142 unit Performs transformations by combining and splitting two-dimensional shapes PROBLEM SOLVING L CCT
34
9
10 a b c MP_NSW_SB3_38329_TXT_4PP.indb 142 29-Aug-23 15:24:04
Length using decimal notation
Length can be measured in metres.
Write m for metre or cm for centimetre to show which unit you would use to measure these lengths.
a Length of a matchbox


b Length of a car
c Length of a book
d Length of a glue stick
e Length of a soccer field
f Length of the playground
Decimal notation can be used to record lengths or distances, e.g. 1 metre 35 centimetres can be recorded as 1.35 m.
Convert each measurement from metres and centimetres to metres using decimal notation.
a 1 m 65 cm = 1.65 m
b 2 m 75 cm =
c 3 m 45 cm =
12
The abbreviation
0 10 20 30 40 50 60 70 80 90 100 rulex
for metre is m. 100 centimetres = 1 metre.
f 4 m 45 cm =
g 6 m 32
=
h 3 m 78 cm =
1 m
i 2 m 98 cm = e 2 m 56 cm = j 5 m 42 cm = 13 Oxford University Press 143 unit COMMUNICATING N Measures and estimates lengths in metres, centimetres and millimetres Represents and compares decimals up to 2 decimal places using place value 34
cm
d
27 cm =
MP_NSW_SB3_38329_TXT_4PP.indb 143 29-Aug-23 15:24:05
DRAFT
Problem solving
Find the difference in cost between the:
a watch and calculator $ d scooter and calculator $
b watch and kettle $ e scooter and kettle $
c kettle and calculator $ f laptop and scooter $
Solve the problems, then check them using a calculator.
a How much would a watch and scooter cost? $
b How much would a kettle and watch cost? $
c How much for a calculator, laptop and watch? $
d How much for 5 calculators? $
e How much for 10 watches? $
f If John bought 2 kettles, how much change would he receive from $100? $

g If Anna bought a calculator and a watch, how much change would she receive from $50? $
h If Kelly bought a scooter and a calculator, how much change would she receive from $200? $
i How much more does a scooter cost than a watch? $
j Jamal bought a watch and a scooter. If he paid for them with four $50 notes, how much change did he receive? $
Oxford University Press 144 unit PROBLEM SOLVING, COMMUNICATING N CCT 35 Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values
addition + and subtraction signs to make each number sentence true. a 20 14 2 = 32 g 20 20 20 = 60 b 35 5 + 10 = 40 h 30 15 8 = 37 c 26 7 + 7 = 40 i 40 17 7 = 50 d 27 7 + 20 = 40 j 45 8 10 = 27 e 15 8 6 = 1 k 77 27 7 = 43 f 26 24 + 20 = 70 l 16 16 16 = 16 12 9 3 11 9 3 1 11 2 10 4 8 5 7 12 6 1 11 2 10 5 7 1 ON OFF 2 3 0 + =$14 $146 $582 $37 $24
Use
1 2 3 MP_NSW_SB3_38329_TXT_4PP.indb 144 29-Aug-23 15:24:07
DRAFT
Missing numbers 35


Ms Wu wrote a number sentence and an answer on the board. Her head blocked out the sentence but we could see the answer was 24. What could the number sentence have been? Write some solutions. The first one has been done for you.
Use + , and × symbols in the number sentences below to make them true.

Oxford University Press 145 unit Completes number sentences involving addition and subtraction by finding missing values Completes number sentences involving multiplication and division by finding missing values REASONING, PROBLEM SOLVING N CCT
a 30 6 = 24 g = 24 b + = 24 h = 24 c 6 × = 24 i = 24 d = 24 j = 24 e = 24 k = 24 f = 24 l = 24
in
a 7 + = 13 f 15 + = 22 k 7 × = 28 b 3 + = 20 g 30 + = 45 l 80 = 30 c 20 = 12 h 30 = 15 m 25 + = 50 d 15 + = 25 i 3 × = 18 n 56 = 30 e 39 = 32 j 5 × = 35 o 24 + = 40
Supply the missing numbers
these questions.
a 4 5 = 9 g 3 4 5 = 12 b 4 5 = 20 h 3 4 5 = 2 c 2 8 = 16 i 5 2 3 = 13 d 15 2 = 13 j 4 5 2 = 22 e 12 13 = 25 k 5 2 3 = 30 f 14 5 = 9 l 3 4 5 = 17 4 5 6 MP_NSW_SB3_38329_TXT_4PP.indb 145 29-Aug-23 15:24:09 DRAFT
Penta means five. A pentagon is a shape with five sides. A regular pentagon has all its sides equal in length and all its angles equal.
Which of the shapes above are: a triangles? b pentagons? c hexagons?
Use the dot paper to draw two pentagons of your own.
b

Oxford University Press 146 unit COMMUNICATING L Compares two-dimensional shapes and describes their features
Regular pentagon Irregular pentagon
the dots to form pentagons. a 3 2 1 5 4 b 1 5 2 3 4 c 1 5 4 2 3 7
35 Pentagons
Join
8 A F I G B C J K L M E D H
a
9
MP_NSW_SB3_38329_TXT_4PP.indb 146 29-Aug-23 15:24:10 DRAFT
35 Square centimetres
Calculate the area of each of the shapes below by counting the square centimetres. The short way of writing square centimetres is cm2.
Which shape’s area was the hardest to measure? Why?
Can you find a better way to count the square centimetres on squares and rectangles? What is it?
Oxford University Press 147 unit Estimates, measures and compares areas using square centimetres and square metres COMMUNICATING, PROBLEM SOLVING N
a b c d e f g h i j cm2 cm2 cm2 cm2 cm2 cm2 cm2 cm2 cm2 cm2
10 11 12 MP_NSW_SB3_38329_TXT_4PP.indb 147 29-Aug-23 15:24:10
DRAFT
Solve the problems.
a Mr Bean saved $136 in May and $138 in April. What are his savings for the two months?
b Juanita had 28 marbles to share among 4 people. How many did each receive?
c Aki had a bag of 252 pegs but used 124. How many pegs were left?
Oxford University Press 148 148 Diagnostic review 4 PART Complete the multiplications.
3 books at $10 each $
6 books at $5 each
a
b
$
pens
$4
c 7
at
each $
calculators
Solve the division facts. e 15 ÷ 3 = h 32 ÷ 4 = f 30 ÷ 5 = i 24 ÷ 4 = g 20 ÷ 4 = j 21 ÷ 3 = 2 PART Solve the additions and subtractions. 4 5 1 3 + 2 4 a 5 7 2 3 + 6 8 b 7 8 1 6 + 1 6 4 6 c 8 4 6 3 4 6 d 9 7 7 9 8 8 e 3 2 5 2 7 3 6 4 f 1 PART Expand the numbers.
4235 =
5362 = + +
=
= +
Order the numbers from smallest to largest. 3576 765 5736 36 676 3 PART Find the missing numbers. a 13 + = 16 b 20 = 16 c + 15 = 35 d × 6 = 24 e 7 × = 42 f 30 ÷ = 6 4 PART Write a decimal to describe each hundredths grid. a . b . c . 5 PART
d 6
at $3 each $
a
4000 + + + b
+ c 6289
+ + + d 7563
+ + e
6 MP_NSW_SB3_38329_TXT_4PP.indb 148 29-Aug-23 15:24:11
DRAFT
5
a Start at X and travel north 4 spaces.
b Turn east and travel 3 spaces.
c Turn south and travel 3 spaces.
d Head east 4 spaces then north 3 spaces.
e Head west 2 spaces.
f What shape did you find?
Oxford University Press 149 Diagnostic review 4 PART
these objects. a Any prism c Cylinder b Cone d Any pyramid 7 PART Record the area of each shape in square centimetres. d b a c Shape Area a cm2 b cm2 c cm2 d cm2 10 PART Colour the shapes that are parallelograms. a b c e f d 8 PART Record each length measurement as a decimal. a 1 m 25 cm = . m b 2 m 37 cm = . m c 6 m 49 cm = . m 11 PART N W E S
Sketch
9 PART
12
Half past 8 c Quarter to
11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 09_MP_NSW_SB3_38329_TXT_4pp.indd 149 31-Aug-23 14:09:04 DRAFT
Draw hands on the clocks to show the times.
a
abacus
An instrument used for calculating.
ascending order
An arrangement of numbers from smallest to largest.
256, 291, 307, 452
associative property
Thou Hund Tens Ones
acute angle
An angle less than 90°
acute angle
addition (+)
The operation that finds the sum or total. am ( ante meridiem )
The morning. Any time from midnight to noon, e.g. 7:30 am is 7:30 in the morning.
analog clock
A clock face with numbers 1 to 12, and two hands.
A series of numbers can be added in any order without changing the result.
5 + 4 + 6 = 15
4 + 6 + 5 = 15
6 + 5 + 4 = 15
A series of numbers can be multiplied in any order without changing the result.
5 × 4 × 3 = 60
4 × 3 × 5 = 60
3 × 5 × 4 = 60
axis of symmetry
An imaginary line that divides a shape exactly in half. If a shape is folded along this line, both sides will match.
angle
The amount of turn between two arms around a common endpoint (the vertex).
arm area
amount of turn vertex arm
The surface covered by any 2D shape. Area can be measured in cm2, m2, hectares and km2.
3 cm
Area = 6 cm2
array
2 cm
An arrangement of objects or symbols into rows and columns.
base
The bottom line of a 2D shape.
base
The bottom face of a 3D object.
base
• pyramids have one base base
For example:
• prisms have two bases. base base
capacity
The amount a container can hold. Capacity can be measured in millilitres (mL), litres (L) and kilolitres (kL).
Dictionary Oxford University Press 150
SHAMPOO 1 L MP_NSW_SB3_38329_TXT_4PP.indb 150 29-Aug-23 15:24:13
DRAFT
centimetre (cm)
A unit for measuring length. 100 cm = 1 metre 0 1 2 3
circle
A plane shape bounded by a continual curve that is always the same distance from the centre point.
column graph (bar graph or bar chart)
A column graph generally uses vertical columns to represent data. In a bar graph or bar chart the bars can be either vertical or horizontal.
coordinate points
Coordinates or grid references locate points or positions on a grid. The horizontal position is given before the vertical position, e.g. the circle is located at (C,3).
corner (vertex)
The point where two or more lines meet to form an angle.
cross-section
The face that is left when a solid (3D) object is cut through, parallel with its base.
cube
commutative property
Two numbers can be added in any order to give the same total.
15 + 13 = 28 13 + 15 = 28
Two numbers can be multiplied in any order to give the same product.
5 × 4 = 20 4 × 5 = 20
compass points
The cardinal compass points are north, south, east and west.
(north)
(east) (west) E W N S
(south)
A 3D object with six square faces, eight corners and twelve edges.
cubic centimetre
A unit of volume. A centimetre cube has a volume equal to one cubic centimetre.
cylinder
An object with two circular faces and one curved surface.
data
Information gathered together, such as a set of numbers or facts.
cone
A 3D object with a circular base, tapering to a point (the apex).
decade
Ten years, e.g. 2013–2023. Also, a group of tens.
decimal
A fraction can be written as a decimal, e.g. 75 out of 100 can be written as 0.75 in decimal form.
Dictionary Oxford University Press 151
0
1 2 3 4 5
Colours
Blue Orange Red Yellow Green
Favourite colour
Number of students
4 3 2 1 A B C D
corner
1 cm 1 cm 1 cm
Baked Beans
0.75 MP_NSW_SB3_38329_TXT_4PP.indb 151 29-Aug-23 15:24:15
DRAFT
decimal point
A point used to separate the fraction part from the whole number.
whole number part
decimal point
denominator
4.75
edge
The intersection of two faces on a 3D object.
fraction part
The bottom number of a fraction that tells how many equal parts there are in the whole.
1 4 numerator denominator
descending order
An arrangement of numbers from largest to smallest, e.g. 108, 99, 76, 54
diagonal
A straight line which joins two non-adjacent corners of a polygon. diagonal
digital clock
A clock which displays only numbers. It has no hands.
dimension
A measurement of length, width (breadth) or height.
height width (breadth) length
division ( ÷ )
The operation that breaks groups or numbers into equal parts. 15 ÷ 3 = 5
double
Multiply by two.
edge
equivalent fractions
Fractions having the same value.
1 2 2 4 even number
A number that can be divided equally by two, e.g. 2, 4, 6, 8, 10, 12. faces
The surfaces of a 3D object. face face face flip (reflect)
To turn a shape over.
fraction
Any part of a whole or group.
front view
The view we see when we look at an object from the front.
3D object
front view
gram
A unit for measuring mass.
1000 grams = 1 kilogram
greater than (>)
The ‘greater than’ symbol shows the relationship between two unequal numbers, e.g.
8 > 5
Dictionary Oxford University Press 152
1 3
1 4
=
=
MP_NSW_SB3_38329_TXT_4PP.indb 152 29-Aug-23 15:24:16
DRAFT
grid references
Grid references locate positions on a map or grid. The horizontal position is given before the vertical position, e.g. the circle is located at C3.
length
The longer of the two dimensions of a shape.
length
less than ( < )
width (or breadth)
The ‘less than’ symbol shows the relationship between two unequal numbers.
5 < 8
grouping
A way of dividing an amount into equal-sized groups, e.g.
hexagon
2
A 2D shape with six straight sides.
line of symmetry
A line which divides a shape in half exactly. Shapes can have more than one line of symmetry.
line of symmetry
regular hexagon irregular hexagon
horizontal
At right angles to the vertical.
vertical line
horizontal line
hundredth
One part of a whole that has 100 parts altogether.
kilogram (kg)
The base unit for measuring mass.
Flour
1 kg
1 kg = 1000 grams
kilometre (km)
A unit of length.
1 km = 1000 metres
litre (L)
A unit of capacity.
1 L = 1000 millilitres
mass
DRAFT
The amount of substance in an object.
1000 grams = 1 kg
1000 kg = 1 tonne
metre (m)
A unit of length.
1 metre = 100 cm
millilitre (mL)
A unit of capacity. An object with a volume of 1 cm3 displaces 1 mL of water.
millimetre (mm)
A unit of length.
multiple
1 cm 1 cm
1000 mL = 1 litre 1 cm
10 mm = 1 centimetre
The result of multiplying a given number by any other number is a multiple of that given number.
Multiples of 4 are: 4, 8, 12, 16, 20, etc.
Multiples of 5 are: 5, 10, 15, 20, 25, etc.
Dictionary Oxford University Press 153
4 3 2 1
A B C D
1 L MILK
MP_NSW_SB3_38329_TXT_4PP.indb 153 29-Aug-23 15:24:18
groups of 4 in 8
multiplication (×)
The operation which finds the product of two or more numbers. Multiplication can be seen as repeated addition.
2 + 2 + 2 + 2 + 2 = 10 5 × 2 = 10
parallelogram
A four-sided 2D shape which has two pairs of opposite sides that are parallel and of equal length.
pattern
net
A flat shape that can be folded to make a 3D object.
number line
A line on which numbers are marked. Number lines can be used to represent calculations.
3 + 5 = 8
0 1 2 3 4 5 6 7 8 9 10
number pattern
Any set of numbers that follow a pattern or sequence.
1, 3, 5, 7, ___ , ___ , (The pattern is + 2)
3, 9, 27, ___ , ___ , (The pattern is × 3)
numeral
Any figure used to represent a number, e.g.
0, 1, 2, 3, 4, 5
A series of shapes, letters, numbers or objects arranged in a recurring sequence, e.g.
4, 14, 24, 34 … or
pentagon
A 2D shape with five straight sides. irregular pentagon
regular pentagon
perimeter
The distance around the edges of a shape.
Perimeter = 3 m + 4 m + 5 m
3 m 5m
4 m
numerator
The top number of a fraction, telling us how many equal parts there are out of the whole.
octagon
Perimeter = 12 m
perpendicular lines
Lines that intersect at right angles.
3
4 numerator denominator
A 2D shape with eight straight sides.
regular octagon
odd number
irregular octagon
A number that is not divisible by two, e.g.
1, 3, 5, 7, 9, 11
parallel lines
Two or more lines that never meet and are exactly the same distance apart along their entire length.
picture graph
A graph that uses symbols to represent quantities.
Favourite animals sheep
horse cat hen cow
place value
The value of a digit determined by its place in the number.
Dictionary Oxford University Press 154
346 495 704 4
4 tens 4
4
4
MP_NSW_SB3_38329_TXT_4PP.indb 154 29-Aug-23 15:24:20 DRAFT
=
=
hundreds
= 4 ones
plan
A diagram from above, showing the position of objects.
quarter
One-fourth of a whole or a group.
= 1 4
1 4 are girls
rectangle
plane shape
A 2D shape.
Example: octagon
pm ( post meridiem )






After midday. Any time between noon and midnight, e.g. 7:30 pm is 7:30 in the evening.


polygon
A 2D shape with three or more angles and straight sides.


















prism
Example: octagon






A 3D object that has a pair of congruent parallel bases that are polygons, and rectangular side faces.
product
The answer achieved by multiplication.
9 × 3 = 27
product
A four-sided 2D shape with four right angles and two pairs of parallel sides that are also equal.
square oblong
reflect (flip)
To turn a shape over. rhombus
A four-sided, 2D shape with all four sides equal. A rhombus has two pairs of parallel sides and its opposite angles are equal.
right angle
An angle of 90° .
pyramid
A 3D object that has only one base and all the other faces are triangular, meeting at a point (the apex).




quadrilateral
A 2D shape with four sides, e.g. a square, a rectangle, a rhombus and a parallelogram. rhombus






rigid
A fixed shape that cannot be pulled out of shape.
rotate (turn)
To turn an object around a fixed point.
rigid non-rigid
point
Dictionary Oxford University Press 155
Dining
Hall Bed 1 Lounge Verandah Bed 2
Kitchen Laundry Bathroom
y y y y x x x x
fixed
MP_NSW_SB3_38329_TXT_4PP.indb 155 29-Aug-23 15:24:22
DRAFT
rounding
Changing an exact value to an estimated value of a more convenient size.
69 70 (rounded to the nearest 10)
785 800 (rounded to the nearest 100)
side view
The view we see when we look at an object from the side.
3D object side view
skip counting
To count by adding the same number each time.
4… 8… 12… 16… 20…
Skip counting by 4
slide (translate)
To move a shape to a new position without turning it.
square kilometre (km2)
A unit for measuring area.
1 km
1 km
1 km × 1 km = 1 km2
square metre (m2)
A unit for measuring area.
1 m
1 m
1 m × 1 m = 1 m2
subtraction (−)
The operation which removes part of a group, and finds the difference.
sphere
A perfectly round 3D object, e.g. a ball.
square
A 2D shape with four equal straight sides and four right angles. A square is also a rectangle.
square centimetre (cm2)
A unit for measuring area.
1 cm 1 cm
1 cm × 1 cm = 1 cm2
DRAFT
9 5 = 4 difference
sum
The answer of addition. The total.
symmetry
A shape has line symmetry if both parts match when it is folded along a line.
tally
To keep count by placing a stroke to represent each item. The fifth stroke crosses the four preceding strokes each time.
= 22
tessellation
A pattern formed by the repetition of shapes so that they fit together without gaps.
Dictionary Oxford University Press 156
MP_NSW_SB3_38329_TXT_4PP.indb 156 29-Aug-23 15:24:24
three-dimensional (3D)
A description of solid objects having three dimensions: length, width (breadth) and height. length
time
twelve-hour time
Time shown by traditional clocks and watches, divided into 12 hours.
twenty-four-hour time
width
height (breadth)
60 seconds = 1 minute
60 minutes = 1 hour
24 hours = 1 day
365 days = 1 year
366 days = a leap year
timeline
A line representing a span of time.
Born School High school Work Married
1998 2003 2008 2013 2018 2023
tonne
A unit of mass.
top view
1000 kg = 1 tonne
The view we see when we look at an object from the top.
3D object
translate (slide)
To move a shape to a new position without turning it.
trapezium
A four-sided, 2D shape with only one pair of parallel sides.
triangle
A 2D shape with three sides and three angles.
turn (rotate)
To move an object around a fixed point.
Time divided into 24-hour time intervals numbered from 1 to 24, so as to distinguish between times in the morning and times in the afternoon.
two-dimensional (2D)
Plane shapes have only two dimensions: length and width (breadth).
vertex
DRAFT
The point where two or more lines meet to form an angle.
vertical
vertex
At right angles to the horizontal. horizontal line vertical line
top view
volume
The amount of space a 3D object occupies.
volume = 2 × 3 × 2 = 12 m3
whole numbers
The counting numbers from one to infinity. 1, 2, 3, 4,
width (breadth)
The shorter of the two dimensions of a shape.
width (breadth)
length
Dictionary Oxford University Press 157
3 m 2 m 2 m
MP_NSW_SB3_38329_TXT_4PP.indb 157 29-Aug-23 15:24:26
b 3 groups of 3 equals 9
c 3 groups of 5 equals 15
d 2 groups of 6 equals 12
e 4 groups of 6 equals 24
f 5 groups of 6 equals 30
g 4 groups of 4 equals 16 h 5 groups of 4 equals 20
groups of 5 equals 20
groups of 5 equals 25
Answers 158 Oxford University Press UNIT 1 2 + 3 4 5 6 7 8 9 10 4 4 5 5 5 6 6 6 6 7 7 7 7 7 8 8 8 8 8 8 9 9 9 9 9 9 9 10 10 10 10 10 10 10 11 11 11 11 11 11 11 11 12 12 13 12 13 14 12 13 14 15 12 13 14 15 16 12 13 14 15 16 17 12 13 14 15 16 17 18 3 10 a b c d e f g h i 2 Hands on. 3 a 14 + 33 = 47 b 25 + 23 = 48 c 36 + 22 = 58 4 Hands on. 5 a 2, 4, 6, 8 b 3, 6, 9, 12 c 5, 10, 15, 20 d 5, 10, 15, 20, 25, 30 e 2, 4, 6, 8, 10, 12, 14 f 4, 8, 12, 16, 20, 24 6 a 2, 4, 6, 8, 10 b 4, 8, 12, 16, 20, 24 c 5, 10, 15, 20, 25 7 a b c d 2 4 6 8 10 12 14 16 18 20 5 10 15 20 25 30 35 40 45 50 4 8 12 16 20 24 28 32 36 40 10 20 30 40 50 60 70 80 90 100 8 9 Hands on. 10 sphere cylinder prism cone pyramid 11 sphere, cone and cylinder 12 a 2 cm c 8 cm e 14 cm b 5 cm d 11 cm 13 a 2 cm c 10 cm e 9 cm b 8 cm d 11 cm f 12 cm 14–15 Hands on. 1 UNIT 1 a 3 d 6 g 10 j 10 b 6 e 7 h 7 k 7 c 7 f 9 i 11 l 8 2 13 5 7 3 10 4 13 6 11 18 9 6 13 18 10 8 5 11 20 20 17 12 11 13 18 7 19 8 6 3 10 9 0 7 2 9 12 0 5 8 10 13 7 0 3 9 8 7 2 13 1 a b c 3 a 3 c 7 e 7 g 5 i 8 b 9 d 8 f 13 h 6 j 14 4 2 3 4 5 6 7 8 9 10 –5 a 345 b 194 c 466 d 575 6 Hands on. 7 a Three hundred and twenty-one b Seven hundred and thirty-seven c Two hundred and ninety-five d Five hundred and seventy-four 8 256 291 307 364 807 999 259 529 952 247 274 472 742 507 605 607 705 a b c d e 9 a c e g b d f h 10 a b c d e f 11 a E b A c B and D d F 12 Hands on.
Hands on.
are poor units for measuring area because they leave gaps. 2 UNIT 1 a 16 e 18 i 17 m 28 b 19 f 16 j 16 n 20 c 18 g 19 k 20 o 20 d 19 h 17 l 29
a 16 b $15 c 20 d 29 3 a $20 b $17 c $16 d $26
Hands on. 5 a 2
of
13
Circles
2
4
groups
3 equals 6
Hands on. Possible answer: 7 a Rana b Brooke c Second row from the bottom and in first column, on the left. d e f g Bobby Harry Sienna Keira Brooke Lauren Hassan Rana Zoe Ella Ethan h Top shelf in the middle. 8 Y O U D I D T H I S V E R Y W E L L 9 Hands on. 10 a Holden c Ford e 2 b Mitsubishi d Toyota UNIT 1 a 18 6 = 12 18 12 = 6 b 16 7 = 9 16 9 = 7 c 29 9 = 20 29 20 = 9 d 29 16 = 13 29 13 = 16 e 47 24 = 23 47 23 = 24 2 a 5 + 8 = 13 b 3 + 9 = 12 c 9 + 5 = 14 3 4 MP_NSW_SB3_38329_TXT_4PP.indb 158 29-Aug-23 15:24:29 DRAFT
i 4
j 5
6
15 60
UNIT
1 hundreds tens ones 2 8 5
a 285 hundreds tens ones 7 4 3
b 743 hundreds tens ones 8 5 4
c 854 hundreds tens ones 9 9 9
d 999 hundreds tens ones 8 7 0
e 870 hundreds tens ones 8 0 9
f 809
2
a 500 + 20 + 7 g 300 + 40 + 7
b 300 + 60 + 3 h 200 + 90 + 6
c 700 + 20 + 5 i 300 + 90 + 0
d 600 + 90 + 4 j 400 + 70 + 0
e 800 + 50 + 6 k 500 + 0 + 8
f 700 + 90 + 7 l 600 + 0 + 9
3
a 45 < 63 h 153 < 298 o 864 > 67
b 72 > 51 i 504 > 376 p 67 < 325
c 86 > 49 j 900 > 899 q 63 > 9
d 37 < 80 k 401 < 921 r 504 > 405
e 8 < 81 l 569 > 385 s 327 < 723
f 21 < 45 m 216 < 621 t 528 > 347
g 89 > 53 n 308 < 925 u 999 > 100
4 a 2 × 5 = 10 or 5 × 2 = 10
b 3 × 5 = 15 or 5 × 3 = 15
c 5 × 5 = 25
d 4 × 5 = 20 or 5 × 4 = 20
5 a 5, 10, 15, 20, 25, 30, 35, 40, 45, 50
b 6, 10, 14, 12, 16, 4, 2, 0, 20, 8
6 a $20 b 25 km c $45
7 a 30 c 50 e 60
b 25 d 45 f 80
8 All answers will be zero.
9 Hands on.
10
a Lake Spin e Forest Park
b Police f Shops
c Tom’s house g Gina’s house
d Town Hall 11 Yes 12 Yes
Answers 159 Oxford University Press
B5, C5 14 C1, D1 15 a True b False c True d True 16 No 17 a 6 b 5 c 4 d 4 e 2 f 19 6 g 110 112 114 116 118 120 122 h 115 117 119 121 123 125 127 6 a 12 – 4 = 8 12 – 8 = 4 b 12 – 7 = 5 12 – 5 = 7 c 15 – 9 = 6 15 – 6 = 9 d 15 – 8 = 7 15 – 7 = 8 e 17 – 9 = 8 17 – 8 = 9 f 20 – 12 = 8 20 – 8 = 12 g 19 – 13 = 6 19 – 6 = 13 7 a 17 – 8 = 9 b 16 – 8 = $8 c 20 – 14 = $6 d 24 – 11 = 13 8 Hands on. 9 1 3 1 2 3 1 2 2 3 a b c 10 Shape Sides Angles Shape Sides Angles a Square 4 4 c Triangle 3 3 b Rectangle 4 4 d Triangle 3 3 11 a Yes b Yes c No 12 Yes 13 a 5 d 20 g 10 j 20 b 10 e 30 h 15 c 15 f 5 i 15 14 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 11 12 1 2 3 4 5 6 7 8 9 10 a 5 past 12 e 15 past 12 b 10 past 12 f 12 o’clock c 20 past 12 g 25 past 12 d past 12 h 22 past 12 1 2 d 14 + 4 = 18 e 2 + 11 = 13 f 8 + 12 = 20 3 Hands on. 4 a 6 d 2 g 16 j 18 b 4 e 10 h 14 c 8 f 12 i 20 5 2, 4, 6, 8, 10, 12, 14, 16, 18, 20 6 a $14 b 16 c 10 d $8 e 18 7–8 Hands on. 9 10 face vertex edge a b c d e vertex face edge vertex face edge edge vertex face face edge vertex 11 a 2 cm c 3 cm e 10 cm b 5 cm d 5 cm f 7 cm 12 8 cm 13–14 Hands on. UNIT 1 a •••••• even •••••• b ••••••• odd •••••• c ••••••••• even ••••••••• d ••••••••••••• odd •••••••••••• e •••••••••••••• even •••••••••••••• f •••••••••••••••• odd ••••••••••••••• 2 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 3 even 4 odd 5 a 7 9 11 13 15 17 19 b 16 18 20 22 24 26 28 c 56 58 60 62 64 66 68 d 41 43 45 47 49 51 53 e 98 96 94 92 90 88 86 f 95 93 91 89 87 85 83 5 MP_NSW_SB3_38329_TXT_4PP.indb 159 29-Aug-23 15:24:31 DRAFT
13
Thursday afternoon bowling
Men Women Girls Boys
12
(Multiply L × W or count all placemats needed to cover table.) 13
Answers 160 Oxford University Press 18
19 a 3 b 7 c 8 UNIT 1 a 12 ÷ 4 = 3 d 20 ÷ 4 = 5 b 12 ÷ 2 = 6 e 15 ÷ 5 = 3 c 15 ÷ 3 = 5 f 18 ÷ 3 = 6 2 a 4 b 5 c 10 d 2 e 20 3 a 6 d 5 g 7 j 14 b 8 e 4 h 12 k 6 c 15 f 9 i 7 l 4 4 a 27 + 14 = 41 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 b 37 + 20 = 57 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 c 28 + 13 = 41 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 d 39 + 23 = 62 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 5 a 35 + 20 + 3 = 58 b 42 + 20 + 5 = 67 c 33 + 30 + 4 = 67 d 46 + 20 + 4 = 70 e 52 + 20 + 6 = 78 6 Shaded: a, b, d, i 7 a No b No c No d Yes e ✔ ✔ ✔ 8 9–10 Hands on. 7 11 100 mL 300 L 1L MILK 12 Hands on. UNIT 1 a 3, 30 d 3, 30 g 9, 90 b 6, 60 e 2, 20 h 8, 80 c 2, 20 f 8, 80 2 a 33 – 15 = 18 and 38 – 20 = 18 b 87 – 29 = 58 and 88 – 30 = 58 c 64 – 37 = 27 and 67 – 40 = 27 d 74 – 56 = 18 and 78 – 60 = 18 e 135 – 27 = 108 and 138 – 30 = 108 3 35, 45, 38, 127 4 a 1 out of 2 1 2 b 3 out of 8 3 8 c 1 out of 4 1 4 d 3 out of 4 3 4 e 5 out of 8 5 8 f 1 out of 8 1 8 g 1 out of 4 1 4 h 7 out of 8 7 8 i 1 out of 2 1 2 5 Ravi 6 Sam 7 Alisha 8 a b c 9 a b c 10 Hands on. 11 a 5 b 6 c 2 8
Hands
Hands on. UNIT 1 a 67 d 61 g 93 j 84 b 85 e 72 h 92 k 76 c 87 f 84 i 92 l 84 2 a 52 km c 82 km e 80 km b 62 km d 76 km 3 a 2, 4, 6, 8, 10, 12, 14, 16, 18, 20 b 4, 8, 12, 16, 20, 24, 28, 32, 36, 40 4 a and b 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 c Hands on. They form a pattern. The table of 2s is
of the table of 4s. 5 a 4 e 16 i 40 m 60 b 12 f 28 j 36 n 80 c 20 g 24 k 44 o 100 d 8 h 32 l 48 6 Hands on. 7 Hands on. 8 23 minutes past 9 Eight twenty-eight Twelve fifteen 35 minutes past 7 45 minutes past 3 Eleven twenty-six a b c d e f 9 a : e : b : f : c : g : d : h : 10 Name Time Fran 9:45 Bree 10:30 Hilda 11:10 Alana 11:45 9 MP_NSW_SB3_38329_TXT_4PP.indb 160 29-Aug-23 15:24:35
on.
part
DRAFT
DIAGNOSTIC REVIEW
g 18 h 60 i 90
j 50 + 8 = 58 k 80 + 9 = 89
l 50 + 9 = 59 m 90 + 9 = 99 Part 2
a 6 18 20 12 24 16
b 3 d 9 f 83 h 80
c 5 e 21 g 30 i 70
Part 3
a 20 b 35 c 24
d 4, 10, 6, 8, 12
e 10, 25, 15, 30, 40
f 10 g 4
Part 4
a hundreds tens ones 2 6 7
b 569 500 + 60 + 9
c 267 200 + 60 + 7
d 853 800 + 50 + 3
Part 5
a Vertex b Face c Edge d
Part 6
b, d, f are parallel
Part 7
Answers 161 Oxford University Press
Part 1 a 9 + 7 7 10 8 6 2 4 3 16 10 11 9 13 15 17 14 b 15 +20 11 7 3 4 1 5 10 35 30 25 21 24 23 27 31
c 79 d 18 e 18 f 19
4
2 14 16 8 20 12
Part 8 Part 9 a 6 cm c 5 cm e 4 cm b 1 cm d 2 cm 1 Part 10 11 12 1 2 3 4 5 6 7 8 9 10 a b 11 12 1 2 3 4 5 6 7 8 9 10 c 11 12 1 2 3 4 5 6 7 8 9 10 Part 11 a Sarah b Josh c Effie UNIT 1 a 7 + 5 = 12 d 12 + 7 = 19 b 7 + 7 = 14 e 13 + 7 = 20 c 7 + 6 = 13 f 11 + 6 = 17 2 a 32 + 6 = 38 e 18 + 4 = 22 b 42 + 5 = 47 f 27 + 6 = 33 c 51 + 7 = 58 g 138 + 4 = 142 d 61 + 8 = 69 h 148 + 6 = 154 3 Hands on. 4 a Any 3 sections b Any 1 section c Any 4 sections d Any 7 sections 5 1 8, 3 8, 4 8, 7 8 6 a 2 8, 4 8, 6 8, 7 8 d 2 8, 3 8, 5 8, 6 8 b 1 8, 2 8, 5 8, 6 8 e 1 4, 2 4, 3 4, 4 4 c 1 8, 2 8, 4 8, 7 8 7 a T d T g T j F b T e T h F k T c T f T i T l T 8 Hands on. (They all equal 1 whole.) 9 a, b, c d 2 e 2 f 3 10–13 Hands on. 14 a 200 c 500 e 50 b 400 d 700 UNIT 1 The missing numbers are: a 60, 70, 80, 90 b 90, 100, 110, 120 c 53, 63, 73, 83 d 38, 48, 58, 68 e 210, 220, 230, 240 f 206, 216, 226, 236 g 180, 190, 200, 210 h 268, 278, 288, 298 10 11 2 The missing numbers are: 60 50 40 30 a 58 48 38 28 e 70 60 50 40 b 215 205 195 185 f 110 100 90 80 c 624 614 604 594 g 160 150 140 130 d 45 35 25 15 h 3 a b c 87 97 107 117 55 65 75 85 727 737 747 757 364 374 384 394 127 137 147 157 95 105 115 125 767 777 787 797 404 414 424 434 167 135 807 444 d 4 132 pencils 5 6 a out of 1 3 3 1 2 3 3 2 out of 1 3 2 3 3 3 1 3 of 6 squares 2 3 of 9 triangles 1 3 of 18 circles c out of 1 3 3 1 2 3 3 2 out of 1 3 2 3 3 3 1 3 of 6 squares 2 3 of 9 triangles 1 3 of 18 circles b out of 1 3 3 1 2 3 3 2 out of 1 3 2 3 3 3 1 3 of 6 squares 2 3 of 9 triangles 1 3 of 18 circles 7 a > d < g < b > e < h < c < f > i = 8 a Add 3 3 6 9 12 15 18 21 b Add 4 4 8 12 16 20 24 28 c Add 6 6 12 18 24 30 36 42 d Add 2 2 4 6 8 10 12 14 e Add 5 5 10 15 20 25 30 35 f Add 8 8 16 24 32 40 48 56 9–13 Hands on. UNIT 1 a 0 10 20 30 40 50 60 70 80 90 100 b 0 50 100 c 0 50 100 d 0 100 e 0 100 12 MP_NSW_SB3_38329_TXT_4PP.indb 161 29-Aug-23 15:24:39 DRAFT
11–13 Hands on. (It is less likely that two heads will be flipped because there is only one combination of two heads but two combinations for heads and tails.)
Answers 162 Oxford University Press 2 a 0 100 b 0 200 c 0 1000 d 0 2000 3 a 10 e 50 i 90 m 1 q 4 b 20 f 60 j 100 n 9 r 5 c 30 g 70 k 3 o 6 s 8 d 40 h 80 l 2 p 7 t 10 4 a 60 c 70 e 50 g 12 i 8 b 30 d 90 f 10 h 16 j 4 5 a 40 b 20 c $60 d 40 e 70 6 Hands on. 7 a, b, c Hands on. d Yes. e Shape Number of sides Number of angles Lines of symmetry 3 3 3 6 6 6 4 4 4 5 5 5 4 4 2 8 No 9 a Heads or tails. b–e Hands on. 10 Hands on.
UNIT 1 a 50 23 = 27 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 b 59 17 = 42 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 c 62 24 = 38 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 d 79 23 = 56 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 13 2 a 40 10 6 = 24 b 50 20 4 = 26 c 60 30 3 = 27 d 34 10 3 = 21 e 35 20 1 = 14 f 46 20 3 = 23 g 57 30 2 = 25 3 a 3 5 b 4 5 c 8 10 d 1 5 e 4 10 f 7 10 4 Possible solutions: a b c d e g h f 3 5 1 5 7 10 5 10 10 10 3 10 1 2 4 5 5 6 a Any star b Any circle c Any two apples 7 Hands on. 8 Hands on (arm). a 1 5 2 5 3 5 4 5 b 6 10 7 10 8 10 9 10 c 3 10 5 10 6 10 7 10 d 2 5 5 10 6 10 4 5 e 1 10 1 5 3 5 9 10 f 2 5 3 5 8 10 9 10 9 a 1 b 4 c No d 4 10–12 Hands on. UNIT 1 a 9 + 1 + 5 = 15 b 8 + 2 + 5 = 15 c 17 + 3 + 2 = 22 d 16 + 4 + 1 = 21 e 28 + 2 + 2 = 32 f 47 + 3 + 2 = 52 g 38 + 2 + 3 = 43 h 54 + 10 + 6 + 1 = 71 i 39 + 10 + 1 + 2 = 52 j 46 + 20 + 4 + 2 = 72 k 67 + 20 + 3 + 4 = 94 2 a 6 c 12 e 25 g 5 i 12 b 30 d 15 f 23 h 24 j 16 3 a True c False b False d False 4 a 2 d 6 g 4 j 5 b 4 e 3 h 4 k 5 c 5 f 4 i 3 l 5 14 5 a c b 6 a 5 b 5 c 7 d 3 7 a 4 c 8 e 18 b 8 d 12 f 16 8 Nets a and d fold to form cubes. 9 Hands on. 10 a Ants b Cicadas – Hands on (probably wrong time of year). c Hands on – Ants are numerous, and butterflies are unusual. d Flies e Hands on.
Sport Boys Girls Soccer Rugby Netball Touch Football 12 a Soccer c 2 e 4 b Netball d 1 f Hands on. UNIT 1 a 8 b 13 c 18 d 23 e 28 012345678 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 2 a 20 b 20 c 40 d 50 e 30 f 70 g 40 h 40 i 90 j 100 3 a 130 b 130 c 640 d 250 e 220 f 370 g 330 h 550 i 790 j 140 4 a 30 b 40 c 60 d 90 e 70 f 90 5 Hands on. 6 Thousands Hundreds Tens Ones a 3764 b 7569 c 3570 d 6964 e 8507 f 2643 15 MP_NSW_SB3_38329_TXT_4PP.indb 162 29-Aug-23 15:24:42 DRAFT
11
3
0, 2, 4, 6, 8, 10, 12 b 0, 3, 6, 9, 12, 15, 18 c 0, 5, 10, 15, 20, 25, 30 d 0, 4, 8, 12, 16, 20, 24 e
6, 12, 18, 24, 30, 36 f 0, 7, 14, 21, 28, 35, 42
Answers 163 Oxford University Press 7 a 95, 96, 97 e 2647, 2648, 2649 b 346, 347, 348 f 7776, 7777, 7778 c 2640, 2641, 2642 g 6942, 6943, 6944 d 3546, 3547, 3548 h 8469, 8470, 8471 8 e 1067 thousands hundreds tens ones 1 0 6 7 d 1144 thousands hundreds tens ones 1 1 4 4 c 8763 thousands hundreds tens ones 8 7 6 3 b 5694 thousands hundreds tens ones 5 6 9 4 a 3724 thousands hundreds tens ones 3 7 2 4 9 a 653 b 970 c 432 d 6531 10 a 2 4 8 16 32 64 b 3 6 12 24 48 96 c 4 8 16 32 64 128 d 5 10 20 40 80 160 e 10 20 40 80 160 320 11 a 128 64 32 16 8 4 2 b 192 96 48 24 12 6 3 c 256 128 64 32 16 8 4 d 320 160 80 40 20 10 5 e 640 320 160 80 40 20 10 12 3 a Double plus 1 Rule 1 2 3 4 5 7 9 b +8 Rule 7 12 10 8 6 6 5 4 Half plus 1 13 a 4 o’clock b Half past 1 c 20 past 6 d 10 to 6 e Quarter past 8 f Quarter to 6 g 25 past 11 14 a 7 o’clock d Quarter past 4 b Half past 10 c Quarter to 8 11 1 2 3 4 5 6 7 8 9 10 11 1 2 3 4 5 6 7 8 9 10 11 1 2 3 4 5 6 7 8 9 10 11 1 2 3 4 5 6 7 8 9 10 15 a 60 b 60 c 120 16 30 UNIT 1 a 7, 17, 27, 37, 47, 57 b 8, 18, 28, 38, 48, 58 c 12, 22, 32, 42, 52, 62 d 99, 89, 79, 69, 59 e 46, 56, 66, 76, 86 f 61, 51, 41, 31, 21 2 a 57, 67, 77, 87 b 17, 37, 57, 77 3 26 a 23 49 13 32 45 39 55 94 32 b 26 58 13 25 38 45 51 96 27 c 37 64 24 35 59 51 72 123 4 a 10 d 25 g 24 b 15 e 12 h 30 c 20 f 20 5 a b c d 4 8 6 14 35 2 3 2 7 2 7 5 2 1 3 5 2 4 7 6 8 9 2 6 10 4 8 14 12 16 18 5 15 25 10 20 35 30 40 45 10 30 50 20 40 70 60 80 90 5 1 3 5 2 4 7 6 8 9 10 1 3 5 2 4 7 6 8 9 6 a b c 4 8 6 14 35 2 3 2 7 2 7 5 2 1 3 5 2 4 7 6 8 9 2 6 10 4 8 14 12 16 18 5 15 25 10 20 35 30 40 45 10 30 50 20 40 70 60 80 90 5 1 3 5 2 4 7 6 8 9 10 1 3 5 2 4 7 6 8 9 7–12 Hands on. 13 a 48 kg b 35 kg c 30 kg UNIT 1 a 79 b 65 c 82 d 63 e 59 f 53 g 159 56 76 77 78 79 43 63 64 65 49 79 80 81 82 63 64 65 66 86 59 60 61 91 22 52 53 159 160 161 162 192 2 Hands on. 16 17 3 3 × 4 = 12 4 × 3 = 12 12 ÷ 3 = 4 12 ÷ 4 = 3 a 3 × 5 = 15 5 × 3 = 15 15 ÷ 5 = 3 15 ÷ 3 = 5 d 6 × 4 = 24 4 × 6 = 24 24 ÷ 4 = 6 24 ÷ 6 = 4 b 6 × 5 = 30 5 × 6 = 30 30 ÷ 6 = 5 30 ÷ 5 = 6 e 8 × 4 = 32 4 × 8 = 32 32 ÷ 8 = 4 32 ÷ 4 = 8 c 5 × 6 = 30 6 × 5 = 30 30 ÷ 6 = 5 30 ÷ 5 = 6 f 4 a 16 ÷ 4 = 4 b 12 ÷ 4 = 3 c 15 ÷ 5 = 3 5 a Tom’s house b Hands on. c Mason’s house d Billy Goat Rd and South St e Hands on. 6 a No b Treasure Town c Happy City d Train or Boat or Road or Walking e Yes 7 a 18 cm c 18 cm e 28 cm b 26 cm d 18 cm 8 Hands on. UNIT 1 a 61 c 85 e 36 g 31 i 61 b 54 d 44 f 70 h 92 j 73 2 a 4 + 6 + 5 = 15 b 8 + 2 + 7 = 17 c 6 + 4 + 9 = 19 d 7 + 3 + 6 = 16 e 5 + 5 + 9 = 19 f 15 + 5 + 7 = 27 g 16 + 4 + 9 = 29 h 17 + 3 + 8 = 28 i 14 + 6 + 7 = 27 j 18 + 2 + 9 = 29 k 9 + 1 + 7 + 3 = 20 l 8 + 2 + 5 + 7 = 22 m 7 + 3 + 5 + 4 = 19
19 + 1 + 6 + 4 = 30
25
5
6
4 = 40
n
o
+
+
+
18 MP_NSW_SB3_38329_TXT_4PP.indb 163 29-Aug-23 15:24:44 DRAFT
a
0,
4 Rule and missing numbers:
a 24 28 32 36 Add 4
b 19 22 25 28 Add 3
c 34 38 42 46 Add 4
d 50 55 60 65 Add 5
5 Hands on.
b
am a prism that has two triangles as faces and three other rectangular faces. am a prism that has six rectangular faces. am a special kind of prism because all my faces are squares.
e
I am a prism that has two hexagonal faces and six other rectangular faces. am a prism that has two octagonal faces and eight other rectangular faces.
8 Hands on – some examples below.
a A cube has 6 square faces, 8 vertices and 12 edges.
b A rectangular prism has 6 faces, 8 vertices and 12 edges.
c A triangular prism has 2 triangular faces, 3 rectangular faces, 9 edges and 6 vertices.
d A hexagonal prism has 2 hexagonal faces, 6 rectangular faces, 12 vertices and 18 edges.
9 a 40 cm2 b 36 cm2
10 Hands on.
11 Possible solutions:
3 cm × 8 cm, 4 cm × 6 cm
DIAGNOSTIC REVIEW
1 The missing numbers are:
164 Oxford University Press
Answers
6
7
4
23
a
+
11 15 19
27 31 35 39
8
12
=
=
=
=
=
=
=
= 52
6 + 7 13 20 27 34 41 48 55 62
3
=
=
=
=
9
7 a c
+ 6 14 20 26 32 38 44 50 56 c
+ 5
17
22
27
32
37
42
47
d
e
+ 9 = 12 = 21
30
39
48
57 = 66 = 75 f
+ 8 17 25 33 41 49 57 65 73
b d
Part 1 a 50 60 70 80 90 100 110 b 32 42 52 62 72 82 92 c 80 70 60 50 40 30 20 d 97 87 77 67 57 47 37 Part 2 a 6 d 5 g 4 i 14 b 6 e 12 h 16 j 10 c 8 f 7 Part 3 a 9 + 5 becomes 9 + 1 + 4 = 14
17 + 4 becomes 17
3 + 1
21
26
7
26
33
77
8
77
85 2 Part 4 a 24 c 32 e 24 b 60 d 35 f 45 Part 5 a 1 2 b 1 4 c 1 8 d 2 5 e 1 3 Part 6 a 4 b 6 c 20 ÷ 5 = 4 Part 7 a 40 b 20 c 70 d 140 e 400 f 300 g 400 h 600 Part 8 a quarter past 11 b quarter to 2 c 11 12 1 2 3 4 5 6 7 8 9 10 d 11 12 1 2 3 4 5 6 7 8 9 10 Part 9 a 8 cm2 b 16 cm2 Part 10
HONEY Part 11 a b c Part 12 Part 13 a 10 cm b 10 cm a a right angle b less than a right angle Part 14 Part 15
Hands on. UNIT
b
+
=
c
+
becomes
+ 4 + 3 =
d
+
becomes
+ 3 + 5 =
SULTANAS SULTANAS
equally likely
a 130, 140, 150 e 530, 630, 730 b 420, 520, 620 f 601, 701, 801 c 505, 605, 705 g 399, 499, 599 d 470, 480, 490 h 358, 458, 558 2 a 100, 200, 300, 400, 500, 600, 700 b 85, 95, 105, 115, 125, 135, 145 c 3800, 3810, 3820, 3830, 3840, 3850, 3860 d 3127, 3227, 3327, 3427, 3527, 3627, 3727 3 a 770, 760, 750 e 250, 150, 50 b 620, 520, 420 f 699, 599, 499 c 550, 450, 350 g 4501, 4401, 4301 d 769, 759, 749 h 3335, 3235, 3135 4 Digits 3 9 5 5 6 1 2 8 3 4 8 6 7 9 Largest 953 651 8432 9876 Smallest 359 156 2348 6789 5 Hands on. 6 a 9 c 12 e 15 g 24 i 0 b 6 d 3 f 18 h 21 j 27 7 × 1 4 10 9 5 7 6 8 3 2 0 a 2 2 8 20 18 10 14 12 16 6 4 0 b 4 4 16 40 36 20 28 24 32 12 8 0 c 3 3 12 30 27 15 21 18 24 9 6 0 d 5 5 20 50 45 25 35 30 40 15 10 0 8 a $21 b 24 c 15 d $2 9 48 10 Hands on. 11 a d g b e h c f i Reflect Reflect Rotate Rotate Translate/Reflect Rotate Translate Reflect Reflect 12 13 14–16 Hands on. 19 MP_NSW_SB3_38329_TXT_4PP.indb 164 29-Aug-23 15:24:47
DRAFT
2 a $20 d $15 g $11 j $22

b $12 e $40 h $20
c $10 f $26 i $40
3 Hands on.
4 a 70 + 23 = 93
b 40 + 43 = 83
c 50 + 37 = 87
d 70 + 30 = 100
e 80 + 34 = 114
f 90 + 19 = 109
g 120 + 23 = 143
h 130 + 40 = 170
i 140 + 35 = 175
j 150 + 35 = 185
5 a 85 – 30 = 55
b 79 – 40 = 39
c 96 – 50 = 46




d 148 – 40 = 108
e 176 – 60 = 116
f 129 – 40 = 89

6 a B3 = e E2 = i A5 =
b B2 = f F1 = j D2 =
c C3 = g G4 =
d E5 = h A3 =
7 a = C4 c = D3 e = B5
b = G3 d = F4
Answers 165 Oxford University Press 17 Blue Blue Blue Yellow Blue Red Yellow Blue Yellow Yellow Yellow Red UNIT 1 a 56 b 28 c 7 d 48 e 66 f 21 g 9 h 9 i 9 j 4 k 6 l 3 2 a 5 7 1 3 4 4 c 9 5 6 7 2 8 e 7 2 2 4 4 8 b 8 8 2 3 6 5 d 7 7 5 4 2 3 3 a 12 ÷ 2 = 6 or 12 ÷ 6 = 2 b 18 ÷ 9 = 2 or 18 ÷ 2 = 9 c 20 ÷ 4 = 5 or 20 ÷ 5 = 4 d 16 ÷ 4 = 4 e 15 ÷ 3 = 5 or 15 ÷ 5 = 3 f 35 ÷ 5 = 7 or 35 ÷ 7 = 5 g 24 ÷ 4 = 6 or 24 ÷ 6 = 4 h 30 ÷ 6 = 5 or 30 ÷ 5 = 6 4 Hands on. 5 a 12 e 3 i 8 m 21 b 20 f 4 j 9 n 45 c 16 g 6 k 2 o 42 d 32 h 7 l 5 p 27 4 7 21 45 2 6 Winning card C 20 27 6 a B, F b C, E c A, D 7 Hands on. 8 Hands on. 9 a 120 mm c 70 mm b 60 mm d 40 mm 10 Hands on. 11 a 20 b 30 c 100 d 90 e 5 12 a 100 b 200 c 800 d 400 e 50 UNIT 1 a 379 b 697 c 757 d 788 e 729 2 a 662 b 582 c 966 d 971 e 835 f 814 g 737 h 672 i 984 j 695 3–4 Hands on. 5 Total change Pencil $3.50 $1.50 Scissors $4.20 $0.80 Eraser $1.40 $3.60 Ruler $2.80 $2.20 Tape $1.75 $3.25 20 21 6 a $1.40 c $2.30 e $4.20 b $2.20 d $1.50 7 a $68 b $99 c $120 d $158 e $189 f $301 g $360 h $506 i $378 8–9 Hands on. 10 a 83 L b 18 L 11 a 40 b 10 c 4 d 5 12 Hands on. UNIT 1 a 319 b 115 c 225 d 528 e 316 f 315 g 226 h 478 i 247 j 129 k 528 l 235 m 819 n 417 o 636 2 a $9 b $13 c $63 d $86 e $72 f $73 3 a 4 b 6 c 2 d 2 4 a 4 b 2 c 2 5 a 6 b 6 c 20 d 3 6 a 2 b 5 c 5 d 3 7 a 1 4 b 1 2 c 1 4 d 1 2 e 3 4 f 1 2 g 1 4 h 3 4 i 1 4 j 1 2 k 3 4 l 1 full rotation 8 a b c d 12 6 1 11 5 7 12 6 1 11 5 7 12 6 11 7 12 6 1 11 5 7 20 past 3 a quarter past 12 10 past 9 20 to 7 9 a b c d 9 3 2 10 8 3 2 10 4 9 10 4 8 9 3 2 10 4 Twelve ten and twenty seconds Twelve twentyfive and five seconds Twelve twenty and fifteen seconds Ten twenty and thirtyfive seconds 10 Hands on. UNIT 1 a 0, 10, 20, 15, 25, 5, 30, 45, 35, 40, 50 b 0, 4, 8, 6, 10, 2, 12, 18, 14, 16, 20 c 0, 20, 40, 30, 50, 10, 60, 90, 70, 80, 100 d 0, 0, 0, 0, 0, 0, 0, 0, 0, 0 e 0, 8, 16, 12, 20, 4, 24, 36, 28, 32, 40 f 0, 2, 4, 3, 5, 1, 6, 9, 7, 8, 10 22 23
8 5 4 3 2 1 A B C D E F G 9 G2 C2 E5 G4 A4 F1
Red White Blue
Yellow Orange 11 a Red and white d 3
Blue e Hands on.
Pink f (No) Hands on. MP_NSW_SB3_38329_TXT_4PP.indb 165 29-Aug-23 15:24:52
10
Pink
b
c
DRAFT
Favourite T-shirts
4 a Hands on – James has not folded his paper into 5 equal parts. b Hands on – 1 5 is larger than 1 10 because the whole has only been divided into 5 pieces not 10.
d 46 + 30 2 = 74
e 27 + 50 1 = 76
f 35 + 50 2 = 83
g 56 + 30 3 = 83
h 63 + 30 2 = 91
j
Answers 166 Oxford University Press 12
T-shirt colours Number of T-shirts 1 2 3 4 5 6 7 8 UNIT
1 a 26 + 40 2 = 64 b 34 + 30 3 = 61 c 28 + 40 1 = 67
i 47 + 50 1 = 96
e
f ✔ m ✔ g
(17) n × (56) 3 a 6 c 10 e 2 g 4 b 4 d 5 f 6 h 5 4 a 2 c $4 e $6 g 10 b 5 d 3 f 8 5 1. 8 4. 4 7. 7 10. 10 13. 5 2. 9 5. 3 8. 9 11. 1 3. 5 6. 6 9. 2 12. 10 G 1 R 2 E 3 A 4 T 5 W6 O 7 R 8 K 9 D10 U11 D12 E13 6–10 Hands on. 11 a 2 kg b 6 kg c 5 kg d 3 kg
Hands on.
Hands on. (Masses of 1 kg can be different shapes and sizes.) UNIT 1 a 16 km b 25 km c Hands on. 2 a 145 b 129 c 150 d 170 L 3 a 1 –2 1 –8 3 –8 6 –8 7 –8 1 –4 4 –8 3 –4 0 1 b 1 –2 1 –10 2 –5 6 –10 4 –5 4 –10 5 –10 3 –5 0 1 24 25 8 Shape Sides Angles a Octagon 8 8 b Rectangle 4 4 c Octagon 8 8 d Hexagon 6 6 e Hexagon 6 6 f Octagon 8 8 9 4 5 6 1 2 3 4 5 6 7 8 1 2 3 4 3 2 1 8 7 6 5 1 2 3 4 5 1 2 3 4 5 6 7 8 1 2 3 4 5 6 Octagon Pentagon Octagon Hexagon Hexagon Octagon a b c d e f 10 a 34 b 27 c 36 11 a Centicubes b Discussion:
and
no spaces between them. 12 a 4 c 4 e 12 g 8 b 6 d 8 f 10 h 16
Hands on. UNIT
a 60 d 160 g 60 j 120 b 100 e 90 h 150 k 200 c 120 f 120 i 180 l 150 2 a 100 c 200 e 180 g 240 b 250 d 140 f 300 h 300 3 Hands on. 4 a 3 b 5 5 a 5 b 4 c 2 d 10 6 a 4 e 4 i 3 m 7 q 8 b 6 f 3 j 9 n 5 r 6 c 8 g 2 k 10 o 4 d 10 h 5 l 9 p 6 7 Hands on. 8 a Joe b Than c Mia d Con 27
76 + 40 3 = 113 2 a ✔ h × (99) b ✔ i × (25) c × (37) j ✔ d × (82) k × (58)
× (36) l ✔
×
12
13
They stack
there are
13
1
5 a 3 4 0 1 b 3 8 0 1 c 1 3 0 1 d 7 10 0 1 e 1 5 0 1 6 a R b T c T d K e T f K g R h R i R j R k T l R m K n T 7–8 Hands on. 9 Yes 10 It is equal to 1 m2 because 2 m × 1 2 m = 1 m2 UNIT 1 a 394 b 174 c 193 d 81 e 282 f 286 g 71 h 472 i 91 j 392 2 6 7 9 4 3 6 2 4 3 a 7 6 8 4 4 6 3 2 2 c 4 7 6 5 3 2 9 4 3 e 7 8 6 2 2 5 5 6 1 b 2 9 5 4 3 2 8 6 2 d Tens Hund Ones Tens Hund Ones Tens Hund Ones Tens Hund Ones Tens Hund Ones 3 a $332 b $151 c $202 d $94 4 a 2534 4 ones 3 tens 5 hundreds 2 7 6 3 1 6 5 4 2 1 2 7 3 9 9 6 4 1 9 3 6 thousands ones tens hundreds thousands b 1367 4 ones 3 tens 5 hundreds 2 thousands ones tens hundreds thousands c 2456 4 ones 3 tens 5 hundreds 2 thousands ones tens hundreds thousands d 3721 4 ones 3 tens 5 hundreds 2 thousands ones tens hundreds thousands e 4699 4 ones 3 tens 5 hundreds 2 thousands ones tens hundreds thousands f 6391 4 ones 3 tens 5 hundreds 2 thousands ones tens hundreds thousands 5 a tens d thousands g thousands b hundreds e tens h hundreds c ones f ones i thousands 6 a 4000 + 500 + 20 + 7 b 5000 + 400 + 30 + 6 c 6000 + 700 + 40 + 8 d 6000 + 700 + 40 e 8000 + 400 + 7 f 9000 + 900 + 30 + 6 7 a 8507, 7503, 5073, 3057 b 3658, 2645, 2500, 1999 c 8436, 3541, 2907, 2657 d 5234, 4532, 3524, 2453 e 2745, 1438, 837, 238 26 MP_NSW_SB3_38329_TXT_4PP.indb 166 29-Aug-23 15:24:54
DRAFT
5 Each pair of sentences has the same total. They are:
8
on. (It doesn’t matter in which order they are multiplied.)
9 Mitchell and Georgia both scored 24. 10
Answers 167 Oxford University Press 4 a ✔ c ✔ e ✔ b ✔ d �
(37)
a 10,
13,
Hands on. 7 a 3 × 4 = 12 4 × 3 = 12 b 5 × 2 = 10 2 × 5 = 10 c 7 × 2 = 14 2 × 7 = 14 d 6 × 5 = 30 5 × 6 = 30 e 6 × 3 = 18 3 × 6 = 18
10 c 16, 16 e 20, 20 g 24, 24 b
13 d 22, 22 f 23, 23 6
Hands
on.
Hands on. 13
on. 14 a 12 b 8 c 6 d 4 e 3 UNIT
a 638 b 819 c 629 d 608 e 924 f 614 g 968 h 626 i 817 j 656 2 a 730 b 736 c 873 d 731 e 831 f 436 g 676 h 446 i 863 j 462 3 a $2.75 b $5.90 c $9.24 d $7.14 4 a 24 ÷ 3 = 8 c 24 ÷ 2 = 12 b 24 ÷ 6 = 4 d 24 ÷ 4 = 6 5 a 6 d 5 g 5 b 4 e 6 h 6 c 6 f 5 i 8 6 29 Part 7 a b Part 8 a b c d Part 9 x x y y x x y y Part 10 a 70 mm b 30 mm Part 11 a 12 b 10 c 10 Part 12 Unlikely UNIT 1 a 31 correct d 24 correct b 57 wrong e 17 correct c 38 correct
She forgot to take one off the number in the tens column. 3 a � = $48 c ✔ b ✔ d � = $175 28 9 a, b, d Bill Ave Brown St Victoria St A 1 2 3 B C D E F G H I Yuk St Collins St Green St Phillip St SHOPPING CENTRE CAR PARK SMITH PARK GREEN PARK Milk Rd Jill St Hook Rd Hope St Hoot St Dolphin St Red St School Rd SCHOOL Jill Sam Sue Arun Ben Mia Joe Con Than c Sue’s house e Hands on. 10 a Yes b East c West 11 a 10 b 2 c 3 d 4 e 1 12 a Yes b No c Yes 13 a Likely or maybe b Unlikely or maybe c Unlikely d Never 14 Hands on. Possible combinations: 7 green 2 blue 1 red 6 green 3 blue 1 red 5 green 3 blue 2 red DIAGNOSTIC REVIEW Part 1 a 987 d 267 g 76 b 412 e 74 h 83 c 473 f 73 i 64 Part 2 a 15 d 32 g 30 j 18 b 35 e 45 h 27 k 30 c 24 f 9 i 70 Part 3 a 5 b 4 c 4 d 4 e 5 Part 4 b c thousands hundreds tens ones 3 5 0 5 a thousands hundreds tens ones 6 2 7 4 3623 3624 3625 d 2072 2073 2074 e 7987 7988 7989 Part 5 a 110, 120 c 2615, 2715 b 235, 245 d 6550, 7550 Part 6 a 12 b 8 c 6 3 MP_NSW_SB3_38329_TXT_4PP.indb 167 29-Aug-23 15:24:56 DRAFT
Hands on. 11 Hands
12
Hands
1
2
2 a hundreds b ones
c thousands d hundreds
e ones f ten thousands
g hundreds h thousands
3 a 2000 + 300 + 40 + 7
b 3000 + 500 + 70 + 8
c 6000 + 300 + 50 + 4
d 30000 + 5000 + 600 + 70 + 2
e 70000 + 4000 + 200 + 60 + 3 4
2 Missing fractions:
a 5 8, 6 8, 7 8 c 5 4, 6 4, 7 4
b 5 10 , 6 10 , 7 10 d 5 5, 6 5, 7 5
3 12 22 32 42 52 62 72 82 92
What did you learn? Hands on.
4 13 23 33 43 53 63 73 83 93
What did you learn? Hands on.
5 Hands on.
6 a 55 – 32 = 23
55 – 23 = 32
b 64 – 42 = 22
64 – 22 = 42
c 77 – 62 = 15
77 – 15 = 62
d 67 – 44 = 23
67 – 23 = 44
e 52 – 39 = 13
52 – 13 = 39
f 72 – 44 = 28
72 – 28 = 44
g 65 – 39 = 26
65 – 26 = 39
h 60 – 45 = 15
60 – 15 = 45
i 199 – 106 = 93 199 – 93 = 106
j 192 – 103 = 89 192 – 89 = 103
7 a b c d e f g h i j k
8 Hands on.
9 a 8 cm3
b 12 cm3
c 16 cm3
10 a 11
I am a shape that has 4 right angles and 4 sides the same length. am drawn with my base on a different orientation.
I am a shape that has 4 right angles.
I have 2 shorter sides the same length and 2 longer sides the same length.
I am a 3-sided shape. have 3 angles the same size and 3 sides the same length.
I am an irregular shape that has 5 sides. My angles and side lengths are not always the same size.
I am a regular shape with 6 sides. All my angles are the same size. All my sides are the same length.
I am a 3-sided shape. have 2 angles the same size and 2 sides the same length.
I am a regular shape that has 5 sides. All my angles are the same size. All my sides are the same length.
I am a regular shape with 8 sides. All my angles are the same size. All my sides are the same length.
I am a quadrilateral. have one pair of parallel sides only.
I am a quadrilateral. None of my angles are the same size, all my side lengths are different. None of my sides are parallel.
I am an irregular shape that has 8 sides. My angles and side lengths are not always the same size.
d 10 cm3
g 12 cm3
e 12 cm3 h 10 cm3
f 12 cm3
c 12 b, e, f, g
168 Oxford
Press
Answers
University
UNIT
276, 277, 357, 589 b 307, 2089, 3544, 3546 c 32 501, 32 525, 32 579, 32 999 d 33 506, 34 506, 35 506, 60 533 e 9999, 27963, 34 006, 35 902 5 a 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 30 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 b c Hands on. (The pattern, of 3 is part of the pattern of 6.) 6 a 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 30 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 b c Hands on. (Diagonals, straight line) 7 Hands on. 8 a 72 b 80, 88, 96 c 104, 112, 120, 128 9–11 Hands on. 12 a 1 b 4 c 5 d 2 e 3 13 a 2 b 5 c 10 14 850 g UNIT 1 Missing numbers and 10th term: a 20, 16, 12 4 c 48, 56, 64 80 b 42, 49, 56 70 d 54, 63, 72 90 31 1 Number Ten thousands Thousands Hundreds Tens Ones a 435 4 3 5 b 3568 3 5 6 8 c 2079 2 0 7 9 d 35 674 3 5 6 7 4 e 56 759 5 6 7 5 9 f 25 807 2 5 8 0 7 32 7 8 a C, D, F, G b A, H c B, E d No 9 a False c True e True g False b False d False f True 10 Y Y Y G R R P P P P 11 a Red, black or blue b No c There are twice as many blue lollies as black. UNIT 1 a 8779 d 3874 g 6086 j 8944 b 8699 e 6684 h 5886 k 7854 c 7768 f 9483 i 5823 l 6777 2 a 725 c 1117 e 1437 b 1325 d 1380 f 1765 3 Hands on. 4 a 1 4 3 4 and b 2 3 1 3 and c 3 8 5 8 and d 2 5 3 5 and 5 a 1 4 b 1 3 c 1 8 d 1 5 e 1 10 6 a b c d 4 4 4 4 5 5 3 3 = 1 = 1 = 1 = 1 7 a 45 – 20 = 25 b 37 – 30 = 7 c 65 – 40 = 25 d 49 – 30 = 19 e 73 – 30 = 43 f 67 – 30 = 37 g 78 – 40 = 38 h 173 – 30 = 143 i 165 – 40 = 125 j 265 – 50 = 215 8 a 50 b 40 c 70 d 20 e 40 f 60 g 50 h 20 i 130 j 210 9 Hands on. 10 a No b Square and rhombus 30 MP_NSW_SB3_38329_TXT_4PP.indb 168 29-Aug-23 15:24:59 DRAFT
a
Answers 169 Oxford University Press Part 2 a $30 c $28 e 5 g 5 i 6 b $30 d $18 f 6 h 8 j 7 Part 3 a 4000 + 200 + 30 + 5 b 5000 + 300 + 60 + 2 c 6000 + 200 + 80 + 9 d 7000 + 500 + 60 + 3 e 36, 676, 765, 3576, 5736 Part 4 Missing numbers are: a 3 c 20 e 6 b 4 d 4 f 5 Part 5 a 0.57 b 0.44 c 0.95 Part 6 a $274 b 7 marbles c 128 pegs Part 7 Hands on. Part 8 a b c d e f Part 9 a–e N W E S f triangle Part 10 a 9 cm2 b 12 cm2 c 8 cm2 d 9 cm2 Part 11 a 1.25 m b 2.37 m c 6.49 m Part 12 a 11 12 1 2 3 4 5 6 7 8 9 10 b 11 12 1 2 3 4 5 6 7 8 9 10 UNIT 1 a + g +, + b h +, c + i +, d − j , − e k , f + l +, − or , + 2 a $10 c $13 e $109 b $23 d $122 f $436 3 a $160 d $120 g $12 j $40 b $51 e $140 h $30 c $620 f $26 i $132 4 Hands on. 5 a 6 d 10 g 15 j 7 m 25 b 17 e 7 h 15 k 4 n 26 c 8 f 7 i 6 l 50 o 16 6 a + d g +, + j ×, + b × e + h +, k ×, × c × f i ×, + l ×, + 7 a 3 2 1 5 4 b 1 5 2 3 4 c 1 5 4 2 3 8 a B, C, F b G, K, L, M c H 9 Hands on. 10 a 6 cm2 f 8 cm2 b 8 cm2 g 15 cm2 c 21 cm2 h 26 cm2 d 16 cm2 i 36 cm2 e 24 cm2 j between 16 and 17 cm2 11 Hands on 12 Discussion: Multiply one side by the other side. DIAGNOSTIC REVIEW Part 1 a 559 b 985 c 7914 d 338 e 119 f 3142 35 4 UNIT 1 a 6431 b 1804 c 2233 d 4326 e 4730 f 3010 g 3235 h 5811 i 3491 j 2254 k 3121 l 2121 2 a $413 b $1243 3 a 15, 15 c 20, 20 e 24, 24 b 18, 18 d 19, 19 f 20, 20 4 Hands on. 5 a 6 + 4 + 8 = 18 d 15 + 5 + 9 = 29 b 17 + 3 + 9 = 29 e 4 + 6 + 17 = 27 c 18 + 2 + 7 = 27 f 5 × 2 × 7 = 70 6 They both make 40 cakes. 7 a 36 tens, 7 ones b 50 tens, 7 ones c 69 tens, 0 ones d 85 tens, 7 ones and 857 ones e 35 hundreds, 7 tens, 6 ones and 357 tens, 6 ones and 3576 ones f 25 hundreds, 4 tens, 3 ones and 254 tens, 3 ones and 2543 ones 8 a 37 b 56
10–12 Hands on. UNIT 1 a 27 100 , 0.27 b 47 100 , 0.47 c 63 100 , 0.63 d 84 100 , 0.84 e 42 100 , 0.42 2 a 0.25 b 0.35 c 0.52 d 0.65 e 0.84 f 0.96 3 0 10 20 30 40 50 60 70 80 90 100 r ulex metre r uler a 0.15 m b 0.35 m c 0.42 m d 0.62 m e 0.84 m f 0.79 m 4 0.15, 0.35, 0.42, 0.62, 0.79, 0.84 5 a 8 c 20 e 24 g 36 i 40 b 12 d 16 f 28 h 32 j 4 6 a 18 c 12 e 60 g 42 b 30 d 24 f 54 h 36 7 a 0, 6, 12, 18, 24, 30, 36, 42, 48, 54, 60 b 0, 7, 14, 21, 28, 35, 42, 49, 56, 63, 70 c 0, 8, 16, 24, 32, 40, 48, 56, 64, 72, 80 d 0, 9, 18, 27, 36, 45, 54, 63, 72, 81, 90 8 a 60 c 21 e 49 g 40 b 48 d 35 f 63 h 54 9–11 Hands on. 12 a cm c cm e m b m d cm f m 13 a 1.65 m e 2.56 m i 2.98 m b 2.75 m f 4.45 m j 5.42 m c 3.45 m g 6.32 m d 1.27 m h 3.78 m 33 34 MP_NSW_SB3_38329_TXT_4PP.indb 169 29-Aug-23 15:25:00 DRAFT
c 72 d 80 e 29 9 a 35 b 27 c 36 d 85 e 38 f 56
MP_NSW_SB3_38329_TXT_4PP.indb 170 29-Aug-23 15:25:00
DRAFT




































































































































