Maths Plus NSW Student Book 4 Full Digital Sample

STUDENT BOOK

Harry O’Brien

Greg Purcell

The Maths Plus NSW Syllabus/Australian Curriculum series, Year K to Year 6, is based on the NSW Education Standards Authority 2023 Mathematics K–6 Syllabus for the Australian Curriculum Mathematics (ACARA). Each book after Year K builds upon prior knowledge and works towards an understanding of the achievement standards for the relevant year level and beyond. Maths Plus provides students with opportunities to sequentially develop their skills and knowledge in the three strands of the Australian Curriculum Mathematics: Number, Algebra, Measurement, Space, Statistics and Probability

Series components

Student Books

Work towards achieving the relevant outcomes by developing skills and competency in understanding mathematical structures, fluency, reasoning and problem solving.

Mentals and Homework Books

Provide concise, essential revision and consolidation activities that correspond with the concepts and units of work presented in the Student Books.

Assessment Books

Include short post-tests with a simple marking system to assess students’ skills and understanding of the concepts in the Student Books.

Student Book features

• All pages are colour coded.

• Australian Curriculum Mathematics content descriptions, proficiency strand references and general capabilities appear on each page.

• The Dictionary (Years 2 to 6) features clear and simple explanations of mathematical terms and language.

Diagnostic term reviews

• Diagnostic term reviews (Years 1 to 6) assist in pinpointing students’ strengths and weaknesses, allowing intervention and re-teaching opportunities where required.

• The Find a topic page allows teachers the freedom to address particular topics and student needs as appropriate, providing essential revision and consolidation opportunities.

Teacher Book and Teacher Dashboard

Provide access to a wealth of resources and support material:

• curricula and planning documents

• interactive teaching tools

• potential difficulties videos

• learning activities

• support and extension activities

• reflection

www.oxfordowl.com.au

Oxford Owl is the home for Oxford Primary professional resources.

• blackline masters and investigation pages

• links to Advanced Primary Maths (Years 3 to 6)

• assessment tests

• answers for student resources

MEASUREMENT AND SPACE

NSW Syllabus Outcomes

Representing numbers using place value

MA2-RN-01 Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands

MA2-RN-02 Represents and compares decimals up to 2 decimal places using place value

Additive relations

MA2-AR-01 Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers

MA2-AR-02 Completes number sentences involving addition and subtraction by finding missing values

Multiplicative relations

MA2-MR-01 Represents and uses the structure of multiplicative relations to 10 x 10 to solve problems

MA2-MR-02 Completes number sentences involving multiplication and division by finding missing values

Partitioned fractions

MA2-PF-01 Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)

MEASUREMENT AND SPACE

Geometric measure

MA2-GM-01 Uses grid maps and directional language to locate positions and follow routes

MA2-GM-02 Measures and estimates lengths in metres, centimetres and millimetres

MA2-GM-03 Identifies angles and classifies them by comparing to a right angle

Two-dimensional spatial structure

MA2-2DS-01 Compares two-dimensional shapes and describes their features

MA2-2DS-02 Performs transformations by combining and splitting two-dimensional shapes

MA2-2DS-03 Estimates, measures and compares areas using square centimetres and square metres

Three-dimensional spacial structure

DRAFT

MA2-3DS-01 Makes and sketches models and nets of three-dimensional objects including prisms and pyramids

MA2-3DS-02 Estimates, measures and compares capacities (internal volumes) using litres, millilitres and volumes using cubic centimetres

Non-spatial measure

MA2-NSM-01 Estimates, measures and compares the masses of objects using kilograms and grams

MA2-NSM-02 Represents and interprets analog and digital time in hours, minutes and seconds

STATISTICS AND PROBABILITY

Data

MA2-DATA-01 Collects discrete data and constructs graphs using a given scale

MA2-DATA-02 Interprets data in tables, dot plots and column graphs

Chance

MA2-CHAN-01 Records and compares the results of chance experiments

MA0-WM-01 working mathematically

Develops understanding and fluency in mathematics through exploring and connecting mathematical concepts, choosing and applying mathematical techniques to solve problems, and communicating their thinking and reasoning coherently and clearly

Representing numbers using place value

Additive relations

Multiplicative relations

Partitioned fractions

Geometric measure

Two-dimensional spatial structure

Three-dimensional spacial structure

DRAFT

Non-spatial measure

STATISTICS AND PROBABILITY

Data

Chance

MA0-WM-01 working mathematically

Extending addition facts

We know that 8 + 6 = 14, so 8 tens plus 6 tens must equal 140.

b Josh has one bag of 50 potatoes and another of 70 potatoes. How many potatoes does he have altogether?

c Hans has $60 in one bank account and $80 in another. How much money does Hans have in total in both accounts?

d Mary had $150 but spent $60. How much does she have left?

e Zara had 180 books but lost 70. How many does she have left?

Multiplication facts, times 3

Write a multiplication fact to describe each array.

Skip count to complete the table of threes and other related facts. a

Use the multiplication facts of 3 to answer the divisions.

To solve 21 ÷ 3 think 3 × = 21

Write a problem to match this number sentence: 8 × 3 = 24.

Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values

Revision of three-dimensional objects

Prisms have two parallel faces that are congruent. All the other faces on a prism are rectangular if the faces are square to the ends.

vertex base

Place the letters in the correct position on the grid to identify the cylinders, cones, spheres, prisms and pyramids.

A B C E G U Z H N

DRAFT

Cylinders Cones Spheres Prisms

Pyramids

Find two items in your school that are:

a prisms

8 9 10

b cylinders

c cones

BLOCK

d spheres

Explain why this object is a prism.

Makes and sketches models and nets of three-dimensional objects including prisms and pyramids

Length can be measured in centimetres. A centimetre is one-hundredth of a metre.

Use a ruler to measure the length of these lines in centimetres.

How many centimetres are in a metre ruler?

and measure the length of each pencil.

Convert each metre measurement into centimetres.

2- and 3-digit subtraction

Learning

4 ones from 2 ones can’t be done. Trade a ten from the tens column to the ones column to make 12 ones. 6 tens becomes 5 tens. 4 ones from 12 ones equals 8 ones.

Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

3 Write how many pieces of each Base 10 material would be needed to make the numbers, then record the numbers on the numeral expanders.

4 Write the place value of each bold number. thousands, hundreds, tens, ones

5 Write the number one less and one more than the shaded number on each line.

6 Order the following numbers from smallest to largest.

a 46, 247, 56, 474

b 357, 323, 531, 784

c 2374, 7423, 3724, 2743

d 2701, 2671, 2761, 3017

e 8603, 3806, 6803, 6380

Decide whether each shape has symmetry by answering yes or no, then draw as many lines of symmetry as you can on the shapes. The first one has been done for you.

DRAFT

Draw the other half of each shape by using its line of symmetry as a starting point.

Compares two-dimensional shapes and describes their features Performs transformations by combining and splitting two-dimensional shapes

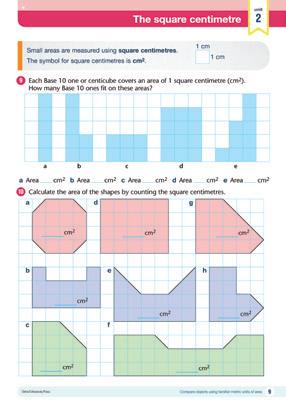
Small areas are measured using square centimetres

1 cm 1 cm 1 square centimetre

DRAFT

2- and 3-digit addition

9 ones plus 3 ones equals 12 ones. Exchange 10 ones for 1 ten. Record 2 in the ones column. Add 1 ten + 3 tens plus 1 ten equals 5 tens.

1 Complete each addition algorithm.

a Space City to Launch Pad.

b Space City to Moon Town.

c Rocket Hill to Blast Off.

d Centre Town to Launch Pad.

e Rocket Hill to Retro City.

f Launch Pad to Rocket Hill.

g Moon Town to Launch Pad.

h Blast Off to Space City.

3 km

Make up a journey on the map and record its distance.

Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

2 Calculate the shortest distance from:

Terms in number patterns

4 Continue the patterns that are modelled by the dice until the seventh term. Then record what you think would be the tenth term in the patterns. a Tenth term

3 6 9 12

Tenth term

4 8 12 16

Tenth term

5 10 15 20

Tenth term

6 12 18 24

5 Complete the number patterns then write a rule for each.

c 21 31 41 51

6

Write two number patterns of your own.

7 Understanding position.

a Which book is on the far right of the top row?

b Which book is in the centre of the bottom row?

c Which book is second from the left in the middle row?

d Which book is fourth from the right in the bottom row?

e Which book is in the centre of the bookshelf?

f Which book is in the top row and third from the right?

g Describe the position of book D.

8 What shapes can be found at these grid references?

Book Y is in the middle row, second from the right.

Ms Wills graphed the number of times her best spellers achieved no mistakes in spelling during Term 1.

a Who scored the highest?

b Who scored the lowest?

c Who scored six less than Wang Shu?

d How many more did Aimee score than Zlatco?

e Whose scores were the same?

f Who scored four fewer than Wang Shu?

g Who scored four more than Angel?

h What was the total number of times that all of the best spellers achieved

A dot plot is a graphical display that uses dots to record the frequency of events. For example, in this survey six people selected C.

Subtraction strategies

Use the number line to help you answer the questions.

Take away the tens part then the ones part to solve the subtractions.

4 Write as many subtraction number sentences as you can that have an answer of 17. 4

Solve the table facts using the arrays if you need them.

Calculate the cost of each family’s shopping.

4 Write a multiplication problem based on the items above.

8 9

8 Colour each object and matching description the same colour.

a I am a prism that has 6 rectangular faces.

b I am a pyramid that the Ancient Egyptians built. I have a square base.

c I am a pyramid that has 5 triangular faces and a pentagon as a base.

d I am a pyramid that has a six-sided shape as a base.

e I am an object with 2 circles as bases.

f I am a prism that has 2 pentagonal faces and 5 rectangular faces.

9 Model some of these objects from materials like matchsticks, toothpicks, modelling clay and playdough.

10 Describe the difference between a prism and a pyramid.

10

Choosing length units

Length can be measured in metres. There are 100 centimetres in each metre. The way of writing metre is m

Estimate within a range then use a metre ruler, trundle wheel or tape measure to measure each distance to the nearest metre.

a The width of your classroom

b The length of your classroom

c The length of a handball court

d The distance between your classroom and the canteen

e The distance between your classroom and the office door

Choose the correct unit to measure, and write cm or m after each measurement.

a The length of a match

b The length of a glue stick

c The length of a car

d The length of a house

e The length of a paper clip

f The length of this page

g The length of a stapler

h The length of your

Convert each centimetre measurement into metres.

We know that 4 × 3 = 12 so 4 × 3 tens must equal 12 tens. For example: 4 × 30 = 120.

Another strategy used to multiply by a multiple of 10 is to use repeated addition. For example: 4 × 20 = becomes 20 + 20 + 20 + 20 = 80.

3 x 90 = 270 90 + 90 + 90 = 270

3 × 30 is 3 groups of 3 tens, which equals 9 tens. 9 tens is 90.

Rounding off numbers allows us to make quick estimates. It also allows us to check the reasonableness of our answers.

Numbers ending in 1, 2, 3 and 4 are rounded down to the closest ten. 32 becomes 30 Numbers ending in 5, 6, 7, 8 and 9 are rounded up to the closest ten. 48 becomes 50 The number 5

the middle but is always rounded up to the nearest ten. 35 becomes

Estimate the purchases by rounding off to the nearest 10.

a A baseball bat and a soccer ball $

b A book and a toy car $

c Four toy cars $

d A baseball bat, a teddy and a soccer ball $

e Three soccer balls $

Applies an understanding of place value and the role of zero to represent numbers to at least tens of thousands Completes number sentences involving addition and subtraction by finding missing values

Polygons are closed shapes with 3 or more angles and straight sides.

9 Put a cross on the shapes which are not polygons. Match each polygon with its name by colouring them the same colour. Remember, shapes are not always the same. Both of these are pentagons. Name

Record the number of sides and angles of each polygon.

Compares two-dimensional shapes and describes their features

Time units

Complete and learn these facts.

a 60 seconds = f 52 weeks =

b 60 minutes = g 12 months =

c 24 hours = h 365 days =

d 7 days = i 366 days =

e 2 weeks =

Would you use seconds, minutes, hours, days, weeks, months or years to measure these periods of time?

a Lunch time f Cricket match

b Football match g Your favourite song

c TV advertisement h Spring

d Christmas holidays i Recess

e Walk 10 metres j Your life

Use the greater than or less than symbols to make these sentences true.

a 5 days is one week. e 90 seconds is 1 hour.

b 75 minutes is 1 hour. f 13 days is 2 weeks.

c 12 hours is 1 day. g 130 minutes is 2 hours.

d 59 seconds is 1 minute. h 17 days is 2 weeks.

Place these swimming times in order for the judges.

1 min 18 sec 1 min 19 sec 1 min 20 sec 1 min 12 sec 1 min 56 sec fourth

16 Why are there more minutes than hours in a day?

> is the greater than symbol.

< is the less than symbol.

Expanding and ordering numbers

Write the numbers on the place value chart.

c 2307

d 60

e 5207

f 1406

g 6237

Place each set of numbers in descending order.

a 8507, 7503, 5073, 3057

b 2645, 3658, 1999, 2500

c 2907, 8436, 3541, 2657

d 3524, 5234, 2453, 4532

e 837, 238, 1438, 2745

Descending order means from the highest to the lowest.

Write the largest number you can using the digits supplied.

Expand each number. The first one has been done for you.

+

Multiplication facts, times 6

Write a multiplication fact to describe each area model.

Skip count the area model to complete the table of sixes and other related facts.

Revise your multiplication facts by solving the problems.

a Willow saved $4 per week for 6 weeks. How much did she save?

b Arjun trains 5 days a week running 6 km per day. How far does he run each week?

c How much for 9 tickets at $6 each?

d Jack put 7 chocolates in each of 6 bags. How many chocolates did he have?

8 Write the missing numbers to make the number sentences equivalent.

Waru, Jarrah and Alinta paddled across the water in their canoe to a deserted island. Because nobody knew the name of the island, Waru called it Monday Island. Jarrah drew a map of the island. Alinta added a grid to the map to make it easier to identify places on the island.

Use the children’s map above to answer these questions.

a What can be found at B3?

b What can be found at E2?

c What can be found at D4?

d What can be found at G4?

e What can be found at F2?

f What can be found at F5?

The children have not finished the map. Add these items to the map.

a Waru found a broken spear at G3. Draw a spear on the map.

b Alinta saw lots of fish at E2. Draw some fish on the map.

c Jarrah found a very tall tree at C4. Draw a tall tree on the map.

d Waru named the bay at D1 Explorers Bay. Label Explorers Bay on the map.

Make a grid map of your classroom.

How tall are students in Year 4?

On this page you will compare the heights of six students in class 4B at another school with six students in your class.

Use the information in the table to create a column graph showing the heights of the class. Names

Column graphs 6

Class heights of 4B

Centimetres

Measure and graph the height of six people in your class.

The horizontal axis gives the names of the children whilst the vertical axis gives their heights.

Compare the heights of the students in your class with those in 4B and explain what you noticed.

Centimetres

Names

Heights of my class

Names

Ava Eli Sam Kia Priya Ali

Division facts

1 Solve the division facts. The arrays may help you.

2 Sketch an array to solve the division facts. The first one has been started for you.

Solve these division facts using known multiplication facts.

Luisa spent $48 on show bags. How many of each bag could she buy with $48?

How many division number sentences can you write with an answer of 6?

Jump strategies – addition and subtraction

7

6 Use jump strategies to solve the additions and subtractions. The first one is done for you.

7 Use the jump strategy to solve these questions mentally.

A parallelogram is a four-sided shape where each pair of opposite sides is parallel and equal in length.

8 Colour the shapes below that are parallelograms.

9 Draw parallelograms to fit the descriptions.

a I am a parallelogram that has 4 right angles and 4 equal sides.

b I am a parallelogram that has 2 pairs of sides that are equal and 4 right angles.

Is this shape a parallelogram?

10

8 9 10

A trapezium is a four-sided shape with only one set of parallel sides.

Which shapes are trapeziums?

Draw some trapeziums. 11

Capacity can be measured in litres. The symbol for litres is L.

For this page, find a 1 litre container, like one of those shown below.

Estimate first, then tally the number of times the egg cup, glass, cup and mug have to be filled in order to fill your 1 litre container.

Estimate, then measure how many litres of water each container holds (other containers can be substituted).

Discuss with a friend why you think there is a need for a unit smaller than a litre. Listen to their reasons to see if they are the same as yours.

Write a multiplication fact to describe the arrays.

Use the array to answer the 7 times table facts.

When we multiply numbers together we call the result the product.

The product of 9 and 7 is 63.

The first ten multiples of 8 are 8, 16, 24, 32, 40, 48, 56, 64, 72 and 80.

Write two sets of numbers that could be multiplied to give a product of 24. × × 5

Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values

Write a fraction for the shaded part of each shape.

Shade the fractions of each shape.

Use the number lines to help you order the fractions from smallest to largest.

Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)

Drawing three-dimensional objects

Prisms step 1 Prisms step 2 Pyramids step 1 Pyramids step 2

Trace the bases of the objects first before joining their corners.

Draw these objects.

Describe this object.

Makes

and sketches models and nets of three-dimensional objects including prisms and pyramids

Estimate the area of the top surface of these objects. Place each object on top of 1 cm grid paper or use a clear plastic grid overlay to measure the area.

Object Estimate Square centimetres

Li Wei made a shape that covered an area of 16 cm2. He said that all shapes with an area of 16 cm2 also have a perimeter of 16 cm. Piper said that he was wrong. a Draw 2 more shapes with an area of 16 cm2. b Who was correct?

Estimates, measures and compares areas using square centimetres and square metres Compares two-dimensional shapes and describes their features

Li Wei's shape

Split strategy for addition

Add the following numbers mentally by adding the tens part then the ones part. The first one is done for you.

a 35 + 27 becomes 50 + 12 = 62

b 35 + 46 becomes + =

c 37 + 36 becomes + =

d 56 + 27 becomes + =

e 47 + 36 becomes + =

f 86 + 27 becomes + =

g 79 + 35 becomes + =

h 67 + 23 becomes + =

Use the split strategy on these larger numbers.

a 126 + 38 becomes 150 + 14 =

b 147 + 35 becomes + =

c 215 + 69 becomes + =

d 347 + 25 becomes + =

e 267 + 47 becomes + =

f 323 + 57 becomes + =

I can add larger numbers.

Round each addend to the nearest ten to make an estimate for each question before using the split strategy to find the exact answer.

Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values

Known multiplication facts can be used to find unknown facts. For example: 12 × 6? Think! 10 × 6 = 60 + 6 + 6 = 72.

Answer the multiplications.

A strategy to use when multiplying by 4 is to double then double again. For example: 8 × 4? Think! Double 8 = 16 then double 16 = 32.

A strategy for multiplying by 6 is to multiply by 3 then double. A strategy for multiplying by 5 is to multiply by 10 then halve.

Picture graphs

Kim thought that Holdens were the most popular cars. While at the shops she observed the 7 most popular cars and made a picture graph to represent her observations.

Cars in a shopping centre car park

Number of cars

Key = 5 cars

Holden Ford Toyota Mitsubishi BMW Hyundai Nissan

Use the information in the key and the picture graph to answer these questions.

a How many cars were in the car park?

b Which brand was seen the most?

c Which brand was observed the least?

d How many Holdens were in the car park?

Observe the types of vehicles passing your school. Record the number of each in the table below.

Sedans Sports 4WD Utilities Vans Trucks

Construct a picture graph to represent your data. Use a key so that each picture represents more than one vehicle. = vehicles

Vehicles passing the school

Number of vehicles

Sedans Sports 4WD Utilities Vans Trucks

Explain how your data was different from Kim’s data.

am and pm time 9

am is used to describe all time between midnight and noon. pm is used to describe all time between noon and midnight.

Calculate how long it is until the next hour.

a If the time is 3:48 am how many minutes is it to 4 am? minutes

b If the time is 9:35 pm how many minutes is it to 10 pm? minutes

c If the time is 6:25 am how many minutes is it to 7 am? minutes

d If the time is 10:05 am how many minutes is it to 11 am? minutes

e If the time is 4:29 pm how many minutes is it to 5 pm? minutes

Draw the times on the clock faces then write them in the digital form below.

a A quarter past 6 b Half past 3 c A quarter to 5 d A quarter past 9

a Expand the numbers by writing them in the place value grid.

Ones 4326 5279 6380 4206 1702

b Write the number two thousand three hundred and twenty-six.

c Order the numbers from smallest to largest.

1357 7537 3571

Round each number to the nearest 100.

259 =

Solve the additions and subtractions.

a Put a cross on C5.

b Draw a circle on B3.

c Draw a triangle on D2.

d Colour the square formed by these grid references.

A5, B5, A4 and B4

a Who had the most marbles?

b Who had 4 marbles?

c Who had 2 marbles?

d Who had 4 more marbles than Alana?

John Ryan Lucy Alana Sienna

3-digit subtraction with trading

Trading in subtraction

9 ones from 3 ones can’t be done. Trade a 10 from the tens column to make 13 ones. 4 tens becomes 3 tens. 9 ones from 13 ones equals 4 ones.

1 hundred.

1 ten from 3 tens to give 2 tens.

Complete these 3-digit subtractions (trading in the ones).

Supply the missing numerals in these algorithms.

Solve each problem, then carefully check them by doing them again.

a The car park has space for 333 cars. Already 136 spots have been taken. How many spots are still available?

b The cruise ship can take 456 passengers. How many more tickets can be sold if 207 people have booked tickets at this stage?

Equivalent fractions

Equivalent fractions are fractions that have the same value. For example:

Ariana ate 1 2 of a pizza. Ying Yue ate 4 8 of a pizza.

Shade and record an equivalent fraction for the ones given.

They ate the same amount.

Ms Patel’s class cut some strips of coloured paper then folded and labelled them to make fractions.

Study the strips of paper above to find the equivalent fractions.

a How many eighths in one-half?

b How many tenths in one-fifth?

c How many tenths in two-fifths?

d How many eighths in one-quarter?

e How many eighths in three-quarters?

f How many fifths in one whole?

Order the fractions from the smallest to largest.

Tell a friend what you know about these fractions.

An angle is the amount of turn between two arms. The point where the arms meet is called the vertex

Angles are classified according to their amount of opening.

Right angle

Obtuse angle

Larger than a right angle

List examples of each angle found in your school environment. Right angle Larger than a right angle (obtuse angle)

Draw an example of each angle.

a Smaller than a right angle.

b Right angle.

Smaller than a right angle (acute angle)

c Larger than a right angle.

Draw a line to a label to match each angle created by the objects.

Acute angle Smaller than a right angle Acute angle

Length can be measured in millimetres. The symbol for millimetres is mm.

Facts: There are 10 millimetres in 1 centimetre and 1000 millimetres in 1 metre.

What is the length in millimetres for each letter on the ruler.

a mm b mm c mm d mm e mm f mm

Estimate the height of these illustrations in millimetres. Measure the actual height and record your measurements on the grid.

Glue stick Flour Dinosaur Skeleton

Estimate

Actual

Use the 5 mm dot paper to draw the following lines. a 70 mm b 75 mm c 90 mm d 110 mm

Name something that could be measured in millimetres. 14

11 Patterns in tables

Display the answers to each problem in the tables below.

a Kris is paid $5 per hour for his job as a paper boy. How much will he earn in 6 hours?

b The tap leaks at a rate of 3 litres per hour. How much water has been wasted in 6 hours?

c Potatoes are put into 4 kg bags. How many kilograms of potatoes are in 6 bags?

d The triathlete runs 6 km every hour in her training session. How far would she run in 6 hours?

e Tomatoes are delivered in 7 kg boxes. How many kilograms would there be in 6 boxes?

TOMATOES

f Each sports booklet has 8 pages. How many pages would there be in 6 booklets?

g Samantha is paid $10 per hour and Tom is paid $9 per hour. How much will they each earn if they work 6 hours?

pay Sam’s pay

Thirds and sixths

What fraction of each shape is shaded?

Shade the given fraction of each shape.

the given fraction of each group.

Use the greater than > or less than < symbols to compare the fractions.

How many of each fraction are needed to make one whole?

halves

quarters

fifths

tenths

Ten fifths is equal to how many wholes?

Which colour is most likely and least likely to occur?

e Design a spinner f Design a spinner where pink is where green is most likely and the most likely green is least and pink and likely to occur. blue have the same chance of occurring.

A mutually exclusive event is an event where only one of two results can happen.

For example: A coin toss cannot be ‘tails’ if it has landed on ‘heads’.

Tick the mutually exclusive events below. Put a cross for the other events.

a A dice will land on an odd or even number.

b A new kitten will be a male or a female.

c A batter will hit or miss the ball.

d A traffic signal can only show red or green.

e A light switch can only be on or off.

f You can only win or lose a soccer game.

Explain why my score cannot be an odd number if I roll any double number.

11 12 13

The base unit for measuring mass is the kilogram. The symbol for kilogram is kg. Everyday objects such as groceries are measured in kilograms.

Hold a 1 kg mass, then use the hefting technique to identify objects that you estimate are less than, more than and about 1 kilogram.

Less than 1 kg About 1 kg More than 1 kg

Use a pan balance to identify three objects to suit each category. You may like to check the items you hefted in the question above.

Less than 1 kg About 1 kg More than 1 kg

A rockmelon is about 1 kilogram.

2 kg

Use 5 kg kitchen scales to find the mass of the items to the nearest kilogram. Estimate the mass first by hefting.

Item Estimate Mass

a Dictionary

b 2 L of water

c Kettle

d Ream of A4 paper

e Sticky tape

f 1 L of milk

g 10 books

Counting patterns

Continue these counting patterns.

Explain what has happened in these sequences. a 2, 4, 6, 8, 10

b 43, 40, 37, 34

c 13, 17, 21, 25

d 27, 33, 39, 45

e 4, 8, 16, 32

f 80, 40, 20, 10

Follow the rules to complete the number patterns.

30, 39, 48, 57

9.

Multiplication facts, times 8 12

Use the area model to answer the 8 times table questions.

a 1 × 8 = e 5 × 8 = i 9 × 8 =

b 2 × 8 = f 6 × 8 = j 10 × 8 =

c 3 × 8 = g 7 × 8 =

d 4 × 8 = h 8 × 8 =

Use the ‘double, double, then double again’ strategy to solve these 8 times questions.

a 3 × 8 = f 9 × 8 =

b 4 × 8 = g 11 × 8 =

c 7 × 8 = h 15 × 8 =

d 5 × 8 = i 13 × 8 =

e 8 × 8 = j 14 × 8 =

6 x 8 = ?

Think, double 6 = 12, double 12 = 24, double 24 = 48.

Jess is trying to solve 12 × 8 on her calculator but the 8 key is broken. Explain how she could solve this on her calculator by multiplying by 4 or 2 instead of 8.

Crack the secret code by exchanging answers for letters.

Symmetrical patterns 12

Draw the other half of the symmetrical patterns.

a c

b d

8 9

Draw the other half of the symmetrical patterns. a b

Compares two-dimensional shapes and describes their features Performs transformations by combining and splitting two-dimensional shapes

Roll a dice 40 times and tally the number of times each dice face lands, then construct a column graph.

Dice Tally

Which face came up the most?

Which face came up the least?

Do you think this would always happen? Explain why.

Use these words to describe the chance that each event has of happening. likely, unlikely, equally likely, certain, impossible

a A dice is rolled and lands on

b A coin tossed once will land on heads.

c The next traffic light we come to will be red.

d A baby is born a boy.

e A dice is rolled once and lands on an even number.

f A dice is rolled once and lands on a number greater than

g A 7 ball is drawn from a bag with 20 balls numbered 1 to 20 .

Jump and bridging strategies – subtraction 13

Display jump strategies to solve the subtractions on the number line.

Solve these questions using the jump strategy.

Use the bridging to decades method to complete these subtractions. The first one is done for you.

a 92 67 becomes 92

b 52 25 becomes

c 93 28 becomes

d 185 46 becomes

e 292 33 becomes

Solve these problems using jump and bridging strategies.

a Norfolk Theatre has 112 seats. How many seats are empty if 78 tickets were sold?

Think

– 20 = 73 subtract 3 then subtract 6 more.

b How much money does Stella have if she had $124, but spent $49 on a skateboard?

A mixed numeral is a number that consists of a whole number and a fraction.

For example: The model displays 1 whole and 1 half.

Max had a chocolate bar that had 4 pieces of chocolate. Each piece of chocolate represented 1 4 of the chocolate bar. Make Max’s chocolate five quarters large. The dotted lines may help you.

9

Combining and splitting shapes

Draw lines on the shapes below to show how they were constructed using squares, equilateral triangles or both. For example:

Divide the hexagons below into different combinations of shapes.

For example: 2 triangles and a rectangle

Millilitres are used to measure small quantities of liquid. The symbol for millilitres is mL. 1000 millilitres = one litre.

0 Order the following containers from smallest to largest by numbering them from 1 to 7 according to their capacity.

List other items packaged in millilitres.

DRAFT

Use a medicine glass to find the capacity of the containers.

Convert these litre measurements to millilitres.

Counting with fractions 14

Mixed numerals are used when counting beyond 1 on a number line. For example: 0, 1, 1 1 2 , 2, 2 1 2 , 3

Complete the number line to count by halves, quarters and fifths.

0 Draw a line to show where each fraction or mixed numeral belongs on the number line.

Put a cross where the fraction 1 4 belongs on each number line below.

Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)

Use halving skills to divide by 2.

Can you see a way to use halving to divide by 8?

What is it?

Write a division fact from each multiplication fact. The first one has been done for you.

Write a problem to suit the number sentence. 42 ÷ 6 = 7

Reflect, translate or rotate

Reflect, translate or rotate the following shapes. Draw your results. The first one has been done for you. Reflect Translate Rotate ( 1 4 turn clockwise)

DRAFT

Help Wally move the box along the path by continuing his pattern of moves.

Mr Anasta’s class did a survey to find their class’s favourite drinks. Record this data using a tally (||||) on the two-way table.

Juice Milk Soft drink Water

Tom Jason Lisa Kelly Maria

Liam Soula Leonard Ruby Jessica Mary

Jack Emma Finn Con Harry Lauren Kate

Melissa Joshua Hayden Mark Mahomed Margarita

Ms Poulos wanted to know whether there were equal numbers of children in each sporting house in her grade and another grade. She made a table to represent the sports houses and the two classes.

a Were the sports houses all equal?

b Which house had the most?

c Which house had the least?

d How could the sports houses be made equal?

Interpret the putt-putt score card to find out who won (remember, the best player has the lowest score):

a the 2nd hole

b the 4th hole

c the 8th hole

d the 5th hole

Total all scores to find out who:

a was the best player

b was the worst player

c was second

Two-way table

Drinks Boys Girls

Decimal notation uses a decimal point to separate the tenths from the whole numbers. A tenth is one tenth of the whole or 1 10 In its decimal form it is written as 0.1.

0 . 1

Whole numbers Tenths

Write the tenth that is shaded in each diagram as a fraction and as a decimal.

Write these fractions as decimals.

7 10 = .

Write these decimals as fractions.

The ruler has been divided into 10 equal parts. Draw lines to match the labels to the ruler.

Shade the rulers to display the decimals.

Represents and compares decimals up to 2 decimal places using place value Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths)

Tens of thousands 15

Write the numbers on the place value chart. The first one has been done for you.

Arrange the cards to make the largest number, then the smallest number using all the digits.

Order the numbers from the smallest to largest.

Write the number for:

a Twenty-six thousand, two hundred and seventy-one b Fifty-five thousand, one hundred and ninety-six

Grouping two-dimensional shapes

Group the 2D shapes by writing the correct letters after each question.

a Shapes with right angles

b Shapes that do not have right angles

c Shapes that are pentagons

d Shapes with angles smaller than a right angle

e Shapes that are quadrilaterals (4 sides)

f Shapes that are trapeziums (1 set of parallel sides)

g Shapes that are parallelograms (2 sets of parallel sides with opposite sides equal in length)

h Shapes with right angles and parallel sides

Draw examples of each shape. Their bases must not be parallel to the bottom of the page. The first one has been done as an example for you.

am is an abbreviation for ante meridiem, which means ‘before midday’. pm is an abbreviation for post meridiem, which means ‘after midday’.

Order these times from earliest to latest in the day. a

I wake up at 7:00 am.

e 7:51 am 7:52 pm 7:53 am

Solve these problems.

a How many hours from 10:30 am to 4:30 pm?

b How many minutes from 11:30 am to 1:10 pm?

c How many minutes from 9:00 am to 2 pm?

8 ones plus 5 ones equals 13 ones. Trade 10 ones for 1 ten then record the 3 in the ones column.

6 tens plus 7 tens plus 1 ten equals 14 tens. Trade 10 tens for 1 hundred then record the 4 in the tens column.

5 hundreds plus 2 hundreds plus 1 hundred equals 8 hundreds.

Multiplication facts, times 9

Use the area model to answer the 9 times table questions. a

Circle the answers to the 9 times tables on the chart.

Why would it be easy to spot a mistake in the 9 times tables on the chart?

Shade the answers to the 3 times tables on the chart and continue the pattern until it makes 90.

Can you see a relationship between the 3 times and 9 times tables? What is it?

Creating categories

Mr Shearston’s class collected some garbage from around the school. They worked out that the garbage could fit into 5 categories and could be recycled.

12

Sort the garbage into categories by colouring a bin for each piece of garbage. The plastic category has been done for you.

Plastic Cans Paper Glass Food

Group the items into 4 categories. Give each category a name and list all its members in the same box.

red green Maths football pink Jupiter

Earth soccer Saturn yellow Uranus softball

Mars Venus hockey English cricket purple

Name Colours Name

Name Name

Grams

Grams are used to measure objects that are not very heavy. The symbol for grams is g.

1 kilogram = 1000 grams

Collect 3 plastic jars with screw-on lids and make your own standard mass set.

a Jar 1

b Jar 2

c Jar 3

Pour sand into Jar 1 until it balances a 100 g mass.

Pour sand into Jar 2 until it balances a 200 g mass.

Pour sand into Jar 3 until it balances a 500 g mass.

Use your standard mass set to find three objects that closely match each mass. About 100 g About 200 g About 500 g

How many of each fruit or vegetable are needed to balance 1 kg?

Item Mass Number

a Tomato 100 g

b Apple 200 g

c Pineapple 500 g

d Potato 250 g

e Strawberry 50 g

17 Renaming groups to multiply

One strategy used to multiply a 2-digit number by a 1-digit number is to multiply the tens then the ones

150 30

For example: 5 × 36 becomes 5 × 30 plus 5 × 6 = 180

i 58 × 3 becomes =

Estimation is often used to check if the answers to multiplications are reasonable.

Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values

Question: What would happen if 30 was divided by 4?

Answer: There would be 7 groups of 4 and a remainder of 2, because 7 × 4 = 28 and 2 more makes 30.

b 44 marbles were shared among 6 children. How many did each child receive? remainder

c 48 dice were shared among 5 groups in the classroom. How many did each group receive? remainder

d 23 football cards were shared among 4 girls. How many did each girl receive? remainder

e 45 cows were grouped in 8s for sale. How many groups were there? remainder

f 50 flowers were planted in 6 gardens. How many flowers in each garden? remainder

Until now we have used the 4 major compass points of north, south, east and west. The extra compass points of north-east (NE), north-west (NW), south-east (SE) and south-west (SW) help us to more accurately plot positions on a map.

9 Study the map before answering the questions below.

a Which state is east of the Northern Territory?

b Which state is west of the Northern Territory?

c Which capital city (in Qld) is north-east of Adelaide?

d Which capital city is north of Uluru?

e Which city is north-east of Wyndham?

f Which city can you find west of Eyre?

g What landmark is south of Darwin?

h Name a city south-west of Sydney.

i Which city is south-east of Whyalla?

j What direction is Adelaide from Hobart?

Draw a cross for an imaginary city that is SW of Uluru and found in South Australia.

11 Draw a circle for an imaginary city in Western Australia that is NE of Perth.

Busselton Eyre

Whyalla

Wyndham

Port Macquarie Cowra

Shepparton

Alice Springs Uluru

DARWIN PERTH ADELAIDE HOBART

MELBOURNE

CANBERRA SYDNEY

BRISBANE

Cairns PACIFIC OCEAN

CORAL SEA

INDIAN OCEAN

Perimeter is the distance around the outside of a shape. (The length of its boundary.)

Measure the perimeter of these shapes.

Use the 1 cm grid paper to construct squares of the given perimeters. Starting points have been given.

Use the 1 cm dot paper to calculate the perimeter of each shape.

3-digit addition

1 hundred plus 3 hundreds plus 5 hundreds equals 9 hundreds Record the 9 in the hundreds column.

5 ones plus 8 ones equals 13 ones Trade 10 ones for 1 ten then record the 3 in the ones column.

1 ten plus 5 tens plus 6 tens equals 12 tens Trade 10 tens for 1 hundred Record the 2 in the tens column.

1 Add the 3-digit numbers without trading.

Add the 3-digit numbers with trading in the ones, tens and hundreds.

Round to the nearest 100 to make an estimate of each problem before solving it. Estimate Answer

a 364 people attended the fete on Saturday and 439 on Sunday. What was the total attendance?

b During the survey 482 drinks were sold in the first week and 425 in the second week. How many drinks were sold during the survey?

Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers

Patterns on a hundreds chart 18

Complete the grid below and then shade this pattern on the hundreds chart. Start at 2 then continue to add 11.

2 13 24

Complete the table to record the 11 times tables. Circle the answers on the same chart.

1 2 3 4 5 6 7 8 9 11 22

Why would it be easy to spot a mistake in the 11 times tables on the chart?

7 Explain the shaded pattern on the chart opposite.

Circle this pattern on the same chart. Start at 5, then add 3, then continue to add 5 and then 3 until you run out of space.

Draw a line to match the rule to the pattern.

Top, front and side views 18

Three-dimensional objects can be viewed from the top, front and side.

The top view only shows what can be seen directly from the top.

The front view only shows what can be seen directly from the front.

front view

The side view only shows what can be seen directly from the side.

Label the views of the object as top, front or side views.

view view view

Construct the prisms made from blocks then draw the front, side and top views of each one.

Front view Side view Top view

Makes and sketches models and nets of three-dimensional objects including prisms and pyramids

Measure the area of each rectangle below in square centimetres.

Can rectangles with different side lengths have the same area?

Sketch shapes that have an area of 7 square centimetres. Two examples have been given below.

Find the area of each right-angled triangle.

PART

a Count forward by 10s. 526

b Count forward by 100s.

378

c Count backwards by 100s. 9025

d Count backwards by 10s. 5428 PART

Use a strategy you have learned to solve the additions and subtractions.

a 86 25 = b 38 + 74 =

c 90 35 = d 83 + 27 =

e 82 24 = f 42 + 48 = PART

Use strategies such as halving to solve the following.

a 12 ÷ 2 = b 26 ÷ 2 =

c 36 ÷ 2 = d 50 ÷ 2 =

e 84 ÷ 2 = f 76 ÷ 2 =

Use strategies such as halving and halving again to solve these.

g 12 ÷ 4 = h 32 ÷ 4 =

i 20 ÷ 4 = j 48 ÷ 4 =

k 56 ÷ 4 = l 64 ÷ 4 =

Shade the shapes to display the mixed numeral.

Use rounding to find the approximate answer.

a 19 × 8

c 41 × 3 ≈ d 32 × 5

Shade the right angle, tick the acute angle and put a cross on the obtuse angle.

Show the times on the digital clocks. Use ‘am’ and ‘pm’.

evening

Place the compass points on the compass. S E W NE SE SW NW

Draw the other half of the symmetrical pattern.

Measure the length of the line in millimetres. mm

a How many grams in a kilogram?

b How many millilitres in a litre?

c How many times would each container need to be filled to fill a 1 litre jug?

a Rotate this shape.

Draw a rectangle with a perimeter of 10 cm and an area of 6 square centimetres.

b Reflect this shape.

Rounding off numbers allows us to make quick estimates. It also allows us to check the reasonableness of our answers.

down to the closest hundred.

You have previously been taught how to round numbers to 10 and 100. Use this knowledge to help you round numbers to 1000.

Round each number to the nearest 10, the nearest 100 and the nearest 1000. The first one has been done for you.

Regrouping factors

Factors are whole numbers that can be multiplied with another number to make a new number. For example: the factors of 16 are 1, 2, 4, 8 and 16.

(2 × 8 = 16 4 × 4 = 16 16 × 1 = 16)

g 35 × 4 becomes × 4 = 7 ×

Multiplication can be made easier by factorising. To factorise a number you simply write it as a product of its factors, e.g.16 can be written as 4 × 4, 2 × 8 or 16 × 1. Represents

19 Turns

Watch the arrow go through its turns.

Start 1 4 turn 1 2 turn 3 4 turn full turn

DRAFT

9 10

Follow the instructions to make a pattern.

Rotate 1 4 turn clockwise in each square.

Rotate 1 2 turn clockwise in each square.

Performs transformations by combining and splitting two-dimensional shapes

Colour the 15 lollies in this bag to match the tags at the side of the page.

a Red has only one chance of being pulled out first.

b Blue has twice as many chances of being pulled out first compared to red.

c Green has four times more chances of being pulled out first compared to blue.

d Yellow has only half as many chances as green of being pulled out first.

10 coloured marbles were placed in a bag. Each time a marble was taken from the bag it was not replaced.

Answer the questions with true or false.

a 1st draw

Jack drew a red marble from the bag. This means that red would now have the same chance of being drawn as green.

b 2nd draw

Sarah drew a green marble. This means that green has the same chance of being drawn as yellow.

c 3rd draw

Max drew a yellow marble. This means that it is now impossible to draw another yellow marble.

Ben had a go at the spinner, and the pointer stopped on red. He told his friend Than that if he spun it again, red would have the same chance of occurring as any other colour. Explain why you agree or disagree with Ben.

• 5 ones take away 3 ones is 2 ones.

• I can’t take 6 tens from 2 tens. Trade 1 hundred for 10 tens.

• 8 hundreds become 7 hundreds.

• 12 tens take away 6 tens is 6 tens.

• 7 hundreds take away 5 hundreds is 2 hundreds.

Complete the number cross.

Another way to express division is the division symbol ) . 4

3 ) 12 means 12 ÷ 3 = 4

5 children. How many did each child receive?

Use the division symbol to show how many ways you can share 40 without any remainders.

Hunter wants to share 22 marbles into 5 bags. He knows that it will not work out equally and that there will be a remainder.

How many birds will there be in each cage?

22 shared among 5 bags means 4 in each bag and 2 left over.

An angle is the amount of turn between two arms.

A good example of angles is the amount of turn between the two arms on a clock face.

Draw the turns on the clock faces from the given starting points.

Look at the clock faces and answer the questions.

a Which angle above is a 1 4 turn?

b Which angles above are less than the

turn?

c Which angles above are greater than the

Tick the number of quarter turns it takes to make one complete revolution of the clock face.

turn?

The square corner on a piece of paper is equal to a 1 4 turn. This common angle is called a right angle

Find three examples of right angles in and around your school. 11

School rulers often show centimetres along one edge and millimetres along the other.

14

3 Convert these centimetre measurements into millimetres.

a 2 cm = mm e 9 cm = mm

b 3 cm = mm f 10 cm = mm

c 5 cm = mm g 1 2 cm = mm

d 7 cm = mm h 1 1 2 cm = mm

Decimal notation

Larger distances can be measured with a trundle wheel. 15

Use a metre rule or a tape measure to record the lengths or widths of the items in metres and centimetres as well as in decimal notation, e.g. 1 m 63 cm = 1.63 m.

Distance m and cm Decimal

a The length of a cupboard m cm m

b The length of a noticeboard m cm m

c The width of your classroom m cm m

d The length of your classroom m cm m

e The length of a handball court m cm m

f The length of a car m cm m

21 Compensation strategy

The compensation strategy can be done by rounding up one of the numbers that you are adding, e.g. 54 + 38 = ? Think 54 + 40 = 94, then take off the 2 that was rounded up. The answer is 92.

The compensation strategy can also be done by rounding down one of the numbers that you are adding, e.g. 58 + 33 = ? Think 58 + 30 = 88, then add the 3 that was rounded down. 88 + 3 = 91

Explain the strategy you would use to solve: 148 + 36 = . 3

The term hundredths can be used to name fractions where the denominator is 100 (100 equal parts).

Write the number of equal parts out of 100 for each part of the metre ruler, then record it as a fraction. The first one is done for you.

5 Lollies

On the shelf in the lolly shop was a large jar of 100 lollies. What fraction of the lollies were:

a Blue? 100

b Black? 100

c Grey? 100

d White? 100

Write the score each person received out of 100 marks for their piano exam. Write each score as a fraction.

a Mia received a total

d Aliya received 12 less of 60 marks. 100 marks than Alex.

b Tom received 15 marks

e Fred received 13 more more than Mia. marks than Aliya.

c Alex received 10 marks

f Jessica only received less than Tom. half of Fred’s mark.

Order the marks from smallest to largest.

Explain why 1 2 is smaller than 53 100.

I don’t like practising!

Angles are classified according to the amount of turn between two arms.

Square corner Larger than a right angle

Smaller than a right angle

Can be made from two right angles

Larger than a straight angle

Sometimes an angle is formed when only one arm of the angle is visible, such as a door opening or a car driving up a hill. In these cases you have to visualise where the other arm would be.

Which of the doors above is closest to a right angle?

Is door B displaying an acute angle?

1 Colour each beaker so that they show:

2 Gather these containers, fill them with water, pour the water into measuring beakers and then colour each beaker below to match the quantity of water in each container.

3 Solve these problems.

Question

Answer

a What is the difference in capacity between the chocolate milk carton and the shampoo bottle? mL

b How many millilitres would two shampoo bottles hold? mL

c What is the difference in capacity between the chocolate milk carton and the soft drink can? mL

d How many millilitres would two chocolate milk cartons hold? mL

22 3-digit subtraction with trading

6 hundreds take away 1 hundred leaves 5 hundreds.

4 ones take away 6 ones: trade 1 ten from the tens column to make 14 ones.

2 tens take away 8 tens. Trade 10 tens from the hundreds column to give 12 tens. 12 tens – 8 tens = 4 tens.

Complete these subtractions. They do not need trading.

The theatre holds 734 seats. How many seats are left if 186 tickets have been sold?

Complete the subtractions with trading in the ones.

Use trading to complete these subtractions.

I can check my subtractions by backtracking.

Fractions of a collection

Fractions can show part of a group.

1 2 of 6 girls = 3 girls

Find the fraction of each group.

Kelly has a collection of 24 cows. Find these fractions of her collection.

a 1 2 of 24 cows =

Solve the problems.

a Jim had $20 and spent 1 2 of it, Tatijana had $24 and spent 1 4 of it. Who spent the most?

b Prani had 12 cakes and Karim had 16 cakes. Prani ate 1 4 of hers and Karim ate 1 8 of his. Who ate the most?

3 Use counters to find fractional amounts of 20, e.g. 1 2 of 20 = 10.

Classify the sets of shapes into tessellating and non-tessellating. Write your answers in the grid. (Tessellate means shapes that fit together without gaps.)

Tessellating Non-tessellating

DRAFT

Make a tessellation of your own using Pattern Blocks and sketch it in the space provided.

Compares two-dimensional shapes and describes their features

REDFERN TO BONDI JUNCTION

3 Interpret the timetable.

a What time does the 12:20 pm train from Redfern arrive at Bondi Junction?

b What time does the 12:50 pm train from Redfern arrive at Edgecliff?

c What time does the 1:20 pm train from Redfern arrive at Martin Place?

d What time does the 12:50 pm train from Redfern arrive at Town Hall?

4 How long does it take the trains to travel from:

a Redfern to Central

b Redfern to Town Hall

c Central to Martin Place

d Edgecliff to Bondi Junction

15 If you caught the 12:50 pm from Redfern, where would you be at these times?

6 How long is it between trains from Redfern?

7 Record the time when you do these things. Use digital time.

Solve the problems on note paper then record your answers below.

a How much would it cost for

c How much would it cost for 3 books? $ 5 games? $

b How much would it cost for

d What costs more: 2 games 3 bracelets? $ or 3 cakes? $

A hundredth is a fraction where the denominator equals 100 (100 parts to the whole).

Shade the grids to display the hundredths. The first one has been done.

Fractions can be written as decimals, e.g. 75 100 can be written as a decimal fraction 0.75

(The decimal point separates the fraction from the whole number.)

Write the equivalent hundredth and decimal to describe the grids.

Place the appropriate letters in the boxes to describe the shortest routes. Which roads would you travel to get from:

a Soula’s house to John’s house? G F D A

b Con’s house to school?

c Anita’s house to the pool?

d John’s house to the shops?

e Con’s house to the shops?

f Pablo’s house to the shops?

Follow the directions from X to find the secret letter.

a Go north 4 spaces.

b Go east 4 spaces.

c Go south 3 spaces.

d Go east 3 spaces.

e Go north 4 spaces.

f Go west 2 spaces.

g Go south 3 spaces.

h What was the letter?

i Without drawing any sloping lines, draw the shortest path between V and Q.

j Draw a much longer path between V and Q.

James found a box of counters and laid them out in their colours.

black red pink yellow green orange

Write true or false for the statements based on the knowledge that James put all the counters back in the box and then selected one counter to show his mum.

True or False

a James is more likely to pick out a black counter than a red one.

b James is less likely to pick out a red one than an orange one.

c James is more likely to pick out a red one than a yellow one.

d James is more likely to pick out a pink one than a yellow one.

e James is less likely to pick out an orange one than a black one.

f James is more likely to pick out a yellow one than an orange one.

3 Order the colours from the one that is least likely to be taken out of the box to the one that is most likely.

Place the marbles in the bag by writing a letter on each marble for each colour.

a There are 4 red marbles.

b There are 3 more green marbles than red ones.

c There are half as many blue marbles as red marbles.

d There are 2 more yellow marbles than green ones.

e The rest of the marbles are pink.

Answer the questions.

a Which is the most likely marble to be drawn out of the bag?

b Which is the least likely marble to be drawn out of the bag?

c Which is more likely to be drawn from the bag: a green or a red marble?

Sometimes it is easier to do a mental strategy than an addition algorithm. Especially if there is no trading.

Add the 3-digit numbers with trading in the ones and tens.

Use any strategy you wish to solve these problems.

a When the train left the city there were 165 passengers on board. At the first station 86 people boarded the train, and another 73 boarded at the next station. How many people altogether were on the train?

b A driver had 3 parcels to deliver. Their masses were 326 kg, 438 kg and 160 kg. What is the total mass of the parcels?

c A hired car was driven 422 km from Sydney to its first destination and then another 428 km to its final destination. What is the total distance travelled?

d On Saturday 378 people attended the fete and on Sunday 486 people attended. How many people attended the fete during the weekend?

Extending division facts

We know that 8 ÷ 2 = 4 so 80 ÷ 2 must equal 40.

Trial and error

Use trial and error to find the answers to the divisions with remainders, e.g. 26 ÷ 4. Try 5 × 4 = 20, 6 × 4 = 24, 7 × 4 = 28. The answer is 6 remainder 2.

b Mr Smith put 150 students on 3 buses. If they all had the same number of students on them, how many were on each bus?

d Jack had 48 marbles that he shared among himself and 5 other children. How many marbles did each child receive?

Which fruit is the most popular in your class? Predict answers to the following questions about the most popular fruits in your class then write the result.

Prediction Survey result

The most popular fruit will be

The least liked fruit will be

Conduct a survey to find out which are the 5 most popular fruits, then tally and graph your results in the space below.

apples

Apples

Most popular fruits, Class Number of children

In groups, repeat your survey using a different class or group from within your school.

Using all the data you have collected, suggest the types of fruit that Australian canteens should sell.

24 Grams

Estimate and then measure the mass of the items in grams using a pan balance.

What would be the mass of your pencil case with these things in it? You may need a calculator to work it out. Pencil case

Convert the kilogram measurements to grams. The first one is done for you.

Mixed addition

Complete the mixed additions to solve the secret code below.

Addition of money. (Keep the decimal point in a straight line.)

Complete this problem.

a Theo bought 3 cans of paint that cost $27 each. He paid with two $50 notes. How much change did he receive? $

b Find a person in the class who solved it in a different way to you and describe their method.

Equivalent tenths and hundredths

Study the grids then complete the table. The first one is done for you.

Use the greater than >, less than <, or equals sign = to make these number sentences true.

Colour the beads to show how Kara coloured them.

a She coloured 1 tenth of them red.

b She coloured 3 tenths of them yellow.

c She coloured 20 hundredths green.

d She coloured 0.30 of them blue.

e She coloured 7 hundredths orange.

f She left 3 hundredths uncoloured.

25

Pentagons and octagons

Write a description for each of the shapes below so that a person who has never seen the shape would be able to draw it from your description.

Make some different pentagons and octagons on paper or geoboards then record them on the dot paper below.

DRAFT

Describe the differences between the 3D object and the 2D shape below.

The square metre

A square centimetre is too small to measure large areas.

Larger areas need to be measured in square metres

The symbol for square metres is m2.

Make two areas of 1 square metre out of newspaper.

Using newspaper, stick pieces together to make a square that is 1 metre wide and 1 metre long.

1 m 1 m 1 square metre

2 metres long, 1 2 metre wide

Explain why both of these shapes have an area of 1 square metre.

DRAFT

Use your newspaper square metres to identify areas in the playground that are less than 1 m2, about 1 m2 and larger than 1 m2.

Less than 1 m2 About 1 m2

Larger than 1 m2

Estimate and then measure these areas using the square metres you and your classmates have made.

floor

Fractions can be written as decimals.

E.g. 75 out of 100 can be written in the decimal form as 0.75.

75 100

The decimal point separates the fractional part from the whole number.

A metre ruler is 100 centimetres long. Each centimetre is equal to one hundredth of a metre.

Write each measurement on the ruler as a decimal. The first one is done for you.

Draw a line to match each decimal to its place on the metre ruler.

Draw a line to match each fraction or decimal to a place on the ruler.

What could the number sentence have been? Write some of your solutions.

Supply a number sentence to balance those already on the balances. Your sentence must use the operation sign provided. The first one has been done for you.

26 Combining and splitting shapes

List the shapes that have been used to make each of these patterns.

a b c d

Demonstrate how each of the shape combinations fit together without gaps by continuing the pattern.

a Octagons and squares

b Hexagons and squares

DRAFT

Continue the patterns. a b

Combine some shapes of your own and sketch the pattern they make on a separate piece of paper.

Compares two-dimensional shapes and describes their features

A standard unit for measuring volume is the cubic centimetre. The abbreviation for cubic centimetre is cm3. A cubic centimetre is a cube that has 1 cm sides.

Objects have different dimensions such as length, width and height.

DRAFT

Hund Tens Ones

9 × 3 = 27. Write the 7 in the ones column and trade the 2 to the tens column.

9 × 5 tens = 45 tens plus the 2 tens traded = 47 tens. Write a 7 in the tens column and a 4 in the hundreds column.

7

× 9 4

Calculate how much each worker would save.

a How much would Pedro save in 5 weeks if he saved $27 each week?

DRAFT

Hund Tens Ones × $

b How much would Grace save in 6 weeks if she saved $35 each week?

Hund Tens Ones × $

c Billy saved $45 each week for 5 weeks.

What are his total savings?

Hund Tens Ones × $

d Chloe saved $36 each week for 7 weeks. What are her total savings?

Hund Tens Ones × $

e If Ji Wu saved $34 each week for 7 weeks, would he have enough to buy a gaming console for $250?

Yes No

f If Oscar saved $48 each week for 5 weeks, would he have saved more than Grace?

Yes No

Hund Tens Ones × $

Hund Tens Ones × $

Janan had 65 stamps to share among his 5 children. This is what he did.

65 shared among 5

5) 65

Share out the tens with each person getting 1.

1

5) 615

Trade the 1 ten left for 10 ones. Now share the 15 ones among 5.

1 3

5) 615

Sometimes divisions don’t work out equally and have remainders. Let’s see how Ms Flockhart shared 73 cakes among 3 classes.

73 shared among 3

3) 73

Share out the tens with each class getting 2. 2

3) 713

Trade the 1 ten left for 10 ones. Now share the 13 ones among 3.

2 4 r 1

3) 713

Answer: 24 remainder 1

Reading a map/grid references

Study the map of the small Australian city to answer the questions. What

Describe one way of getting from the Railway Station to Barney’s Retreat.

Where am I?

• I left the railway station and turned left into Edward St.

• I drove along Edward St and turned right into Docker St.

• I drove along Docker St until I got to Kincaid St, then I turned left.

• I parked my car in Kincaid St.

Chance experiments

Wang Wei has three marbles in a bag that are coloured red, yellow and blue. There are six different ways that the marbles can be drawn out of the bag. Colour the marbles to show the possible combinations that he could draw out.

Hint: R Y B is different from B Y R

Chantel’s class put three different coloured counters in a bag. They drew a counter from the bag, recorded the colour, then replaced it in the bag. They did this ten times.

Red Yellow Blue

Were the results as you would expect?

Do the same experiment with a bag with marbles and draw the marbles out 40 times. Record your data using tally marks in the table below.

Red Yellow Blue

If you had a single draw of a coloured marble from the bag, is any one colour more likely to be drawn out than another?

The nets of four cubes have been made for a colour game. Colour the red faces on the nets to match the chance of that cube showing red.

c d

Round each number to the nearest 10, 100 and 1000.

Nearest 10 Nearest 100 Nearest 1000

Complete the division facts.

a 24 ÷ 3 =

b 32 ÷ 4 =

a 4358

b 7499

c 6502

d 9001

Round each number to the nearest 100 to estimate each answer.

c 35 ÷ 5 =

Solve these divisions that have remainders.

d 26 ÷ 5 = remainder

e 32 ÷ 6 = remainder

f 41 ÷ 5 = remainder

Write a fraction and a decimal to describe the shaded part of each grid.

a b . .

Write a decimal to describe the grids.

c . What is the place value of the bold digit below?

d 37.13

Find the fractions of each collection.

e 1 2 of 10 =

f 1 4 of 8 =

g 1 10 of 10 = h 1 5 of 10 =

a Which colour is most likely to be spun?

b Which colour is least likely to be spun?

c Does yellow have a greater chance of being spun than orange?

Name these shapes.

How long are these trips?

a From Beach St to Lake St

b From Bay Rd to the school

c From Beach St to the school

d From Lake St to the school

a Draw a right angle.

b Draw an acute angle.

Calculate the volume of this object built from centicubes (cm3).

a Colour the 1 litre container to the correct level if all the containers below were poured into it.

the tessellating pattern blue.

How many millilitres in:

b 1 litre and 500 mL mL

c 2 litres and 375 mL mL

Draw the arrows in their new positions.

28 4-digit addition

5 ones plus 8 ones equals 13 ones. Trade 10 ones for 1 ten then record the 3 in the ones column.

1 thousand plus 3 thousands plus 4 thousands equals 8 thousands. Record the 8 in the thousands column.

1 hundred plus 8 hundreds plus 5 hundreds equals 14 hundreds. Trade 10 hundreds for 1 thousand. Record the 4 in the hundreds column.

1 ten plus 5 tens plus 6 tens equals 12 tens. Trade 10 tens for 1 hundred then record the 2 in the tens column.

2 Round each number to the nearest 1000 to estimate the total before solving the problem.

What was the total attendance at the football games if 9784 people attended the game in Sydney and 4218 attended the game in Melbourne?

Estimate Answer

3 Manuel completed 4 addition sums. His teacher asked him to check them by doing a subtraction. He has done the first one, can you do the rest?

a 14 + 6 = 6 + 14 =

b 17 + 5 = 5 + 17 =

c 13 + 8 = 8 + 13 =

d 120 + 25 = 25 + 120 =

e 118 + 17 = 17 + 118 =

f 215 + 16 = 16 + 215 =

28 Commutative property

4 George said that it did not matter in which order numbers are added. Check to see if George is correct by adding the following pairs of number sentences.

a 6 × 3 = 3 × 6 =

b 5 × 4 =

c 6 × 5 =

d

e 8 × 6 =

f 6 × 7 = 7 × 6 =

g 8 × 9 = 9 × 8 =

h 7 × 9 = 9 × 7 =

6 Write 2 number sentences for each problem before solving them. Problem

a Will had 93 marbles and Eli had 32. How many marbles did they have altogether? + = + =

7 + 9 = 16

Cool!

5 Bella said that you can also multiply numbers in any order. Check to see if Bella is correct by multiplying the following pairs of numbers.

DRAFT

So is 5 x 7 equal to 7 x 5?

× = × = unit Oxford University Press

6 REASONING, COMMUNICATING, PROBLEM SOLVING L N CCT Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving addition and subtraction by finding missing values Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems

MP_NSW_SB4_38336_TXT_3PP.indb 117 28-Jul-23 19:36:16

The local council conducted a survey to find the size of a typical family.

Sindhu Burns Jones North Chang Otford Quinn

5 2 6 3 4 6 4

Ryan Salem Stuart Rashed Vidler Walsh Talko

5 3 4 4 4 6 3

Make a table of the number of families with 2, 3, 4, 5 or 6 family members.

Family size 2 3 4 5 6

Number of families 1

Use the data you have gathered in your table to create a bar graph that shows the number of families of 2, 3, 4, 5 or 6.

Conduct a survey of your own about the size of families in your school. Use the space below to record your data in a table.

Make statements about any patterns or trends you noticed in your data.

Who might make use of this kind of information? Why?

How could the data be useful for your school principal?

Kilograms and grams

13 Read the scales to record the masses in kilograms.

The masses of smaller objects are measured in kilograms and grams

Kitchen scales are often used for these smaller masses.

The large numbers on the scales are the whole kilograms. The smaller markings are steps of 100 grams.

16 Find three items and measure their masses using kitchen scales.

29 Square numbers

Square numbers are numbers that can be arranged in the shape of a square array. They are equal to a number multiplied by itself.

DRAFT

Con the fruit and vegetable shop owner wants to know how many bags of different quantities he could make up from the box of 48 tomatoes he bought at the market.

a How many bags of 3 could he make?

b How many bags of 4 could he make?

c How many bags of 5 could he make?

d How many bags of 6 could he make?

e How many bags of 8 could he make?

f How many bags of 9 could he make?

Knowing your tables makes division easy. Show

Solve the quick division problems.

a 32 stickers shared among 4 children

b 37 chips shared among 5 children

c 43 chocolates shared among 8 boys

d 65 bananas shared among 4 girls

Rounding to the nearest 5 cents

In Australia we don’t have 1 cent and 2 cent coins. This means that prices are rounded to the nearest 5 cents.

Prices ending in 3 cents, 4 cents, 8 cents and 9 cents are rounded up to the nearest multiple of 5 cents.

Prices ending in 1 cent, 2 cents, 6 cents and 7 cents are rounded down to the nearest multiple of 5 cents.

DRAFT

If a customer buys several items at a shop it is only the total that is rounded.

Examine some shopping dockets at home to see whether rounding occurs when electronic cards are used. What did you notice?

A primary school class did fund raising for 5 months for charity. They recorded the amounts they raised on a pie graph, a column graph and a pictograph.

Which one of the graphs is the easiest to read the exact amounts raised?

Which of the graphs above would be the next best to read amounts raised?

One of the graphs shows the whole amount broken into slices. It is not easy to read the exact amounts from this graph. Which graph is this?

Use the graphs above to find how much money was raised in:

May

Does the pictograph clearly show that more money was raised in August than July?

In October, an amount of $50 was raised by the class. Extend the pictograph to show October’s fund raising.

30 4-digit addition

1 Add the 4-digit numbers with trading in the ones.

2 Add the 4-digit numbers with trading in the ones, tens or hundreds.

4 How much did each person spend? (You may need a calculator.)

a Mr Li had an entrée of prawns, a main meal of fish and an apple pie for dessert. $

b Molly had oysters, steak Diane and pavlova. $

Make up a meal consisting of an entrée, main and dessert that costs between $23 and $26.

Decimal place value

Decimal notation uses a point to separate the whole numbers from the tenths and hundredths. Zero can be used as a place holder for hundredths that are less than 10, e.g. 7 hundredths = 0.07.

A number of children measured their heights using metre rulers. Given their heights in centimetres, complete the table stating how many metres and centimetres tall they are, then record it using decimal notation. The first one has been started for you.

$

Use any strategy you wish to solve these problems.

a How much would it cost Ben to buy a TV and a couch? =

b How much would it cost Erica to buy a refrigerator and a dishwasher? =

e If Fiona was given $199 cash back for her old TV, how much did she pay for a new one?

c How much more does Hannah need to save if she wants the TV but only has $1878? =

d How much did Ryan pay for the refrigerator if he was given a discount of $299? =

f How much would it cost Tom to buy the dishwasher and the washing machine?

g What is the difference in price between the washing machine and the dishwasher? =

h How much would it cost to buy the couch and the refrigerator? =

How much money did Dapto Cricket Club spend on these items?

1 Complete the algorithms using the contracted form to discover the winning bingo card. Circle the winning card.

2 Solve the problems.

a Harry had four times more marbles than Joel. If Joel had 45 marbles, how many marbles did Harry have?

b Lena saved $37 per week for 6 weeks. How much did she save altogether?

3 Calculate the answers to these multiplications mentally. The first one is done for you.

Question

Draw a line to match the decimals to a place on the number line.

Is 0.9 greater than 1?

Draw a line to match the decimals to a place on the number line.

Draw a line to match the decimals to a place on the number line.

9

Nets of 3D objects and dimensions 31

Colour each object and its net the same colour. Make sure you use a different colour for each pair.

Objects Objects

Objects have different dimensions such as length, width and height.

Each object below has been built from centicubes. Measure its three dimensions in centimetres.

Each of these measurements has a decimal point. The decimal point separates the fractional part of a number from the whole.

Measure the heights of 9 volunteers in your class, carefully completing the table as you go. An example has been given to get you started.

Six children from Ms Gem’s class entered the high jump and recorded their scores.

Bianca 1.12 m Moana 0.69 m Solomon 0.93 m

Zeedan 0.72 m Nathan 1.04 m James 0.89 m

Order the jumps from smallest to largest.

1.23 m

32 Number patterns

Use the rule and a calculator to extend these patterns.

Complete the pattern up to 7 terms then find the required terms. a

What would be the eighth term? What would be the tenth term? b

What would be the ninth term? What would be the tenth term?

c 24 28 32 36 f

What would be the ninth term? What would be the twelfth term? Follow the 2-step rules to complete the number patterns.

then add

Completes number sentences involving addition and subtraction by finding missing values Represents and uses the structure of multiplicative relations to 10 × 10 to solve problems Completes number sentences involving multiplication and division by finding missing values

then add

then

Fraction and decimal patterns

Use the number lines to help you complete the fraction patterns.

Use the constant addition function on your calculator to make the following patterns. The first once has been started for you.

Complete the decimal counting patterns.

Represents and compares halves, quarters, thirds and fifths as lengths on a number line and their related fractions formed by halving (eighths, sixths and tenths) Represents and compares decimals up to 2 decimal places using place value

Data investigation

Connor said that most children are born in the month of September.

a Make a prediction about the month in which most students in your class were born.

b Make a prediction about the month in which the least students were born.

c Conduct a survey of the students in your class to find out if Connor was correct. Record your information using tally marks on the grid.

d Construct a column graph to display the data.

Months in which students in our class were born

The horizontal axis states the months. The vertical axis gives the number of students.

Was Connor correct about September being the month in which most children are born?

Were the survey results as you expected them to be?

Write a comment about your survey results.

Billy and Zena drew this large picture with chalk on the playground. They wanted to know how big it was, so they drew square metres in chalk over the drawing.

a How many square metres did the drawing cover?

b Can you see a quick way to count the square metres?

c Explain how you counted the squares.

Construct a handball court on the school playground. What you will need

Dimensions of court Metre ruler Chalk

A large 90° angle tester

A small group of children to help you

A day when it isn’t raining

With a partner, work out a way to calculate these areas of your handball court.

a The area of the whole court

b The area of half the court

c The area of a quarter of the court

Check the accuracy of your court by measuring its diagonals. They should be the same.

33 10 times, 100 times, 1000 times larger

Multiply each number by ten.

15

25

36

50

Multiply each number by 100.

15

28

36

40

Multiply each number by 1, 10, 100, 1000 and 10 000. Record your answers in the place value chart.

What happened when you multiplied each number by ten?

A zero was placed at the beginning of the number.

A zero was placed to the right of the number.

What happened when you multiplied each number by one hundred?

A zero was placed to the right of the number.

Two zeroes were placed to the right of the number.

Did you notice a pattern?

6, 60, 600, 6000, 60 000.

Complete the number expander for the number 4327.

How many tens are in these numbers?

a 37

b 435

c 2362

d 3574

How many hundreds are in these numbers?

a 374

b 567

c 2345

d 25 674

How many thousands are in these numbers?

a 3562

b 27 435

When numbers are added it doesn’t matter which order they are added in. For example: 3 + 4 + 6 = 13 and 6 + 4 + 3 = 13.

Complete the sets of number sentences to see if this is true.

When numbers are multiplied it doesn’t matter which order they are multiplied in. For example: 3 × 4 × 5 = 60 and 5 × 4 × 3 = 60.

Complete the sets of number sentences to see if this is true.

Rewrite each number sentence to make it easier to solve.

a 7 + 9 + 3 becomes + = +

b 28 + 17 + 2 becomes +

+

c 18 + 15 + 5 becomes + = +

d 34 + 17 + 16 becomes +

+

e 7 × 2 × 5 becomes × = ×

f 7 × 4 × 5 becomes ×

×

Selects and uses mental and written strategies for addition and subtraction involving 2- and 3-digit numbers Completes number sentences involving multiplication and division by finding missing values

Ms Evans did a survey in her class of the countries that her students’ parents came from. She asked her class to present the country of origin data in 3 different ways. Make a table from the tallied data Ms Evans supplied. Greece Italy Vietnam Australia Lebanon

Make a bar graph from the table you have made above. Parents’ country of origin

2 4 6 8 10 12 14 16 18 20

Number of children

Computer graph making

Make a column graph and a pie graph of the data presented in the table and graph above.

You may need to fill in a data grid that looks like this.

Examples of what the graphs may look like are displayed below.

Litres and millilitres

Three sets of beakers have been filled with red, green and orange cordial. Record in millilitres, then litres and millilitres, how much cordial is in each pair.

How many more millilitres are needed to make 1 litre?

Convert the millilitre measurements to litres and millilitres. The first one has been done for you.

Generating multiplication facts 34

The numbers 24, 36, 40 and 35 are represented in 4 separate fact family number bonds. Complete the number bonds then write related facts for each number bond. The first one has been started for you.

Find the missing numbers in the multiplication balances.

Complete the multiplications. The first one is done for you.

Find the missing numbers in the multiplications below.

A landscape gardener ordered the following materials. How much did they cost him?

Use the legend to answer the questions.

a How many towns are on the island?

b How many tourist attractions are marked on the map?

c How many bridges are on the island?

d Is it possible to drive to the tourist attractions?

e Which town can only be reached by road?

f How many rivers are on the island?

g Which town can only be reached by train?

h Name one town that can be reached by road, train and by walking.

i If I was at Guitar, would I have to pass through Rap to get to Ego?

j Which town is south of Rock?

k Which two towns does the ferry help join?

Use north, south, east and west to give the directions from:

a Hotville to Cool

b Jazz to Rock

d Explorer’s Hut to Ego

e Cool to Ghost Rock

c Rock to Hotville f

Lagoon to Long Lake

Displacement experiment 1—to compare the volume of 3 objects

a Half fill a clear measuring jug with water and mark the water level.

b Find 3 different shaped objects, such as stones, that can be submerged in your container and tie a piece of string to each one.

c Submerge the first object in the container and mark the water level before taking it out.

d Submerge the second object and mark the water level before taking it out.

e Submerge the third object and mark the water level before taking it out.

f Which object has the greatest volume?

g Discuss your experiment results with a friend and see if their results were similar to yours.

Displacement experiment 2—to measure the amount of overflow

a Place a container such as a jug on a tray.

b Fill the jug to the brim with water.

c Gently place a large rock or stone in the jug.

d Watch as water flows over the top of the jug and onto the tray.

e Measure the water in the tray by pouring it into a beaker. mL

Which experiment do you think gave the more accurate measure of volume?

35 4-digit subtraction with trading

• Subtract the ones. Can’t take 6 from 0 so trade a ten for 10 ones.

10 take away 6.

• Subtract the tens. Trade a 100 for

10 tens. 14 tens take away 8 tens.

• Subtract the hundreds.

6 hundreds take away 1 hundred.

• 8 thousands take away 2 thousands.

• Even + Even = Even, e.g. 42 + 36 = 78

• Even − Even = Even, e.g. 96 − 54 = 42

• Odd + Odd = Even, e.g. 33 + 45 = 78

• Odd − Odd = Even, e.g. 89 − 35 = 54

• Even + Odd = Odd, e.g. 44 + 33 = 77

• Even − Odd = Odd, e.g. 76 − 25 = 51

• Even × Even = Even, e.g. 6 × 4 = 24

• Even × Odd = Even, e.g. 6 × 3 = 18

• Odd × Even = Even, e.g. 9 × 6 = 54

• Odd × Odd = Odd, e.g. 5 × 5 = 25

Knowing the properties of odd and even numbers can help when checking my answers.

Complete the examples to test the odd and even number rules.

Complete a ‘spot test’ on Sally’s answers. Tick the ones you think are correct and cross the ones you think are wrong. The first one has been done for you.

Tom the truck driver is superstitious, so he only carries even amounts on his truck. Complete the calculations below to see whether he would carry these loads.

a 15 bags of potatoes each with a mass of 5 kilograms

b 36 boxes of grapes with a mass of 9 kilograms each

Use multilink or similar building blocks to construct the objects drawn on the isometric dot paper.

The references on the map below are more specific. Although they are read the same, horizontal before vertical, they are different. They are called coordinates and they show the accurate point where two lines meet.

For example, (C,3) is where the circle is on the same grid.

What letter is found at:

Find the street that is:

a parallel to Rafter St and found at (L,7)

b parallel to Barty St and found at (G,6)

c parallel to Seles St and found at (F,1)

d parallel to Park St and found at (G,8)

e parallel to Hopman Rd and found at (A,1)

Write a set of directions describing how to get from K to G.

Estimate an answer to the following by rounding to the nearest 100.

e 387 + 496

f 2417 + 394

g 1201 + 1299

j A farmer has 8

with 26 cows in each. How many cows does the farmer have?

k The 4 grades at Woy Wong Primary School have 52 pupils in each. How many pupils are there in the primary school?

l Ben had a balance of $9560 in his bank account. How much is left if he spent $2590 on a new bike?

Record the decimal fractions on the decimal place value grid. Ones

Convert these measurements into metres using decimal notation.

Order the

a Which colour is most likely to be spun?

b Is it more likely that blue will be spun than red?

How we get to school

Sam filled two containers to the 400 mL mark then placed a rock in each of them.

How much more water was displaced by the second rock than the first? mL

Insert a column graph to show the data shown on the pie chart.

abacus

An instrument used for calculating.

ascending order

An arrangement of numbers from smallest to largest.

256, 291, 307, 452

associative property

Thou Hund Tens Ones

acute angle

An angle less than 90°.

acute angle

addition (+)

The operation that finds the sum or total. am (ante meridiem)

The morning. Any time from midnight to noon, e.g. 7:30 am is 7:30 in the morning.

analog clock

A clock face with numbers 1 to 12, and two hands.

A series of numbers can be added in any order without changing the result.

5 + 4 + 6 = 15

4 + 6 + 5 = 15

6 + 5 + 4 = 15

A series of numbers can be multiplied in any order without changing the result.

5 × 4 × 3 = 60

4 × 3 × 5 = 60

3 × 5 × 4 = 60

axis of symmetry

An imaginary line that divides a shape exactly in half. If a shape is folded along this line, both sides will match.

DRAFT

The amount of turn between two arms around a common endpoint (the vertex).

base

The bottom line of a 2D shape.

base

of turn

The surface covered by any 2D shape. Area can be measured in cm2, m2, hectares and km2. 3 cm

2 cm

The bottom face of a 3D object.

base

Area = 6 cm2

array

An arrangement of objects or symbols into rows and columns.

For example:

• pyramids have one base base

For example:

• prisms have two bases.

capacity

base base

The amount a container can hold. Capacity can be measured in millilitres (mL), litres (L) and kilolitres (kL).

centimetre (cm)

A unit for measuring length. 100 cm = 1 metre

circle

A plane shape bounded by a continual curve that is always the same distance from the centre point.

column graph (bar graph or bar chart)

A column graph generally uses vertical columns to represent data. In a bar graph or bar chart the bars can be either vertical or horizontal.

Number of students

commutative property

Two numbers can be added in any order to give the same total.

15 + 13 = 28 13 + 15 = 28

Two numbers can be multiplied in any order to give the same product.

5 × 4 = 20 4 × 5 = 20

compass points

The cardinal compass points are north, south, east and west.

cone

(north)

(east) (west) E W N S

(south)

coordinate points

Coordinates locate points on a grid using ordered pairs. The horizontal position is given before the vertical position, e.g. the circle is located at (C,3).

corner (vertex)

The point where two or more lines meet to form an angle.

cross-section

The face that is left when a solid (3D) object is cut through, parallel with its base.

cube

A 3D object with six square faces, eight corners and twelve edges.

cubic centimetre

A unit of volume. A centimetre cube has a volume equal to one cubic centimetre.

cylinder

corner

1 cm 1 cm

1 cm

A 3D object with a circular base, tapering to a point (the apex).

An object with two circular faces and one curved surface.

data

Information gathered together, such as a set of numbers or facts.

decade

Ten years. Example: 2013–2023

Also, a group of tens.

decimal

A fraction can be written as a decimal, e.g. 75 out of 100 can be written as 0.75 in decimal form.

Blue Orange Red Yellow Green

Favourite colour Colours

decimal point

A point used to separate the fraction part from the whole number.

whole number part

decimal point

denominator

4.75

fraction part

The bottom number of a fraction that tells how many equal parts there are in the whole.

1 4 numerator denominator

descending order

An arrangement of numbers from largest to smallest.

108, 99, 76, 54

edge

The intersection of two faces on a 3D object.

equivalent fractions

Fractions having the same value.

edge

1 2 2 4 even number

A number that can be divided equally by two. E.g. 2, 4, 6, 8, 10, 12. faces

The surfaces of a 3D object. face face face

diagonal

A straight line which joins two non-adjacent corners of a polygon.

digital clock

A clock which displays only numbers. It has no hands.

dimension

diagonal

flip (reflect)

To turn a shape over.

fraction

12:01

A measurement of length, width (breadth) or height.

height width (breadth) length

division (÷)

The operation that breaks groups or numbers into equal parts. 15 ÷ 3 = 5

double

Multiply by two.

Any part of a whole or group.

front view

The view we see when we look at an object from the front.

3D object

front view

gram

A unit for measuring mass.

1000 grams = 1 kilogram

greater than (>)

The ‘greater than’ symbol shows the relationship between two unequal numbers.

8 > 5

grid references

Grid references locate positions on a map or grid. The horizontal position is given before the vertical position, e.g. the circle is located at C3.

kilometre (km)

A unit of length.

1 km = 1000 metres

grouping

A way of dividing an amount into equal-sized groups.

2 groups of 4 in 8

hexagon

A 2D shape with six straight sides.

length

The longer of the two dimensions of a shape. width (or breadth) length

less than (<)

The ‘less than’ symbol shows the relationship between two unequal numbers.

5 < 8

line of symmetry

A line which divides a shape in half exactly. Shapes can have more than one line of symmetry.

line of symmetry

regular hexagon

horizontal

irregular hexagon

At right angles to the vertical.

vertical line

horizontal line

hundredth

One part of a whole that has 100 parts altogether.

kilogram (kg)

The base unit for measuring mass.

Flour 1 kg

1 kg = 1000 grams

litre (L)

A unit of capacity.

1 L = 1000 millilitres

mass

DRAFT

The amount of substance in an object.

1000 grams = 1 kg

1000 kg = 1 tonne

metre (m)

A unit of length.

1 metre = 100 cm

millilitre (mL)

A unit of capacity. An object with a volume of 1 cm3 displaces 1 mL of water.

millimetre (mm)

A unit of length.

multiple

1000 mL = 1 litre

1 cm 1 cm

1 cm

10 mm = 1 centimetre

The result of multiplying a given number by any other number is a multiple of that given number.

Multiples of 4 are: 4, 8, 12, 16, 20, etc.

Multiples of 5 are: 5, 10, 15, 20, 25, etc.

multiplication (×)

The operation which finds the product of two or more numbers. Multiplication can be seen as repeated addition.

2 + 2 + 2 + 2 + 2 = 10 5 × 2 = 10

parallelogram

A four-sided 2D shape which has two pairs of opposite sides that are parallel and of equal length.

pattern

net

A flat shape that can be folded to make a 3D object.

number line

A line on which numbers are marked. Number lines can be used to represent operations.

3 + 5 = 8

0 1 2 3 4 5 6 7 8 9 10

number pattern

Any set of numbers that follow a pattern or sequence.

1, 3, 5, 7, ___ , ___ , (The pattern is + 2)

3, 9, 27, ___ , ___ , (The pattern is × 3)

numeral

Any figure used to represent a number, e.g.

0, 1, 2, 3, 4, 5

A series of shapes, letters, numbers or objects arranged in a recurring sequence, e.g.

4, 14, 24, 34 … or

pentagon

A 2D shape with five straight sides.

regular pentagon

perimeter

irregular pentagon

The distance around the edges of a shape.

Perimeter = 3 m + 4 m + 5 m

3 m 5m

4 m

numerator

The top number of a fraction, telling us how many equal parts there are out of the whole.

octagon

Perimeter = 12 m

perpendicular lines

Lines that intersect at right angles.

3

4 numerator denominator

A 2D shape with eight straight sides.

picture graph

A graph that uses symbols to represent quantities.

Favourite animals

regular octagon irregular octagon

odd number

A number that is not divisible by two, e.g.

1, 3, 5, 7, 9, 11

parallel lines

Two or more lines that never meet and are exactly the same distance apart along their entire length.

The value

digit determined by its place in the number.

plan

A diagram from above, showing the position of objects.

quarter

One-fourth of a whole or a group.

= 1 4 1 4 are girls

plane shape

A 2D shape.

Example: octagon

pm (post meridiem)

After midday. Any time between noon and midnight, e.g. 7:30 pm is 7:30 in the evening.

polygon

A 2D shape with three or more angles and straight sides.

prism

A 3D object that has a pair of congruent parallel bases that are polygons, and rectangular side faces.

product

Example: octagon

rectangle

A four-sided 2D shape with four right angles and two pairs of parallel sides that are also equal.

square oblong

reflect (flip)

To turn a shape over.

rhombus

A four-sided 2D shape with all four sides equal. A rhombus has two pairs of parallel sides and its opposite angles are equal.

The answer achieved by multiplication.

9 × 3 = 27

product

right angle

An angle of 90°.

rigid

pyramid

A 3D object that has only one base and all the other faces are triangular, meeting at a point (the apex).

quadrilateral

A 2D shape with four sides, e.g. a square, a rectangle, a rhombus and a parallelogram. rhombus

A fixed shape that cannot be pulled out of shape.

rotate (turn)

To turn an object around a fixed point.

rounding

Changing an exact value to an estimated value of a more convenient size.

69 70 (rounded to the nearest 10)

785 800 (rounded to the nearest 100)

side view

The view we see when we look at an object from the side.

3D object side view

skip counting

To count by adding the same number each time.

4… 8… 12… 16… 20…

Skip counting by 4.

slide (translate)

To move a shape to a new position without turning it.

sphere

A perfectly round 3D object, e.g. a ball.

square kilometre (km2)

A unit for measuring area.

1 km

1 km

1 km × 1 km = 1 km2

square metre (m2)

A unit for measuring area.

1 m

1 m

1 m × 1 m = 1 m2

subtraction (−)

The operation which removes part of a group, and finds the difference.

square

A 2D shape with four equal straight sides and four right angles. A square is also a rectangle.

square centimetre (cm2)

A unit for measuring area.

1 cm

1 cm

1 cm × 1 cm = 1 cm2

DRAFT

9 5 = 4

difference

sum

The answer of addition. The total.

symmetry

A shape has line symmetry if both parts match when it is folded along a line.

tally

To keep count by placing a stroke to represent each item. The fifth stroke crosses the four preceding strokes each time.

= 22

tessellation

A pattern formed by the repetition of shapes so that they fit together without gaps.

three-dimensional (3D)

A description of solid objects having three dimensions: length, width (breadth) and height. length

time

twelve-hour time

Time shown by traditional clocks and watches, divided into 12 hours.

twenty-four hour time

width

height (breadth)

60 seconds = 1 minute

60 minutes = 1 hour

24 hours = 1 day

365 days = 1 year

366 days = a leap year

timeline

A line representing a span of time.

Born School High school Work Married

1998 2003 2008 2013 2018 2023

tonne

A unit of mass.

top view

1000 kg = 1 tonne

The view we see when we look at an object from the top.

3D object

translate (slide)

To move a shape to a new position without turning it.

trapezium

A four-sided 2D shape with only one pair of parallel sides.

triangle

A 2D shape with three sides and three angles.

turn (rotate)

To move an object around a fixed point.

Time divided into 24-hour time intervals numbered from 1 to 24, so as to distinguish between times in the morning and times in the afternoon.

two-dimensional (2D)

Plane shapes have only two dimensions: length and width (breadth).

vertex (corner)

The point where two or more lines meet to form an angle.

vertical

DRAFT

top view

vertex

At right angles to the horizontal. horizontal line vertical line

volume

The amount of space a 3D object occupies.

volume = 2 × 3 × 2 = 12 m3

whole numbers

The counting numbers from one to infinity. 1, 2, 3, 4,

width (breadth)

The shorter of the two dimensions of a shape.

width (breadth)

length

80 + 40 = 120 800 + 400 = 1200

g 9 + 6 = 15 90 + 60 = 150 900 + 600 = 1500

3 a $170 d $90

b 120 potatoes e 110 books

c $140

4 a 2 × 3 = 6 c 5 × 3 = 15

b 3 × 3 = 9 d 4 × 3 = 12

5 a 3, 6, 9, 12, 15, 18, 21, 24, 27, 30

b 9, 15, 21, 18, 24, 6, 3, 0, 30, 12

6 a 1 c 2 e 6 g 7 i 10

b 3 d 4 f 5 h 8 j 9

7 Hands on.

8 Cylinders f j

Cones b c g

Spheres a h

Prisms d e k l

Pyramids i m

9 Hands on.

10 Hands on. (It has 2 bases and 3 rectangular sides.)

11 a 15 cm c 6 cm e 11 cm

b 7 cm d 9 cm f 3 cm

12 100 cm

13 a 10 cm d 11 cm g 5 cm

b 8 cm e 9 cm

c 12 cm f 7 cm

14 a 100 cm e 800 cm i 250 cm

b 300 cm f 700 cm j 25 cm

c 400 cm g 50 cm k 75 cm

d 600 cm h 150 cm l 125 cm

5 a 13, 16, 19, 22 Add 3

b 21, 26, 31, 36 Add 5

c 61, 71, 81, 91 Add 10

d 25, 22, 19, 16 Subtract 3

e 24, 20, 16, 12 Subtract 4

f 80, 75, 70, 65 Subtract 5

6 Hands on.

7

b Year book 2014 f

c O g Top row, fourth

d Year book 2017 from the left.

9 a Wang Shu e Aimee and Nick

b Angel f Simone

c Angel g Aimee and Nick

d 3 h 48

10

Most books donated to library

Poppy Con Hassan Max Cooper Lara

b The Walters family Item Cost

5 kg of potatoes $10

2 kg of mushrooms $12

3 cabbages $ 9

7 kg of beans $14

3 kg of tomatoes $12 Total $57

7 Hands on.

8 a I am a prism that has 6 rectangular faces.

b I am a pyramid that the Ancient Egyptians built. I have a square base.

c I am a pyramid that has 5 triangular faces and a pentagon as a base.

d I am a pyramid that has a six-sided shape as a base.

e I am an object with 2 circles as bases.

f I am a prism that has 2 pentagonal faces and 5 rectangular faces.

9 Hands on.

10 Hands on. (Prisms have two bases and rectangular sides. Pyramids have one base and triangular sides.)

11 Hands on.

13 Hands on. (Prism with two hexagonal bases and 6 rectangular sides.) 14

Words This means that it is: a Two forty-five am 45 minutes past 2 in the morning b Nine fifteen pm 15 minutes past 9 in the evening

Six twenty-nine pm 29 minutes past 6 in the evening

Part 4

a 12, 20, 16, 8, 24, 36, 28

b 12, 24, 30, 18, 42, 36, 48

c 21, 35, 28, 56, 42, 49, 63

Part 5

a Shade one part of the shape.

b Shade three parts of the shape.

c True d True e False f False

7

8 a Most likely orange

Least likely green

b Most likely green

Least likely red

c Most likely yellow

Least likely red

d Most likely pink

Least likely blue e Hands on.

10 Hands on. (Rolling a double number, odd or even, must equal an even number.)

11–13 Hands on.

1 a 3, 6, 9, 12, 15, 18

b 8, 16, 24, 32, 40, 48

c 15, 30, 45, 60, 75, 90

d 17, 20, 23, 26, 29, 32

e 10, 14, 18, 22, 26, 30

f 0, 6, 12, 18, 24, 30

g 23, 27, 31, 35, 39, 43

h 42, 47, 52, 57, 62, 67

i 56, 50, 44, 38, 32, 26

j 60, 56, 52, 48, 44, 40

2 a Adding 2

b Subtracting 3

c Adding 4

Adding 6

Doubling

UNIT

6

on. (Example: multiply by 4 then multiply by 2 or vice versa.)

6 Because the answers make a pattern.

7 Hands on (see chart above)

8 Hands on (yes).

9 Hands on. (9 times tables are part of 3 times tables. Both follow a diagonal pattern.)

DIAGNOSTIC REVIEW

UNIT

1 a 868 b 879 c 585

d 999 e 689

2 a 782 b 962 c 683

d 886 e 982

3 a 815 b 949 c 893

d 822 e 723

4 a 324 b 924 kg

Hands on. 14 a 1000 g f 500 g

b 2000 g g 2500 g

c 3000 g h 250 g

d 5000 g i 1250 g

e 7000 g j 750 g

UNIT

1 a 118 f 846 k $7.88

b 992 g 1695 l $6.85

c 992 h 1040 m $6.85 d 1040 i 9564 n $77.72

6 a Possible solution: Eight sides the same length. Bottom edge is flat. Opposite sides are parallel. Divide a circle into eighths and then join the edges.

b Possible solution: Five sides the same length. Bottom edge is flat. Divide a circle into fifths and then join the edges.

7 Hands on.

8 Hands on. (One has 3 dimensions and the other has 2 dimensions. One is a cube and the other is a square.)

9 Hands on.

10 Hands on. (The shape of 1 m x 1 m has just been rearranged.)

11–12 Hands

Part

DIAGNOSTIC REVIEW

12-15 Discussion, most likely responses are: 12

16

17 2 1 2 notes should be added to represent $20 + $20 + $10

UNIT

1 a 43, 86, 129, 172, 215, 258, 301

b 123, 148, 173, 198, 223, 248, 273

c 40, 95, 150, 205, 260, 315, 370

d 706, 666, 626, 586, 546, 506, 466

e 4, 8, 16, 32, 64, 128, 256

f 64, 32, 16, 8, 4, 2,

DRAFT

16 a 1500 millilitres 1 litre 500 mL

b 1400 millilitres 1 litre 400 mL c 1700 millilitres 1 litre 700 mL

17 a 800 mL d 500 mL g 760 mL

b 750 mL e 720 mL h 820 mL

c 650 mL f 400 mL

18 Millilitres Litres and millilitres

a 1800 mL 1 L and 800 mL

4

b 1600 mL 1 L and 600 mL

c 1900 mL 1 L and 900 mL

d 1250 mL 1 L and 250 mL

e 2300 mL 2 L and 300 mL

f 5400 mL 5 L and 400 mL

g 3450 mL 3 L and 450 mL

h 4500 mL 4 L and 500 mL

DIAGNOSTIC