Maths Plus NSW SB and AB 6 Draft Sample Pages

Harry O’Brien

Greg Purcell

NEW SOUTH WALES SYLLABUS

The Maths Plus NSW Syllabus/Australian Curriculum series, Year K to Year 6, is based on the NSW Education Standards Authority 2023 Mathematics K-6 Syllabus for the Australian Curriculum Mathematics (ACARA). Each book after Year K builds upon prior knowledge and works towards an understanding of the achievement standards for the relevant year level and beyond. Maths Plus provides students with opportunities to sequentially develop their skills and knowledge in the strands of the Australian Curriculum Mathematics: Number, Algebra, Measurement, Space, Statistics and Probability

Series components

Student Books

Student Book features

• All pages are colour coded.

Number and Algebra

and Space

Statistics and Probability

Work towards achieving the relevant outcomes by developing skills and competency in understanding mathematical structures, fluency, reasoning and problem solving.

Mentals and Homework Books

Provide concise, essential revision and consolidation activities that correspond with the concepts and units of work presented in the Student Books.

Assessment Books

Include short post-tests with a simple marking system to assess students’ skills and understanding of the concepts in the Student Books.

• Australian Curriculum Mathematics content descriptions, proficiency strand references and general capabilities appear on each page.

• The Dictionary (Years 2 to 6) features clear and simple explanations of mathematical terms and language.

Diagnostic term reviews

• Diagnostic term reviews (Years 1 to 6) assist in pinpointing students’ strengths and weaknesses, allowing intervention and re-teaching opportunities where required.

• The Find a topic page allows teachers the freedom to address particular topics and student needs as appropriate, providing essential revision and consolidation opportunities.

SAMPLEPAGES

Teacher Book and Teacher Dashboard

Provide access to a wealth of resources and support material:

• curricula and planning documents

• interactive teaching tools

• potential difficulties videos

• learning activities

• support and extension activities

• reflection

• blackline masters and investigation pages

• links to Advanced Primary Maths (Years 3 to 6)

• assessment tests

• answers for student resources

www.oxfordowl.com.au

Oxford Owl is the home for Oxford Primary professional resources.

MEASUREMENT AND SPACE

NSW Syllabus Outcomes

MA3-RN-01

Applies an understanding of place value and the role of zero to represent the properties of numbers

MA3-RN-02

Compares and orders decimals up to 3 decimal places

MA3-RN-03

Determines percentages of quantities, and finds equivalent fractions and decimals for benchmark percentage values

MA3-AR-01

Selects and applies appropriate strategies to solve addition and subtraction problems

MA3-MR-01

Selects and applies appropriate strategies to solve multiplication and division problems

MA3-MR-02

Constructs and completes number sentences involving multiplicative relations, applying the order of operations to calculations

MA3-RQF-01

Compares and orders fractions with denominators of 2, 3, 4, 5, 6, 8 and 10

MA3-RQF-02

Determines

MA3-GM-01

Locates and describes points on a coordinate plane

MA3-GM-02

MA3-GM-03

of measures and quantities

MEASUREMENT AND SPACE

Selects and uses the appropriate unit and device to measure lengths and distances including perimeters

Measures and constructs angles, and identifies the relationships between angles on a straight line and angles at a point

MA3-2DS-01

Investigates and classifies two-dimensional shapes, including triangles and quadrilaterals based on their properties

MA3-2DS-02

Selects and uses the appropriate unit to calculate areas, including areas of rectangles

MA3-2DS-03

Combines, splits and rearranges shapes to determine the area of parallelograms and triangles

MA3-3DS-01

Visualises, sketches and constructs three-dimensional objects, including prisms and pyramids, making connections to two-dimensional representations

MA3-3DS-02

Selects and uses the appropriate unit to estimate, measure and calculate volumes and capacities

MA3-NSM-01

Selects and uses the appropriate unit and device to measure the masses of objects

SAMPLEPAGES

MA3-NSM-02

Measures and compares duration, using 12- and 24-hour time and am and pm notation

MA3-DATA-01

Constructs graphs using many-to-one scales

MA3-DATA-02

Interprets data displays, including timelines and line graphs

MA3-CHAN-01

Conducts chance experiments and quantifies the probability

MAO-WM-01 Working mathematically

STATISTICS AND PROBABILITY

Develops understanding and fluency in mathematics through exploring and connecting mathematical concepts, choosing and applying mathematical techniques to solve problems, and communicating their thinking and reasoning coherently and clearly

MA3-NSM-02

MA3-DATA-01

MA3-DATA-02

MA3-CHAN-01

MAO-WM-01 Working mathematically

STATISTICS AND PROBABILITY

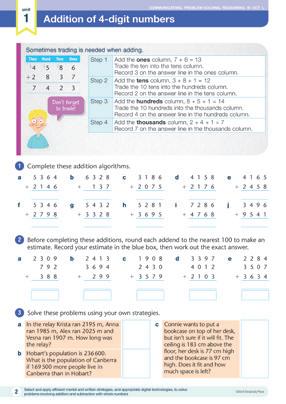
Addition of 4-digit numbers/estimation

Step 1 Add the ones column, 7 + 6 = 13

Trade the ten into the tens column. Record 3 on the answer line in the ones column.

Step 2 Add the tens column, 3 + 8 + 1 = 12

Trade the 10 tens into the hundreds column. Record 2 on the answer line in the tens column.

Step 3 Add the hundreds column, 8 + 5 + 1 = 14

Trade the 10 hundreds into the thousands column. Record 4 on the answer line in the hundreds column.

Step 4 Add the thousands column, 2 + 4 + 1 = 7

Record 7 on the answer line in the thousands column.

Complete these addition algorithms.

SAMPLEPAGES

Before completing these additions, round each addend to the nearest 100 to make an estimate. Record your estimate in the blue box, then work out the exact answer.

Solve these problems using your own strategies.

a In the relay, Krista ran 2195 m, Anna ran 1985 m, Alex ran 2025 m and Vesna ran 1907 m. How long was the relay?

b Hobart’s population is 236 600. What is the population of Canberra if 169 500 more people live in Canberra than in Hobart?

c Connie wants to put a bookcase on top of her desk, but isn’t sure if it will fit. The ceiling is 183 cm above the floor, her desk is 77 cm high and the bookcase is 97 cm high. Does it fit and how much space is left?

Use your knowledge of number facts to answer these questions.

Multiply by 10, then halve to multiply by 5.

Use the ‘double, then double again’ strategy to multiply by 4. Or use the ‘double, double, double’ strategy to multiply by 8.

Round to the nearest 10 or 100 to make an estimate of these multiplications.

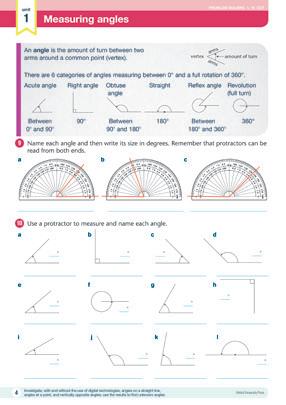
Measuring angles

An angle is the amount of turn between two arms around a common point (vertex). vertex arm arm amount of turn

There are 6 categories of angles measuring between 0° and a full rotation of 360°.

Acute angle Right angle Obtuse Straight Reflex angle Revolution angle (full turn) Between 90° Between 180° Between 360° 0° and 90° 90° and 180° 180° and 360°

Name each angle and then write its size in degrees. Remember that protractors can be read from both ends.

Use a protractor to measure and name each angle.

SAMPLEPAGES

Longer distances are measured in kilometres. There are 1000 m in 1 kilometre.

SAMPLEPAGES

km km km km km

f Kedron km

g Coopers Plain km

h Hamilton km

i Mt Gravatt km

j Woolloongabba km

k Graceville km

Record these distances in kilometres using decimal notation. a 9154 metres = 9.154 km

Sheree keeps a log book of all her work trips. On the left is the odometer reading at the start of her day. Show the odometer reading at the end of the day if she made three trips.

Addition and subtraction strategies

Add these numbers mentally.

Give an estimate for each question by rounding each number. The first one has been done for you.

Subtract these numbers

7 Estimate first, then solve the problems mentally.

SAMPLEPAGES

a Joseph had 155 sheep in one paddock and 38 sheep in another. How many sheep did he have altogether?

b Marina had 379 g of flour and 122 g of sugar. If she mixed them together, how much would the mixture weigh?

c Jessica had a collection of 156 hair clips but sold 39 of them. How many hair clips does she have left?

d Sai travelled 1106 km on Tuesday and 488 km on Wednesday. How far has he travelled altogether?

e Uncle Sam’s Car Sales had 173 vehicles in the lot. If 58 of them were damaged by hail, how many were not damaged?

Percentages, fractions and decimals

A percentage is another way of recording a fraction with a denominator of 100. Per cent means ‘out of 100’. A percentage sign % is used to display percentages. EXAMPLE 85 100 can be written as 85%.

SAMPLEPAGES

Compares and order decimals up to 3 decimal places

Determine percentages of quantities, and find equivalent fractions and decimals for benchmark percentage values

Revising shapes 2

Name each shape, then record the number of sides and angles on each.

Which shapes above are parallelograms?

Draw an example of each shape. The 5 mm dot paper may help you.

What am I? I am not a square but I have 4 sides of equal length and my opposite angles are equal.

Investigate and classify two-dimensional shapes, including triangles and quadrilaterals based on their properties

Square centimetres and square metres 2

The formula used to calculate the area of a rectangle is Area = length × width. Width Length

Break these shapes into rectangles in order to work out their area. The first one has been done for you.

Selects and use the appropriate unit to calculate areas, including areas of rectangles

Subtracting 4-digit numbers/problems

Step 1 7 from 6 can’t do. Trade a 10 from the tens column. There are now 7 tens in the tens column and 16 ones in the ones column. 16 7 = 9

Record 9 on the answer line in the ones column.

Step 2 5 tens from 7 tens = 2 tens

Record 2 on the answer line in the tens column.

Step 3 9 hundreds from 4 hundreds can’t do. Trade 1000 from the thousands column.

There are now 5 thousands in the thousands column and 14 hundreds in the hundreds column. 14 9 = 5

Record 5 on the answer line in the hundreds column.

Step 4 2 thousands from 5 thousands = 3 thousands.

Record 3 on the answer line in the thousands column.

1 Complete each algorithm.

2 Fill in the empty boxes to complete these algorithms.

Trade the 2 hundreds left for 20 tens. Now share the 26 tens. Each school gets 5. 1 5 | 7 6 7 1 5 5 | 726 7 1 5 3r2 5 | 72617

Trade the 1 ten left over for 10 ones. Now share the 17 ones. Each school gets 3 and there is a remainder of 2.

a Which team won the game?

b What was the average score of each Wombat?

c What was the average score of each Galah?

d Which Wombats scored higher than the team average?

e Which Galahs scored less than the team average?

Create 4 different divisions that have a remainder of 2. 6 r2 r2 r2 r2 MP_NSW_SB6_38350_TXT_4PP.indb 11 25-Aug-23 17:54:22 SAMPLEPAGES

Mr Cook did a survey to find out how children most commonly use technology, then recorded the data in the form of a picture graph.

KEY = 10 children

Use the key to answer the questions.

a How many children answered Study?

b How many children answered Internet?

c How many children answered Messaging?

d How many more answered Internet than Study?

e What were the least common answers?

Technology use

Games Study Messaging Internet Email

Ms Zhang’s class did a survey to find out the most common eye colour of senior students. They made a table of the data. Eye colours

Colour Tally

Blue

Green

Brown Hazel

Create a picture graph using the given key.

= 4 children

Answer the questions.

a Which eye colour was the most common?

b Which eye colour was the least common?

c How many more children had blue eyes than brown eyes?

Blue Green Brown Hazel

Explain how you could make this survey more reflective of the whole population of people in NSW.

Constructing angles

3

Use a protractor to construct the following acute, obtuse and right angles with the vertex on the left. •

acute angle 20°

vertex

obtuse angle 160°

acute angle 30°

obtuse angle 120°

right angle 90°

acute angle 25°

acute angle 60°

Use a protractor to construct the following angles with the vertex on the right. The first one has been done for you.

obtuse angle 170°

SAMPLEPAGES

acute angle 80°

acute angle 20°

obtuse angle 110°

obtuse angle 130°

right angle 90°

Addition/distance by car 4

Use the chart below to calculate the distance between airports and the total distance of each journey. The first one has been done for you. Round numbers to 100 and check your answers by estimating the distances.

Mark 2 flights on the map. Colour one blue and the other red. Calculate the total distance of each flight. 3 Blue flight Red flight

6

Write the numbers represented on each abacus. Don’t forget ‘0’ as a place holder.

Draw beads on the abacuses to represent the numbers.

Reading

When we read numbers, we read them in groups of hundreds, tens and ones. The following chart best illustrates this concept.

Note: A space separates the millions, thousands and ones when the number is larger than 9999.

Write the numbers on the place value grid before adding the correct spaces to the number. The first one has been done for you.

b 32451

c 736041

d 5425006

e 7325400

f 76245236

Negative numbers

There are many instances in the real world where we need negative numbers. Negative numbers are numbers less than zero written with a minus sign (–) in front of them.

Estimate the distance between these points.

a The shipwreck and the octopus.

b The shipwreck and the hut.

Display how these problems can be calculated on a number line. The first one has been started for you.

=

Apply an understanding of place value and the role of zero to represent the properties of numbers

Classifying three-dimensional objects

Draw a line to match each net and three-dimensional object to its name.

Triangular pyramid

Hexagonal prism

Triangular prism

Rectangular prism

Square pyramid Cylinder

Classify the objects by stating the number of faces, edges and vertices on each.

Object Faces Edges Vertices

a Triangular pyramid

b Hexagonal prism

c Triangular prism

d Rectangular prism

SAMPLEPAGES

Vertex Edge Face

e Square pyramid

What am I?

a I am made from 4 triangles, which are the same shape, and a square. I also have 5 vertices, 5 faces and 8 edges.

b I am made from 2 identical pentagons and 5 identical rectangles. I also have 15 edges, 10 vertices and 7 faces.

Doing multiplication

• 3 × 5 = 15 Write 5 in the ones column and trade the 10 ones for 1 ten. Put 1 in the tens column.

• 3 × 3 tens equals 9 tens, plus 1 ten equals 10 tens. Trade the 10 tens for 1 hundred. Write the 0 in the tens column and a 1 in the hundreds column.

• 3 × 6 hundreds equals 18 hundreds, plus 1 hundred equals 19 hundreds. Write the 9 in the hundreds column and the 1 in the thousands.

Complete these algorithms.

Round to the nearest 10 or 100 to estimate each question, then answer them.

Solve the problems.

a 807 people attended a tennis match. If they all paid $8 each, how much money was collected? $

b Maria bought a new phone. How much did it cost her if she paid a $100 deposit and made 8 monthly payments of $129? $

11:30

Decimals, percentages and fractions

Use your calculator to convert the fractions to decimals by dividing the numerator by the denominator. Once you have converted them to a decimal, write them as a percentage as well.

Write some fractions, then convert them to decimals then percentages with a calculator.

Courtney has 5 cards with 2D shapes drawn on them. She is going to put them in a bag and will get her friend to pick one out.

Predict the probability of each shape card being selected as her first pick. Record each probability as a fraction.

Experiment

a Make a set of cards like the ones above.

b Place your cards in a bag and make 20 draws using all 5 cards each time.

c Use tally marks ( IIII ) to record your results.

Explain how your experiment results compare with your predictions in Question 7.

Jimmy has bought 100 lollies for his party. They only came in 4 colours. He put his hand in his bag and pulled out the following sample of 10 lollies.

R Y B R B B R P B P

Use the sample above to estimate how many lollies of each colour could be in the bag of 100 lollies.

a red b blue c pink d yellow

If there were a bag of 500 lollies and Jimmy pulled out the same 10 lollies, how many of each colour do you estimate could be in the bag?

a red b blue c pink d yellow

Estimating mass

10 Estimate and then use calibrated scales or balance scales to measure the mass of each item. Estimate only one mass at a time.

h Did your estimates improve as you worked through the exercise?

11 Ten grams

Estimate first and then measure to see how many of each object are needed to balance 10 g.

How many of each item are needed to fill the boxes below?

2673

Division strategies/problems

Trade the 1 hundred left over for 10 tens. Now share the 17 tens. Each paddock gets 3 tens. 5 2673 5 3 5 26 1 73 5 3 4r3 5 261723

Trade the 2 tens left over for 20 ones. Now share the 23 ones. Each paddock gets 4 ones. There is a remainder of 3.

a 5 | 2 6 5 5 b 6 | 3 7 8 6 c 4 | 2 7 2 8 d 4 | 3 9 6 4 e 6 | 4 0 2 6 f 5 | 2 7 5 4 g 6 | 2 7 7 7 h 6 | 2 9 2 6 i 5 | 3 7 7 5 j 4 | 2 6 7 5

a 5 9 3 r 3 | 4 7 4 7 b 5 3 0 r 4 | 4 7 7 4 c 4 4 2 r 6 | 3 5 4 2 d 6 3 2 r 3 | 3 7 9 5 e 4 3 3 r 1 | 3 0 3 2 Music

Island Railway

SAMPLEPAGES

e

Pop

Find a 4-digit number that can be divided by 2, 4 and 8, then complete the divisions. 4

MP_NSW_SB6_38350_TXT_4PP.indb 22 25-Aug-23 17:54:29

5 paddocks.

Number patterns

Follow the instructions to answer the questions. This machine makes 20 milk cartons every minute.

Minutes 1 2 3 4 5 6 7 Cartons 20

This bath fills at a rate of 15 litres every minute.

a Complete the number pattern and record the rule.

b How many cartons would the machine make in 10 minutes?

Minutes 1 2 3 4 5 6 7 Litres 15

This car travels 13 km on every litre of petrol.

Litres 1 2 3 4 5 6 7 Kilometres 13

Radio station Double Z plays 12 songs every hour.

Hours 1 2 3 4 5 6 7 Songs 12

6 7

Number pattern

a Find the pattern in the table below, then complete it.

First number 1 2 3 4 5 6 7

Second number 18 45 63

b Describe the relationship between the first number and the second number.

c What would be the 10th term in the pattern?

c Complete the number pattern and record the rule.

d How many litres would be in the bath after 12 minutes?

e Complete the number pattern and record the rule.

f How far would the car travel on 15 L of petrol?

g Complete the number pattern and record the rule.

h How many songs would be played in 12 hours?

Number pattern.

SAMPLEPAGES

a Find the pattern in the table below, then complete it.

First number 11 12 13 14 15 16 17

Second number 24 26 29

b Describe the relationship between the first number and the second number.

c What would be the 10th term in the pattern?

Side-by-side column graphs

The Walters family is leaving soon on a world trip. They need to know what clothes to take, so they are looking very carefully at the temperatures of the cities they will visit.

Cities of the world: temperatures

Answer these questions.

a Which city has the highest maximum temperature?

b Which city has the lowest maximum temperature?

c Which city has the highest minimum temperature?

d Which city has the lowest minimum temperature?

e How many degrees hotter is Bangkok than Athens?

f How many degrees hotter is Athens than New York?

g Which city has the greatest difference in minimum and maximum temperatures?

h Which city has the smallest difference in minimum and maximum temperatures?

What is the difference between the minimum and maximum temperatures of these cities?

SAMPLEPAGES

What is the average maximum temperature of Melbourne, Sydney and Darwin?

Which minimum temperature appears most often?

Which maximum temperature appears most often?

Constructs graphs using many-to-one scales

Melbourne Sydney Darwin Bangkok Athens

London New York

Tokyo Beijing

Complete the clockfaces for each digital time. The first one is done for you.

SAMPLEPAGES

I think 30 days is equal to 720 hours.