
6 minute read
Predicting the behaviour of reinforced concrete structures
Predicting the behaviour of
reinforced concrete structures
Prof George Markou Dr Nikolaos P Bakas
A multidisciplinary project that involves machine learning algorithms in civil engineering was launched in 2018. The main objective of the project was to develop design formulae to predict the maximum capacity of reinforced concrete structural members through the use of software-generated data that would be made available free of charge to train machine learning algorithms.
This innovative approach has been extended to the structural problem that aims to predict the fundamental period of reinforced concrete structures during the seismic design and assessment of framing systems by accounting for the soil structure interaction phenomenon. Investigating the dynamic response of structures is of significant importance, particularly when it is essential to predict the fundamental period of structures in an accurate and realistic manner. This is crucial to reassure safe designs and a sustainable built environment when designing for seismic loading conditions.
ARTIFICIAL INTELLIGENCE AND MACHINE LEARNING
Artificial intelligence (AI) and machine learning algorithms are involved in a variety of scientific and industrial tasks, and contribute to the solution of corresponding problems, from data modelling and analysis to automatic literature reviews, face recognition and autonomous vehicles.
In recent years, researchers and professionals have been fetching AI algorithms in an unusually wide range of scientific, technological and business fields. Regardless of the database, their basic purpose is to develop a prediction algorithm, which will be accomplished by a mathematical model to describe the complex interactions among some input variables and a corresponding response. The relationship between independent and dependent variables is often highly non-linear, and mathematical models aim to form a generalised relationship that can objectively link them.
The final aim is to develop a numerical model that can predict new outputs for new, out-of-sample inputs, which may be within the given domain (interpolation) or even outside the domain (extrapolation). Predicting out of the limits of a given domain is a hard and highly unstable problem without interesting results. However, a numerical solution was recently published, with extended prediction horizons. This significant breakthrough provided new insights and mathematical tools for this research project.

Designing seismic-resistant reinforced concrete structures is of significant importance when establishing a safer built environment and avoiding loss of life due to earthquake excitations. Understanding the intrinsic characteristics of structures – and specifically their expected dynamic response – is a key factor in the design process. In addition, accounting for the soil-structure-interaction effect when evaluating the dynamic response of a structure is decisive in predicting its dynamic behaviour. The computation of the fundamental period is one of the most important characteristic parameters of structures when analysing and designing in seismically active regions as it strongly affects the magnitude of the computed seismic forces. The simplicity of the current design formulae in predicting the fundamental period appears to be evident, since a detailed modal analysis requires an excessive computational effort in relation to a time-consuming mesh-generation routine. Therefore, the requirement for a more precise computation of the fundamental period of reinforced concrete structures, also accounting for any soilstructure-interaction conditions, is apparent.
In order to alleviate the accuracy limitations related to simplified formulae for the prediction of the fundamental period of reinforced concrete structures, and to account for different soil-structureinteraction conditions, 3D detailed analysis tools were utilised for the generation of a database of 475 cases. The structural systems considered in this research comprised bare frames, since infill walls are not accounted for in Eurocode 8 and the South African National Standard (SANS), given that these non-structural members do not contribute to the resistance of the frame under ultimate limit-state conditions.
According to the numerical investigation performed through the use of different reinforced concrete frames, it was found that the geometry of the frame, the use of dual framing systems and the soil-structure-interaction effects were parameters that controlled the fundamental mode of reinforced concrete buildings. The following parameters were therefore used as variables in the newly developed expressions: fundamental period, height of the building, ratio of the
INVESTIGATING THE DYNAMIC RESPONSE OF STRUCTURES IS OF SIGNIFICANT IMPORTANCE, PARTICULARLY WHEN IT IS ESSENTIAL TO PREDICT THE FUNDAMENTAL PERIOD OF STRUCTURES IN AN ACCURATE AND REALISTIC MANNER. THIS IS CRUCIAL TO REASSURE SAFE DESIGNS AND A SUSTAINABLE BUILT ENVIRONMENT WHEN DESIGNING FOR SEISMIC LOADING CONDITIONS.
total area of shear wall sections along the direction of the seismic action to the total area of the walls and columns at the ground floor, the Young’s modulus for the soil, the length of the building along the seismic action direction, the width of the building perpendicular to the seismic action direction, and the depth of the soil.
Based on the generated dataset, a machine learning algorithm was used to develop a predictive formula (with different characteristics and intrinsic features) and predict the fundamental period of reinforced concrete structures, with and without accounting for the soil-structureinteraction effect. The developed fundamental period formula was validated with 60 out-of-sample fundamental period cases.
According to the numerical investigation, the developed fundamental formula exhibited high prediction accuracy. In addition, the proposed formula was compared to three fundamental period formulae found in the literature that claimed improved predictive capabilities compared to the design code
Conclusion
Based on the research presented in this project, a free-distribution application was developed, which is currently being tested by the research team in the Department of Civil Engineering’s Structures Division. The software application is integrated with the predictive model developed from this research project and will be made available free of charge to anyone who wishes to use it to predict the fundamental period of structures through the use of an AI-generated model. It must be noted that this is the first predictive model of its kind currently found in the international literature. Future research will extend the variety of building geometries, thus increasing the range of structural geometries to which this formula can be applied. Larger models will be used to further investigate (A)
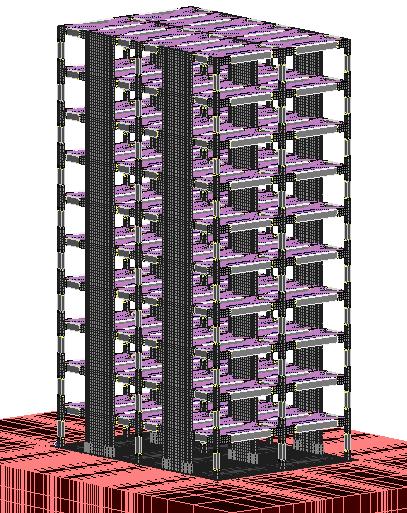

(B)
Finite element meshes of ten-storey reinforced concrete models with (A)
formulae that are currently used in the seismic design of reinforced concrete buildings. Based on the research findings, the proposed fundamental period formula was found to significantly outperform all the current expressions found in the literature, including the prediction capabilities of the different design code formulae. Specifically, the proposed fundamental period formula derived a 5.68% absolute average error on the validation dataset, where Eurocode 8 – the most accurate from the investigated fundamental period design formulae – derived a 45.24% absolute average error. The significant inability of the under-investigation design code formulae was therefore further highlighted through the validation stage, where it was found that the design codes’ inability to realistically predict the expected fundamental period of reinforced concrete buildings was evident.
and without (B) shear walls the dynamic behaviour of reinforced concrete structures to enhance the training dataset. The investigation of infill walls and masonry structures will be the subject of future research to develop more accurate and
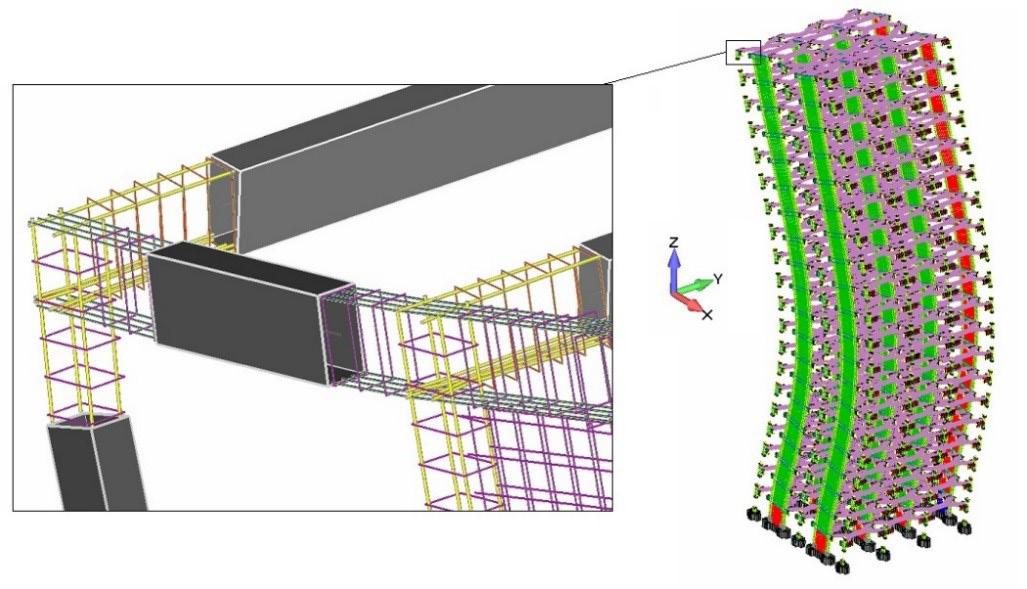
objective fundamental period formulae that will enable civil engineers to obtain realistic predictions for both the design of new structures and the assessment of the dynamic response of existing buildings.
Modal shape of a fixed-base 20-storey reinforced concrete building with shear walls, together with the deformed shape of the 368 773 embedded rebar elements.










