HANDS ON GRADE 3 MATH
TEACHER’S GUIDE
TABLE OF CONTENTS
MISSION 1 Number Forms Memory Game
MISSION 2
Decomposing Numbers
3 Counters for Place Value
4 Rounding Whole Numbers
5 Adding and Subtracting Whole Numbers
6
Multiplying Numbers by a Multiple of 10 or 100
MISSION 8
Understanding fractions
MISSION 9 Building Fractions
MISSION 10
Fraction Forms Memory Game
MISSION 11
Finding Fractions on a Number Line
MISSION 12
Finding Equivalent Fractions
MA.3.FR.1.1
MA.3.FR.1.2
MA.3.FR.1.3
MA.3.FR.2.1
Read and write numbers from 0 to 10,000 using standard form, expanded form, and word form.
Compose and decompose four-digit numbers in multiple ways using thousands, hundreds, tens, and ones. Demonstrate each composition or decomposition using objects, drawings and expressions or equations.
Plot, order, and compare whole numbers up to 10,000.
Round whole numbers from 0 to 1,000 to the nearest 10 or 100.
Add and subtract multi-digit whole numbers including using a standard algorithm with procedural fluency.
Multiply a one-digit whole number by a multiple of 10, up to 90, or a multiple of 100, up to 900, with procedural reliability.
Multiply two whole numbers from 0 to 12 and divide using related facts with procedural reliability.
Exploremultiplicationoftwowholenumberswithproductsfrom0 to144,andrelateddivisionfacts.
Represent and interpret unit fractions in the form 1/n as the quantity formed by one part when a whole is partitioned into n equal parts.
Represent and interpret fractions, including fractions greater than one, in the form of m/nas the result of adding the unit fraction 1/n to itself mtimes.
Read and write fractions, including fractions greater than one, using standard form, numeral-word form and word form.
Plot, order and compare fractional numbers with the same numerator or the same denominator.
MA.3.FR.2.2 Identify equivalent fractions and explain why they are equivalent. 92-100
MISSION 13
Applying Properties of Multiplication MA.3.AR.1.1
Solving with a Dollar
15 Evaluating Equations
HANDS ON GRADE 3 MATH TEACHER
S GUIDE
TABLE OF CONTENTS
Apply the distributive property to multiply a one-digit number and two-digit number. Apply properties of multiplication to find a product of one-digit whole numbers.
Solve one– and two-step real-world problems involving any of four operations with whole numbers.
Determine and explain whether an equation involving multiplication or division is true or false.
Determine the unknown whole number in a multiplication or division equation, relating three whole numbers, with the unknown in any position.
Restateadivisionproblemasamissingfactorproblemusingthe relationshipbetweenmultiplicationanddivision.
Using analog and digital clocks tell and write time to the nearest minute using a.m. and p.m. appropriately.
MISSION 24
Identifying Geometric Figures
MISSION 25
Identifying Quadrilaterals
MISSION 26
Drawing Lines of Symmetry
MISSION 27
Finding Area Using Arrays and Base-Ten Blocks
MISSION 28
Finding Area Using Unit Squares
MISSION 29
Understanding Areas and Perimeters
MISSION 30
Finding Area of Irregular Figures
MA.3.GR.1.1
MA.3.GR.1.2
MA.3.GR.1.3
MA.3.GR.2.1
MA.3.GR.2.2
MA.3.GR.2.3
MA.3.GR.2.4
HANDS ON GRADE 3
TABLE OF CONTENTS
Describe and draw points, lines, line segments, rays, intersecting lines, perpendicular lines and parallel lines. Identify these in twodimensional figures.
Identify and draw quadrilaterals based on their defining attributes. Quadrilaterals include parallelograms, rhombi, rectangles, squares and trapezoids.
Draw line(s) of symmetry in a two-dimensional figure and identify line-symmetric two-dimensional figures.
Explore area as an attribute of a two-dimensional figure by covering the figure with unit squares without gaps or overlaps. Find areas of rectangles by counting unit squares.
Find the area of a rectangle with whole-number side lengths using a visual model and a multiplication formula.
Solve mathematical and real-world problems involving the perimeter and area of rectangles with whole-number side lengths using a visual model and a formula.
Solve mathematical and real-world problems involving the perimeter and area of composite figures composed of nonoverlapping rectangles with whole-number side lengths.
Interpret data with whole-number values represented with tables, scaled pictographs, circle graphs, scaled bar graphs or line plots by solving one– and two-step problems.
Collectandrepresentnumericalandcategoricaldatawithwholenumbervaluesusingtables,scaledpictographs,scaledbargraphs orlineplots.Useappropriatetitles,labelsandunits.
MA.3.NSO.1.2 – Compose and decompose four-digit numbers in multiple ways using thousands, hundreds, tens and ones. Demonstrate each composition or decomposition using objects, drawings and expressions or equations.
OBJECTIVE: Students will identify different ways that a four-digit number can be written using decomposition.
MATERIALS: (Per Group of 4)
10 red counters
60 yellow counters
60 blue counters
60 green counters
4 small paper cups
1 Decomposition sheet
1 marker
LESSON DISCUSSION:
• Explain that a four-digit number can be decomposed in more than one way.
• Explain how regrouping works (10 ones equal 1 ten, 10 tens equal 1 hundred, and 10 hundreds equal 1 thousand.)
Procedure:
Step 1: Print one Decomposition sheet for each group of 4 students.
Step 2: Provide each group with 10 red counters, 30 yellow counters, 30 blue counters, 30 green counters, 4 small paper cups, 1 Decomposition sheet, and 1 marker.
Step 3: Instruct the students to arrange the 4 small paper cups in a row.
Step 4: Tell the students to label the first cup “THOUSANDS”, the second “HUNDREDS,” the third “TENS,” and the fourth “ONES.”
Step 5: Explain to the students that these cups will serve as the place value exchange.
Step 6: Ask the students to take out their Decomposition sheets. As a class, do the first one as an example. Use the colored tokens to decompose the first number. Explain to the students that each red counter represents 1 thousand, each yellow counter represents 1 hundred, each blue counter represents 1 ten, and each green counter represents 1 one.
Step 7: The first number on the Decomposition sheet is 1,234. To decompose this number, place one red counter in the THOUSANDS cup, two yellow counters in the HUNDREDS cup, three blue counters in the TENS cup, and four green counters in the ONES cup. Explain to the students that the counters in the cups represent one way to decompose 1,234. Record this decomposition in the second column of their Decomposition sheet.
Step 8: Instruct the students to take one yellow counter from their HUNDREDS cup. Ask them again what this one yellow token represents (1 hundred).
Step 9: Tell the students to exchange the one yellow counter with ten blue counters, as 1 hundred equals 10 tens. Put the ten blue counters into the TENS cup. Ask the students to write another way to decompose 1,234 using the current number of counters in the cups. Have the students record their chosen ways in the third column of their Decomposition sheet.
Step 10: Instruct the students to take two blue counters from the TENS cup. Ask them again what these two blue counters represent (2 tens).
Step 11: Tell the students to exchange these two blue counters with twenty green counters, as 1 ten equals 10 ones and 2 tens equal 20 ones. The students should add twenty green counters in their ONES cups. Ask the students to write another way to decompose 1,234 using the current number of counters in their cups. The students should record their way in the fourth column of their Decomposition sheets.
Step 12: Have the students use the methods taught to them to complete the rest of the numbers on the Decomposition sheet as a group.
Essential Question for Discussion or Journaling:
Explain how decomposing a multi-digit whole number is related to writing the multi-digit whole number in expanded form.
Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green: Red: Yellow: Blue: Green:
| Hands On Math | Educational Bootcamp
Red:
DIRECTIONS: Decompose each number three different ways.
Explain why using 10 tens instead of 1 hundred does not change the value of the number.
Name: LEVELED PRACTICE 2 – MA.3.NSO.1.2
DIRECTIONS: Decompose each number using ones only, then using tens and ones only, and then using hundred and ones only.
Decompose the number 4,752 in three different ways. How would you draw a picture to represent each of these decompositions?
DIRECTIONS: Use decomposition to fill in the missing values.
4,250 = 4 thousands + 2 hundreds + _____ ones = _____ hundreds + 5 tens
8,060 = 8 thousands + _____ ones = _____ hundreds + 6 tens
1,504 = _____ hundreds + 4 ones = 1 thousands + _____ tens + 4 ones
3,780 = 3 thousands + _____ ones = 3 thousands + _____ tens
5,714 = 5 thousands + _____ hundreds + 14 ones = 5 thousands + _____ tens + 4 ones
7,980 = 7 thousands + 9 hundreds + _____ ones = 7 thousands + _____ tens
9,030 = 9 thousands + _____ ones = _____ hundreds + 3 tens
2,400 = _____ thousands + 40 tens = 2 thousands + _____ ones
Explain why using 100 ones instead of 1 hundred does not change the value of the number.
Ma.3.NSO.2.1 – Add and subtract multi-digit whole numbers including using a standard algorithm with procedural fluency.
Objective: Students will learn how to fluently add and subtract whole numbers using knowledge of place value.
Materials: (Per TEACHER)
1 classroom dry erase board
1 dry erase marker
1 dry eraser
Materials: (Per group of 2 students)
1 regular deck of playing cards
1 Write and Wipe holder
1 Adding and Subtracting Worksheet
1 dry erase marker
1 dry eraser
2 numbered bingo chips
2 tens base-ten blocks
Lesson Discussion:
• Review the different terms in place value, such as ones, tens, hundreds, and thousands.
• Teach the students how to perform addition and subtraction using place value. Help the students understand that when adding or subtracting multidigit numbers using place value, always start from the “ones” place value then move left.
• The students will only add and subtract multi-digit whole numbers in this activity.
PART A:
Teacher Preparation:
Step 1: Remove the tens cards and face cards (Jokers, Jacks, Queens, and Kings) from each deck of cards for each group of 2 students.
Step 2: Write the number 10 on 2 bingo chips for each group of 2 students.
Step 3: Place an Adding and Subtracting worksheet into a Write and Wipe holder for each group.
Step 4: Divide the students into groups of 2. Distribute the materials to each group of 2 students.
Procedure:
Step 1: Write the following example on the classroom dry erase board.
Step 2: Explain that the 3 cards in the top row form a three‐digit number, and the 2 cards in the bottom row form a two‐digit number.
Step 3: Ask the students if they know how to find the sum of 537 and 95. Have the students pull the same cards from their deck to follow along with the example. They should put it in the following formation and take out their bingo chips.
Step 4: Explain that to find the sum of multi‐digit numbers, they should add the numbers with the same place value, starting from the ones place and moving left. Tell the students they should treat each place value as an individual addition problem.
Step 5: Have the students find the sum of the numbers in the ones place. Since 7 + 5 = 12 and 12 = 2 + 10, write 2 in the ones place on the dry erase board, and add the 10 to the tens place drawing the bingo chip to represent the 10 being carried over.
Step 6: Have the students go to the next place value and add the numbers in the tens place. While 3 + 9 = 12, we have one 1 tens from the sum of the ones place. So, we have 12 + 1 = 13 for the tens place. Since 13 = 10 + 3, write 3 in the tens place, and add the 10 to the hundreds place, drawing another bingo chip to represent the 10 being carried over.
Step 7: Now have the students to go to the hundreds place value and add the numbers in the hundreds place. Since we only have 5 in the hundreds place, we add the one 1 hundreds from the sum of the tens place to 5. Hence, 5 + 1 = 6.
Step 8: Tell the students that the sum of 537 and 95 is 632.
Step 9: Have the students turn to Part A of their Adding and Subtracting worksheet, return the cards to their deck, and shuffle the cards together.
Step 10: Tell the students that for the 6 problems on Part A of the worksheet, they are to draw 5 cards from their deck, write the numbers that are represented by the cards in the corresponding boxes, and add.
Step 11: Tell the students that whenever the problem calls for them to carry over, they are to indicate it by coloring in the circle next to the number that has the 10 carried over as they indicated with the bingo chip in Step 5 and 6.
PART b:
Step 1: Explain to students that they will be subtracting multi‐digits numbers.
Step 2: Draw the following 5 cards on the dry erase board. Ask the students to pull the same cards from their deck, and place them in the following formation.
Step 3: Explain that when the students start the activity, they will have to draw 1 card at a time. The students cannot have a card with a value of 1 in the tens or hundreds place of the minuend (top number.) They will have to redraw if an Ace is pulled. For the cards pulled to represent the tens and hundreds for the minuend, they must search through their deck to find a card with a value 1 less than the card they drew in an instance where they need to “borrow” when subtracting. For example, if they drew a 2, they will have to find an Ace in their deck to represent 1 and place it behind the 2. In the example below, the card drawn in the tens place was a 5 and the card drawn in the hundreds place is a 2. Therefore, students must find a 4 and place it behind the 5, and an Ace (value of 1) and place it behind the 2.
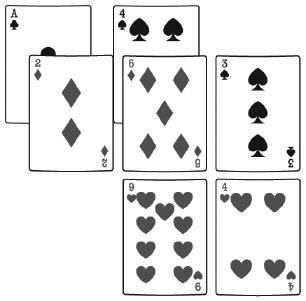
Step 4: Tell the students to subtract the numbers in the ones place, 3 − 4. Explain that 10 must be borrowed from the tens place in order to subtract. To indicate the borrowing, tell the students to place 1 tens base– ten block next to the 3 to represent 13 − 4, which equals to 9, and remove the 5 card from the tens place to show the 4 in the tens place.
Step 5: Ask the students to subtract the numbers in the tens place, 4 − 9. Explain that 10 must be borrowed from the hundreds place in order to subtract. To show the borrowing, tell the students to place 1 tens base-ten block next to the 4 to represent 14 − 9, which equals to 5, and remove the 2 card from the hundreds place to show the ace in the hundreds place.
Step 6: Ask the students to subtract the numbers. The should get an answer of 159.
Step 7: Have the students turn to Part B of their Adding and Subtracting worksheet. Tell them to replace the cards in their deck, and reshuffle the deck.
Step 8: Tell the students that for the 6 problems on Part B of the worksheet, they will draw 5 cards from their deck, write the numbers that are represented by the cards into the corresponding boxes, and subtract.
Step 9: Tell the students that whenever the problem calls for them to borrow to solve, they should indicate it by coloring in the tens base-ten block next to the number that needs to borrow the 10 like they indicated with the base-ten block in steps 4 and 5.
Extension Activity
Step 1: Have the groups take turns coming up to the board to write and solve addition and subtraction problems up to 1,000 that require carrying over and borrowing. Continue until every group has had a turn and is comfortable with the material.
Essential Question for Discussion or Journaling:
Why is understanding place value important when adding and subtracting?
DIRECTIONS: Create place value charts to solve.
Use a place value chart to find the difference of 618 and 543.
Use a place value chart to find the sum of 347 and 245.
Use a place value chart to find the difference of 716 and 698.
Use a place value chart to find the sum of 387 and 416.
Explain what it means to add and subtract.
DIRECTIONS: Use addition and subtraction to solve the questions below.
A company sent 315 emails on Monday, 245 emails on Tuesday, and 196 on Wednesday. How many emails were sent on these 3 days?
Write a word problem that requires you to find the sum, then the difference to solve. 1
It is 485 miles from Point A to Point B and 725 miles from Point A to Point C. What is the distance from Point B to Point C?
The school has 313 students in Grade 3. Of the students, 215 are going on a field trip to the museum. Of the students going on the field trip, only 65 of the students brought their own lunch. How many students will have to buy lunch at the museum?
DIRECTIONS: Solve the following questions.
What is the result if 298 is subtracted from the sum of 245 + 310?
What is the result if 128 is added to the difference of 714 − 454?
What is the result if the difference of 984 − 846 is doubled?
What is the result if the sum of 117 + 245 is doubled?
What is the result if the difference of 626 − 478 is added to 328?
List 2 key words that signal you should subtract and 2 key words that signal you should add in a word problem.
Ma.3.NSO.2.3 – Multiply a one-digit whole number by a multiple of 10, up to 90, or a multiple of 100, up to 900, with procedural reliability.
Objective: Students will learn how to multiply one‐digit whole numbers by multiples of 10 and 100.
Materials: (Per
TEACHER)
1 dry erase board
1 dry erase marker
1 dry eraser
Materials: (Per student)
1 dry erase board
1 dry erase marker
1 dry eraser
Lesson Discussion:
• Review how to multiply whole numbers by 10 or 100.
• Review the properties of multiplication, especially the associative property of multiplication.
• Teach how to multiply one‐digit whole numbers by multiples of 10 and 100. Help the students understand that an equation such as 9 × 70, is the same as 9 × (7 × 10) = (9 × 7) × 10, and the equation 9 × 700 is the same as 9 × (7 × 100) = (9 × 7) × 100.
• The students will learn how to multiply one‐digit whole numbers by multiples of 10 within the range of 10 to 90, and by multiples of 100 within the range of 100 to 900.
PART A:
Procedure:
Step 1: Ask the students if they can find the product of the multiplication problem below. Tell the students to copy the expression shown below on their dry erase board.
3 × 50 =
Step 2: Explain to the students that since 50 = 5 × 10, we change the value of 50 in the multiplication problem to 5 × 10.
3 × (5 × 10) =
Step 3: Tell the students that when using the associative property of multiplication, we learn that in an expression solely involving multiplication, the grouping or order of performing operations does not affect the final answer. Thus, for the multiplication sentence shown in step 2, we can either find the product of 5 × 10 first or find the product of 3 × 5 first.
3 × (5 × 10) = (3 × 5) × 10
Step 4: Tell the students to try finding the product of 3 × 5 first and then see what happens to the multiplication problem.
Step 5: Tell the students that since 3 × 5 = 15, the multiplication becomes an expression involving multiplication by 10.
(3 × 5) × 10 = 15 × 10
Step 6: Tell the students to find the product of 15 × 10 using their knowledge of multiplying whole numbers by 10.
15 × 10 = 150
Step 7: Explain to the students that they could solve the problem (3 × 50) by just mentally multiplying 3 and 5, and then adding a zero. Explain that adding the zero is the same thing as multiplying by 10.
Step 8: Tell the students to erase their boards and write the expression below.
3 × 500 =
Step 9: Explain to the students that since 500 = 5 × 100, we can change the value of 500 in the multiplication problem to 5 × 100.
3 × (5 × 100) =
Step 10: Tell the students that when using the associative property of multiplication, we learn that in an expression solely involving multiplication, the grouping or order of performing operations does not affect the final answer. Thus, for the multiplication sentence shown in step 9, we can either find the product of 5 × 100 first or find the product of 3 × 5 first.
3 × (5 × 100) = (3 × 5) × 100
Step 11: Tell the students to find the product of 15 × 100 using their knowledge of multiplying whole numbers by 100.
15 × 100 = 1,500
Step 12: Now explain to the students that they could solve the problem (15 × 100) by just mentally multiplying 15 and 1, then adding two zeroes. Explain that adding two zeroes is the same thing as multiplying by 100.
Step 13: Let the students practice by using steps 1 to 6 to find the product of the multiplication problems shown below.
6 × 40 =
8 × 300 =
PART B:
Materials: (Per group of 4 students)
1 set of “dominoes” from Domino template
Teacher Preparation:
Step 1: Cut out 1 Domino template for each group of 4 students.
Procedure:
Step 1: Divide students into groups of 4. Distribute 1 set of dominoes from the Domino template to each group. Tell the students to shuffle the dominoes and to each take 4 at random.
Step 2: Whoever has the domino with "Domino #1" written on it drops the first domino. Students take turns dropping a domino that that matches the left or right of the domino(es) already on the table. If a student does not have a domino that matches, the next student tries to make a match. The dominoes can be matched either vertically or horizontally.
Essential Question for Discussion or Journaling:
How can place value help when multiplying one‐digit whole numbers by multiples of 10 and multiples of 100?
(3 × 6) × 10 9 × 400
(8 × 7) × 10 6 × 800
(9 × 4) × 10 3 × 600
(6 × 8) × 10 8 × 700
(3 × 6) × 100 6 × 80
(8 × 7) × 100 9 × 40
(9 × 4) × 100 8 × 70
(6 × 8) × 100 3 × 60 (5 × 2) × 10
× 900 (4 × 5) × 10
× 300 (2 × 3) × 10
×
(7 × 9) × 10
× 200 (5 × 2) × 100 2 × 30 (4 × 5) × 100 7 × 90 (2 × 3) × 100 5 × 20
(7 × 9) × 100 4 × 50
Domino #1
LEVELED PRACTICE 1 – MA.3.NSO.2.3
DIRECTIONS: Use your knowledge of multiplication facts and mental math to find the products.
How do you multiply a one-digit whole number by a multiple of 10?
DIRECTIONS: Match the number sentences in the left column with the corresponding products in the right column
How do you multiply a one-digit whole number by a multiple of 100?
DIRECTIONS: Use multiplication to solve the following word problems.
It takes Barbie 20 minutes to make 1 friendship bracelet. How many minutes will it take her to make 6 friendship bracelets?
Amy can type 40 words every minute. How many words can Amy type in 9 minutes?
There are 8 rows of chairs in the auditorium. If there are 30 chairs in each row, how many total chairs are there in the auditorium?
There are 30 windows in each floor of the hotel. If the hotel has 7 floors, how many windows are there in total?
Explain the mental strategy that you can use when multiplying a one-digit whole number by a multiple of 10 or 100.
MA.3.FR.1.2 – Represent and interpret fractions, including fractions greater than one, in the form of m/n as the result of adding the unit fraction 1/n to itself m times.
OBJECTIVE: Students will learn how to build fractions, including those greater than one, using unit fractions.
MATERIALS: (Per Student)
2 sheets of paper (8.5 × 11 inches, white)
2 sheets of construction paper (8.5 × 11 inches, any color)
1 box of crayons (shared with group of 4 students)
1 pair of scissors (shared with group of 4 students)
LESSON DISCUSSION:
• Review the concept of fractions with the students. Recall that a fraction of the form 1/n represent a fraction that is one part of a whole divided into n equal parts.
• Recall that the number above the fraction bar, called the numerator, represents equal parts of a whole.
Procedure:
Step 1: Instruct the students to take 1 sheet of construction paper and fold it horizontally and then vertically so that each rectangular fold represents 1/4 of the paper. Tell the students to fold the paper horizontally again, thus dividing each 1/4 into 2.
Step 2: Tell the students to open the construction paper. Instruct them to use a pair of scissors to cut along the folds on either side of the construction paper.
Step 3: Explain to the students that since they divided one whole paper into 8 equal parts, 1 part represents 1/8 of the whole. Tell the students to use a crayon to label each cut piece of construction paper as 1/8.
Step 4: Instruct the students to repeat steps 1 to 3 on the other construction paper. Ultimately, each student should have 16 sections, each labeled 1/8. Tell the students to set these pieces aside.
Step 5: Instruct the students to take 1 sheet of paper and fold it horizontally and then vertically so that each rear fold represents 1/4 of the paper. Tell the students to fold the paper horizontally again, thus dividing each 1/4 into 2.
Step 6: Tell the students to open the paper and use a crayon to trace the folds of their paper.
Step 7: Instruct the students to repeat steps 5 and 6 on the other sheet of paper. Ultimately, each student should have two sheets of paper, each divided into 8 equal parts.
Step 8: Instruct the students to color 5 boxes on one of the sheets of paper with a crayon. They can pick any boxes to color.
Step 9: Instruct the students to take pieces of construction paper and cover the colored portions of the paper. They should only use 5 pieces.
Step 10: Explain to the students that since each piece of construction paper represents 1/8, and they used 5 pieces to cover the colored portion of the paper, which represents 5/8, then five pieces is equivalent to 5/8.
Step 11: Instruct the students to color 8 boxes on the other sheet of paper with a crayon.
Step 12: Instruct the students to take the pieces of construction paper and try to use as few pieces possible to cover the colored portion of the paper. They should only use 8 pieces.
Step 13: Explain to the students that since each piece of construction paper represents 1/8, and they used 8 pieces to cover the colored portion of the paper, which represents one whole, then eight 1/8 pieces is equivalent to 8/8, or one whole.
Extension Activity
Step 1: Instruct the students to place the two sheets of paper side by side and ask them to identify the shaded parts. They should say 13/8, or 1 whole and 5/8.
Step 2: Ask the students how many pieces of construction paper they think are needed to cover the colored portion of the papers. They should say 13 pieces.
Essential Question for Discussion or Journaling:
Use visual models to explain why two one-half sized parts can be added together to make one whole.
DIRECTIONS: Answer each question below.
1 How many thirds must be added together to make two wholes?
2 How many fourths must be added together to make four wholes?
3 How many halves must be added together to make 5/2?
4 How many fifths must be added together to make 7/5?
5 How many eighths must be added together to make 10/8?
Use visual models to explain how many fourths are needed to make three wholes.
DIRECTIONS: Write the following fractions as a sum of unit fractions.
Represent the fraction 8/3 as a sum of unit fractions. Show this with a model. 1 5 6 = 2 3 4 5 3 5 = 5 3 = 9 4 = 7 2 =
DIRECTIONS: Match each fraction in the left column with the equivalent fraction on the right column
How many fifths would you need to create one whole? Use a number line to show your thinking.
MA.3.FR.2.2 – Identify equivalent fractions and explain why they are equivalent.
OBJECTIVE: Students will learn how to explain fraction equivalence and compare fractions.
MATERIALS: (Per Student)
1 Square template
5 fraction transparencies
1 scratch sheet of paper
1 pencil
LESSON DISCUSSION:
• Define the terms fractions, numerator, and denominator with the students.
• Show how to compare fractions.
• Explain how to identify equivalent fractions by observing and looking at their relative sizes.
Part a:
Teacher preparation:
Step 1: Copy and cut out 1 square from the square template for each student.
Step 2: Use a ruler to draw 4 × 4 squares onto the transparency sheets. Fit 4 squares onto each transparency sheet.
Step 3: Divide the transparency squares in the following manner so that each student has a transparency square that shows 1/2 , 1/3 , 1/4 , 1/6 , 1/8:
Step 4: Pass out the materials to each student.
Procedure:
Step 1: Explain that the transparency divided into 2 equal sections is the “ 1/2 square fraction sheet.”
Step 2: Explain that the transparency divided into 3 equal sections is the “ 1/3 square fraction sheet.”
Step 3: Explain that the transparency divided into 4 equal sections is the “ 1/4 square fraction sheet.”
Step 4: Explain that the transparency divided into 6 equal sections is the “ 1/6 square fraction sheet.”
Step 5: Explain the transparency divided into 8 equal sections is the “ 1/8 square fraction sheet.”
Step 6: Instruct the students to take the paper square template and place the 1/2 square and the 1/4 square fraction sheets on top of the paper. Tell the students to make sure that the paper template square and the squares on the transparencies are aligned.
Step 7: Ask the students to observe the fractions. Explain that 1 section of the 1/2 square is the same or equivalent to 2 sections of the 1/4 square. This means that one 1/2 is equivalent to two 1/4 or 2/4.
Step 8: Let the students use different combinations of fraction sheets to identify equivalent fractions. Suggest that students use the 1/3 square and 1/6 square, or the 1/4 square and 1/8 square, when trying to find equivalent fractions. The students will write the equivalent fractions they can identify on their sheets of paper.
Part B:
Materials: (Per group of 4)
1 set of Fraction Circles
1 dry erase board
1 dry erase marker
1 dry eraser
1 one minute sand timer
Procedure:
Step 1: Tell the students to empty the bag of fraction circles in front of them.
Step 2: Explain to the students that they should make as many 1/2 circles as possible. Tell the students to write down the fractions that equal 1/2 on their board.
Step 3: For every 1/2 circle that the groups create, they get 1 point. The group with the most points at the end of a minute is the winner.
Part C:
Materials: (Teacher)
1 sheet of paper
1 pair of scissors
1 marker
Materials: (Per group of 4)
1 set of Fraction Circles (1/2 , 1/3 , 1/4 , 1/5 , 1/6 , 1/8 , 1/10 , and 1/12)
Procedure:
Step 1: Tell the students to empty the bag of fraction circles in front of them.
Step 2: Have the students separate the yellow circle (1 whole) and place it in the middle of the group.
Step 3: Have the students take turns selecting 1 fraction piece and placing it in front of them until there are no more fraction pieces in the pile. Some students will have more fraction tiles than others.
Step 4: Tell one of the students who have the brown (1/2) fraction piece to place it onto the yellow circle (1 whole.) The students in the groups continue clockwise from that student and attempt to make a 1/2 circle with the pieces that they chose.
Step 5: The student with the least amount of fraction pieces after making as many halves as possible is the winner.
Extension Activity
Step 1: Divide a sheet of paper into 2 halves using the scissors. On half of the paper, write a large number 0, and on the other half, write a large number 1.
Step 2: Call 7 students at random. Tell the 7 students to stand in a line, with each student standing equal distance from the other.
Step 3: Give the paper with the number 0 to the first student in the line and the paper with the number 1 to the last student in the line.
Step 4: Ask the 5 students not holding a number which fractions they would represent on a number line, what fraction would they represent. The answer should be 1/6 , 2/6 , 3/6 , 4/6 , and 5/6.
Step 5: Continue repeating steps 2 through 4 using 5 students, 9 students, and 11 students.
Essential Question for Discussion or Journaling:
Describe how to identify equivalent fractions.
DIRECTIONS: Use the models below to create equivalent fractions.
If you plot two equivalent fractions on a number line, would they be placed at different points? Explain your answer.
Name:
LEVELED PRACTICE 2
DIRECTIONS: Complete the models to represent each problem. Write the fractions and compare using >, <, or = symbols.
Manny and Jeff want to use models to show the fraction of their homework they have completed. Manny has completed 3 of 4 assignments and Jeff has completed 1 of 4 assignments. Who completed more of their homework?
Arnold and Emma are making graphs to compare how much of a book they have read. Arnold read half of the chapters. Emma read 4 of the 6 chapters. Who read more of the book?
Samson ordered 2 pizzas for his family, 1 cheese and 1 vegetable. By the end of dinner, 5 slices of the cheese pizza and 7 slices of the vegetable pizza had been eaten. Which pizza had more slices eaten from it?
Explain what equivalent fractions are. 1 2 3
DIRECTIONS: Compare the fractions using comparison symbols (>, <, or =.)
Justify your answers using fraction models
Describe a real-life situation in which it would be important to know what equivalent fractions are.
MA. 3.AR.1.1 – Apply the distributive property to multiply a one-digit number and two-digit number. Apply properties of multiplication to find a product of one-digit whole numbers.
OBJECTIVE: Students will learn how to identify the properties of multiplication and apply the properties as strategies in solving multiplication and division problems.
MATERIALS: (Per group of 4 students)
1 set of Properties of Multiplication Cards
LESSON DISCUSSION:
• Explain the identity property of multiplication. Help students understand that the multiplicative identity is 1. It means that any number multiplied by 1 is itself. Example: 8 × 1 = 8 or 20 × 1 = 20
• Explain the commutative property of multiplication. Help students understand that in multiplication, the order in which 2 numbers are multiplied does not matter. Example: 3 × 6 = 6 × 3 or 12 × 4 = 4 × 12
• Explain the associative property of multiplication. Help students understand that in expressions solely involving multiplication, the order of performing an operation does not matter. Example: (2 × 9) × 4 = 2 × (9 × 4) or (3 × 2) × 5 = 3 × (2 × 5)
• Explain the distributive property of multiplication. Help students understand that the distributive property holds on multiplication over addition. Tell the students that the sum of products is equal to the product of sums. Example: 12 × 3 = (6 × 3) + (6 × 3) or 17 × 5 = (10 × 5) + (7 × 5)
Part A:
Teacher Preparation:
Step 1: Print out side A and B back and front of the Properties of Multiplication Cards handouts so the equations correctly align with their answers and cut them out for each group of 4 students.
Step 2: Divide the students into groups of 4 and distribute 1 set of Properties of Multiplication Cards to each group.
Procedures:
Step 1: Have each group shuffle the playing cards. Have each student in the group select 6 cards. Tell students to make sure that none of their group members can see the "answer" portion of their game cards.
Step 2: One student will go first and it will continue clockwise. The first student will show the equation on 1 of their cards to the student their left. That student has an opportunity to win the card and place it in a "win" pile if they correctly determine what property the equation represents. If the student does not guess correctly, the next student will have a chance to win the card and so on until the card is identified correctly.
Step 3: Have the students continue step 2 until all the cards have been identified. The student with the largest "win" pile is the winner of the activity.
Part B:
MATERIALS: (Per group of 4 students)
1 premade spinner from template
1 deck of playing cards
1 thirty second timer
1 pre-drawn dry erase board (or Write and Wipe holder)
1 dry erase marker
1 dry eraser
1 pencil
1 tally table
Teacher Preparation:
Step 1: Remove the face cards (Jacks, Queens, and Kings) from each deck of cards and shuffle.
Step 2: Print out 1 Spinner template for each group and tape it to the back of the spinner.
Step 3: Create a template on dry erase boards to be used by each team. To create the template, turn the board in a vertical position. Use a permanent marker to draw the illustration shown below. Alternatively, print the illustration and insert it in a Write and Wipe holder.
Step 4: Distribute the materials to each group of 4 students.
Step 5: Each student in the group takes turns spinning the spinner. Depending on where the spinner lands, students draw the number of cards indicated.
Step 6: The students will create a multiplication equation using the cards and the property they selected. Tell the students that the Ace card is equivalent to 1. Students cannot have any of the same numbers. If this occurs, the repeated card must be returned to the deck and a new card must be drawn.
Step 7: The students will locate the appropriate equation template on the dry erase board and use the numbers they drew to fill in the template to correctly represent the property of multiplication they selected. For example, if the student lands on Identity property, they draw 1 card. They will write the value of the card in boxes 1 and 2. For example, if they drew the 6 card, it would look like the following:
If the student lands on Associative property, they draw 3 cards. They will write the value of the first card in boxes 1 and 4. They will write the value of the second card in boxes 2 and 5. They will write the value of the third card in boxes 3 and 6. For example, if they drew the 9, 8, and 4 cards, it would look like the following:
If the student lands on Commutative property, they draw 2 cards. They will write the value of the first card in box 1 and 4. They will write the value of the second card in boxes 2 and 3. For example, if they drew the 9 and 5 cards, it would look like the following:
If the student lands on Distributive property, they draw 3 cards. They will write the value of the first card in boxes 1, 3, and 5. They will write the value of the second card in box 2. They will write the value of the third card in box 4. Box 5 will have to have the sum of boxes 2 and 4 written in it. For example, if they drew the 9, 3, and 7 cards, it would look like the following:
Step 8: Students have 30 seconds to correctly draw the cards and fill out the board. If they successfully do so, they earn 1 point on the tally table. They write an I (for Identity), A (for Associative), C (for Commutative), or D (for Distributive) to indicate which property they got correctly. If they do not answer correctly within the allotted time, it is the next student’s turn.
Step 9: Have the students continue taking turns. The first student to correctly get 2 of each property on the tally table is the winner.
Essential Questions for Discussion or Journaling:
(1) Explain the commutative property of multiplication and give an example.
(2) Explain the associative property of multiplication and give an example.
(3) Explain the distributive property of multiplication and give an example.
Hands On Math
Draw 1 card
Draw 2 cards
Draw 3 cards
Draw 3 cards
LEVELED PRACTICE 1 – MA.3.AR.1.1
DIRECTIONS: Identify the property of multiplication shown in each of the following equations
1 4 × 3 = 3 × 4
3 7 × (8 × 9) = (7 × 8) × 9
2 × 11 = (2 × 1) + (2 × 10)
Use drawings to show the associative property of multiplication.
DIRECTIONS: Find the missing values for each equation and determine which property is represented
1 (8 × 5) = (8 × 3) + (8 × _____)
PROPERTY:
2 9 × 3 = _____ × 9
PROPERTY:
3 2 × (3 × 4) = (_____ × 3) × 4
PROPERTY:
4 (6 × 7) = (_____ × 4) + (_____ × 3)
PROPERTY:
Use drawings to show the commutative property of multiplication.
Name:
LEVELED PRACTICE 3
DIRECTIONS: Solve the following word problems.
1 Mandy plans to purchase 5 packs of baseball cards, with each pack containing 4 cards. Her friend Arnold suggests that if she buys 4 packs, with each pack containing 5 baseball cards, she will end up with the same total number of cards. Is Arnold's statement correct? Explain your answer.
2 Jimmy needs to find the product of 8 × 14. William suggested that if Jimmy were to add the products of 4 × 10 and 8 × 4, he would get the same answer. Is William correct? Explain your answer.
3 Josephine is buying doughnuts for a catering event. She wants to place an order for 5 Package A Options. This package includes 10 boxes of doughnuts, each with 6 inside. To find how many doughnuts she ordered in all, she multiplied the number of packages by the number of boxes. Then she multiplied the product of that by the number of doughnuts in each box. What is another way she could find the total using multiplication?
Use drawings to show the distributive property of multiplication.
MA.3.AR.2.3 – Determine the unknown whole number in a multiplication or division equation, relating three whole numbers, with the unknown in any position.
MA.3.AR.2.1 – Restate a division problem as a missing factor problem using the relationship between multiplication and division.
OBJECTIVE: Students will learn how to find the missing factor in a multiplication sentence using division.
MATERIALS: (Per group of 2)
90 bingo chips
1 Math Mission: Inverse Operations game board
1 Chip Collection cup
2 resealable bags
2 pawns
1 die
LESSON DISCUSSION:
• Review strategies of dividing whole numbers.
• Explain that multiplication and division are inverse operations. Help the students understand that 1 operation can undo the other (i.e., multiplication can undo division, and division can “undo” multiplication).
Teacher Preparation:
Step 1: Print out 1 Math Mission: Inverse Operations game board on an oversized sheet of paper for each group.
Step 2: Count out 30 chips for the Chip Collection cup and 30 chips for each student. Distribute the materials to each group of 2 students.
Procedure:
Step 1: Explain to the students that the objective of the game is to be the first player to land on the finish line with no chips remaining in their bag.
Step 2: Have the students place the Chip Collection Cup on the game board with 30 chips inside of it.
Step 3: Instruct each group to place the pawn they selected on the "Start" section of their game board.
Step 4: Tell each student in the group to roll the die once. The student who rolls the highest number goes first.
Step 5: The first player rolls and moves the amount of spaces indicated.
Step 6: Students follow the instructions written on the tile they land on.
Step 7: When a student lands on an equation tile, they must find the missing value that makes the equation correct. Once the correct answer is verified, the student may deduct the number of chips from their chip bag as indicated on the tile and place them into the Chip Collection cup.
Step 8: The students will continue alternating turns until there is a winner.
Step 9: The winner of the game is the first person to reach the finish line with no chips. If students reach the finish line with chips remaining in their bag, they must return to the start. If after each player has reached the finish line, but still have chips in their cup, the player with the least number of chips is the winner.
Extension Activity
Step 1: Let each student develop a missing factor problem. Remind the students that they should know the correct answer to their problem.
Step 2: Call 1 student at random. Let the student share their missing factor problem with the other students, and let the other students answer the problem.
Essential Questions for Discussion or Journaling:
(1) What are the properties of multiplication?
(2) Describe division in terms of multiplication.
125 | Hands On Math | Educational Bootcamp
DIRECTIONS: Match each division equation to the question that can help solve the problem
What number multiplied by 6 equals 36?
÷ _____ = 6 What number multiplied by 8 equals 64?
÷ _____ = 3 What number multiplied by 5 equals 25?
÷ _____ = 5 What number multiplied by 2 equals 18?
_____ = 9
÷ 8 = ? 18 ÷ _____ = 2
number multiplied by 3 equals 21?
number multiplied by 9 equals 72?
Which equation below gives you the greater answer? Explain why.
× 9 = ? or
DIRECTIONS: Use your knowledge of order of operations to find the correct answers
Rewrite the following word problem to be a division equation.
"Samantha buys 5 cans of dog food for each of her 3 dogs. How many cans of dog food does she buy?"
DIRECTIONS: Solve the following word problems.
1 Jesse wants to evenly arrange 21 flower pots into 3 rows in his garden. Write the division equation that could be used to find how many flower pots will be in each row.
2 Julissa volunteers 24 hours a week at the animal shelter, spreading her time across 6 days. She volunteers for the same amount of time each day. Write the division equation that could be used to find the amount of hours she volunteers for each day.
3 Timmy was asked to put 32 eggs into 8 trays. Each tray will have the same number of eggs. Write the division equation that could be used to solve for the number of eggs in each tray.
Write a word problem utilizing the expression 50 ÷ ? = 10 to solve. Give the answer to the word problem you wrote.
MA.3.M.1.1 – Select and use appropriate tools to measure the length of an object, the volume of liquid within a beaker and temperature.
OBJECTIVE: Students will learn how to use the correct tools when measuring length of an object, volume of liquid, and temperature.
MATERIALS: (Per Teacher)
1 50 ml clear beaker
1 ruler
1 thermometer
3 desks
1 Items to Measure template
1 brown paper bag
LESSON DISCUSSION:
• Define length, volume, and temperature.
• Show the different measuring tools, including rulers, yardsticks, meter sticks, weighing scales, balance scales, beakers, graduated cylinders, droppers, and thermometers to the students.
• Explain that rulers, yardsticks, and meter sticks are used to measure the lengths of an object. Weighing scales and balance scales are used to measure the mass of an object. Beakers, graduated cylinders, and droppers are tools to measure the volume of liquids. Thermometers are used to take temperature.
Part A:
Teacher Preparation:
Step 1: Print one sheet of Items to Measure template.
Step 2: Cut each card from the template and place them inside a brown paper bag.
Step 3: Arrange three desks in front of the class. Place a clear beaker on one of the desks, a ruler on another desk, and a thermometer on the third desk.
Procedures:
Step 1: Explain to the students that the objective of the game is to identify the correct measuring tool that should be used to take the measurement of an object.
Step 2: Pick one card from the brown paper bag and read it aloud.
Step 3: Have the students line up behind the desk that has the correct tool to measure the item written on the card. For example, if the card reads “length of a crayon,” the students will line up behind the desk with the ruler.
Step 4: From each desk where there is a student behind it, pick one student to explain their reasoning.
Step 5: Keep selecting cards from the paper bag until all students understand how to pick the correct measuring tools.
Part B:
Materials: (Per TEACHER)
1 ball of yarn
1 pair of scissors
MATERIALS: (Per group of 4 STUDENTS)
1 ruler
1 Measuring Length portion of the Measuring template
1 dry erase marker
1 dry eraser
1 Write and Wipe holder
Teacher Preparation:
Step 1: Cut the pool of yarn into pieces of different lengths. Cut five pieces of yarn for every group of 4 students.
Step 2: Print one sheet of Measuring template for each group and place it in a Write and Wipe holder. Explain to the students that they will only be using the Measuring Length portion of the Measuring template.
Procedures:
Step 1: Provide the materials to each group of 4 students. Give each group of students a set of 5 pre-cut pieces of yarns.
Step 2: Instruct the students to measure the length of each piece of yarn. The students will record their findings in the Measuring Length chart.
Step 3: Once all the groups have finished measuring the lengths of their pieces of yarn assigned to them, let each group discuss their findings with the rest of the class. Tell each group to explain the process they used to measure the lengths of the yarns.
Part C:
MATERIALS: (Per group of 4 STUDENTS)
1 50 mL clear beaker
1 ruler
4 marbles of different masses in a resealable bag
1 container of 200 mL of water
1 Measuring Volume portion of the Measuring template
1 dry erase marker
1 dry eraser
1 Write and Wipe holder
Teacher Preparation:
Step 1: Explain to the students that they will only be using the Measuring Volume portion of the Measuring template.
Step 2: Distribute the materials to each group of 4 students.
Procedure:
Step 1: Instruct each group to take the 50 mL beaker and fill it with 25 mL of water from the 200 mL container of water.
Step 2: Tell the students to measure the volume of the water in the beaker and record their findings in the table in the “Volume Before Displacement” column.
Step 3: Instruct the students to take one of the marbles and drop it into the beaker. Have the students write “Marble 1" on the first line of the "Object" column to represent this marble.
Step 4: Tell the students to observe how the water level inside the beaker rises. Explain to the students that since water cannot be compressed, the marble displaces the amount of water equal to the space it occupies at the bottom of the beaker. The space occupied by the marble is equal to its volume and is also equal to the amount of water displaced.
Step 5: Instruct the students to measure the new height of the water level in the beaker and record their findings in the table next to "Marble 1" under the “Volume After Displacement” column.
Step 6: Tell the students to find the difference between the “Volume Before Displacement” and the “Volume After Displacement.” Explain that the difference indicates the amount of water displaced by the marble and will also indicate the volume of the marble.
Step 7: Tell the students to remove the marble, dry it off, and place it back in the resealable bag.
Step 8: Have the groups repeat steps 3 to 7 with the other marbles and to continue completing the table.
Step 9: Have the groups place all of the marbles back into the bag once they have been dried.
Extension Activity
Step 1: Ask the students if they noticed any patterns regarding the weight of the object and the height of the water level in their data. They should have noticed that the heavier the object was, the higher the water level rose.
Step 2: Ask the students what the relationship between the water displacement and the volume is in this activity.
Step 3: Ask students to give examples of objects they believe may cause the water in their cups to overflow and why.
Part D:
MATERIALS: (Per group of 4 STUDENTS)
1 thermometer
1 Measuring Temperature portion of the Measuring template
1 small beaker to hold water
1 dry erase marker
1 dry eraser
1 Write and Wipe holder
Teacher Preparation:
Step 1: Explain to the students that they will only be using the Measuring Temperature portion of the Measuring template.
Step 2: Distribute the materials to each group of 4 students.
Procedures:
Step 1: Instruct each group of students to test the temperature of the water from the bathroom sink, the water fountain, and the temperature on the playground, and record their findings in “Measuring Temperature” table.
Step 3: Once all the groups have finished taking temperatures, let each group discuss their findings with the rest of the class. Tell each group to explain the process they used to measure the temperature.
Essential Questions for Discussion or Journaling:
(1) List the appropriate units of measurement to use when measuring lengths of objects.
(2) List the appropriate units of measurement to use when measuring volumes of liquid.
(3) List the appropriate units of measurement to use when measuring temperature.
Name:
DIRECTIONS: Measure the length of the pencils shown below.
Explain how to decide which unit of measurement to use when measuring length. When do you use inches, feet, yards, or miles?
DIRECTIONS: Identify the volumes of liquid shown in the following figures.
Explain how to decide which unit of measurement to use when measuring volume. When do you use liters, grams, gallons, or kilograms?
DIRECTIONS: Identify the temperatures shown below.
: _________________________
: _________________________
: _________________________
Will the temperature of a hot coffee be higher or lower than the temperature of an iced coffee? Explain your answer.
MA.3.M.1.2 – Solve real-world problems involving any of the four operations with whole-number lengths, masses, weights, temperatures or liquid volumes.
OBJECTIVE: Students will learn how to solve word problems involving any of the four operations with lengths, volumes, and masses.
MATERIALS:
10 craft sticks
1 roll of tape
(Per GROUP OF 4 STUDENTS)
1 roll of measuring tape
1 sheet of Measuring Lengths portion of the Measuring template
1 Write and Wipe holder
1 dry erase marker
1 dry eraser
LESSON DISCUSSION:
• Define length, volume, and mass to the students.
• Explain that length defines how long or how short objects are.
• Explain that volume represents the amount of space a solid or threedimensional object occupies.
• Explain that mass tells how heavy or light objects are.
Part A:
Teacher Preparation:
Step 1: Print one sheet of Measuring template for each group and place it in the Write and Wipe holder.
Step 2: Distribute the materials to each group of 4 students. Explain to students that they will only be using the Measuring Lengths portion of the Measuring template.
Procedures:
Step 1: Tell the students to use the tape to stick two craft sticks together, end-to -end. Instruct the students to set this aside.
Step 2: Tell the students to use the tape to stick three craft sticks together, endto-end. Instruct the students to set this aside.
Step 3: Tell the students to use the tape to stick four craft sticks together, endto-end. Instruct the students to set this aside.
Step 4: Instruct the students to use the measuring tape to measure the length of one craft stick and record their findings on the Measuring Length table.
Step 5: Next, tell the students to use the length of one craft stick to predict the length of the two craft sticks that were connected end-to-end, the three craft sticks that were connected end-to-end, and the four craft sticks that were connected end-to-end and record their predictions in the Measuring Length table.
Step 6: Next, instruct the students to use the measuring tape to measure the length of the two craft sticks that were connected end-to-end, the three craft sticks that were connected end-to-end, and the four craft sticks that were connected end-to-end. The students will record their finding in the Measuring Length table.
Step 7: Ask the students to compare their predictions with the actual lengths of the craft sticks. Ask the students if their predictions were the same as the actual lengths. For those groups who made the correct predictions, ask them how they came up with the correct predictions.
Extension Activity
Step 1: Ask the students if they noticed any patterns regarding the length of one craft stick in comparison to the length of the connected craft sticks. They should notice that the length of two craft sticks connected end-to-end is approximately twice the length one craft stick. Explain that the placement of the tape could affect the length of the connected craft sticks.
Part B:
Materials: (Per Group of 4
1 graduated cylinder (50 mL)
1 container of water with 200 mLs
STUDENTS)
1 Measuring Volumes portion of the Measuring template
1 Write and Wipe holder
1 dry erase marker
1 dry eraser
Teacher preparation:
Step 1: Assign each student in the group a letter from A to D.
Step 2: Distribute the materials to each group. Explain to the students that they will be using the Measuring Volumes portion of the Measuring template.
Procedures:
Step 1: Have Student A in each group start the activity by placing 10 milliliters from the container of water into the graduated cylinder.
Step 2: All group members should check to make sure the correct amount of water has been placed inside. More or less water can be added as necessary until every member of the group agrees that there are 10 milliliters in the cylinder.
Step 3: Tell the groups to fill out Part B data table for Student A. The column “Before Turn” should say 0 for the Student A, but should increase by ten for each following student. The students should agree that on the “After Turn” on the Student A row, the number 10 should be written.
Step 4: Have the groups repeat steps 1 to 3 until all the members of the group have added 10 milliliters to the graduated cylinder without removing any of the water previously inside it and correctly filled out the data table appropriately.
Step 5: Explain that adding water changes the volume of the amount of liquid held in a container. By each student adding 10 mL on their turn, the volume increases by 10 mL each time.
Extension Activity
Step 1: Ask the students what the relationship between the water displacement and the volume is in this activity.
Part C:
Materials:
(Per Group of 4 STUDENTS)
1 mini resealable bag with dry pasta
1 mini resealable bag with dry black beans
1 mini resealable bag with dry red beans
1 mini resealable bag with gravel
1 beam balance
1 20 gram weight
3 10 gram weights
4 5 gram weights
7 1 gram weights
1 Measuring Masses portion of the Measuring template
1 Measuring Mass worksheet
1 Write and Wipe holder
1 dry erase marker
1 dry eraser
Teacher Preparation:
Step 1: For each group of 4 students, fill 1 mini resealable bag with dry pasta, 1 with dry black beans, 1 with dry red beans, 1 with gravel. No bag should exceed a weight of 77 grams.
Step 2: Make sure that the resealable bags of pasta, red beans, black beans, and gravel have a discernible difference in weight.
Step 3: Print out a Measuring Mass worksheet for each group and place it in the back of the Write and Wipe holder behind the Measuring template for each group.
Step 4: Distribute the materials to each group. Explain to students that they will be using the Measuring Masses portion of the Measuring template.
Procedures:
Step 1: Have each student in the group select 1 of the 4 mini resealable bags. If there are fewer than 4 students per group, 1 student may need to select more than 1 bag to complete the activity.
Step 2: Have the groups work as a team to use the beam balance and gram weights to answer the questions on the Measuring Masses table and the Measuring Mass worksheet.
Step 3: Explain to the students that even though the bags are the same size, the weight of the objects in the bag and the amount of the objects in the bag will affect the mass.
Essential Questions for Discussion or Journaling:
(1) Describe how the amounts of liquids and solids are measured.
(2) List the appropriate units of measurement used when measuring solids.
(3) List the appropriate units of measurement used when measuring liquids.
One craft stick
Two craft sticks
Three craft sticks
Four craft sticks Student Number
DIRECTIONS: Use the beam balance to determine whether each item in the table below has more or less mass. Circle the correct answer.
Gravel has more / less mass than pasta.
Red Beans have more / less mass than black beans.
Pasta has more / less mass than black beans.
Gravel has more / less mass than red beans.
Black beans have more / less mass than gravel.
Red Beans have more / less mass than pasta.
1. What is the approximate mass of the gravel?
2. What is the approximate mass of the red beans? ____________________
3. What is the approximate mass of the black beans?
4. What is the approximate mass of the pasta?
5. Which bag has the greatest mass?
6. Which bag has the least amount of mass?
7. Do the 2 bags of beans combined have a greater, lesser, or equal mass as the 2 bags of gravel and pasta combined?
DIRECTIONS: Answer the following questions.
1 2 3
If the 2 beakers hold the same amount of liquid, how many liters of liquid do you estimate is in Beaker B?
Which of the following is the best estimate for the weight of an elephant? Explain your answer. ______ 4,000 kilograms ______ 400 grams
The length of a paperclip is 2 inches. What do you think is the length of five such paperclips connected end-to-end? Explain your answer.
Identify 5 things you can measure using kilograms.
DIRECTIONS: Answer the following questions.
1 2 3
The beaker on the right shows how much of a chemical Milo needs for an experiment. If he wants to repeat the experiment 5 times, how much of the liquid does he need? If he wants to use only half of the chemical for each of the 5 experiments, how much of the chemical will he need?
Full amount for 5 experiments: __________
1/2 of the amount for 5 experiments: __________
Which of the following is the best estimate for the mass of a bag of peanuts? Explain your answer. ______ 4,000 kilograms ______ 400 grams
The length of a paperclip is 4 centimeters. Lorie wants to make a chain by connecting such paperclips end-to-end. If Lorie wants the chain to be 40 centimeters long, how many paperclips does she need? Explain your answer.
Identify 5 things you can measure using liters.
Name:
LEVELED
DIRECTIONS: Solve the following word problems.
1 2 3
Marta sells aquariums. Today, she sold 3 aquariums that hold 10 liters of water, 6 aquariums that hold 9 liters of water, and 1 aquarium that holds 100 liters of water. If you combined the capacity of all the aquariums sold, how many liters would they hold in all?
Jack bought 6 kilograms of candy for Halloween. He wanted the bag to last the whole night. On average, how many kilograms of candy would he have to distribute each hour to make sure the candy lasted 6 hours?
A carpenter has a piece of wood 12 yards long. He wants to cut the piece of wood into 4 pieces of equal length. What will be the length of each piece?
Identify 5 things you can measure using meters.
MA.3.GR.1.2 – Identify and draw quadrilaterals based on their defining attributes. Quadrilaterals include parallelograms, rhombi, rectangles, squares and trapezoids.
OBJECTIVE: Students will learn how to identify and draw quadrilaterals based on the properties given.
MATERIALS: (Per Group of 2)
9 index cards to be made into Property Cards
2 sets of geometric shapes (square, hexagon, triangle, rhombus, trapezoid, parallelogram)
2 sorting circles
2 dry erase boards
2 dry erase markers
2 dry erasers
LESSON DISCUSSION:
• Discuss what a quadrilateral is.
• Identify the different kinds of quadrilaterals, namely a square, rectangle, rhombus, parallelogram, and trapezoid.
• Explain the properties of quadrilaterals.
Teacher Preparation:
Step 1: Make 9 property cards for each group of 2 students. The cards should be labeled as follows: two sets of parallel lines, one set of parallel lines, right angles, four sides, acute angles, quadrilateral, obtuse angles, three sides, and six sides. Each group should also be given 2 sorting circles, 2 dry erase boards, 2 dry erase markers, and 2 dry erasers. Each group should also have 2 of the following geometric shapes: square, hexagon, triangle, rhombus, trapezoid, and parallelogram.
Procedure:
Step 1: Have each pair of students place their sorting circles on their desk so they form a Venn diagram.
Step 2: Explain to students that they will compare 2 shapes using the 9 Property Cards to determine the differences and similarities. For example, if the students are told to find the similarities and differences of a hexagon and triangle, their Venn diagram should look like the following figure:
obtuse angles six sides three sides acute angles
Step 3: The students will compare the shapes in the following order:
Extension Activity
Step 1: Ask the students to draw a castle on dry erase boards using only squares, rectangles, parallelograms, rhombuses, and trapezoids.
Step 2: Have the students switch boards with their team member. Have the students write how many of each type of quadrilateral they found on the bottom of their drawing.
Essential Question for Discussion or Journaling:
Describe some physical properties of shapes that help to identify them.
1. Hexagon & Triangle 6. Hexagon & Rhombus 11. Hexagon & Square
2. Triangle & Square 7. Parallelogram & Rhombus 12. Trapezoid & Triangle
3. Trapezoid & Square 8. Parallelogram & Square 13. Hexagon & Parallelogram
4. Hexagon & Trapezoid 9. Square & Rhombus 14. Triangle & Rhombus
5. Parallelogram & Triangle 10. Trapezoid & Rhombus 15. Trapezoid & Parallelogram
Name:
DIRECTIONS: Compare the shapes in each set.
1
Number of sides
2 Select all the attributes that the 2 shapes below share.
Classification type of shape
Number of angles
Number of right angles
Select all the attributes that the 2 shapes below share.
Number of sides
Classification type of shape
Number of angles
Number of right angles
3 Select all the attributes that the 2 shapes below share.
Number of sides
Classification type of shape
Number of angles
Number of right angles
Explain what a polygon is. What are the most important properties of a polygon?
Name:
DIRECTIONS: Solve the following word problems.
Michelle drew a shape that has 4 equal sides and 2 acute angles. What shape did Michelle draw?
Jake found a piece of metal that has 4 sides, 2 obtuse angles, and 1 pair of parallel sides. What is the shape of the piece of metal?
A carpenter carved a shape in the wood that has 4 right angles and 4 equal sides. What shape did the carpenter carve?
What are the different types of quadrilaterals? Explain how they are different from each other. How are they the same?
Name:
DIRECTIONS: Identify if the following statements are TRUE or FALSE. Explain your answers
1
All squares are rectangles, but not all rectangles are squares.
All parallelograms are rectangles, but not all rectangles are parallelograms.
All rhombuses are parallelograms, but not all parallelograms are Rhombuses.
Study the shape below. Is the shape a quadrilateral or not? Explain your answer.
MA.3.GR.1.3 – Draw line(s) of symmetry in a two-dimensional figure and identify line-symmetric two-dimensional figures.
OBJECTIVE: Students will learn how to identify and draw lines of symmetry of two-dimensional figures.
MATERIALS: (Per group of 2)
1 math geoboard
10 rubber bands
LESSON DISCUSSION:
• Explain what lines of symmetry are. Students should understand that a line of symmetry divides a figure into two mirror images.
• It is important to stress to the students that not because a line divides a figure into two shapes of equal size and same shape means the line automatically becomes the line of symmetry. Cite for example the diagonal of a rectangle. The diagonal divides the rectangle into two shapes of same size and shape but the two shapes are not mirror image of each other.
Part A:
Procedure:
Step 1: Using a rubber band, instruct the students to create a square on their math geoboards.
Step 2: Instruct the students to use an additional rubber band to partition the square vertically into 2 equal areas.
Step 3: Tell the students to hold up their math geoboards when they are done partitioning the square and verify they have done so correctly.
Step 4: Ask the students what they notice about the 2 areas. The students should say the two areas have the same shape and size. The students should also notice that the two areas are mirror images of each other; that is, they can imagine folding the square along the vertical line and the 2 areas will fit over each other perfectly. Tell the students that this vertical line is 1 line of symmetry of a square.
Step 5: Instruct the students to clear their math geoboards and prepare a new square.
Step 6: Instruct the students to use rubber bands to partition the square into horizontally into 2 equal areas.
Step 7: Tell the students to hold up their math geoboards when done partitioning the square and verify they have done so correctly. Ask the students what they notice about the 2 areas. The students should notice they have the same shape and size.
Step 8: Ask the students whether this is another line of symmetry of a square. Let the students justify their answers.
Extension Activity
Step 1: Instruct the students to find the other lines of symmetry of a square.
Part B:
MATERIALS: (Per group of 2)
1 math geoboard
10 rubber bands
Procedure:
Step 1: Instruct the students to create a trapezoid on their math geoboards using 1 rubber band.
Step 2: Have the students partition the trapezoid vertically into two equal areas using 1 more rubber band. The students should get a shape similar to the figure below.
Step 3: Ask the students what they notice about the two areas. Then ask whether this is a line of symmetry of a trapezoid.
Step 4: Tell the students to remove the rubber bands from their Geoboards.
Step 5: Instruct the students to create a rectangle on their math geoboards using 1 rubber band.
Step 5: Have the students find the lines of symmetry of the rectangle. The students should get a shape similar to the figure below.
Step 6: Tell the students to remove the rubber bands once they have identified the correct lines of symmetry.
Step 7: Instruct the students to create an equilateral triangle on their math geoboards using 1 rubber band.
Step 8: Have the students find all the lines of symmetry of the equilateral triangle. The students should identify a shape similar to the figure below.
Essential Question for Discussion or Journaling:
Give three real-life examples of things, animals, or shapes that have at least one line of symmetry.
DIRECTIONS: Draw the lines of symmetry on each of the following regular polygons
Why is a diagonal of a rectangle not a line of symmetry?
DIRECTIONS: Identify the lines of symmetry of these figures.
Line A
Line B
Line C
Line D
Line A
Line B
Line C
Line D Line A
Line B
Line C
Line D
How many lines of symmetry does a parallelogram have? Explain your answer.
Name:
LEVELED PRACTICE 3 – MA.3.GR.1.3
DIRECTIONS: Determine the number of lines of symmetry of the following letters
2
3 NUMBER OF LINES OF SYMMETRY: ___________________
NUMBER OF LINES OF SYMMETRY: ___________________
NUMBER OF LINES OF SYMMETRY: ___________________
Study the figure on the right. Does the figure have lines of symmetry? If the figure has lines of symmetry, how many lines of symmetry does the figure have? 1
MA. 3.GR.2.4 – Solve mathematical and real-world problems involving the perimeter and area of composite figures composed of non-overlapping rectangles with whole-number side lengths.
OBJECTIVE: Students will learn how to use the properties of addition and multiplication to find the area of irregular figures.
MATERIALS: (Per group of 2)
1 dry erase grid board
1 dry erase marker
1 dry eraser
1 metric ruler
1 Figure 1 irregular shape on cardstock
1 Figure 2 irregular shape on cardstock
2 five by two inch rectangles on unique color cardstock
1 ten by two inch rectangle on unique color cardstock
1 six by five inch rectangle on unique color cardstock
1 nine by three inch rectangle on unique color cardstock
3 three by three inch rectangles on unique color cardstock
1 four by three inch rectangle on unique color cardstock
1 Irregular Figure Area worksheet
1 Write and Wipe holder
LESSON DISCUSSION:
• Explain that area represents the number of unit squares needed to cover a flat surface to the students.
• Teach the students how to find the area of a rectangle using the formula Area = length × width. Explain to the students that the area of a rectangle is equal to the product of the length and the width.
• The students will only practice calculating the areas of squares and
Teacher Preparation:
Step 1: Use cardstock and a metric ruler to measure, draw, and cut out the figure with the dimensions below in inches for each group of 2 students. This is Figure 1:
Step 2: Use cardstock and a metric ruler to measure, draw, and cut out the figure with the dimensions below in inches for each group of 2 students. This is Figure 2:
Step 3: Use a different colors of cardstock to provide each group of 2 students with the following rectangular shapes:
(1) 2 - 5" × 2" (Color 1)
(2) 1 - 10" × 2" (Color 2)
(3) 1 - 6" × 5" (Color 3)
(4) 1 - 9" × 3" (Color 4)
(5) 3 - 3" × 3" (Color 5)
(6) 1 - 4" × 3" (Color 6)
Procedure:
Step 1: Tell the students to use 2 of the rectangular cardstock cut-outs to partition Figure 1 into smaller rectangular shapes.
Step 2: Instruct the students to use the area formula for rectangles to find the area of the 2 rectangles individually using their dry erase grid boards.
Step 3: Explain to the students that since the 2 rectangles do not overlap, the area of the irregular figure is the same as the sum of the areas of the 2 rectangles. Instruct the students to calculate the area of each rectangle to find the area of the irregular figure: 6 × 5 = 30 and 10 × 2 = 20. Now, add the areas of each: 30 + 20 = 50. The area of the irregular shape will be 50 square units.
Step 4: Instruct the students to clear their dry erase grid boards and the rectangular pieces from the irregular shape.
Step 5: Have the students partition Figure 1 again, but this time using 3 rectangular pieces, as shown below.
Step 6: Instruct the students to calculate the area of each rectangle to find the area of the irregular figure.
Step 7: Have the students explain why the areas were the same even though they were partitioned into more pieces.
Step 8: Repeat steps 1 though 7 for Figure 2.
Step 9: Have the students partition Figure 2 into 4 rectangular pieces instead of 3, and repeat the steps again.
Extension Activity
Step 1: Print out 1 Irregular Figure Area worksheet for each group and place it in a Write and Wipe holder.
Step 2: Tell the students to clear their dry erase grid boards.
Step 3: Instruct the students to follow the directions on the worksheet to complete the activity.
Essential Questions for Discussion or Journaling:
Explain why multiplying length times (x) width gives the same area as counting the unit squares.
Complete the following steps: 1) Partition Figure 1 Part A and Part B into a set of 3 different rectangles each. 2) Write the dimensions of each rectangle you created. 3) Find the area of each rectangle. 4) Find the area of the irregular figure.
Complete the following steps: 1) Partition Figure 2 Part A and Part B into 3 different rectangles each. 2) Write the dimensions of each rectangle you created. 3) Find the area of each rectangle. 4) Find the area of the irregular figure.
Bootcamp
× 2
5 × 2
10 × 2
4 × 3
3 × 3
3 × 3
DIRECTIONS: Find the perimeter of each figure below.
PERIMETER:___________ = 1 square unit
PERIMETER:___________ = 1 square unit
PERIMETER:___________ = 1 square unit
Why is it important to know how many square units each square represents when finding the area?
DIRECTIONS: Complete the following tasks.
1 2 3
Select all the figures that have an area of 15 square centimeters.
Select all the figures that have an area of 12 square inches.
Select all the figures that have an area of 10 square feet.
Find 3 ways to divide a rectangle to show 24 square units.
DIRECTIONS: Find the area and perimeter of each figure below.
PERIMETER: __________
AREA: _______________
PERIMETER: __________
AREA: _______________
PERIMETER: __________
AREA: _______________
Explain a way to find the area and perimeter of an irregular figure.
ma.3.DP.1.2 – Interpret data with whole-number values represented with tables, scaled pictographs, circle graphs, scaled bar graphs or line plots by solving one– and two-step problems.
Ma.3.DP.1.1 – Collect and represent numerical and categorical data with whole-number values using tables, scaled pictographs, scaled bar graphs or line plots. Use appropriate titles, labels and units.
Part A:
OBJECTIVE: Students will learn how to read information from graphs.
MATERIALS: (Per TEACHER)
1 Bar Graph template on oversized paper
1 Pictograph template on oversized paper
1 Circle Graph template on oversized paper
1 Line Plot template on oversized paper
1 sheet of Data Question handout
1 pair of scissors
1 roll of tape
MATERIALS: (Per GROUP of 4 students)
1 whistle
LESSON DISCUSSION:
• Explain to the students the concept of different types of graphs, including bar graphs, circle graphs, pictographs, and line plots.
• Explain to the students how to read information from each type of graph.
Teacher Preparation:
Step 1: Print one copy of the Data Question handout. Use a pair of scissors to cut each card. Separate the cards into the categories of graphs (Bar Graph, Pictograph, Circle Graph, and Line Plot.)
Step 2: Print one copy of the Bar Graph template, Pictograph template, Circle Graph template, and Line Plot template on oversized paper. Place these graphs onto the classroom dry erase board. Alternatively, you can draw these graphs on the classroom dry erase board so that they are big enough for a large classroom to see.
Procedure:
Step 1: Divide the students into groups of 4 students.
Step 2: Provide each group with one whistle. Tell each group to assign one student who will be the one to blow the whistle.
Step 3: Tell the students that at the start of the activity, the whistle blower and 1 other student from each group will stand in front of the board ready to answer questions that relate to one of the graphs on the board. When either the whistle blower or the other teammate know the answer to the question, the whistle blower will blow the whistle and the first to do so will have the opportunity to answer the question correctly and win 1 point for their team. Keep score on the classroom board.
Step 4: Pick 1 question at random to begin the activity. Have 2 students from each group come up to the board. Call on the first group that blows the whistle. Award a point if the answer is correct. If the groups’ answer is incorrect, another group will have a chance to steal.
Step 5: The group who correctly answered the last question will get to choose the next category (bar graph, circle graph, pictograph, or line plot.) From the chosen category, a new question will be picked from the board.
Step 6: The whistle blower from each group will stay at the board, but a new member from each team will join them to be ready for the next answer.
Step 7: Continue asking questions from the cards until all have been answered. The group with the most points is the winner.
Essential Questions for Discussion or Journaling:
(1) How do you read information from a pictograph?
(2) How do you read information from a bar graph?
(3) How do you read information from a line plot?
(4) How do you read information from a circle graph?
Part B:
OBJECTIVE: Students will learn how to create and draw graphs and plots from data sets.
MATERIALS: (Per group of 4 STUDENTS)
1 dry erase grid board
4 dry erase markers of different colors
1 dry eraser
1 Write and Wipe holder
1 Bar Graph Data Table portion of Data Tables Side A sheet
1 sheet of a Supermarket circular
LESSON DISCUSSION:
• Introduce the students to the concept of creating graphs and plots. Ensure they understand that a graph or a plot represents a set of data, facilitating easy comparison of data sets.
• Discuss the properties and components of a bar graph. Emphasize that a bar graph has two axes, each representing a part of the data. The x-axis represents the data categories, while the y-axis represents the quantity of each category.
• Discuss the properties and components of a pictograph. Ensure the students understand that a pictograph uses images to represent a certain quantity. Pictographs require a legend or key to indicate the value each image represents.
• Discuss the properties and components of a line plot. Ensure they understand that a line plot is displayed on a number line to show the distribution of numerical variables, with each x representing a value.
Teacher Preparation:
Step 1: Make a copy of the Data Table Side A and B sheets for each group of 4 students and place them in the Write and Wipe holder. Explain to students they will only be using the Bar Graph Data Table portion of the Data Table Side A sheet.
Step 2: Place students into groups of 4 and distribute the materials to each group.
Procedure:
Step 1: Tell the students to choose 4 different items from the supermarket circular that they would like to use to complete this activity. This will serve as their data.
Step 2: Tell the students to record the name and cost of each item on the data table, arranged from cost to highest cost.
Step 3: Instruct the students to draw 1 vertical line and connect a horizontal line to it, forming a right angle on their dry erase grid boards.
Step 4: Tell the students to label the horizontal line as “Items from Supermarket Circular,” and write the names of the items they chose.
Step 5: Tell the students to label the vertical line as “Cost.” To make a scale to display the data, instruct the students to divide the vertical line into segments of equal lengths. There must be enough segments to contain the highest priced item in their data table.
$ (Dollar Amount)
$ (Dollar Amount)
$ (Dollar Amount)
$ (Dollar Amount)
$ (Dollar Amount)
Step 6: Tell the students to draw a vertical bar from the horizontal axis above each item name. The bar should be scaled to fit all the items.
Step 7: Give the students time to finish their bar graphs.
Step 8: Just by looking at their bar graphs, the students should answer the following questions about their findings:
• Which item has the highest price?
• Which item has the lowest price?
• What is the difference in price between the highest-priced item and the second highest-priced item?
• How much more or less does one item cost than another item?
Step 9: Have the students answer the questions under the data table as a group.
Extension Activity
Step 1: Ask the students to explain why they believe scales on a bar graph are important to accurately represent data.
Step 2: Below their bar graph, have the students make another bar graph using the same data, but without a scale. Tell them to compare the graphs and explain why their data is misrepresented in the second graph. Explain to students that the graph represents the data as if the prices of the items all differ by the same dollar amount.
Part C:
MATERIALS: (Per TEACHER)
60 red tokens
60 blue tokens
60 green tokens
60 yellow tokens
MATERIALS: (Per group of 4 STUDENTS)
1 dry erase grid board
4 dry erase markers of different colors
1 dry eraser
1 Write and Wipe holder
1 Pictograph Data Table portion of Data Tables Side B sheet
1 brown paper bag
Teacher Preparation:
Step 1: Mix the 60 red, 60 blue, 60 green, and 60 yellow tokens in a pile.
Step 2: Fill each brown paper bag with several tokens. It does not matter how many tokens are in each bag as long as the token colors are mixed.
Step 3: Explain to students that they will be using the Pictograph Data Table portion of Data Tables Side B sheet.
Procedure:
Step 1: Distribute the materials to each group of 4 students, including the bags of assorted tokens.
Step 2: Tell the students to open their bags of tokens and separate the tokens according to color. Ask them to record the number of tokens for each color in the Pictograph Data Table.
Step 3: Instruct the students to draw a table consisting of 2 columns and 5 rows on their grid boards. Tell the students to label the first column “Token Color” and the second column “Number of Tokens.” In the first column, the students should write the available colors of tokens in their bag (blue, green, red, and yellow). Their table should look like this:
Step 4: Tell each group to decide what picture they will use to represent tokens. It can be anything. Then, tell the students to decide on the number of tokens each picture will represent. For example, students can decide that use a picture of a token () to represent tokens, and each picture will represent 2 tokens.
Step 5: Tell the students to add this information at the bottom of their table. Their table should look like the example below:
TOKEN COLOR
NUMBER OF TOKENS
KEY: = 2 tokens
Step 6: Tell the students to fill the table by drawing the number of tokens for each color using the key as a basis. For example, if there are 20 blue tokens in the brown bag, and the key that the students used was = 2 tokens, then they should draw ten in the row labeled “Blue.”
Step 7: Give the students enough time to finish their pictographs.
Step 8: Just by looking at their pictographs, the students should answer the following questions about their findings:
• Which token color has the greatest number in the bag?
• Which token color has the least number in the bag?
• How many more or less red tokens are in the bag than blue tokens?
Part D:
MATERIALS: (Per group of 4 STUDENTS)
1 dry erase grid board
4 dry erase markers in different colors
1 dry eraser
1 Write and Wipe holder
1 Line Plot Data Table portion of Data Tables Side B sheet
1 brown paper bag
Procedure:
Step 1: Distribute the materials to each group of 4 students.
Step 2: Ask each student how many siblings each has. They will then record the findings in the Line Plot Data Table sheet.
Step 3: Once they have finished, tell the students to tally their tables. They will count how many of their classmates have 0 siblings, have 1 sibling, have 2 siblings, and so on.
Step 4: Instruct the students to draw a straight horizontal line on their grid boards. Tell the students to place 6 evenly spaced marks on the number line and label these marks “0, 1, 2, 3, 4, and 5 or more.” Lastly, tell the students to label the number line below as “Number of Siblings.” Their boards should look like the example below:
Step 5: Using the data from their Line Plot Data Table sheet, the students will draw 1 X per student. For example, if there are three students with 1 sibling each, the students must draw 3 X’s above the number 1.
Step 6: Give the students enough time to finish their line plots.
Step 7: Just by looking at their line plots, the students should answer the following questions about their findings:
• How many students have 0 siblings?
• How many students have 2 siblings?
• How many more or less students have 3 siblings than 2 siblings?
• How many more or less students have 4 siblings than 5 or more siblings?
Essential Questions for Discussion or Journaling:
(1) How can information be represented in a pictograph?
(2) How can information be represented in a bar graph?
(3) How can information be represented in a line plot?
| Hands On Math | Educational Bootcamp
How Much More?
How much more is item 4 than 3?
How much more is item 3 than 2?
How much more is item 2 than 1?
How much more is item 4 than 1?
How Much Less?
How much less is item 1 than 3?
How much less is item 2 than 4?
How much less is item 1 than 3?
How much less is item 2 than 3?
Token Color
Number of tokens
Blue Green Red Yellow
Hands On Math
DIRECTIONS: Use the information from the line plot below to answer the following questions
Lila is part of a group that plants trees for Earth Day. She kept record of the number of trees each member planted in the line plot.
Number of Trees Planted
How many members planted five trees?
How many members planted more than three trees?
How many members planted less than two trees?
How many members participated in the tree planting event on Earth Day?
Describe one disadvantage of displaying data using a line plot.
DIRECTIONS: Use the information from the data table below to create a bar graph.
Write the steps needed to create a bar graph using information from a tally table.
DIRECTIONS: Use the information from the circle graph below to answer the following questions
How many picked cats as their favorite pet?
How many picked hamsters as their favorite pet?
How many more picked dogs than cats as their favorite pet?
How many more picked birds and hamsters than fish and lizards as their favorite pet?
Describe one disadvantage of displaying data using a circle graph.
Hands On Grade 3 Leveled Practice Answer Key
MA.3.NSO.1.1 - Leveled Practice 1 (Page 4)
1. Expanded Form: 4,000 + 500 + 80 + 2
Word Form: Four thousand five hundred eighty-two
2. Expanded Form: 5,000 + 70 + 6
Word Form: Five thousand seventy-six
3. Expanded Form: 8,000 + 400 + 50
Word Form: Eight thousand four hundred fifty
4. Expanded Form: 7,000 + 200 + 5
Word Form: Seven thousand two hundred five
5. Expanded Form: 6,000 + 800 + 90
Word Form: Six thousand eight hundred ninety Think Tank: There are 4 thousands, 0 hundreds, 5 tens, and 0 ones in 4,050.
MA.3.NSO.1.1 - Leveled Practice 2 (Page 5)
1. C
2. A
3. F
4. E
5. B
6. D
Think Tank: There are 6 thousands, 0 hundreds, 8 tens, and 0 ones in six thousand eighty.
MA.3.NSO.1.1 - Leveled Practice 3 (Page 6)
1. 5,000 + 800 + 40 Five thousand eight hundred forty
2. 6,580 Six thousand five hundred eighty
3. 5,064 5,000 + 60 + 4
4. 9,000 + 80 Nine thousand eighty
5. 2,017 2,000 + 10 + 7
6. 3,105 Three thousand one hundred five
7. 4,000 + 80 + 7 Four thousand eighty-seven
8. 7,707 7,000 + 700 + 7
Think Tank: The digit 0 in a multi-digit whole number represents a place value that has no value. For example, in the number 205, the 0 is in the tens place, meaning there are no tens in this number.
MA.3.NSO.1.2 - Leveled Practice 1 (Page 10)
Note: Student responses will vary for questions #1-6. Think Tank: Because 10 tens is equivalent to 1 hundred.
MA.3.NSO.1.2 - Leveled Practice 2 (Page 11)
TENS AND ONES ONLY HUNDREDS AND ONES
1. 8,342 ones 834 tens 2 ones 83 hundreds 42 ones
2. 2,040 ones 204 tens 0 ones 20 hundreds 40 ones
3. 6,570 ones 657 tens 0 ones 65 hundreds 70 ones
4. 3,825 ones 385 tens 25 ones 38 hundreds 25 ones
5. 1,400 ones 140 tens 0 ones 14 hundreds 0 ones
6. 4,060 ones 406 tens 0 ones 40 hundreds 60 ones
Think Tank: Student responses will vary.
MA.3.NSO.1.2 - Leveled Practice 3 (Page 12)
1.50, 42 5. 7, 71
2.60, 80 6. 80, 98
3.15, 50 7. 30, 90
4.780, 78 8. 2, 400
Think Tank: Because 100 ones is equivalent to 1 hundred.
MA.3.NSO.1.3 - Leveled Practice 1 (Page 18)
1. 2. 3. ONES ONLY
5.
Think Tank: On a number line, numbers increase as you move to the right and decrease as you move to the left. So, if one number is to the right of another, it is greater because it is further along the line where the numbers are increasing.
MA.3.NSO.1.3 - Leveled Practice 2 (Page 19)
1. < 2. <
3. < 4. >
5. < 6. <
7. < 8. >
Think Tank: The place values of the digits in a number are used to compare two numbers by starting from the leftmost digit (highest place value), which is the greatest place value. If the digits are equal, the comparison proceeds to the next digit to the right (next lower place value). This continues until a difference is found or until all the digits have been compared. The number with the larger digit at the first place of difference is the greater number.
MA.3.NSO.1.3 - Leveled Practice 3 (Page 20)
1. 2,468 < 2,684 < 2,846
2. 1,598 < 1,859 < 1,985
3. 4,022 < 4,202 < 4,220
4. 3,608 < 3,806 < 3,860
5. 6,054 < 6,405 < 6,540
6. 5,038 < 5,803 < 5,830
Think Tank: When writing three or more numbers in order from least to greatest, write the smallest numbers first by comparing the digits in the place values. Use the symbol < to indicate order from least to greatest.
MA.3.NSO.1.4 - Leveled Practice 1 (Page 25)
1. 220
2. 750
3. 300
4. 800
Think Tank: Student responses will vary. Acceptable responses: any number from 450 to 549, inclusive.
MA.3.NSO.1.4 - Leveled Practice 2 (Page 26)
1. 350, 400
2. 750, 700
3. 920, 900
4. 190, 200
5. 540, 500
Think Tank: Student responses will vary. Acceptable responses: any number from 35 to 44, inclusive.
MA.3.NSO.1.4 - Leveled Practice 3 (Page 27)
1. 53
2. 95
3. 314 and 341
4. 426
5. 945 and 954
Think Tank: The number 249.
MA.3.NSO.2.1 - Leveled Practice 1 (Page 36)
Note: Student responses should include their work in a place value chart.
1. 75
2. 592
3. 18
4. 803
Think Tank: Adding is the process of bringing two or more numbers together to make a new total, while subtracting is taking one number away from another.
MA.3.NSO.2.1 - Leveled Practice 2 (Page 37)
1. 756 emails
2. 240 miles
3. 33 students
Think Tank: Student responses will vary.
MA.3.NSO.2.1 - Leveled Practice 3 (Page 38)
1. 257
2. 388
3. 276
4. 724
5. 476
Think Tank: Student responses will vary.
MA.3.NSO.2.2 - Leveled Practice 1 (Page 46)
1. B 2. D
3. A 4. C
5. A 6. B
Think Tank: 5 × 7 = 35, 35 ÷ 7 = 5, 35 ÷ 7 = 5
MA.3.NSO.2.2 - Leveled Practice 2 (Page 47)
1. B 2. D
3. A 4. C
5. A 6. D
Think Tank: 6 × 7 = 42 Array should show 6 groups of 7 or 7 groups of 6.
MA.3.NSO.2.2 - Leveled Practice 3 (Page 48)
1. B 2. B
3. D 4. A
5. A 6. B
Think Tank: 5 × 8 = 40, 8 × 5 = 40, 40 ÷ 8 = 5, 40 ÷ 5 = 8; Student responses will vary for the second part of this problem.
MA.3.NSO.2.3 - Leveled Practice 1 (Page 53)
1. 160 2. 280
3. 240 4. 240
5. 600 6. 3,000
7. 2,800 8. 4,500
Think Tank: You can either multiply using the standard algorithm or you can multiply the first digit of the multiple of 10 by the multiplier and add zero to the product.
MA.3.NSO.2.3 - Leveled Practice 2 (Page 54)
1. B
2. D
3. F
4. E
5. C
6. A
Think Tank: You can either multiply using the standard algorithm or you can multiply the first digit of the multiple of 100 by the multiplier and add two zeroes to the product.
MA.3.NSO.2.3 - Leveled Practice 3 (Page 55)
1. 120 minutes
2. 360 words
3. 240 chairs
4. 210 windows
Think Tank: Multiply the one-digit whole number by the first digit of the multiple of 10 or 100, and add one (when multiplying by a multiple of 10) or two zeroes (when multiplying by a multiple of 100) to the product.
MA.3.NSO.2.4 - Leveled Practice 1 (Page 60)
1. True 2. False
3. False 4. True
5. False
6. True
7. True 8. True
Think Tank: Student responses will vary.
MA.3.NSO.2.4 - Leveled Practice 2 (Page 61)
1. True 2. False
3. False 4. True
5. True 6. True
7. True 8. True
Think Tank: Use the related multiplication fact that is 9 × 12 = 108.
MA.3.NSO.2.4 - Leveled Practice 2 (Page 61)
1. 4 2. 8
3. 4 4. 5
5. 9 6. 48
7. 9 8. 36
Think Tank: The product of 4 and 5 is 20, and when 20 is divided by 4, the quotient is 5. They are inverse operations.
MA.3.FR.1.1 - Leveled Practice 1 (Page 69)
1. 1/2 2. 1/4
3. 1/3 4. 1/4
5. 1/6 6. 1/6
Think Tank: A fraction is a way of representing a part of a whole. When we say "fraction of a whole," we mean a part of something that is complete or entire. For example, if a pizza is the whole, then a slice of that pizza would be a fraction of the whole pizza.
MA.3.FR.1.1 - Leveled Practice 2 (Page 70)
1. 2. 3.
Think Tank: The numerator is the top number in a fraction, which represents the part that we are considering or have. The denominator is the bottom number, which represents the total number of equal parts. For example, in the fraction 3/4, 3 is the numerator which means we have 3 parts, and 4 is the denominator which means the whole is divided into 4 equal parts.
MA.3.FR.1.1 - Leveled Practice 3 (Page 71)
1. The fraction bar below has 6 equal sections. Each section represents the fraction 1/6. Shade in 1 of the sections. The fraction represented by the shaded portion is 1/6.
2. The fraction bar below has 4 equal sections. Each section represents the fraction 1/4. Shade in 1 of the sections. The fraction represented by the shaded portion is 3/4.
3. The fraction bar below has 8 equal sections. Each section represents the fraction 1/8. Shade in 1 of the sections. The fraction represented by the shaded portion is 3/8.
Think Tank: Student responses will vary.
MA.3.FR.1.2 - Leveled Practice 1 (Page 76)
1. Six
2. Sixteen
3. Five
4. Seven
5. Ten
Think Tank: Twelve one-fourth sized parts are needed to make three wholes.
MA.3.FR.1.2 - Leveled Practice 2 (Page 77)
1. 1/6 + 1/6 + 1/6 + 1/6 + 1/6
2. 1/5 + 1/5 + 1/5
3. 1/3 + 1/3 + 1/3 + 1/3 + 1/3
4. 1/4 + 1/
5.
Think Tank:
/3 + 1/3 Student models may vary.
MA.3.FR.1.2 - Leveled Practice 3 (Page 78)
1. E 2. B 3. A 4. C
5. D
Think Tank: There are five one-fifth sized pieces in a whole.
MA.3.FR.1.3 - Leveled Practice 1 (Page 82)
1. Word Form: Eight-fifths
2. Word Form: Three-sixths
3. Word Form: Four-sixths
Numeral-Word Form: 8 fifths
Numeral-Word Form: 3 sixths
Numeral-Word Form: 4 sixths
4. Word Form: Nine-tenths
5. Word Form: Ten-twelfths
Numeral-Word Form: 9 tenths
Numeral-Word Form: 10 twelfths
Think Tank: Use the fractional vocabulary term for a denominator 2, which is halves. Then use the word form of the numerator. Hence, 3/2 in word form is three-halves.
MA.3.FR.1.3 - Leveled Practice 2 (Page 83)
1. E 4. F
2. A 5. B
3. D 6. C
Think Tank: Use the fractional vocabulary term for a denominator 2, which is halves. Then use the number form of the numerator. Hence, 3/2 in word form is 3 halves.
MA.3.FR.1.3 - Leveled Practice 3 (Page 84)
1. Six halves 6 halves
2. 3/4 3 fourths
3. 9/3 Nine thirds
4. Five eighths 5 eighths
5. 11/2 Eleven halves
6. 5/12 5 twelfths
7. Four tenths 4 tenths
8. 11/12 Eleven twelfths
Think Tank: No, saying "five sixths" is not the same as writing "6 fifths". "Five sixths" means the whole is divided into six equal parts and we have five of those parts. However, "6 fifths" means the whole is divided into five equal parts and we have six of those parts, which is more than one whole.
MA.3.FR.2.1 - Leveled Practice 1 (Page 90)
1. 1/4, 2/4, 3/4
2. 1/3, 2/3
3. 1/6, 2/6, 3/6, 4/6, 5/6
Think Tank: To represent a fraction on a number line, mark off equal parts corresponding to the denominator. Then, count parts equal to the numerator. For instance, to represent 3/4, divide the line into 4 parts and mark the first 3.
MA.3.FR.2.1 - Leveled Practice 2 (Page 91)
Think Tank: The 1/2 mark will be positioned identically on both number lines because it represents the same relative position on each scale. On the line from 0 to 1, it's halfway, and on the line from 0 to 2, it's also halfway. Even though the second line is divided into smaller increments, the position of 1/2 remains the same because it's a relative measurement.
MA.3.FR.2.1 - Leveled Practice 3 (Page 92)
1. 6/8 is bigger than 5/8 because it is farther from 0 in the number line.
2. 9/10 is bigger than 7/10 because it is farther from 0 in the number line.
3. 4/12 is bigger than 3/12 because it is farther from 0 in the number line.
Think Tank: Student responses will vary.
MA.3.FR.2.2 - Leveled Practice 2 (Page 100)
Note: Student responses may vary for questions #1-3.
Think Tank: No, if you plot two equivalent fractions on a number line, they would be placed at the same points. This is because equivalent fractions represent the same value.
MA.3.FR.2.2 - Leveled Practice 2 (Page 101)
1. Manny
2. Emma
3. Vegetable
Think Tank: Equivalent fractions are those that represent the same part of a whole. If the fractions are put in their lowest terms, the fractions will be exactly the same.
MA.3.FR.2.2 - Leveled Practice 3 (Page 102)
1. >
2. <
3. <
Think Tank: Student responses will vary.
MA.3.AR.1.1 - Leveled Practice 1 (Page 110)
1. Commutative
3. Associative
5. Distributive
2. Identity
4. Commutative
6. Associative
Think Tank: Student responses will vary.
MA.3.AR.1.1 - Leveled Practice 2 (Page 111)
1. 2; Distributive
2. 3; Commutative
3. 2; Associative
4. 6; Distributive
Think Tank: Student responses will vary.
MA.3.AR.1.1 - Leveled Practice 3 (Page 112)
1. Yes, because 5 × 4 = 20 and 4 × 5 = 20.
2. No. To get the same answer, he would have to find the answer to the equation (8 × 10) + (8 × 4).
3. Student responses will vary.
Think Tank: Student responses will vary.
MA.3.AR.1.2 - Leveled Practice 1 (Page 116)
1. × 2. ×
3. + 4. ×
5. ÷ 6. +
Think Tank: It is important to know the order of operations because if you solve an equation out of order, the result may be incorrect.
MA.3.AR.1.2 - Leveled Practice 2 (Page 117)
1. 2 2. 3
3. 9 4. 17
5. 13 6. 6
Think Tank: Using your knowledge of PEDMAS, determine which part of the equation to solve first. Once you get the answer, use it to solve the other part of the equation.
MA.3.AR.1.2 - Leveled Practice 3 (Page 118)
1. 5 × 10 + 3 = 53
2. 24 - 12 - 6 = 6
3. $150 - ($15 + $55) = $80
Think Tank: Student responses will vary.
MA.3.AR.2.1 - Leveled Practice 1 (Page 121)
1. D
2. A
3. E 4. C 5. F 6. B
Think Tank: The equation 8 × 9 will give the greater answer. This is because 8 × 9 equals 72, while 72 ÷ 8 equals 9. Therefore, 72 is greater than 9.
MA.3.AR.2.1 - Leveled Practice 2 (Page 122)
1. 7 × 9 = 63 2. 6 × 9 = 54
3. 5 × 4 = 30 4. 7 × 2 = 14
5. 7 × 6 = 42 6. 3 × 5 = 15
Think Tank: Student responses will vary.
MA.3.AR.2.1 - Leveled Practice 3 (Page 123)
1. 21 ÷ 3 = 7
2. 24 ÷ 6 = 4
3. 32 ÷ 8 = 4
Think Tank: Student responses will vary.
MA.3.AR.2.2 - Leveled Practice 1 (Page 127)
1. True 2. True
3. True 4. False
5. False 6. True
Think Tank: 4 × 5 = 2 × 10 is true because both sides of the equal sign are equal to 20. 3 × 6 = 4 × 9 is false because 3 × 6 = 18 and 4 × 9 = 36.
MA.3.AR.2.2 - Leveled Practice 2 (Page 128)
1. False, because 4 × 2 = 8, while 20 ÷ 4 = 5.
2. True, because both sides of the equal sign are equal to 6.
3. False, because 6 × 4 = 24, while 24 ÷ 2 = 12.
Think Tank: The grocery store’s claim is true. 4 × 3 = 12 and 6 × 2 = 12
MA.3.AR.2.2 - Leveled Practice 3 (Page 129)
1. True, because both sides of the equal sign are equal to 32.
2. True, because both sides of the equal sign are equal to 5.
3. False, because 14 ÷ 7 = 2, while 6 × 2 = 12.
Think Tank: Possible responses:
3 × 2 = 36 ÷ 6 ,
3 × 3 = 36 ÷ 4
3 × 4 = 36 ÷ 3
3 × 6 = 36 ÷ 2
3 × 13 = 36 ÷ 1
MA.3.AR.3.1 - Leveled Practice 1 (Page 135)
1. Even 2. Even 3. Odd
4. Odd 5. Even 6. Even
7. Even
8. Even
Think Tank: The number 27 is odd. Visual models may vary.
MA.3.AR.3.1 - Leveled Practice 2 (Page 136)
Odd Numbers: 215, 527, 657, 811, 945
Even Numbers: 100, 398, 400, 782, 802
Think Tank: In whole numbers, the ones place determines whether a number is odd or even. In this case, there is a 1 in the ones place and the number 1 is odd. The number 901 is odd.
MA.3.AR.3.1 - Leveled Practice 3 (Page 137)
1. Cannot be done; Odd
2. 28 + 28; Even
3. 44 + 44; Even
4. Cannot be done; Odd
5. 127 + 127; Even
6. 83 + 83; Even
Think Tank: Student responses will vary.
MA.3.AR.3.2 - Leveled Practice 1 (Page 140)
1. 12, 6, 9, 3
2. 12, 36, 42, 24, 18
3. 12, 16, 28, 32
4. 16, 24, 40, 56
5. 10, 25, 50, 65
6. 28, 35, 49
Think Tank: Student models will vary.
MA.3.AR.3.2 - Leveled Practice 2 (Page 141)
Multiples of 3: 6, 12, 15, 18, 42, 66, 24, 96, 81, 30, 72
Multiples of 4: 8, 12, 56, 24, 40, 96, 72
Multiples of 5: 15, 40, 35, 30
Think Tank: 6 + 6 + 6 + 6 + 6 + 6 = 36 or 6 × 6 = 36.
MA.3.AR.3.2 - Leveled Practice 3 (Page 142)
1. No, because 50 is not a multiple of 8.
2. Yes, because 30 is a multiple of 6.
3. No, because 42 is not a multiple of 4.
Think Tank: A number is a multiple of a given number if that number can be expressed as a product of the given number and another whole number.
MA.3.AR.3.3 - Leveled Practice 1 (Page 152)
1. True 2. False
3. False
4. True
5. True
6. True
7. False
Think Tank: Any number times an even number will always give you an even product because you are multiplying it an even number of times.
MA.3.AR.3.3 - Leveled Practice 2 (Page 153)
1. Output: 256, 381, 504, 553
2. Output: 39, 78, 124, 157
3. Output: 15, 25, 30, 45
4. Output: 1, 2, 3, 5
5. Output: 994, 153, 199, 245
6. Output: 12, 24, 36, 40
Think Tank: The output always increases in the addition and the multiplication tables.
MA.3.AR.3.3 - Leveled Practice 3 (Page 154)
1. Divide by 3
2. Multiply by 3
3. Subtract 3
4. Add 5
5. Divide by 3
6. Add 6
Think Tank: The sum of 2 even numbers is always even. The product of 2 even numbers is always even.
MA.3.M.1.1 - Leveled Practice 1 (Page 162)
1. 10 inches
2. 8 inches
3. 5 inches
Think Tank: Student responses will vary.
MA.3.M.1.1 - Leveled Practice 2 (Page 163)
1. 40 mL
2. 20 mL
3. 70 mL
Think Tank: Student responses will vary.
MA.3.M.1.1 - Leveled Practice 3 (Page 164)
1. 75° Fahrenheit
2. 50° Fahrenheit
3. 25° Fahrenheit
Think Tank: The temperature of a hot coffee will be higher than the temperature of an iced coffee because a hot coffee is hotter than an iced coffee.
MA.3.M.1.2 - Leveled Practice 1 (Page 172)
1. Approximately 1.5 liters
2. 4,000 kilograms
3. 10 inches because five 2 inches is the same as 2 inches × 5 = 10 inches.
Think Tank: Student responses will vary.
MA.3.M.1.2 - Leveled Practice 2 (Page 173)
1. If he repeats the experiment 5 times, he will need 50 mL. If he used half the amount for each of the 5 experiments, he would use 25 mL.
2. 400 grams
3. 10 paperclips because 40 cm ÷ 4 cm = 10.
Think Tank: Student responses will vary.
MA.3.M.1.2 - Leveled Practice 3 (Page 174)
1. 184 liters
2. 1 kilogram per hour
3. 3 yards
Think Tank: Student responses will vary.
MA.3.M.2.1 - Leveled Practice 1 (Page 177)
1. 7:30 AM
2. 11:30 AM
3. 8:00 PM
4. 4:15 PM
5. 2:30 PM
Think Tank: Each number on an analog clock shows how many minutes have passed in the hour. Each number means 5 minutes. For example, if the minute hand points to 3, it means 15 minutes have passed. This is because 3 times 5 equals 15. So, each number on the clock stands for 5 minutes.
MA.3.M.2.1 - Leveled Practice 2 (Page 178)
1. 4:30 2. 9:47
3. 12:22 4. 3:56
5. 7:12 6. 10:08
Think Tank: Each number on an analog clock represents 5 minute intervals in relation to the minute hand.
MA.3.M.2.1 - Leveled Practice 3 (Page 179)
Think Tank: It is important to know how to read time on an analog clock because digital formats of time are not always available. Determining elapsed time is also important and stopwatches may not always be available.
MA.3.M.2.2 - Leveled Practice 1 (Page 183)
1. 2 hours
3. 1 hour 44 minutes
5. 2 hours 28 minutes
Think Tank: Elapsed time is determined by counting the number of hours and minutes between the start and end times.
MA.3.M.2.2 - Leveled Practice 3 (Page 184)
Think Tank: Addition because to find the end time, we need to add the elapsed time to the start time.
MA.3.M.2.2 - Leveled Practice 3 (Page 185)
1. 2 hours 45 minutes
2. 3 hours 30 minutes
3. 7 hours 30 minutes
Think Tank: Subtraction because to find the start time, we need to subtract the elapsed time from the end time.
MA.3.GR.1.1 - Leveled Practice 1 (Page 190)
Think Tank: A line goes on in both directions, while a ray goes on in one direction only. A line segment does not go on in both direction (has end points in both ends).
MA.3.GR.1.1 - Leveled Practice 2 (Page 191)
1. 2. 3.
Think Tank: Intersecting lines cross each other at exactly one point, while parallel lines do not cross each other.
MA.3.GR.1.1 - Leveled Practice 3 (Page 192)
1. E
2. B
3. C
4. G
5. A
6. F
7. D
Think Tank: Intersecting lines cross each other at any angle, while perpendicular lines cross each other at exactly a right angle.
MA.3.GR.1.2 - Leveled Practice 1 (Page 196)
1. 2.
3.
Number of sides
Classification Type of Shape
Number of Angles
Number of Right Angles
Number of sides
Classification Type of Shape
Number of Angles
Number of Right Angles
Number of sides
Classification Type of Shape
Number of Angles
Number of Right Angles
Think Tank: A polygon is a closed figure composed of straight sides. The most important properties of a polygon are its sides, angles, and vertices.
MA.3.GR.1.2 - Leveled Practice 2 (Page 197)
1. Rhombus
2. Trapezoid
3. Square
Think Tank: The different types of quadrilaterals are rectangle, square, rhombus, parallelogram, and trapezoid. They differ based on the properties of their sides and angles. All quadrilaterals are closed figures with 4 straight sides.
MA.3.GR.1.2 - Leveled Practice 3 (Page 198)
1. True
2. False
3. True
Think Tank: The shape is not a quadrilateral because it does not have 4 straight sides.
MA.3.GR.1.3 - Leveled Practice 1 (Page 203)
1. 2. 3.
Think Tank: The diagonal of a rectangle is not a line of symmetry because if you fold the rectangle along its diagonal, the two halves do not fit perfectly to each other.
MA.3.GR.1.3 - Leveled Practice 3 (Page 204)
1. Line B
2. Line D
3. Line C
Think Tank: A parallelogram does not have a line of symmetry because it is not possible to cut a parallelogram in half, with each side mirroring the other side.
MA.3.GR.1.3 - Leveled Practice 3 (Page 205)
1. One line of symmetry
2. One line of symmetry
3. One line of symmetry
Think Tank: The figure has one horizontal line of symmetry.
MA.3.GR.2.1 - Leveled Practice 1 (Page 209)
1. 16 units2 2. 8 units2
3. 9 units2 4. 12 units2
5. 6 units2 6. 4 units2
Think Tank: A square unit is the metric unit used to measure area. Area is always measured in square units of a fixed size or unit of measurement.
MA.3.GR.2.1 - Leveled Practice 2 (Page 210)
1. Drawings may vary
2. Drawings may vary
3. Drawings may vary
Think Tank: No, you cannot create a square that has an area of 15 units where each side is a whole number because a square is found by multiplying the length of one side by itself and no two whole numbers multiply to get a product of 15.
MA.3.GR.2.1 - Leveled Practice 3 (Page 211)
1. Area is 16 square units. Drawings may vary.
2. 4 units. Drawings may vary
3. 6 units. Drawings may vary
Think Tank: Student responses will vary.
MA.3.GR.2.2 - Leveled Practice 1 (Page 214)
1. 24 inches2
2. 15 inches2
3. 40 inches2
Think Tank: To find the area of a rectangle, multiply the length of the rectangle by its width.
MA.3.GR.2.2 - Leveled Practice 2 (Page 215)
1. 35 inches2
2. 81 centimeters2
3. 37 feet2
Think Tank: If you are given a square with only 1 side length, it is important to remember that all sides of a square are the same. Therefore, you can multiply the given side length by itself in order to find the area.
MA.3.GR.2.2 - Leveled Practice 3 (Page 216)
1. 8 feet2 (Equations may vary.)
2. 49 inches2 (Equations may vary.)
3. 56 feet2 (Equations may vary.)
Think Tank: Student responses will vary.
MA.3.GR.2.3 - Leveled Practice 1 (Page 220)
1. 22 inches
2. 26 centimeters
3. 24 feet
Think Tank: Add the side lengths of rectangle to find its perimeter.
MA.3.GR.2.3 - Leveled Practice 2 (Page 221)
1. Perimeter: 22 inches; Area: 28 square inches
2. Perimeter: 30 centimeters; Area: 36 square centimeters
3. Perimeter: 30 feet; Area: 44 square feet
Think Tank: If you have the area and one side length of a rectangle, you can find the other side length by dividing the area by the given side length. Once you have both side lengths, you can find the perimeter by adding all sides.
MA.3.GR.2.3 - Leveled Practice 3 (Page 222)
1. Area: 36 square centimeters
2. Perimeter: 36 inches; Area: 72 square inches
3. Student responses will vary.
Think Tank: Counting the unit squares is like multiplying the number of squares along the length with the number along the width. This is the same as multiplying the lengths of the sides.
MA.3.GR.2.4 - Leveled Practice 1 (Page 231)
1. 16 units
2. 16 units
3. 16 units
Think Tank: It is important to know how many square units each square represents to correctly identify the area. Not all figures are drawn using 1 unit squares.
MA.3.GR.2.4 - Leveled Practice 2 (Page 232)
Think Tank: Student responses will vary.
MA.3.GR.2.4 - Leveled Practice 3 (Page 233)
1. Perimeter: 60 inches
Area: 175 square inches
2. Perimeter: 32 centimeters
Area: 28 square centimeters
3. Perimeter: 44 inches
Area: 72 square inches
Think Tank: To find the perimeter of an irregular figure, add the side lengths. To find the area of an irregular figure, divide the figure into smaller, nonoverlapping rectangles, find the area of these rectangles, and add the areas.
MA.3.DP.1.1 - Leveled Practice 1 (Page 243)
NUMBER OF PINEAPPLES PICKED GIRLS FREQUENCY
Key: = 2 pineapples
(Drawing of picture graph may vary.)
Think Tank: When creating a picture graph, first determine your key for how many tally marks each picture will represent. Then, draw a picture to represent the number of tally marks for each value. Be sure to add a key so people can accurately read your data.
MA.3.DP.1.1 - Leveled Practice 2 (Page 244)
(Drawing of bar graph may vary.)
Think Tank: To make a bar graph: (1) Draw a chart. (2) Determine the scale you want to use based on the data. (3) Label your axis. (4) Plot the data using a bar that corresponds to the data. (5) Title your graph.
MA.3.DP.1.1 - Leveled Practice 3 (Page 245)
(Drawing of line plot may vary.)
Think Tank: Create a line and represent the values that are being tallied on the number line. Count the number of tally marks. Make X’s equivalent to the number of tally marks associated with each value.
MA.3.DP.1.2 - Leveled Practice 1 (Page 253)
1. 1
2. 9
3. 7
4. 25
Think Tank: Student responses will vary.
MA.3.DP.1.2 - Leveled Practice 2 (Page 254)
1. 25 bottles
2. 15 bottles
3. 5 bottles
4. 20 bottles
Think Tank: Student responses will vary.
MA.3.DP.1.2 - Leveled Practice 3 (Page 255)
1. 22
2. 11 3. 6 4. 2
Think Tank: Student responses will vary.



























































































