SAMPLE: Mathematics and Statistics for Aotearoa New Zealand Year 8

Unit 1 Number structure

3. Exponents and square roots

mathematics and statistics for

Aotearoa New Zealand

5. Integers

1. Identify fractions, decimals and percentages

2. Compare, order and convert between fractions, decimals and percentages

3. Multiplication of fractions and decimals. Finding percentages

Unit 6 Measurement

Unit 1: Topic 1

Whole numbers and decimals

Writing numbers

53 654 2024 2.45 100

Numbers can be shown in various ways:

Numeral form: 53 654

Word form: two thousand and twenty-four

Expanded form: 2 + 4 10 + 5 100

Exponent form: 102

Guided practice

1 Write 53 654 in word form.

2 Write 2024 in expanded form.

DRAFT

3 Write one hundred and two thousand, five hundred and forty-nine in numeral form.

Ordering numbers This can be done in ascending or descending order.

4 a Write the numbers from the top of the page in numerals in ascending order.

b Write 3.45, 345 and 34.5 in descending order.

c Write 5 3 , 52 and 5 4 in descending order.

Comparing numbers

Large numbers can be abbreviated using exponents or recognised abbreviations. For example, a house by the water might be worth $1.2M ($1 200 000) and a person might earn $70K a year ($70 000).

5 Identify the larger number in each pair by writing > (is greater than) or < (is less than) on the line between them.

a 102 70

b $3500 $25K

c 150 000 1.5M

d 21 3 3

e Why is it not possible to place either symbol between this pair: 10 3 1000?

Independent practice

1 Write these numbers in word form.

a 12 145

b 12.145

c 10 000 + 2000 + 100 + 40 + 5

DRAFT

d 10 + 2 + 0.1 + 0.04 + 0.005

2 Write these numbers in exponent form.

a 1000

b 100 000

c 10 000

d 4 × 4 × 4 × 4 × 4

e 9 × 9 × 9 × 9 × 9 × 9 × 9

3 Place value

Identify the value of the red digit in these numbers by looking at its place in the full number. Write the value of the digits in words. For example, for 35 876, the red digit is worth five thousand.

a 35 8 078

b 57 498 089

c 14 763 009

d 1 654 896 423

4 Write the numbers on the place value grid. Put in a decimal point when necessary.

a 5M

b 1.25M

c 1 2 of 5

d 350K

e 5 × 10 5

f 1.75B

g 57B h 1 2 of 1.5

DRAFT

5 Look at the table in question 4. Write the value of the digit 5 in each number in word form. a b c d

6 Write these lists in ascending order.

a 2.5, 255, 0.25, 25, 0.025

b 3.5M, 3.55, 3.53, 3500, 0.35

c 750K, 750M, 75 000, 70.005, 70.05

d 4.7, 7.4, 4.07, 4.704, 0.47

7 Write these lists in descending order.

a 3.5, 355, 0.35, 35, 0.035

b 8.58, 8500, 0.85, 8.5M, 8.55

c 2005, 250B, 20.5M, 25 000, 250K

d 3.05, 30.5, 0.035, 0.35, 30.05

DRAFT

8 Fill in the gaps to compare each number pair by writing < or >.

Extended practice

Rounding numbers

You probably know that numbers are sometimes rounded. For example, if you needed to find the answer to 98 + 99, you could round the numbers to the nearest ten and estimate the answer to be close to 100 + 100.

Numbers are also rounded if knowing the exact number is not important. For example, a newspaper might report that 20 000 people were watching a match, even if the actual number was 19 576.

Numbers can be rounded to the nearest ten, nearest hundred, nearest thousand, or whatever is appropriate.

1 Round these numbers to the nearest thousand.

a 1875

b 19 235

c 996

d 32 828

Before a number is rounded, you need to know which place value column you are rounding to. For example, if you wanted to round the number in question 1d (32 828) to the nearest ten thousand, you focus on the digit to the right of it. Here’s how:

We can see that the circled digit is less than 5. So, we round down to the nearest ten thousand. 32 828 rounded to the nearest ten thousand is 30 000. (All the columns to the right are now empty. So, a zero goes in each empty space.)

DRAFT

The ABC of rounding

Decide on the column you are rounding to.

If the digit is 5 or above, round UP

If the digit is less than 5, round DOWN Circle the digit to the right. Tth th h t u 3 2 8 2 8

2 Round 32 828 to the nearest.

a hundred

b ten

3 If a prize of $22 935 had to be rounded, would you prefer it to be rounded to the nearest ten thousand, thousand, hundred or ten? Give a reason for your answer.

4 Round to the nearest thousand.

a 38 759

b 41 445

c 25 555

d 451 445

5 Round to the nearest hundred thousand.

a 178 280

b 413 633

c 298 729

d 1 132 405

6 Round to the nearest tenth.

a 1.82

b 0.48

c 37.09

d 7.99

DRAFT

7 Scientists say that the Moon is an average of 384 399 kilometres away from Earth.

a What would be your rounded distance to the Moon. Give a reason for the way you have rounded the distance.

b Why do you think the average distance is given and not the exact distance?

Unit 1: Topic 2

Prime factorisation

Factors

The factors of a number are numbers that it can be divided by. A factor is always a positive integer (a whole number).

For example, 2 can be divided by 1 and 2. So, the factors of 2 are 1 and 2 (two factors).

4 can be divided by 1 and 2 and 4. So, the factors of 4 are 1, 2 and 4 (three factors).

Guided practice

1 Write the factors of these numbers.

a 3

b 6

c 10

d 5

Prime and composite numbers

Positive integers are either prime numbers or composite numbers.

A prime number has just two factors (1 and itself). 2 is a prime number. A composite number has more than two factors. 4 is a composite number.

The number 1 is a special number. It is the only number that is neither a prime number nor a composite number.

DRAFT

2 What is the only number between 6 and 10 that is a prime number?

3 List the factors of 28.

4 Which number between 31 and 40 has:

a the same number of factors as 28?

b more factors than 28?

5 There is only one even prime number. What is it?

Complete the chart to show the factors of the prime and composite numbers from 11 to 20.

Independent

practice

Prime factors

(Reminder: 1 is NOT a prime number.)

Prime factors are the prime numbers that multiply together to make a whole number. For example, 2 and 3 are the prime factors of 6. How do we know? We can show it in a factor tree:

The prime factors of 6 are 2 and 3 6 = 2 × 3

1 Fill in the blanks in these factor trees to show each composite number as the product of its prime factors.

The prime factors of 10 are and

The only prime factor of 9 is

The prime factors of 15 are and = ×

The prime factors of 35 are and = ×

The prime factors of 21 are and = ×

DRAFT

The prime factors of 39 are and = ×

For some composite numbers we need a larger factor tree. For example, if we start with 8, we end up with 2 × 4. But 4 is not a prime number. We need to keep going until we end up with prime numbers. The factor tree for 8 looks like this:

Now we end with prime numbers. So, the only prime factor of 8 is 2. 8 = 2 × 2 × 2

2 Complete each factor tree to show each composite number as the product of its prime factors.

The prime factors of 20 are and 20 = × ×

The prime factors of 18 are and = × ×

The prime factors of 28 are and = × × ×

DRAFT

It is sometimes easier to use exponents to show the prime factorisation of a number. For example, on the right is a factor tree for 16. It is quicker to show the prime factorisation as 24 .

The prime factors of 36 are and = ×

× The only prime factor of 16 is 2. 16 = 2 × 2 × 2 × 2 = 24

3 Complete the factor trees. Use exponents where possible to show the prime factorisation of each starting number.

a 68 2 × ×

The prime factors of 68 are and 68 = 2 × 2 × 2 × = 2 ×

b 27 9 × ×

DRAFT

The prime factor of 27 is 27 = × × = c 40 2 × × ×

The prime factors of 40 are and 40 = × × × = × d 56 7 × × ×

The prime factors of 56 are and 56 = × × × = ×

Extended practice

Highest common factor (HCF)

Reminder: The HCF of a pair of numbers is the number with the greatest value that is a factor of each number. For example: the factors of 12 are: 1, 2, 3, 4, 6, 12 the factors of 18 are: 1, 2, 3, 6, 9, 18

We can see that 1, 2, 3 and 6 are common factors (they are in both lists).

The 6 digit is red because it is the HCF (the number with the greatest value that is a factor of each number).

1

a Write the factors of this pair of numbers. 8 24

b Draw a circle round the common factors of each number.

c Draw a star over the highest common factor (HCF) of 8 and 24.

d The HCF of 8 and 24 is

2 Find the HCF of each pair, or group of numbers.

a The HCF of 24 and 40 is:

b The HCF of 35 and 42 is:

c The HCF of 6, 12 and 28 is:

d The HCF of 18, 36 and 42 is:

DRAFT

3 You can use the HCF to simplify fractions. For example, we can make 5 10 simpler like this:

The factors of 5 are: 1 and 5. The factors of 10 are: 1, 2, 5 and 10.

The HCF is 5.

If we divide the numerator and denominator by the HCF (5), the simple fraction is 1 2

Simplify these fractions by finding the HCF. a 9 12 b 12 18 c 25 125 d 16 28

4 What is the mystery number?

It is a 2-digit odd number that is a multiple of both 7 and 5. It is a factor of both 210 and 315. What is the mystery number?

Unit 1: Topic 3 Exponents

and square roots

In mathematics we often look for shortcuts.

This is a shortcut for 3 + 3 + 3 + 3 + 3:

3 × 5 = 15

We can also use exponents as shortcuts.

A short way of writing 3 × 3 is 32.

The base number is 3.

The small 2 is the exponent. It can also be called the index or power

The exponent tells us to use the base number (3) in a multiplication two times.

The base number is 3. So, 32 = 3 × 3 = 9.

Guided practice

32 base number exponent (or index, or power)

1 Write the multiplication as a base number and exponent. Remember to write the exponent at the top and smaller than the base number.

Multiplication Base number and exponent

e.g. 3 × 3 × 3 3 3

a 2 × 2 × 2 × 2 × 2

b 4 × 4 × 4

c 8 × 8 × 8 × 8

d 5 × 5 × 5 × 5 × 5

e 7 × 7 × 7 × 7 × 7 × 7

f 10 × 10 × 10 × 10

2 Fill in the gaps in the table.

Base number and exponent The base number is used in a multiplication: The multiplication is: The value of the number is:

e.g 42 two times 4 × 4 16

a 3 3 three times

b 24

c 5 3

d 6 2

e 9 2

f 10 3

For 3 3 we can say 3 to the third power, 3 to the power of three or 3 cubed.

Square roots

To understand what is meant by the square root of a number, we need to look at square numbers.

4 squared can be written as 42. In a diagram it looks like this:

A square root goes the opposite way.

4 squared is 16. So, the square root of 16 is 4.

The symbol for square root is √ . We can write it like this: √ 16 = 4

= 4 × 4 = 16

×

3 Find the square root of these numbers by saying, “What number, times itself, makes the number?”

4 All the starting numbers in question 3 were square numbers. What if the starting number is not a square number? We can give the approximate square root.

Example: √ 7 = ? We know that √ 4 = 2 and √ 9 = 3.

So √ 7 is between 2 and 3.

e.g 7 4 and 9

a 10

b 42

c 20 d 52

= 2 and √9 = 3 2 and 3

Independent practice

Base numbers with exponents, such as 27, can look small. However, when you expand the number, you might be surprised at how large the actual value is. For example, the value of 27 is greater than 100.

1 Find the value of these numbers. Begin by expanding the number. Your teacher may ask you to use a calculator for some of them. a 27 = 2 × 2 × 2 × 2 × 2 × 2 × 2 =

2 Circle the number with the greater value in each pair.

a 9 4 or 8 5 b 5 3 or 35

3 Find the value of the exponent.

a 5 to the power of = 15 625 b 10 to the power of = 1 million?

4 Find the approximate square root, then the actual square root (to 2 decimal places). You will need to use a calculator with a square root function for the actual square root.

DRAFT

5 Identify and circle all the prime numbers by making sure that they only have two factors: 1 and the number itself.

Remember also that 1 is a special number and is neither prime nor composite.

30292827262524232221

40393837363534333231

50494847464544434241

60595857565554535251

70696867666564636261

6 a The highest prime number on the grid is b True or false? All the prime numbers are odd.

c True or false? More of the composite numbers between 1 and 100 are even than odd.

7 We can find the square root of some less-common numbers if the number is written as a product of prime factors. (You may need to look back at Topic 2 as a reminder.) Study the first example and complete the second one.

DRAFT

To find the square root of the starting number: Find the prime factors. Multiply the prime factors.

Using prime factorisation we see that

196 = 2 × 2 × 7 × 7

196 = 22 × 72

The prime factors of 196 are 2 and 7.

Multiple the prime factors: 2 × 7 = 14

So, √ 7

196 = 14

Check: 142 = 196

The prime factors of 225 are _____ and _____.

Multiply the prime factors: _____ × _____ = _____

So, √ 225 = _____

Check: 2 = _____ 225 = _____ × _____ × _____ × _____ 225 = ________2 × ________2

Extended practice

Negative exponents

A base number can have a negative exponent, like this: 2−2.

Negative is the opposite of positive.

A positive exponent involves multiplication. For example, 22 = 2 × 2 = 4.

The opposite of multiplication is division. A negative exponent involves division.

A negative exponent tells us how many times to divide 1 by the base number

2−2 tells us to divide 1 by the base number (2) and then divide by 2 a second time.

First time: Divide 1 by 2 = 1 2 or 0.5

Second time: Divide 1 2 by 2 = 1 4 or 0.25

So, 2−2 = 1 ÷ 2 ÷ 2 = 0.25

1 Your teacher may ask you to use a calculator for some of the following.

a 8 −1 = 1 ÷ 8 =

b 8 −2 = 1 ÷ 8 ÷ 8 =

c 4−1 =

d 4−2 =

e 10 −2 =

f 10 −3 =

2

DRAFT

In the last question we started with 1 and then divided. A different way of looking at positive exponents it is to start at 1 and then multiply. For example, 32 = 1 × 3 × 3 = 9.

Find the value of these by expanding in the same way as the example.

a 6 3

b 4 4

3 You may have heard of perfect numbers. A perfect number is a number that equals the sum of its factors (apart from itself). The number 6 is a perfect number; its factors (apart from itself) are 1, 2 and 3, the sum of which is 6. However, 4 is not a perfect number, since its factors of 1 and 2 equal 3. There is just one other perfect number below 100. What is it?

Unit 2: Topic 1

Calculations involving rounding and estimation

In Topic 3 of Unit 1, you looked at rounding numbers. On the right is a reminder of the ABC of rounding.

The ability to round numbers quickly is important in order to estimate when calculating. Why is being able to estimate useful? Imagine Maia is saving for a bat and a ball.

To pay for it, the shop needs the exact amount. If the price of a bat and a ball is $14.90, plus $9.90, that’s how much she has to pay. However, by rounding the amounts, she knows approximately how much she needs to save: $15 + $10 = $25.

Guided practice

The ABC of rounding

Decide on the column you are rounding to.

Circle the digit to the right.

If the digit is 5 or above, round UP

If the digit is less than 5, round DOWN

1 Nikau loves to use a calculator. However, he never estimates and sometimes he gets the wrong answer as he presses the keys quickly. Look at his calculator answers in this table. Decide whether the calculator answer can be trusted by rounding the numbers and estimating the answers. If the calculator answer is wrong, write the exact answer.

Rounding decimals

Calculations can sometimes lead to a huge number of decimal places. For example, if you divide $300 between 6 people, they get $50 each. But, what about $300 ÷ 7? Aria’s estimate was excellent: “It would be a bit less than the answer to 300 ÷ 6. Maybe 40?”

The calculator gave the answer in milliseconds: 42.857 1429. (A scientific calculator would provide you with even more decimal places.)

Nobody could count $42.857 1429 in coins. In the real world, we need to round decimal numbers sensibly.

2 Round these numbers to two decimal places. (Remember the ABC of rounding.)

a 3.918

b 32.758

c 43.812

d 70.4448

e 7.38775

f 67.042249

g 395.958

h 0.775755

i 7.38279

j 99.994

DRAFT

Independent practice

New Zealand is in the top ten of island countries by size. However, compared to the United Kingdom, which is similar in land area, the population of New Zealand is very small.

Use the facts and figures in the table to complete the activities.

Comparing New Zealand with the United Kingdom (UK)

New Zealand population 5.2M (to the nearest 100 000)

UK population

DRAFT

New Zealand: land area

68.34M to the nearest 10 000)

UK: land area

269 055 km2

New Zealand: length of roads

243 610 km2

UK: length of roads

Paved roads:

5981.3 km North Island

4924.4 km South Island

Unpaved roads:

31 400 km

Paved roads:

422 100 km

Unpaved roads:

54 350 km

1 Circle any of the following that could be the actual population of New Zealand. a 5 189 675 b 522 076 c 5 100 494 d 5 154 989 e 5.22M

2 Circle any of the following that could be the actual population of the United Kingdom. a 68.9M b 68 327 453 c 68 347 297 d 68 344 539 e 68.342M

3 Which of the following is the best estimate for the difference between the land areas of the two countries? 25 000 km2, 2500km2, 26 000 km2 or 2600 km2?

4 By how much is the land area of New Zealand bigger than that of the UK?

5 Estimate the total land area of the two countries. Explain the way that you have rounded the numbers for your estimate.

6 What is the actual total land area of the two countries?

DRAFT

7 Which of these is the best estimate for the total length of paved roads in New Zealand? 11 500 km, 10 500 km or 11 000 km?

8 What is the difference between the length of paved roads on the North Island and the South Island?

Rounded estimate: Actual:

9 The length of the paved roads in New Zealand is approximately how many kilometres less than those in the United Kingdom? 421 000 km, 401 000 km or 411 000 km?

10 What is the difference between the length of unpaved roads in New Zealand and the United Kingdom?

Rounded estimate:

Extended practice

Actual:

Amazing but fairly useless fact: If every single person from Australia came to live in New Zealand, there would still be more than twice as many people living in the United Kingdom than in New Zealand. Use the facts and figures about New Zealand in the table below to complete the tasks.

Some facts and figures about New Zealand

DRAFT

Approximate length and width of New Zealand (in miles) 1000 miles top to bottom 280 miles side to side

New Zealand population In the year 1955: 2 13 9 672

In the year 2024: 5.214M

Auckland population In the year 1955: 387 00 0

In the year 2024: 1 69 3 00 0

Christchurch population In the year 1955: 197 00 0 In the year 2024: 408 00 0

Wellington population In the year 1955: 132 65 4 In the year 2024: 424 00 0

Pet population In the year 2024: 4.6M

1 According to the data in the table, what proportion of the New Zealand population in 2024 lived in Auckland?

a As a rounded fraction:

b As a percentage to one decimal place: (Your teacher may ask you to use a calculator).

Show your working below.

DRAFT

c If you were writing the information about the proportion of people who live in Auckland for a newspaper report, would you use the fraction or the percentage figure? Give a reason for your answer.

2 Did you know that humans only make up around 5% of the population of the country? Which of these is the best estimate for the number of animals and humans that live in New Zealand?

95M, 99M, 104M

3 To the nearest ten thousand, by how many did the population of Christchurch increase between 1955 and 2024?

4 a Which city’s population had an increase of around 300% from 1955 to 2024?

b According to the data, what was the actual percentage increase (to one decimal place)?

5 Auckland had a large population increase between 1955 and 2024. A TV reporter would be unlikely to give the exact figures, but would round the information to make it easily understood by the viewers. Describe the increase as accurately as possible but, at the same time, giving the information in a user-friendly way.

6 The 1955 figure for the population was exact. However, the number for 2024 was rounded to the nearest hundred thousand. Why do you think it was shown as a rounded number?

DRAFT

7 The length and width of New Zealand is shown in kilometre. A kilometre is approximately 5 8 of a mile. Convert the information from miles to approximate kilometres and then (with your teacher’s permission) check the figures in a computer search engine.

Unit 2: Topic 2

Multiplication

We often think of multiplication as being connected with division. For example, 25 × 5 = 125 and 125 ÷ 25 = 5.

However, multiplication is also directly connected with addition. It is perhaps not surprising that, when they invented the multiplication symbol in the 1600s, they just rotated the addition symbol that had been invented in the 1400s. Maybe you have heard the saying: A good mathematician is a lazy mathematician? It doesn’t mean that people who are good at maths are lazy, it just means that they look for ways to save time.

Multiplication is, in fact, a number that is added together a lot of times. Instead of adding 19 + 19 + 19 + 19 + 19 + 19, we can save time by multiplying. We can call

Guided practice

1 Write the answers to these addition problems. Use a ‘lazy’ method (but not a calculator!).

a 23 + 23 + 23 + 23 + 23 + 23 + 23 + 23 =

b 150 + 150 + 150 + 150 + 150 + 150 =

c 95 + 95 + 95 + 95 + 95 + 95 =

d 347 + 347 + 347 + 347 + 347 + 347 + 347 + 347 + 347 =

DRAFT

In question 1, you might have thought of an even ‘lazier’ method than writing an algorithm to get the answers. For example, in 1b you might have thought something like 6 × 150 is the same as 3 × 300, so the answer is 900

2 Find the answers to these addition problems. Calculate mentally if you wish.

a 49 + 49 + 49 + 49 + 49 =

b 205 + 205 + 205 + 205 + 205 + 205 =

c 198 + 198 + 198 + 2 + 2 + 2 =

d 999 + 999 + 999 + 999 + 999 + 999 + 999 + 999 + 999 =

Independent practice

In multiplication there are “laws” that people have thought up. These “laws” are ideas that help people in their maths because they never change and are always true. In the same way that the law of gravity says that an apple goes downwards when it leaves the tree, there is a law in maths called the commutative law. This means that the answer to a multiplication doesn’t change if you move the numbers around. For example, 12 × 3 has the same answer as 3 × 12.

The associative law is similar and is useful if there is a list of numbers in the problem. Let’s look at 25 × 7 × 2. We could think of it as (25 × 7) × 2. We could also say 25 × (7 × 2) or even (25 × 2) × 7. Which do you think would save time?

1 Show how you find the answer to the following.

a 4 × 8 × 5 =

b 25 × 3 × 4 =

c 4 × 3 × 15 =

d 7 × 5 × 6 =

The distributive law tells us that you can ‘distribute’ (or split up) the multiplication to make it easier to solve.

DRAFT

For example, what is 7 × 13? If you know your 13 times table, you know the answer is 91. If you don’t, you can think of the problem like this:

2 Show how you find the answer to the following.

7 × 13 = 7 × 1 ten plus 7 × 3 ones or 7 × 13 = 7 × (10 + 3) = 70 + 21 = 91

a 5 x 17 = 5 x ( + ) = + =

b 24 x 7 = x ( + ) = + =

c 37 x 4 = x ( + ) = + =

d 8 x 35 = x ( + ) = + =

In contracted (short) multiplication, we write 19 × 6 like this, and we use the distributive law because we split 19 × 6 into 10 × 6 + 9 × 6: The distributive law is very useful when doing an extended (long) multiplication, such as 41 × 28. This becomes 41 × 2 tens + 41 × 8 ones. Before solving this, we need to practice the shortcut for multiplying by tens. Some people say that, to multiply by 10, you just add a zero. But this is not true. (Think of $2.35 × 10. It certainly does not equal $2.350!)

Reminder: when multiplying by 10, the digits move one column larger. So, for 23 × 10, the 2 tens move over to the hundreds and the 3 ones move to the tens. What about the space left by the 3? We put in a zero to fill the space.

tens become

hundreds

ones become 3 tens If there’s an empty space, the zero fills it.

3 In parts a and b below, what is the digit 5 worth after the calculations? In parts c and d, what is the digit 5 worth in each number?

a 59 × 10 b 546 × 10 c 3.5 d 5328

4 Try to calculate these mentally.

You can use the 10 trick to multiply by more than one ten. For example, 23 × 20 = 23 × 2 tens = 230 + 230 = 460.

a 18 × 20 =

b 13 × 30 =

DRAFT

c 15 × 50 =

d 25 × 40 =

5 Use the distributive law to show how you can split these into two multiplications.

Example: 24 × 28 = 24 × 20 + 24 × 8

a 23 × 19 =

b 37 × 26 =

c 82 × 25 =

d 53 × 26 =

Extended practice

Long multiplication gets its name because it spreads a long way down the page.

48 × 28 = 48 × 2 tens plus 48 × 8 ones

This is how we set out 48 × 28 as a long multiplication.

+ Add the two products.

1 Use the long multiplication method for the following.

× 20

The zero fills the empty space because we are multiplying by 2 tens

DRAFT

2 Anahera is saving for a new game. She saves $15 a week for 13 weeks. Does she have enough for the $200 game?

Show how you get the answer.

Working-out space

3 a Write the number of years and months since you were born.

b How many months old are you?

c Counting a year as 365 days, how many days old are you?

4 The Po – hutu Geyser erupts around 17 times a day. Based on that average, how many times does it erupt in:

a August?

b June and July?

c a leap year?

5 Did you know that in a worldwide survey in 2024, New Zealand topped the list of the most ice cream eaten per person per year? The amount is averaged out at 28.4 litres per New Zealander. How much ice cream is that for:

a a group of 15 people?

DRAFT

b your class?

Unit 2: Topic 3

Division

The vocabulary of division

When you were younger, you probably called division ‘sharing’ and wrote number sentences such as 25 shared by 4 = 6 remainder 1, or 25 ÷ 4 = 6 r 1.

Here are four words that are used to describe division:

The dividend is the starting number – the one that is going to be divided. The divisor is the number that the dividend will be divided by.

The quotient is the result of the division.

The remainder is what is left after the dividend has been split up. (Not all divisions end up with a remainder.)

÷ 4 = 6 r 1

Guided practice

1 There is one digit missing from each dividend. Fill in the gaps.

2 There is one digit missing from each quotient. Fill in the gaps.

3 The divisor is missing from these problems. Fill in the gaps.

4 The remainder is missing from these problems. Fill in the gaps.

5 If $22 is shared between 4 people, which division word describes the $22?

Independent practice

Using remainders sensibly

In the real world, people have to decide what to do if there is a remainder. If two people were sharing three small pizzas, they almost certainly wouldn’t leave the remainder as 3 ÷ 2 = 1 r 1. They would share it and say, “We get 1 1 2 each”. It would be different if $3, or three tennis balls, had to be shared between them. They would need to decide what to do.

If the quotient has a remainder, we can use fractions or decimals or just leave the remainder. The pizza remainder would become a fraction, the $3 would be divided using decimals, but the tennis ball would have to stay as a remainder.

DRAFT

1 In these division algorithms, there is the same dividend, the same divisor and the same quotient, but the remainder has been used differently. Match a division with each situation.

22 marbles and 5 people

22 apples and 5 people

$22 and 5 people

2 Re-write using the symbol. Show the remainders as fractions. Simplify the fractions where possible.

a 64 234 ÷ 7 = b 39 266 ÷ 4 =

c 43 484 ÷ 6 = d 59 340 ÷ 9 =

3 Re-write using the symbol. Use decimals for the remainder. Round the answer to the nearest one hundredth when necessary.

a 28 537 ÷ 4 = b 74 354 ÷ 6 =

c 75 389 ÷ 8 =

e 147 534 ÷ 9 = d 58 341 ÷ 7 = f 275 369 ÷ 5 =

DRAFT

Long division

Long division looks complicated but all you need is a little patience. There are five steps for long division. This shows the five steps using a 1-digit divisor. Some people make up a little saying to remember the steps.

Don’t Make Sandwiches, Bring Rhubarb

Steps for long division

1: Divide

2: Multiply

3: Subtract

4: Bring down

5: Repeat (or remainder)

It works in the same way with a 2-digit divisor.

Don’t Make Sandwiches, Bring Rhubarb

Steps for long division

1: Divide

2: Multiply

3: Subtract

4: Bring down

5: Repeat (or remainder)

4

Use the D.M.S.B.R. method for the following. Use ‘r’ for the remainder if necessary.

g 4935 ÷ 21 = h 5870 ÷ 18 = i 9215 ÷ 14 =

Extended practice

Estimation and rounding are an important part of long division, especially when large numbers are involved. For example: How many 19s are in 83? My estimate is 4 because 20 × 4 = 80. Check by multiplying: 19 × 4 = 76. So, we were close: 83 ÷ 19 = 4 r 7. How many 295s are there in 1775? My estimate is 6 because 6 × 300 = 1800. Check by multiplying: 295 × 6 = 1770. So, we were close: 1775 ÷ 295 = 6 r 5.

1 Estimate the quotient to the nearest whole number. Then write a division algorithm to find out how close you were.

a 372 ÷ 31 = Estimate: b 624 ÷ 24 = Estimate

DRAFT

c 532 ÷ 19 = Estimate:

Use this information about flightless birds to complete the activities. Don’t forget to use rounding and estimation to help you.

2 True or false? An ostrich is more than twice as heavy as an emu.

3 True or false? It would take 20 kiwis to balance one emu?

4 To the nearest whole number, how many kiwis would it take to balance one ostrich?

5 How many kiwi heights are the same as the height of an emu. Write the remainder as a decimal to two decimal places.

DRAFT

6 How many kiwi heights are the same as the height of an ostrich. Write the remainder as a decimal to two decimal places.

7 How many times heavier is each bird than its egg? Write your answer to the nearest whole number.

a kiwi

b emu

c ostrich

Kiwi

Emu

Ostrich

Unit 2: Topic 4 Order of operations (GEMA)

The order of operations

If you want your calculator to divide 125 by 5 and the answer shown is 625, there is only one person to blame. A calculator always does what it is asked to do. So, if you press × instead of ÷, it won’t argue with you and ask you if you’re sure that’s what you want. It will multiply 125 × 5.

A calculator ALWAYS does what it has been told to do. However, what if the calculator has been programmed to calculate in a different way than you want it to work?

If you press 2000 + 12 × 2 =, the calculator might begin with 2000 + 12 and then multiply by 2 which equals 4024.

A different calculator might multiply 12 × 2 and then add 2000 which equals 2024.

It all depends on the way the calculator has been programmed. The order in which we (and calculators) should work is called the order of operations, which we call GEMA. Here’s how it works:

1st G Grouping

2nd E Exponents

3rd M Multiply and Divide

4th A Add and Subtract

Anything that is grouped together in a bracket is calculated first.

2 × (3 – 1) = 2 × 2 = 4

Next comes exponents (and/or square roots).

4 x 32 = 4 × 9 = 36

√ 64 + 4 = 8 + 4 = 12

Then work from left to right doing division and/or multiplication.

10 + 6 ÷ 2 = 10 + 3 = 13

2 × 3 + 2 = 6 + 2 = 8

10 ÷ 2 – 3 + 2 × 6 = 5 – 3 + 12 = 14

Finally work from left to right doing addition and/or subtraction.

4 + 2 × 3 = 4 + 6 = 10

5 × 4 – 3 = 20 – 3 = 17

25 – 10 + 3 = 18

Guided practice

1 Test out your calculator with some of the operations on this page to check whether it is following GEMA. If possible, try the same with a different calculator. How well did the calculator(s) function?

Independent practice

1 Use the rules of GEMA to solve the following problems without a calculator. Remember to work from left to right.

a 6 + 21 ÷ 3 = b 3 × 4 – 2 =

c 45 – 9 × 4 = d (3 + 13) ÷ 4 =

e 8 + 3 × 15 = f 42 × 2 =

g √ 64 ÷ 2 = h 3 × (15 – 3) =

i 72 − 32 = j (21 ÷ 3)2 =

k 16 – (8 ÷ 2) × 3 + 3 =

l 14 + √ 81 – 7 =

m (2 + 8)2 – 72 = n 3 + (18 ÷ 2) – 32 =

o 18 ÷ 3 + 7 × 6 = p 6 × 5 – 4 × 3 ÷ 2 =

Equations are number sentences in which there are two sides that balance each other. For example, 2 + 3 = 4 + 1.

2 Using GEMA, decide whether the following expressions balance. If they balance, write them like this:

2 × 5 + 3 = 3 + 2 × 5

a 24 ÷ 4 + 2 and 24 ÷ (4 + 2)

DRAFT

b 2 × 42 and 42 × 2

c 3 × 6 − 3 and 15 + 2 × 5

d 5 × (4 + 1) and 52

If they do not balance, write them like this: 5 + 3 × 2 (5 + 3) × 2 ≠

3

e √ 9 × 32 and 3 3 =

f 18 ÷ 2 + 42 and 18 ÷ (2 + 42) = ≠

g 7 + 3 × 2 and (7 + 3) × 2

h (√ 25 + 3) × 2 and √ 25 + 3 × 2

Not only do you need to take note of the brackets, you also need to work from left to right. If there is a division and then a multiplication, the division comes first. For example:

Expression:

Step 1: Grouping (brackets)

12 ÷ (8 – 2) + 3 × 5

12 ÷ 6 + 3 × 5

Step 2: × and ÷ working from left to right (so divide first) 2 + 3 × 5

Step 3: Multiply before adding 2 + 15

Step 4: Add 17

DRAFT

Evaluate the following. Use the example above to show the steps you use to find the answer.

If there are brackets inside other brackets, evaluate the inside brackets first. For example:

Expression:

Step 1: Grouping (inside brackets first)

Step 2: Grouping (division first)

5 × (16 ÷ [32 – 5] − 2)

5 × (16 ÷ 4 − 2)

5 × (4 -2)

Step 3: Grouping 5 × 2

Step 4: Multiply 10

4 Evaluate the following. Use the example above to show the steps you use to find the answer.

Extended practice

1 Write an expression that would solve this problem. Remember to use GEMA.

Kai has $40 of birthday money to spend.

DRAFT

She meets her Aunty at the gate who says, “I will give you a quarter of what you have as an extra gift.” Kai is so excited she doesn’t notice that her Aunty gives her $2 less than she had promised. Kai buys 3 pens at $3 each and then counts her money. How much does she still have left? Show the steps you use to solve the problem.

2 Jack was going shopping. He was planning how much money he would need. He wanted three books at $12 each, a pair of jeans at $40 and four paint brushes at $1.50 each.

a Write an expression to show how much he would need.

3

b When he actually went shopping, he found that the jeans were discounted by 10%. However, there was a 10% increase on the price of each paint brush. How much did Jack end up saving? Show the steps to find the amount he actually spent and calculate the amount he saved.

DRAFT

A building company has put aside $4000 in order to buy three mobile phones for its workers. When the invoice arrives, it shows that the phones are $1295 each. In addition, if the invoice is paid within 7 days, there is a 10% discount on the total price. How much is left out of the $4000 if they pay the invoice within 7 days? Write an expression and show the steps to solve it.

4 A shop is selling computer games for $90. Wiremu and his brothers, Tamati and Niko, want to buy three between them. When they arrive at the store there is a special offer of a 25% discount. They decide to buy 6 games. How much do they each pay?

Write a numerical expression to represent this situation and then find the price paid by each brother.

Unit 2: Topic 5 Integers

Integers

All whole numbers are integers. They can be positive numbers (I have 8 pairs of shoes) or negative numbers (the temperature at the Coronet Peak was −8ºC.) In maths, a number with a minus sign in front of it, such as –8, is known as negative 8. Positive numbers don’t need a symbol in front of them, which is perhaps just as well or shops would need to have signs saying things like +$10! The only number that is neither negative nor positive is zero. Integers can be shown on a number line:

–5–4–3–2–1012345

Guided practice

1 a Write the missing numbers on this number line. –4–3–10 35

b What is the “mystery” integer? We can call it x x < 1 but > −1 x =

2 Plot these letters on the number line S: 4, I: 2, I: −2, K: −4, W: 0

–5–4–3–2–1012345

3 Use the number line to answer the questions.

a Which two numbers are 5 places from zero?

b Which number is 2 places less than zero?

c Which number is 2 places to the left of positive 1?

d Which number is 2 places to the right of positive 1?

e Which number is 5 places to the left of negative 1?

f Which number is 8 places to the right of negative 10?

4 Which numbers are missing from this number line?

Independent practice

Comparing positive and negative integers

1 Plot these numbers on the number line with a dot.

–6 –7 –8 –9 –10

a −5

b 7

c −3

2 Using the number line in question 1, write the correct symbol (> or <) between these numbers.

a −5 7

b −3 −5

c −10 −7

d 1 −3

DRAFT

3 It is possible to plot fractions and decimals on a number line. For example, the red dot shows the position of negative 1 2 . The blue dot shows negative 1 1 4

0 –1 –2 –3 –5 4 –1 2

a Work out the position of negative two and a third. Plot it on the number line with a dot and label it as an improper fraction.

b Work out the position of 1.75. Plot it and label it on the number line.

4

a Plot these points on the vertical number line.

2, −5, 3, 0, −2, 4

b Write the numbers in ascending order.

c If this were a scale on a thermometer in °C, the coldest temperature would be

5 Use the number line and the symbols > or < to make these expressions true.

a 5 3

b −4.5 −3

DRAFT

6 If the temperature is −2ºC and the air warms up by 5º, then the new temperature is

7 Nikau visits the Ocean Swim Centre. The centre is at sea level. Fill the gaps in the sentences below with the appropriate number of metres and the word ”positive” or “negative” before the number indicating height or depth.

High board: 4 m

Ocean Swim Centre

Low board: 2 m

Depth of sea: 8 m

a When Nikau is on the low board, she is at m.

b When she is on the high board, she is at

c When she dives two metres under the water, she is at m.

d When she is at the water’s edge, she is at m.

e If she dropped a coin into the sea it would be at m.

8 Imagine the water at the Ocean Swim Centre is 14ºC. Nikau previously visited the swim centre in January and the air temperature was 21ºC.

DRAFT

a Would the water feel warmer or colder than it was in January when she dived in?

b How many degrees warmer or colder is the water? If she dived into the water in July and the air temperature was −2ºC …

c Would the water feel warmer or colder when she dived in?

d How many degrees warmer or colder is the water?

9 Use the number line to complete these problems. For example, −3 + 2 = −1. –5–4 –6 –7

a −3 + 1 =

b −4 – 1 =

c −2 + 3 =

d −7 – 2 =

e 2 – 5 =

f −8 + 7 =

Extended practice

The extremes of recorded temperatures from five countries are shown in this table.

1 Use the data in the table to complete these tasks.

a Which country has the lowest difference between low and high temperatures, and what is the difference?

b Which country has the highest difference between low and high temperatures, and what is the difference?

DRAFT

c Which country has a temperature difference of 82º?

d What is the difference between the highest and lowest temperatures recorded in New Zealand?

e If next year’s low temperature for the UK is 3.9º lower, what would the new low temperature be?

2 This is part of a statement for Bob the Baker’s bank account. Fill in the gaps to show what happens to the balance of the account.

June 1

June 10

3 In question 7 of the Independent practice section, you worked with heights above sea level and depths below sea level. The altitude of the place where you live is measured in metres above sea level. (There are not many places on land that are below sea level.) The highest point on Earth is at the top of Mount Everest, which is at an altitude of 8848 metres. However, it is not the tallest mountain.

The bottom of the tallest mountain is actually below the ocean. Your challenge is to try and find out some facts about this giant. You could start by asking questions such as: Where is the mountain? How tall is it? What is its name? How much taller than Mount Everest is it? How much of the mountain is below the ocean?

If you find this challenge easy, you could do some research into other high and low record breakers, such as:

What is the highest and lowest temperatures recorded where you live?

Where in New Zealand was the lowest recorded temperature recorded and when did this happen?

DRAFT

Unit 3: Topic 1

Identify fractions, decimals and percentages

Part of any whole thing is a fractional part. The same fractional part can be shown as a decimal (0.5), fraction ( 1 2 ) or as a percentage (50%). Certain situations use different ways to show fractions. In a race, for example, we would be unlikely to hear that the winner had finished in 10 seconds and 50% of a second.

Guided practice

1 Each of these shows the same fractional part. Choose the most appropriate way to represent them. a

2 Fill in the gaps so that the values for each row are the same.

1 2

3 10

h 1 1000

3 Write the following in ascending order.

a 2.5, 2 1 0 , 0.25, 21%

b 30%, 0.3, 3%, 1 3

DRAFT

c 0.12, 12.5%, 12 1000 , 1.2

Independent practice

The following three fractional parts all represent the same value.

DRAFT

1 Represent the following as fractions, decimals and percentages. Write the value under each diagram.

Plotting fractions or decimals on a number line is sometimes hard to do accurately. For example, although 0.25 comes halfway between 0.2 and 0.3, a value such as 0.28 would not be so easy.

2 Estimate the positions of the following decimals on these number lines by drawing an arrow to the correct place.

3

DRAFT

Estimate the positions of the following fractions on these number lines by drawing an arrow to the correct place. Use a ruler to help you.

Extended practice

Coaches of sporting teams sometimes ask for something that is technically impossible. If 100% means the whole thing , is it possible to give 110% effort?

Of course, it depends on the context. Is it possible for there to be more than a fraction of a pizza left over when friends get together? Yes!

With fractions, if we want to represent a number greater than one whole, we use mixed numbers, or improper fractions.

Show the following amounts as improper fractions.

DRAFT

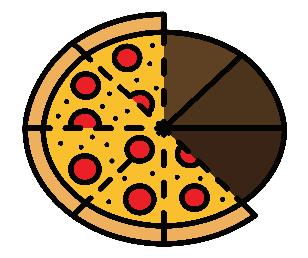
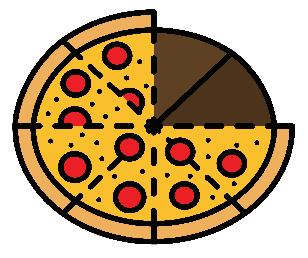
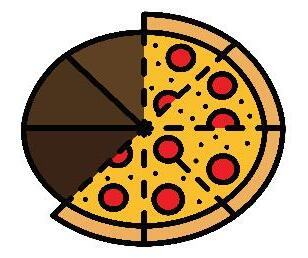
1 The amount left from this pizza party is 9 8 (improper fraction) or 1 1 8 (mixed number).

2

Show the following amounts as mixed numbers.

3

Follow the instructions to mark the following.

a On this number line, 0 and 1 3 have been marked. Mark the position of 1.

Explain how you found the position of 1.

01 3

b On this number line, 0 and 7 4 have been marked. Mark the position of 3 4 .

Explain how you found the position of 3 4

c On this number line, 0 and 0.45 have been marked. Mark the position of 1.5.

Explain how you found the position of 1.5.

DRAFT

0 0.45

d The progress bar shows that 35% of the program has been loaded. Mark the position of 80%.

Explain how you found the position of 80%.

When we look at fractions, we see that the smaller the fraction, the larger the denominator. This happens because the denominator tells us how many equal parts the whole has been split into. So, in 1 2 the denominator is a low number because the whole has only been split into two parts. Whereas 1 100 means that the fractional parts are a lot smaller. The fraction wall below shows one whole split into various fractions.

Guided practice

1 Label one fractional part of each row on this fraction wall.

DRAFT

2 Find fractions that are

One whole

3

Revise the equivalence of basic fractions, decimals and percentages by completing this table.

Independent practice

Simplifying fractions

1

Many fractions are equivalent to other fractions. 9 12 is equivalent to 6 8 and 3 4 However, we say that 9 12 , in its simplest form, is 3 4 That’s because there is no simpler fraction that is equivalent to it.

DRAFT

2 Some fractions have no equivalence because they cannot be simplified further. Circle the fractions that are already in their simplest form. 8 12 , 2 11 , 3 7 , 2 10 , 4 7 , 9 12 , 5 11

Simplifying a fraction is a simple process. We just need a knowledge of factors. We find the common factors of both the numerator and the denominator. The common factor of 5 and 25 is 5.

Next we divide the numerator and the denominator by the common factor. So, 20 25 in its simplest form is 4 5

3 Divide the numerator and denominator by the common factor to simplify these fractions.

4 If there is more than one common factor, divide by the highest common factor (HCF).

18 24 18 24 3 4 1, 2, 3, 6, 9 1, 2, 3, 4, 6, 12, 24 ÷ 6 ÷ 6 =

Find the highest common factor and then simplify.

DRAFT

5 With large numbers, instead of writing all the factors, we can use repeated division. To simplify 72 96 , we can see that they are both even numbers, so begin by dividing by 2. We repeat until the numbers can no longer be divided by 2. Do the numerator and denominator still have a common factor? Yes: 3! So, we divide by 3. If we simplify 72 96 , the answer is 3 4 Use the repeated division method to simplify the following.

They’re both even numbers so, we can divide by 2

Now we divide by the HCF (3)

Converting from a decimal to a fraction

If somebody younger didn’t know that 3 4 = 0.75, how could you explain it? When we see a fraction such as 3 4 , we can imagine a division symbol. We can see the fraction as 3 ÷ 4.

÷ 3 4

If we divide 3 by 4 the answer will not be a whole number.

4 3 3 0 2 0 0 7 5

6 Convert these fractions to decimals by dividing the numerator by the denominator. Round to 3 decimal places if necessary.

DRAFT

You may have noticed that in question 6d, the 3 was repeating in the decimal columns. We call this a recurring decimal. (Recurring means it occurs again and again.) Once you find that a digit recurs in a decimal, place a dot (or a line) over the digit. For example, in question 6d, 1 3 = 0.33 or 0. 3. If 2 digits repeat, as in question 6h, put a line over both digits: 5 11 = 0. 45

7 Write the following as decimals. Use a recurring symbol if necessary.

Extended practice

1 Find the mystery fraction.

The denominator has exactly four factors. The numerator is the square of an odd number. The difference between the denominator and the numerator is 1. What is the mystery fraction?

DRAFT

2 You will need two calcuators for this task: a basic calculator (usually capable of displaying up to 9 digits) and, if possible, an advanced calculator capable of displaying 16 or more digits.

a Enter the calculation for 3 7 on the basic calculator. Write down the decimal equivalence, according to the calculator.

b Enter the calculation for 3 7 on the advanced calculator. Write down the decimal equivalence according to the calculator.

c What pattern of repeated digits you notice in the second answer?

3 Prove that the 6-digit repeating pattern in question 2c is a recurring one by calculating to 18 decimal places.

4 If a string of digits recurs, as in question 3, we place a dot over the first and last digits of the recurring sequence. For example, 1 7 as a decimal is 0.142857142857

Show the recurring decimal for the following fraction conversions.

a 3 11

b 2 7

5 Find two or three more examples of sequences of digits that recur when a fraction is converted to a decimal.

6 Sensible conversion of fractions to decimals.

It can be interesting to look at recurring digits when fractions, such as 4 7 , are converted to decimals. However, in the real world we seldom need so many decimal places.

DRAFT

a As a rounded decimal, how do you think 1 7 of a 4-metre plank would be measured?

b Imagine you had travelled 1 7 of a 400-kilometre journey, how would you round the distance you had travelled? Explain your rounding.

7 Is there a situation in which you think it is necessary to round to more than three decimal places?

Unit 3: Topic 3

Multiplication of fractions and decimals. Finding percentages

Multiply decimals is like multiplying whole numbers, but we mustn’t forget where the decimal point goes. 147 × 3 = ?

When multiplying with decimals, it is very useful to estimate so that you already know the approximate answer. 14.7 × 3 = ?

(14.7 rounds to 15. So, the answer should be close to 15 × 3 = 45.)

Guided practice

1 Complete the following. Estimate the answer to the decimal multiplication before completing it.

Multiplying fractions by whole numbers

Think about 1 3 × 4. It means we have 4 of 1 3 1 3 + 1 3 + 1 3 + 1 3 = 4 3

DRAFT

What about 2 3 × 4?

2 3 + 2 3 +

Now can you see patterns? The denominator is not changing. A numerator is multiplied by a whole number. 2 3 ×

2 Shade the diagram to show 3 8 × 4. Then write the multiplication in the box. Simplify the improper fraction.

3 8 × 4

3

Finding the percentage of a number

Finding basic percentages of a number, such as $40, is quite easy because for 50% we can say 1 2 of $40, for 25% we can say 1 4 and 10% is 1 10 of $40.

So, for 50% of something we can recognise 50% as a half and divide the amount by 2.

Find the answers.

a 50% of 224

DRAFT

b 25% of $36

c 10% of $25 d 25% of 72

e 50% of $8.50 f 10% of $3

Independent practice

1 Remembering to put the decimal point in the correct place, write multiplications to solve the following. a 1.95 × 7 b 26.9 × 4 c 64.6 × 3

178.35 × 5

40.87 × 7

2.468 × 8 g 3.307 × 9

× 4

DRAFT

When multiplying decimals by a 2-digit number, it’s like doing a long multiplication. Here’s a reminder of a way to multiply by 2 digits. 4 83 8 2 8 4

69 0 4314 × Add the two products. +

48 × 8

48 × 20

The zero fills the empty space because we are multiplying by 2 tens

However, if the number to be multiplied was 4.8 instead of 48, it can be confusing to know which column is which.

4. 8 × 2 8

This is a way to overcome the problem. Suppose we want to multiply 2.95 by 15. We start off be rounding and estimating. We know what the approximate answer will be: 2.95 × 15 can be rounded to 3 × 15 = 45. So, if the answer is not close to 45, something has gone wrong!

• Step 1: Round and estimate (very important!).

• Step 2: Count the number of decimal places: it’s 2.

• Step 3: Ignore the decimal point and complete an ordinary multiplication.

• Step 4: Put in decimal point so that there are the same number of decimal places as at the beginning.

• Step 5: CHECK! Is the answer close to the estimate? Yes! (Phew!)

DRAFT

2 Follow the same process to complete the following. Remember to follow the five steps above.

3

d 1.93 × 46

Estimate: e 3.02 × 55

Estimate: f 1.125 × 18

Estimate:

Multiply to find the answers to the following. Write the answer as an improper fraction and then as a mixed number. Simplify the fractions where possible.

a 5 8 × 3 b 5 4 × 5

c 3 8 × 7

7 3 × 4 e 7 4 × 6

DRAFT

In maths, when finding a fraction or a percentage, the word “of” can be replaced by the multiplication symbol. This is how it works:

50

100 × 40 1 = 2000 100 = 20

50% of $40 is × 40 = 20.

To find a percentage such as 5% of 25, we can use the commutative law (moving numbers around) so that it doesn’t affect the value.

It works like this:

50 100 × 25 =

4 Find:

a 5% of 30.

b 45% of 125.

c 15% of 70.

DRAFT

Extended practice

To multiply a decimal by powers of ten is just a matter of remembering the “ten trick”.

1 Taking note of the number of zeros for each question, mentally multiply the following.

Multiplying decimals by decimals is similar to the long multiplication of decimals in question 2 of the Independent practice section. You still follow the same five steps, but this time, you count the total number of decimal places in order to put the decimal point back in the correct place. If you look back, you will see that when we multiplied 2.95 × 15. We did a multiplication of 295 × 15 and then put the decimal point back into the answer.

Imagine the problem is 2.95 × 1.5. There are three decimal places, so we count three decimal places in the answer.

2 Follow the same process to complete the following. Remember to follow the five steps.

DRAFT

Unit 3: Topic 4

Addition and subtraction of fractions

Adding 4 9 + 1 9 is as simple as saying 4 + 1 = 5. So, 4 9 +

9 =

9 . We can add them because they are “like” fractions. To be alike they must have the same denominator. But what about 4 9 + 1 3 ? They don’t have the same denominator. Adding them would be like trying to convince people that adding four bananas and one apple makes 5 banapples. 4 9 and 1 3 are not alike. However, we can use our knowledge of equivalent fractions to give them the same denominator.

1 3 is equivalent to 3 9 , so we can change the addition to 4 9 + 3 9 = 7 9 .

Guided practice

1 Use your knowledge of equivalent fractions to add the following. Shade the diagram and fill in the gaps.

We can use a similar process to subtract one fraction from another. This diagram shows that 5 6 –1 3 is equivalent to

2 Use the same process to complete this subtraction.

3 Use your knowledge of equivalent fractions to complete the problems. Draw diagrams to help if you wish.

Independent practice

1 Add these fractions. Write the answer as an improper fraction. Then write the answer as a mixed number. Simplify answers if necessary.

For example, 7 10 + 4 5 = 7 10 + 8 10 = 15 10 = 1 5 10 = 1 1 2

a 7 9 + 1 3 =

b 7 8 + 7 8 =

c 9 5 + 7 10 =

d 9 6 + 2 3 =

e 8 6 + 2 3 =

f 4 8 + 7 4 =

2

Change the mixed numbers to improper fractions and then subtract using your knowledge of equivalence. Finally, change the answer back to a mixed number, simplifying fractions where necessary.

For example, what is 1 1 3 –1 6 ?

1 2 3 –1 6 = 5 3 –1 6 = 10 6 –1 6 = 9 6 = 1 3 6 = 1 1 2

DRAFT

a 1 3 4 –3 8 =

2 1 4 –1 2 = c 2 7 10 – 1 1 5 = d 3 1 4 – 1 1 2 =

DRAFT

3 Solve the following addition and subtraction problems. Simplify fractions where necessary.

a 2 25 + 1 5 = b 7 18 –1 9 = c 24 20 –4 5 = d 9 2 + 1 3 4 = e 1 3 10 + 7 5 =

What if one fraction does not have a direct equivalence with the other? For example, what is 1 4 + 1 5 ? We need to find the lowest common multiple (LCM) of each fraction. Here’s how it works: Find the lowest common multiple of each denominator.

4: 4, 8, 12, 16, 20, 24

5: 5, 10, 15, 20, 25

The LCM is 20, so we convert both fractions to twentieths.

So, 1 4 + 1 5 = 5 20 + 4 20 = 9 20 .

4 Use the same method to complete the following. Convert improper fractions to mixed numbers where necessary. a 1 6 + 1 9 =

The LCM is b 5 6 + 1 4 = The LCM is

DRAFT

c 1 1 5 –2 3 =

The LCM is

d 5 8 − 1 4 =

The LCM is

e 4 8 + 1 3 12 =

The LCM is f 1 5 9 –1 2 =

DRAFT

The LCM is

Extended practice

1 A framing factory puts people’s photos into frames. They allow 2 1 2 minutes to select the frame timber, 3 1 3 minutes for cutting and 2 1 4 minutes for mounting the photo. How long do they allow for each photo to be finished:

a in whole minutes and a fraction of a minute?

b in minutes and seconds?

2 Some friends get together for a pizza party.

Wiremu eats 2 3 of a pizza. Maia eats 3 4 of a pizza.

Ari eats 5 8 . Tamati has 1 1 2 pizzas and Anahera has 5 6 .

a How much do they eat altogether?

b How many full pizzas do they need to order?

DRAFT

c How much is left to take home?

3

There were 17 100 people at a rugby game. 1 9 of them were supporters of the away team. Of the home team, 2 3 were adults, 1 5 were juveniles and the rest were ground staff.

a How many away supporters were there?

b How many adult supporters of the home team were there?

c How many juveniles were there?

d What fraction of the people were ground staff?

DRAFT

e How many ground staff were there?

4

A marathon race is just over 42 km. In the Mighty Manapanau Marathon, a ninth (104 people) of the runners gave up after 20 minutes. The same number gave up after half an hour. 3 18 got a cramp and had to stop. 2 9 walked the last few kilometres and one third finished the race. 1 18 took a wrong turn and ended up at the beach.

a How many people started the race?

b How many people finished the race?

c What fraction of the runners gave up?

DRAFT

d A quarter of the beach-goers had a swim. How many is that?

Unit 3: Topic 5

Proportional reasoning

Ratios are used to compare numbers or quantities to each other. There are 6 smiley faces and 4 sad faces.

We can say that the ratio of smiley faces to sad faces is 6 to 4. The ratio is written as 6:4

Guided practice

1 Write the ratio of smiley faces to sad faces by counting.

2

In the example at the top of the page the ratio was written as 6:4. The ratio can be simplified.

In its simplest form the ratio is 3:2, because there are 3 smiley faces for every 2 sad faces.

Write the ratio of smiley faces to sad faces in its simplest form

Ratio in its simplest form 3:2

Simplifying ratios is as simple as simplifying fractions

Independent practice

In a pack of jellybeans imagine that the ratio of white ones to black ones is 2:3.

If we know how many white ones there are, we can use the ratio to work out the number of black ones.

Imagine there are 8 white ones. 8 is four times bigger than 2, so to find the number of black ones, we multiply 3 by four. 2 × 4 = 8 and 3 × 4 = 12.

The pack has 8 white jellybeans and 12 black jellybeans.

Drawing a diagram can help.

1 Use the same ratio of white jellybeans to black jellybeans (2:3) to work out the number of black jellybeans in each pack if there are: a 6 white ones b 10 white ones c 16 white ones

2 Use the same ratio of white jellybeans to black jellybeans (2:3) to work out the number of white jellybeans in each pack if there are: a 9 black ones b 15 black ones c 30 black ones

Ratios can compare more than two numbers.

In this pattern there are three colours. For every blue square there are 2 yellow squares and 3 green squares. The ratio is 1:2:3.

DRAFT

3 What is the ratio of blue to yellow to green squares in these patterns?

a The ratio is

b The ratio is

c The ratio is

4 Colour this so that the ratio is 3 blue squares to 1 yellow square to 2 green squares. (3:1:2).

5 Look at this this bead pattern.

Describe the way that the bead pattern is made:

a in words b as a ratio

6 Choose a ratio to colour this 24-bead pattern using red, green and blue

Describe the way that the bead pattern is made:

a in words b as a ratio

7 To make 8 pancakes Jo uses 120 g Ratios can be shown in a table. Fill in of flour, 250 mL of milk and 1 egg. the gaps in this table.

DRAFT

8 Sam has 18 sheep, 48 goats, 6 horses and 12 cows.

a Write the ratio of sheep, to goats, to horses, to cows in its simplest form.

b Jo has the same types of animals as Sam and they are in the same ratio. However, Jo only has 4 cows. How many of each of the other animals does Jo have?

Extended practice

1 Proportion is different to ratio.

The ratio of sad faces to smiley faces here is 1:3

Proportion compares one number to the whole object or group.

To find the proportion of sad faces we look at the total number of faces (8).

Next we look at the number of the faces that are sad (2)

The fraction of the group that has sad faces is 2/8, which can be simplified to ¼.

So, the proportion of sad faces is ¼.

The proportion can also be written as a percentage (25%) or a decimal (0.25).

Write the proportion of smiley faces as:

a a fraction b a percentage c a decimal

2 The ratio of oranges to apples in a box of twenty pieces of fruit is 1:4.

DRAFT

We can use the ratio and proportion to work out the number of oranges and the number of apples:

Add 1 orange and 4 apples: 1 + 4 = 5 (( There are 5 “portions”)

What proportion are oranges? one fifth

What proportion are apples? four fifths

1/5 of 20 is 4, so there are 4 oranges

4/5 of 20 is 4 lots of 4, so there are 16 apples.

How many oranges and how many apples are in each box if the total number is: a 10? oranges b 25? oranges apples apples c 50? oranges d 35? oranges apples apples

3 Use the information to work out the numbers of oranges and apples in each box. a b c d

Financial planning

It was the week of Maia and Ari’s holiday. Their mum said that they needed to learn about budgets. She gave them each $25 for the whole day and an expense sheet to see how they went until lunch time.

Guided practice

1 a Fill in the blanks on Ari’s expense sheet. Ari’s Expense sheet

Maia

me

b Ari had a negative balance. Explain why.

c Maia lent her brother the money. Her balance was $11.50. What do you estimate would be her closing balance?

2 Budgeting looks at two main things: income and expenditure. Income is the money that someone has, or expects to get. Expenditure is what they want (or need) to spend it on.

Imagine that you could have helped Ari prepare a budget for the morning. How might the budget have looked so that he had money left for the afternoon? Include up to five expenses.

3 Ari was in debt by lunchtime. What do you understand by “being in debt”?

Independent practice

Budgeting in the real world

Some people have a weekly budget. This can help prevent debt. A balanced budget is one in which the expenditure is the same (or less than) the income. On the previous page, Ari’s budget for the day was not balanced because he spent more money than he was supposed to do.

DRAFT

1 Harper is 19 years old. She lives with her family and gives them money each month for food, electricity and other expenses. She has a daytime job and also does babysitting in the evening. This is her budget for a month.

Harper’s monthly budget

a Fill in the totals for Harper’s monthly income and expenditure.

b Is Harper’s budget balanced? Explain why.

Saving and investing

This is when people put some of their money aside to use later, maybe for a holiday or for an unexpected expense. This could be in a savings jar or in something like a bank.

2 Harper has $100 left over at the end of each month. She decides to put a quarter of it in her savings jar and the rest in a savings account in a bank.

a How much is in her savings jar after 6 months?

b How much is in her savings account after 12 months?

Bank interest

If people put money into a savings account, a bank will usually give interest on the amount. This is a percentage of the amount that the saver has put into the account on top of the amount paid in. So, if Harper leaves her money in the bank, she ends up with more than she started with. The way that interest is calculated is complicated and changes from bank to bank and from time to time. 5% per annum (p.a) is typical. Per annum is Latin for per year

DRAFT

3

Imagine that the amount of interest Harper’s bank gives is 5% p.a. If she leaves $900 in the account for a year, how much is her savings account worth?

4 If Harper leaves the whole amount of her money in the account after 12 months, how much is it worth after:

a the second year?

b the third year?

Everyday bank accounts

Many people have what is called a current account at a bank. This is one that they pay money into regularly and use it for everyday expenses. Customers usually receive an online statement each month.

Use the information to complete the tasks.

H. Shamui

June

5 Fill in the blue cells in Ms H. Shamui’s bank statement.

6 What was the total of direct debits for the month?

7 What was the total of debit card payments and ATM withdrawals for the month?

8 a The standing order goes into a savings account each month. How much will Ms H. Shamui have paid into the account after one year?

b After 12 months, she cancels the standing order. If she leaves what she has paid in for one more year, how much will there be in the account 12 months later?

DRAFT

When somebody owes money, they are in debt (as was Ari at the beginning of this topic). There are two types of debt. Good debt

An example of good debt is when someone owes money and they know they can pay it back. They arrange to do that when they take out the loan. An example of good debt is someone who takes out a car loan or a mortgage for a house.

Bad debt

Bad debt is when someone uses someone else’s money to pay for something without knowing whether they can pay it back.

Debit cards and credit cards

On the last page you saw how Ms H. Shamui sometimes paid using debit card When a debit card is used, the money automatically goes from her current account at the bank to the payee (the person being paid).

A credit card looks very similar, but there is one big difference: the person using it doesn’t pay straight away. The credit card company gives the payee the money and the card owner owes the credit card company the money within a month.

1 Imagine Reysha pays $200 for some ear pods with her credit card. If she pays the money back on time, she is free from debt. Credit card companies do not usually charge any interest if the amount owing is paid within a month.

So how does the company make money from credit cards? They do this by making a tempting offer to the customers. On the statement, as well as telling the customer how much they owe, they show the minimum payment that can be made.

If someone chooses to pay less than the full amount owing, the bank charges interest. The way it does this is complicated so, for now, imagine that the rate is 10% per month. Here is part of the credit card payment details:

a By how much did the amount owing to bank change by the end of February?

b What was the total amount of interest paid to the bank up to the end of February?

c Continue the debit, credit and balance columns to the end of April.

d What is the main problem for the credit card holder?

2 Why do you think minors are not allowed to have credit cards?

DRAFT

Percentage discounts

Is it really a bargain? Sometimes shops try to trick us into thinking that items are a bargain when they may not be. For example, do they think we might not buy a $9.99 book if the price sticker said $10? There hardly seems to be a day that goes by without shops offering items at discount prices. The discounts are often shown as a percentage.

Guided practice

1 Why do you think price stickers are often shown as $1.99, $2.99 and so on?

2 If a book is advertised at $9.99 and the discount is 50% or 10%, we don’t need a calculator to work out the new price. Work out the new price of the book with each of the discounts below. Round the new price to a sensible amount.

50%

DRAFT

20%

30%

3 Describe the method you used to find the answers in question 2. (If you used a calculator, it’s okay to say that.)

Extended practice

4 Sometimes the amount of discount can be a bit harder to work out. A knowledge of fractions can help, as can starting from a percentage that you are familiar with. For example, imagine you are at a shop and the $7 socks you want have a discount of 5%. 10% is easy to work out ($0.70). 5% is a half of 10%. So, the socks are discounted by half of $0.70 which is $0.35. Use the same method to find 5% of: a $9.00

Independent practice

1 To find 50%, we halve the amount. Use your knowledge of the conversion between fractions and percentages to show the fraction equivalent of these common percentages: a 10% b 33 1 3 % c 20% d 66 2 3 % e 1% f 25% g 12.5% h 20%

DRAFT

2 In the Guided practice section, you looked at a short cut for finding a discount price of 5%. Use a similar method to suggest a method for finding the following percentage discounts. a 15% b 75%

3 Any percentage can be turned into a fraction because percent means “out of 100”. So, 7% is the same as 7 100 . Write the following as fractions.

73%

31%

17%

43%

We can find less-common percentages of an amount (such as 11%) by converting to decimals. To explain the method, let’s start by finding a 1 4 of $10. (We already know that 25% of $10 is $2.50, so we will know if the method works.)

1 4 = 25 100

25

100 = 0.25

0.25 of $10 is the same as 0.25 × 10.

0.25 × 10 = 2.5

So, 25% of $10 = $2.50.

4 It works in the same way for any percentage. For example, what is 11% of $10?

Estimate first: 10% of $10 is $1.00. So ,the answer should be close to $1.00.

11

100 = 0.11

0.11 × 10 = 1.1

So, 11% of $10 = $1.1 (or $1.10).

Find the following. Don’t forget to estimate the answer first.

a 11% of $10

c 52% of $100

e 24% of $20

b 19% of $10

d 11% of $50

f 48% of $20

5 Most calculators have a key to help work out percentages quickly.

DRAFT

Circle the two ways of keying a calculator so that it will calculate 10% of $135 correctly. Estimate the answer first so that you know whether the calculator is giving the correct answer.

a 10, x % 135 =

b %, 10, x, 135, =

c 10, % x 135, =

d 135, 10, x, % =

e 135, x, 10, %, =

6 Use a calculator to find the values. Don’t forget to estimate first

a 11% of $40

b 19% of 40

c 52% of $40 d 13% of $85

e 24% of $90 f 48% of $50

7 In the finance world, we often see percentages that are not whole numbers. To calculate something like 2.5% of an amount, we can still use the method from earlier. However, we need to use place value skills. For example, what is 2.5% of $10?

2.5% = 2.5

100

2.5

100 = 25

100 = 0.025

0.025 × 10 = 0.25

DRAFT

So, 2.5% of $10 = $0.25.

a Try the method above to work out 4.5% of $10

b Use a calculator to check your answer to question a.

8

Round the figures to estimate the answers for the following. Then use a calculator method of your choice to work out the exact answer. Be sure to round the calculator to the nearest cent.

a 9.6% of $16

b 4.9% of $20

c 9.9% of $85

d 19.5% of $80

e 5.18% of $30

f 15.15% of $60

g 9.7% of $9500

h 10.95% of $5999

Estimate: $1.60 Exact answer:

Estimate: Exact answer:

Estimate: Exact answer:

Estimate: Exact answer:

DRAFT

Estimate: Exact answer:

Estimate: Exact answer:

Estimate: Exact answer:

Estimate: Exact answer:

Extended practice

1 We often see advertisements, or signs in shop windows similar to this one.

Shops are not allowed to lie to customers in their advertising.

a Why do you think shops use the word “up to…” in their signs?

b If a shop displays a similar sign, how many of the 100 items in the shop need to have a discount of 70%?

2 Imagine someone borrows $15 999 to buy a car at an interest rate of 5.16% p.a. (per year). If they pay the money back by the end of the year, how much interest will they have paid?

Estimate: Calculator answer:

3 A supermarket usually sells a pack of nuts for $13.50. They have them on sale for 8% off the original price.

a What is the discount amount?

b What is the new price of the nuts?

4 School sweatshirts are on sale for 35% off the original price. If the sweatshirts are $75.50, what is the discounted price?

5 An old building is 195 metres high. Demolition begins on Monday. Each day, 16% of the building’s height is removed.

DRAFT

a How much is removed at the end of the first day?

b If x is the amount left after five days, write an expression to show how much is left. Start with x =.

c Use the expression to calculate how many metres are left after 5 days

d The demolition company works a five-day week. On which day will the whole building be down?

Unit 5: Topic 1

Number properties

Vocabulary for algebra

A variable is an amount that can have different values. For example, when a sunflower is growing, the height changes every week.

A rule is something that is always true in a calculation between two (or more) variables. For example, every week the sunflower gets 16 cm taller).

A pronumeral is a letter or symbol that takes the place of a number. For example, we can let a be the number of weeks and b can represent the height of the sunflower.

A formula is a short way of writing a rule. For example, b = a × 16 (height = number of weeks × 16).

We can show how the height changes in a table of values

of weeks a 1 2 3 4

(cm) b 16 32 48 64

Guided practice

DRAFT

1 a The climbing plant, wisteria, can grow 24 cm a month. If a is the number of months and b is the amount it grows, write a formula for the rate that a wisteria plant grows.

Extended practice

b Show the information about the way that a wisteria plant grows in a table of values.

c Use the formula to calculate the amount of growth for a wisteria in one year.

2 Use the following rule to write a formula and table of values that suits this situation: A pizza machine makes its own dough. It prepares and bakes 20 pizzas an hour.

a Formula:

b Table of values:

Independent practice

DRAFT

Laws of mathematics

A law, in maths, is something that is always true and correct. Here’s a reminder of some of them.

Commutative law

This is when the order of doing calculations can be changed without the answer changing. Addition and multiplication are commutative.

For example, 3 + 4 = 4 + 3 and 2 × 6 = 6 × 2.

Subtraction and division are not commutative.

For example, 5 – 3 ≠ 3 – 5 and 12 ÷ 4 ≠ 4 ÷ 12

1

Use the commutative law. Draw number lines if you wish.

Example: 4 + −2 is easier to work out if we change it to −2 + 4.

–5–4–3–2–1012345 –2 + 4 = 2

a 5 + −1 =

b 13 + −8 =

c 11 + 4 + −3 =

b 10 + 4 + −8 =

Associative law

2

This is when you can place brackets in a calculation and it doesn’t change the outcome.

Addition is associative. Example: −3 + 7 + 5 = (−3 + 7) + 5 = 4 + 5 = 9.

Multiplication is associative. Example: 2 × 5 × 12 = (2 × 5) × 12 = 10 × 12 = 120.

Use the associative law to make the problems easier to solve.

Example: 17 + 3 + 19

= (17 + 3) + 19

DRAFT

= 20 + 19 = 39

a 27 + 21 + 19 =

b 13 × 5 × 4 =

Distributive law

This tells us that multiplying a number by two (or more) numbers added together gives the same answer as doing each multiplication separately. This is easier to understand with number examples: 3 × 43 is the same as (3 × 40) + (3 × 3).

3

Use the distributive law to make these easier to solve.

a 5 × 47

b 6 × 32

Inverse properties

A basic law of addition and multiplication is that they can be used inversely. Examples: 2 + 9 = 11 and 11 – 9 = 2, 4 × 25 = 100 and 100 ÷ 25 = 4.

4 Write expressions to show that the inverse operation rule does not apply to subtraction and division. Use the ≠ symbol.

a subtraction: 4 – 3 = 1 but 1 – 3

b division: 12 ÷ 4 = 3 but

5

To make 25 mL of apple juice it takes 1 apple.

a Using the pronumerals j for the amount of juice and k for the apples, write “ a formula that could be used to show the amount of juice that can be made from a variable quantity of apples.

DRAFT

b Show the information in a table of values for making quantities of up to 200 mL of apple juice.

Extended practice

1 In the Independent practice section, you looked at inverse operations. Look at the information about apple juice in question 5. Using an inverse operation and the information in the table of values, write a formula that can be used to calculate how many apples are needed to make a variable amount of apple juice.

2 Use the formula in question 1 to calculate the number of apples needed for the following quantities of apple juice.

DRAFT

3 Using the original formula for the amount of juice that can be made from any number of apples, complete this sentence: The amount of juice from half an apple is

4 A demolition company is taking down an old building that is 195 metres high. They can demolish 31.2 metres a day. They work a five-day week and begin the work on Monday. Use the information to complete the following tasks.

a How much of the building is left at the end of the first day?

b If x is the amount left after five days, write an expression to show how much is left. Start with x =.

c Use the expression to calculate how many metres are left after 5 days.

d The demolition company works a five-day week. On which day will the whole building be completely demolished?

5 A new building is set to replace the demolished building (in question 4). Each new floor adds 2.9 metres to the height of the building. Complete the following table of values to show how the new building will grow as it is being built.

6

Use the information in question 5 to complete the following tasks.

a Using x for the building height and y for the number of floors, write a formula that could be used to calculate the height of the building after any number of floors have been built.

b Use the formula to find the height of the building after 50 floors have been built.

c The finished building height is 280.2 metres. This includes a 4.7 m antenna on the top. Write an expression that will show how many floors the building has, then solve it.

DRAFT

Unit 5: Topic 2

Prime numbers, composite numbers and divisibility

If you have completed Topic 3 in Unit 1 (Exponents and square roots), you will be familiar with the difference between prime and composite numbers. Here’s a reminder.

Prime number: Any number greater than 1, that has only two factors (1 and itself). For example, 7 is a prime number because the only factors are 1 and 7.

Composite number: Any positive whole number that is divisible by at least one number other than 1 and itself. For example, 6 is a composite number because it has 4 factors: 1, 2, 3 and 6.

Guided practice

1 There is a prime number missing from each row in the chart above. Fill in the missing prime numbers.

2 What is the lowest composite number?

3 How do you find out whether a number has 2, 3 or 4 as one of its factors? As a start, almost 75% of the first 100 numbers are composite. This is not surprising when you think that every even number greater than 2 is a composite number. A divisibility chart can help to find factors. Use this divisibility chart to complete the tasks.

2 the last digit is even. The last digit in 37 792 is even (37 792 ÷ 2 = 18 896).

3 the sum of the digits in the number is divisible by 3. In 825 the sum of the digits is 8 + 2 + 5 = 15. 15 can be divided by 3 (825 ÷ 3 = 275).

4 the last 2 digits can be divided by 4.

Circle the numbers that are divisible by:

In 748 the last 2 digits are 48. 48 can be divided by 4 (748 ÷ 4 = 187).

Extended practice

Independent practice

This divisibility chart can be used to find out whether 2, 3, 4, 5, 6, 8, 9, 10 and 12 are factors of other numbers.

Is this a factor of the number? Yes, if …

2 the last digit is even.

3 the sum of the digits in the number is divisible by 3.

4 the last 2 digits can be divided by 4.

Example

The last digit in 37 792 is even (37 792 ÷ 2 = 18 896).

In 825 the sum of the digits is 8 + 2 + 5 = 15. 15 can be divided by 3 (825 ÷ 3 = 275).

In 748 the last 2 digits are 48. 48 can be divided by 4 (748 ÷ 4 = 187).

5 the number ends in 5 or a 0. 23 685 ends in 5 (23 685 ÷ 5 = 4 737)

6 the number is even and it is divisible by 3.

8 the last three digits read as a number that can be divided by 8.

9 the sum of the digits in the number is divisible by 9.

10 the number ends in 0.

DRAFT

12 the number is divisible by 3 and 4.

1 Circle the numbers that are divisible by

828 is even and the sum of its digits is 8 + 2 + 8 =18. 18 is divisible by 3 (828 ÷ 6 = 138).

In 4744 the last 3 digits are 744. 744 is divisible by 8 (93).

4744 ÷ 8 = 593

In 37 512 the sum of the digits is 3 + 7 + 5 + 1 + 2 = 18. 18 is divisible by 9.

37 512 ÷ 9 = 4168

369 420 ends in 0.

369 420 ÷ 10 = 36 942

5472 is divisible by 3 (Total of digits = 18) and by 4 (last 2 digits divisible by 4), so 5472 is divisible by 12 (= 456).

2 Use the divisibility chart to help you decide whether these numbers are prime or composite.

Number Besides itself and one, circle any other factors (12 and under).

a 105 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

b 127 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

c 297 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

d 181 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

e 223 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

f 287 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

g 323 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

Is it prime or composite?

3 These division problems have 2-digit divisors. Use a strategy that does not involve using a calculator to find each quotient.

DRAFT

a (no remainder)

323 ÷ 17 =

b Show the remainder as a recurring decimal.

347 ÷ 15 =

c Show the remainder as a fraction.

385 ÷ 14 =

d Take the remainder to 3 decimal places.

1057 ÷ 8 =

4

In question 2, you used divisibility tests to see whether a number was prime or composite. This worked because the potential factors were 12 and below. However, the test gave a false result for question 2g (323), which in not a prime number. 323 has two factors between 12 and 20) of 17 and 19 and it is a composite number. Which are the two factors of 323 between 12 and 20?

5 Use mental strategies to decide on the most likely answer for the following:

a 208 ÷ 26 = 6, 7 or 8?

b 580 ÷ 29 = 18, 20 or 22?

a 369 ÷ 41 = 7, 8 or 9?

6 Put in the last digit of these numbers to make them divisible by the divisor shown.

a 35 23

b 52 31

c 75 20

d 45 69

Extended practice

DRAFT

The divisor is 9.

The divisor is 6.

The divisor is 8.

The divisor is 7.

You will have noticed that there were no divisibility rules for multiplying by 7 or 11 on the chart in the last section.

For centuries, mathematicians have tried to discover a divisibility test for 7. Some suggestions are so complicated that most people ignore them. For example, here’s one idea:

Take the digits of the number in reverse order, that is, from right to left, multiplying them successively by the digits 1, 3, 2, 6, 4, 5, repeating with this sequence of multipliers as long as necessary. Then, add the products. If the resulting sum is divisible by 7, then the original number is divisible by 7.

1

Before you have a look at the following ideas for a divisibility test for 7 and 11, what do you think is the largest 2-digit number that is divisible by 7?

Divisibility rules for 7 and 11

Is this a factor of the number? Yes, if …

Example 7

the last digit is doubled then subtracted from the remaining digits (read as a number) and the answer is divisible by 7.

Total the digits in every odd column starting at the units.

Total the digits in every even column starting at the tens

Subtract. If the answer is 0, or is divisible by 11, the original number is divisible by 11.

203: 20 – (3 × 2) = 14. 14 is divisible by 7, so 203 is divisible by 7. (203 ÷ 7 = 29)

NB if the answer to the subtraction is 0, the number is divisible by 7 (e.g. in 126: 12 – (6 × 2) = 0. (126 ÷ 7 = 18)

11 319: Total of digits in ones, hundreds and tens of thousands = 13.

Total of digits in tens and thousands = 2

13 – 2 = 11.

11 is divisible by 11, so 11 319 is divisible by 11 (= 1029).

2 Try the divisibility test to see if 7 is a factor of any of the following:

406 1792 10 01

3 Try the divisibility test to see if 11 is a factor of any of the following:

319 31 411 23 425

4 Here is another divisibility test for 7:

A number is divisible by 7 if 5 times the one’s digit PLUS the rest of the digits is divisible by 7.

DRAFT

Use it to find out whether 7 is a factor of 3668.

5

The difficulty with the rule in question 4 is that you still might have to do a written division at the end of the solution (406 ÷ 7 in question 4).

Here is simplified version said to have been discovered by a 12-year-old in Nigeria only a few years ago:

A number is divisible by 7 if 5 times the one’s digit PLUS the rest of the digits is divisible by 7. Repeat until there are 2 digits left. If they are divisible by 7, the original number is divisible by 7.

This was his example: 1687: 168 + 7 × 5 = 203; 20 + 3 × 5 = 35. 35 is divisible by 7 then so are 203 and 1687.

Try the test with 2996.

DRAFT

6 Discussion point: given that almost everybody has access to a calculator, are divisibility tests for 7 or 11 necessary?

Unit 5: Topic 3

Algebraic expressions

As you are probably aware, good mathematicians look for time-saving shortcuts. So, instead of adding a list, such as 35 + 35 + 35 + 35 +35 + 35 +35 + 35 + 35 + 36, a mathematician spots that the list can become 35 × 10 + 1 = 351. In algebra, one shortcut is to dispense with the multiplication sign when working with pronumerals. So, if we look at the formula that was used to show how fast sunflowers grow in Unit 5, Topic 1, we said that the number of weeks (pronumeral a ) × 16 gives the height (pronumeral b ). The formula became: 16 × a = b. We can be shorten this and simply write 16a = b

Guided practice

1 Simplify these expressions by removing the multiplication sign.

a 3 × w = b 7 × b =

c 10 × y = d 1.5 × z =

DRAFT

2 It is OK to miss out the multiplication in an algebraic expression (such as 2 × a = 2a ). Explain why it is not OK to miss out the multiplication sign in an expression that contains only numerals (such as 2 × 7).

3 Fill in the gaps to match each algebraic expression with its simplified version.

Extended practice

Term

A term can be a single number or variable, or numbers and variables. Terms are separated by either + or – signs.

8x + 9

TermTerm

4

In an algebraic expression, terms can be added or subtracted if they are like terms. Terms with the same variables are the same. For example, 2a + 2a = 4a, but 2a + 2b cannot be put together because they are not like terms. Imagine we have 2 oranges and 2 apples, we cannot say 4 appleoranges!

Simplify these algebraic expressions by adding or subtracting the like terms

+

Independent practice

Simplifying algebraic expressions

DRAFT

Expressions with grouping symbols and multiplication signs can be simplified by removing the multiplication sign. For example, a multiplied by b can be written simply as ab

Examples:

3 × (a – 2) can be simplified as 3(a – 2), z × (4 + y ) can be simplified as z (4 + y ) (4 – s ) × 3 can be simplified as 3(4 – s ). To simplify multiplication with brackets, you need to put 3 in front of the brackets, that is a mathematical rule.

1 Simplify these expressions by removing the multiplication sign where necessary.

a 5 × (j + 3) = b 3 × (4 − b ) =

c a × (b 2 + 4) = d (5 – y ) × 3 =

e 3 × 4 × f × (z – 2) =

Division in algebraic expressions can also be simplified in order to remove the ÷ symbol. In the same way that 1 ÷ 2 can be written as 1 2 , a ÷ b can be written as a b

2 Write these division expressions in their fraction form.

a y ÷ 5 = b b ÷ 3 =

c 5 ÷ z = d 2a ÷ 3 =

e ab ÷ 3 = f c ÷ 2 =

g ac ÷ 4 = h (3 × 4) ÷ d =

Expanding expressions

An algebraic expression can be expanded by carrying out the operations that are shown. For example, 2(a + 3a ) becomes 2(4a ) = 8a

DRAFT

3 Expand these expressions by removing the grouping symbols. The first one has been started for you.

a 4(a + 3) = 4a +

b 5(b – 2) =

c 2(d – 4) =

d c + 5(a + 4) =

Substituting numbers for pronumerals

When we know the number that a pronumeral has taken the place of, we can substitute the number back in to the expression. If we know the value of a and of b in the expression at the top of the page (ab ), we can substitute the numbers into the expression. Let’s imagine that a has a value of 5 and b has a value of 10. If we substitute the numbers for the pronumerals, this becomes 5 × 10. (Notice that we need to put back the multiplication sign so as not to confuse ourselves!)

4 Find the value of the expressions in questions 2f, g and h by substituting the following numbers for the pronumerals:

a = 2 b = 5 c = 8 d = 3

a c 2 = b ac 4 = c 3 × 4 d =

5 Find the value of the expressions in question 3 by substituting the following numbers for the pronumerals:

a = 2 b = 5 c = 8 d = 3

a 4(a + 3) = 4a + b 5(b – 2) =

c 2(d – 4) = d c + 5(a + 4) =

Extended practice

1 If the expression 3a(a + 2) was expanded, explain why it would become 3a 2 + 6a and not 3a 2 + 6a 2

DRAFT

2 Look at the first four terms of this stick pattern.

a Which two formulae show the way this stick pattern grows if y = the number of sticks and z = the number of pentagons? A y = 1 + (z × 4) B y = 5 × z − (z

y = 5 × z

3 For the matchstick pattern above, prove that one of the equations is not true. (HINT: Choose one of the terms and substitute a numeral for z.)

4 In question 3, you were asked to find equations that were true or untrue by substituting numerals for the pronumerals. You also saw that there can be more than one rule to show the way a pattern grows. For example, the pattern below can be described as You start with one stick and then add two to make each triangle or You count 3 sticks for the first triangle and 2 matches for every other triangle

a For the stick pattern, let a = the number of sticks and b = the number of triangles.

Is one or both of the following formulae true for the number of sticks needed to make the pattern?

Formula A: a = 1 + (b × 2) or

Formula B: a = 3 × b – (b – 1)

b Substitute 10 for the number of triangles. How many sticks are needed?

5 Working with other polygons, draw geometric ‘stick’ patterns from, for example, squares, hexagons etc. Find one (or more than one) rule for the way the pattern grows. Try to write an algebraic expression for each rule you find. Make sure you prove whether or not a rule is true by substituting a numeral into the expression.

DRAFT

Unit 5: Topic 4

Linear equations

If you are one of the people who get panicky when they hear words like algebra and solving equations, DON’T! You’ve already been doing it comfortably for years. (Kai has 3 pens in one pocket and 4 in another becomes 3 + 4 = 7. There, that’s an equation solved.) In order to work with equations, here is a reminder about some of the vocabulary needed in algebra.

Expression: An expression is a number sentence with at least two numbers (or pronumerals) and at least one operation. For example, 25 × 3 or a – b.

Equation: An equation is a number sentence in which there are two expressions and both are equal. For example, 3 × 4 = 2 × 6 or 2a = a + a. In an equation, both sides must balance.

We can show it in a diagram. The scales are balanced because the mystery number (a ) in the red cup + 3 more beads balances 5 beads in the green cup.

You can probably already guess the mystery number, but we can show it mathematically. To keep everything balanced, whatever we do to the left (red) side, we must do to the right (green) side. So, we remove the 3 extra beads from the red side and we also take away 3 beads from the right side. Now we are left with a = 5 – 3 = 2.

take away 3

Guided practice

DRAFT

1 Use the same method to solve the following.

a x + 4 = 9

x + 4 − 4 = 9

So, x = b y + 5 = 15

Independent practice

1 Solve these equations by recognising that the same thing must be done to both sides to keep the balance even.

a b + 5 = 25

c m + 3 = 20

b y − 4 = 12

– 4 4 missing

n – 2 = 14

Linear equations

A linear equation is one that describes a line. It can have numbers and pronumerals but there are never exponents such 22, x2, 23 , x3 etc. For example, x + 5 = 13 is a linear equation, whereas x2 + 5 = 13 is not a linear equation. The linear equation above is like the equations on the first page. To solve it, we could use diagrams or just write the steps to solve it.

Equation: x + 5 = 13. We need to take the 5 away from a, BUT what we do to the left-hand side (LHS), we must do to the right-hand side (RHS).

Step 1: x – 5 = 13 – 5

x = 13 – 5 x = 8

DRAFT

2 Solve the following.

a x + 9 = 21 b x − 6 = 14

c 5x = 35 d x 2 = 14

e x + 25 = 15 f x 15 = 5

3 Sometimes we can solve an equation by guessing, checking and improving on the guess. Here’s an example of the guess, check, improve method:

Find the value of b if 3b = 162

Try b = 50: 3 × 50 = 150 (too low)

Try b= 55: 3 × 55 = 165 (too high)

Try b = 54: 3 × 54 = 162 (just right!)

Therefore b = 54

Find the value of z if: a 4z = 96

Word problems

We can use algebra to solve a word problem. Here’s an example: Lisa’s uncle is four times older than she is. He is 44 years old. How old is Lisa?

Let a = Lisa’s age.

4a = 44

a = 44 ÷ 4

DRAFT

a = 11 Lisa is 11 years old.

4 Write an equation and use a similar method to solve these word problems.

a Noah’s Great Grandma is 100. She is eight times older than he is. How old is Noah?

b Maia has 37 stickers in her book. Her brother Ari has four times as many. How many stickers does Ari have?

Extended practice

1 Use an appropriate method to solve the following equations.

a 2g = 26 b h + 9 = 34 c 0.5x = 4

d 3 – m = 1 e 2b + 4 = 25 f 2(3 + b ) = 24

g 4(3 + x ) = 18 h b + 1.5 = 4 i 1 3x = −2

2 Use the example to solve the equations.

Example: Find the value of c if a = 3 and b = 5.

c = 2a + b c = 2 × 3 + 5 c = 6 + 5 Therefore, c = 11.

DRAFT

a Find the value of y if w = 4 and x = 7.

y + 8 = w + 3x

b Find the value of m if j = 5 and k = 10. m = k j

c Find the value of y if w = 5 and x = 12.

4y = 2w + x 2

d Find the value of m if j = 8 and k = 2.5.

2(m + 3) = 2k + j 2 =

3 Look at this expression and give a possible value for a 5a < 20 A possible value for a is .

4

Explain why b cannot have a value of 5 in this expression: 3b < 14.

5

Imagine that the total of the ages of two sisters is 17. One is 3 years older than the other. What are the ages of the sisters? You could use algebra to simplify the problem. Which of the following two linear equations will solve the problem if we let a be the age of the younger sister, and b be the age of the other? You could try the guess, check, improve method to substitute a number into each equation.

a a + (a + 3) = 17

b b = a + 3

c How old are the two sisters?

6 Backtracking is a useful tool to check your working. It’s like doing an inverse operation in a problem: We start with p. Add 2. Multiply by 3. The answer is 21. Could p be worth 5? If we backtrack the operations the value of p is 5.

Therefore

Write and solve an equation that shows the equation to be true.

DRAFT

Unit 5: Topic 5

Interpret and explain patterns

Patterns

In the animal world, there are those, like bees, who seem to love patterns, and those, like the tree sparrow, that aren’t too fussy about them.

We humans seem to love patterns in our lives. Imagine finding house number 329 on a road if there were no pattern to the way the houses were numbered. We can find patterns in numbers and in shapes.

Guided practice

1 Complete and describe the patterns in these number sequences.

a 1, 2, 4, 8, , , The pattern is .

b 225, 196, 169, 144, , , The pattern is

DRAFT

c 512 343, 216, 125, , , The pattern is

2 In this table you see the first four terms of the sequence in question 1a.

1 2 4 8

a Complete the table for the next three terms.

b Make a table for the number sequence in question 1b.

c Which number will be at the ninth term in the number sequence?

3 Create number sequences using the following rules.

a Start at 5 and find the next four terms by adding 15: 5, , , ,

b Start at 3 and find the next four terms by multiplying by 2 and subtracting 1: 3, , , ,

c Make a table for the number sequence in question 3b.

Independent practice

DRAFT

1 In Unit 5, Topic 3, you looked at stick patterns.

a Remind yourself of the rule for the way the pattern grows by writing a formula for a variable number of triangles. Let a be the number of sticks and b be the number of triangles.

b Use the formula to show the number of sticks needed for 50 triangles.

2

This is a diagram for a square pattern made with matchsticks.

Complete the table.

No. of squares

No. of sticks

3 The sequence for the first five terms of the triangle pattern has been plotted on this grid.

Pattern of Triangles

Number of triangles made 25 20 15 10 5 Number of matches used 12345

4

Pattern of Squares

Number of squares made 25 20 15 10 5 Number of matches used 12345

Plot the sequence for the square pattern from question 2 on a similar grid.

For the stick pattern of triangles we used = 1 + (the number of triangles × 2).

The formula from this becomes y = 1 + (z × 2) if y is the number of sticks and z is the number of triangles.

So, if we want to know how many sticks are used in a pattern of twenty triangles, we can work it out like this:

Let z = 20

DRAFT

y = 1 + (20 × 2)

y = 1 + 40

y = 41

Therefore, the number of sticks used in twenty triangles is 41.

a Think of the square stick pattern. Using the same pronumerals, write a formula for finding the number of sticks used in a variable number of squares.

b Use the formula to calculate the number of sticks need for 30 squares.

5

6

Here is the inverse of the formula in question 4: z = y – 1 3

Let’s see whether it is true for finding the number of squares (z ) that can be made using a variable number of sticks (y ).

a Inspect the formula by substituting 16 for y.

b What is the result if you use 17 as the variable for y?

c Explain why the formula does not work for any variable of y.

If the stick pattern of triangles was arranged like this, how would it affect the formula in order to show the number of sticks needed for any number of triangles?

7 Draw the first five terms of a stick pattern of squares that would make this equation true if y is the number of sticks and z is the number of squares: z = 4y.

DRAFT

Extended practice

1 Complete the table for the first six terms of this pattern made with sticks.

2 After making the first pentagon, what is the rule for adding sticks to the pentagon pattern: Using your rule what is the number of sticks that would be needed for the 12th term of the pattern .

3 On the blank grid, plot the sequence for the first eight terms of the pentagon pattern.

4 Draw a line through the points you plotted. Describe the line.

5 Write a linear equation that is true about the stick pattern of pentagons.

Unit 5: Topic 6

Working with spreadsheets

Spreadsheets

Computer spreadsheets have lots of uses, from doing complicated calculations to presenting data on a beautiful graph. However, relying on a spreadsheet is a bit like relying on a calculator for your maths work: they are really useful, but they are only as smart as the person who enters the information. If you want a calculator to multiply 283 × 17, it will do it in the blink of an eye, but if your finger hits the + key instead of the × key … well, you know the rest!

Guided practice

1 A spreadsheet has a whole screen full of ‘boxes’ called cells. The cells are arranged in numbered rows and in columns that each have a letter. The name of the cell that says, ‘hello’ is A6.

a Which cell is highlighted (i.e. has a box around it)?

DRAFT

b Write the number 3 in cell A2 and the number 4 in cell B4.

2 For this activity, you will need access to a computer with a spreadsheet program, such as Excel or Numbers

a Pages in a spreadsheet are called sheets. If you open a new sheet. It will have lots of empty cells and a menu bar at the top. Click A2 and enter 3. Enter 4 in B2.

b To change the colour of the cells, or the font, you can use the Format buttons. Ask if you need help to find them.

c Click on A2 and change the cell colour. Click on B2 and change the font colour. It should end up looking something like this:

d If you have time, format other things, such as the font size, the font or place a border around a cell. Don’t forget, you need to click on a cell for the computer to know that you want to work on that cell.

Independent practice

Entering data on a spreadsheet

1 Here is a list showing the number of stickers seven people have.

Noah 25

Aria 43

Maia 3 3

Anna 26

Jack 19

Kai 32

Olive 11

DRAFT

a Open a new sheet on a spreadsheet.

b Start at A1 and enter the names from cells A1 to A7.

c Start at B1 and enter the numbers down to B7. It should look like this:

d If you have time, or to practise your skills, you could ask your teacher if you can format the list. For example, you could change one of the names to your own. You don’t need to delete anything, just click on the cell and rewrite.

2 Often we want the program to work on a group of cells, such A1 to B7. To do this, click on A1, hold the SHIFT key and click on B7. The cells should look something like this:

3 Sorting the data

We are going to ask the computer to sort the data alphabetically.

4

a Make sure cells A1 to B7 are highlighted and then click Data on the menu button.

b Click on the button that will sort the list from A to Z.

c It should now look like this:

d What happened to the data about the number of stickers?

e What would be the problem if you just highlighted cells A1 to A7 and clicked ‘sort alphabetically?

With your teacher’s permission, try the above with your own set of data.

5 A spreadsheet can work like a super-calculator. However, some symbols for basic calculations are different. Here are five basic symbols used on a spreadsheet: –, *, +, / and ‘enter’ (or ‘return’ on a Mac)

Match them with the normal calculation symbols by writing them in this table.

DRAFT

6 We are going to tell the spreadsheet program to do some calculations for us.

a Open a new sheet.

b Enter x7 in cell A1 and the numbers 1 to 10 in cells A2 to A11.

c Enter the number 7 in cell B2. It should look like this:

7

d Click on cell B2. Click and hold on the dot at the bottom right-hand corner.

Drag down until you reach cell B11. When you let go, it should look like this:

Now we are going to get the program to work for us! We are going to use something that is already built into the program. It is called a Function. The function we are looking for is a formula to multiply. A function always begins with an = sign.

a Click on cell C2 to highlight it. We want to multiply A2 × B2. So, we enter =A2*B2 (no spaces and * for multiplied by ). When you enter this, the cells will change colour, like this:

b Press enter/return and the multiplication is complete!

8 Now we want all the rows to be multiplied by 7. BUT there’s a shortcut (phew!)

a Click on the bottom corner of cell C2 (just as you did in question 6 above).

b Drag all the way down to C11 to see what happens. It should now look like this all the way down to 10 × 7.

9 Let’s try an addition. On the first column, you had the numbers 1 to 10. The program will add any list of numbers but, for now, we’ll just get it to add all those numbers. We use a different formula.

a Click on A12.

b Type (no spaces and no need for upper case letters): = SUM(A2:A11).

DRAFT

Press enter/return. Hey presto! The sum is 55.

If you make a mistake, you will get an error message. Don’t panic! Just click the “undo” key and start again. (Don’t forget to put the brackets and the colon in the right places.)

Try other calculations. For example, your 17 times table.

Extended practice

More things we can do with a spreadsheet

1 Enter the following information from the results in the Rugby World Cup 2023 into a new Excel (or other) sheet. Don’t worry about the points difference at this stage. Start by entering ‘Country’ in cell A1.

2 We want the program to subtract the points against from the points scored to find the difference. Ask the program to do that for France like this:

a Click on cell D2.

b Enter B2–C2 but, before pressing Enter/Return, click on the bottom righthand corner of cell D2 and drag down to D6. You should see the following information:

c Enter the information into the table in your book.

3

4

The table does not look very interesting, but we can use another spreadsheet function to improve this by turning it into a graph. The spreadsheet’s name for a graph is a chart

a Click on cell A1, press shift and click on D6 to highlight all the cells. From the Insert menu, choose Chart, and then choose Column Graph

The column graph should look something like this:

DRAFT

If you have time, you can edit the chart title and colours on the graph. You could even add a flag for each country.

There are many more functions that you can use on a spreadsheet, such as finding the average of a set of numbers. Carry out some research to find out more. The world is your oyster once you have spreadsheet skills!

Unit

1

The metric system

New Zealand began using the metric system in the 1970s. Nowadays, around 95% of the countries in the world have adopted the system.

One metre is known to be one ten-millionth of the distance between the north pole and the equator, though it is doubtful whether anybody has actually tried that out with a trundle wheel!

A kilogram (kg) is said to be exactly the mass of one litre (L) of water. 10millionmetres

Guided practice

1 Using powers of ten, which of these represents the distance from the north pole to the equator?

2 1 L of water has a mass 1 kg. Write the mass of these amounts of water in kilograms with decimal notation.

a 200 mL b 375 mL

c 1250 mL d 1 mL

e 20 mL f 950 mL

g 1 L 750 mL h 10 mL

DRAFT

3 Convert these masses to the equivalent amount of water. Write the answers in mL. a 1.3 kg

4 A water truck is empty. Its mass is 2.75 tonnes (t). When it is filled with water, the gross mass is 4.075 t. How much water is the truck carrying?

Independent practice

1 Fill in the gaps in this table to show the sizes of these units of length in relation to each other.

2 Round to the nearest metre.

a 3.509 m b 3.09 m c 2597 cm d 2200 mm

3 Convert and round to the nearest centimetre. a 23 mm

4 This line is exactly 2cm long.

DRAFT

a Without using a ruler, estimate the length of each of these lines to the nearest centimetre.

b Measure the following lines and complete the table.

5 Complete the table to show the conversions between these lengths.

6 Calculate the perimeter (P) of these regular shapes. They are not drawn to scale. Show the answer in cm and in mm.

7 Measure to find the perimeters of these rectangles. Show the answer in mm.

DRAFT

8 Calculate the missing lengths (x and y) and show the perimeter (P) of these shapes. They are not drawn to scale

Extended practice

Plasterboard is sold in various sizes. For some reason the lengths are in metres but the widths are in millimetres. The table shows the available sizes at a store.

2.7 m

3 m

3.3 m

3.6 m

4.2 m

4.8 m

5.4 m

6 m

DRAFT

Availability: Available, Not available, Special order Ahmed and Amira are doing work at home. They know that plasterboard is quite easy to cut, but they don’t want to buy too much because it is quite expensive. They have measured everything in centimetres.

1

They need a board for a cupboard wall. It is 325 cm high and 120 cm wide.

a What size board should they get?

b What size of board will be left over?

2 This is a scale drawing that they made of another wall. The only height shown is the door. Take note of the scale of the drawing.

cm Scale 2cm : 1 m

They have worked out that they can buy just seven plaster boards for the room, and they only need to make one cut.

a Use the table above to decide which pieces they should buy.

DRAFT

b Draw on the diagram to show how they can place the boards. Write the dimensions on each piece of board.

3 The plasterboard costs $39.50 per square metre. How much does the board that Ahmed and Amira got for the room cost?

Unit 6: Topic 2

and time

Have you ever been on a trip that took lots longer than you thought it would? A journey of 60 km where the speed limit is 60 km/h should only take an hour. Of course, that’s not always the case. If the average speed is only 30 km/h, the journey takes twice as long. Three factors affect a journey: distance, speed and time.

A car that goes at 30 km/h for 2 hours travels 60 km. The diagram shows that the speed × the time = the distance travelled. We can use the formula s × t = d : 30 × 2 = 60.

x

Distance = Speed x Time d = s × t

Guided practice

1

How far does a cyclist go if she cycles at:

a 15 km/h for 2 hours?

b 20 km/h for half an hour?

DRAFT

If we know a car goes 140 km in 2 hours, we can calculate the speed. The diagram shows that the distance ÷ the time = the speed. We can use the formula s = d ÷ t : 140 ÷ 2 = 80 km/h.

Distance

Speed = Distance ÷ Time s = = ÷ d t

SpeedTime

2 How fast is a cyclist going if she travels 24 km in 2 hours?

If we know a car travels 280 km at a speed of at 70 km/h, we can calculate how long it takes. The diagram shows that the distance ÷ the speed = the time. We can use the formula t = d ÷ s : 280 ÷ 70 = 4 hours. SpeedTime

Time = Distance ÷ Speed t = = ÷ d s

3 How long does it take a cyclist to travel 18 km at 12 km/h?

Independent practice

Here’s a reminder of the three triangles that show the relationship between distance, speed and time.

SpeedTime Distance

Distance = Speed x Time d = s × t = x

DRAFT

Speed = Distance ÷ Time = ÷ s = d t

Time = Distance ÷ Speed = ÷ t = d s

You may wish to refer to the diagrams to complete the following tasks.

1

A French high-speed train leaves Paris at 8:30 and arrives at Bordeaux at 11:00. It travels at 200 km/h. How far does it travel?

2 A 19 th century train from Glenbrook, south of Auckland, goes at 30 km/h for the 7.5 km trip. How many minutes does the trip last?

3 In the old days, car travel was a bit precarious. A car might take around 5 hours to complete a journey of 120 km. What would the average speed of the car have been?

DRAFT

4 The average time for the runners to cover 42.195 km in the Auckland marathon in 2023 was about four and a half hours.

a At a rounded speed, which of these shows the average speed of the runners for the four and a half hours: 6 km, 7 km/h, 8 km, 9 km?

b There were some runners who were older than 70. Some didn’t finish, whereas one completed the course in 6 hours. What was their average speed? Round the answer to two decimal places.

5

The Tour de France is a famous cycle race. The riders sometimes reach speeds of up to 80 km/h.

However, in the high mountains, they go much slower.

a Some riders average 20 km/h on a 10 km climb. How many minutes do they keep up that speed?

b One of the stages was 288.75 km. The leader averaged 35 km/h for the ride. How long was he riding for?

6 A tortoise travels at 0.5 km/h for an hour and a quarter.

How far does it travel?

Extended practice

DRAFT

In 2009, Jamaican runner Usain Bolt became a new world recorder holder for the 100-metre sprint race. A really good Olympic sprinter can run 100 metres in 10 seconds. How fast do they run? Because they run a short race, we don’t calculate their time in km/h, but in metres per second (m/ps). This chart shows some times for completing 100 metres. Various times to cover 100 metres have been plotted on this grid. Usain Bolt’s time is shown in gold. However, the cheetah’s time is even faster.

1

Remembering the formula to find speed when distance and time are known, calculate the times for the following in metres per second (m/ps). Take the time to 1 decimal place if necessary.

a an Olympic runner

b the cheetah

c the walker

2 a Estimate the time for the Year 7 person.

b What was the speed of the Year 7 person?

3 Look carefully at the graph to work out how long it took for the walker and Julia to complete 20 metres.

a Work out how long it took them to finish the 100 metres.

b What was the speed of the walker?

4 Usain Bolt’s record time in 2009 was only a few hundredths of a second faster than anyone else – but it was still a world record. Find out the actual time.

5 Put the information from the graph on a spreadsheet. Then use the following formula to show the speeds.

(Don’t forget to click and hold the bottom right-hand corner of cell D2 and drag down through the other rows so that the program does all the calculations for you.)

6 If you get the opportunity, you could add further names to the list to compare times and speeds (perhaps your own?).

Distance and displacement

On this phone map, we see that the car must travel 3.5 km. However, its displacement (the distance from where it started) is only 2.5 km. We sometimes refer to displacement as “as the crow flies”, because it is in a straight line.

Displacement 2.5 km

Total distance: 3.5 km

7 Olive swims at 3 km/h for 30 minutes in an Olympic-sized pool.

a How far does she swim?

b She swims three more lengths to warm down. Explain why her displacement is only 50 m.

DRAFT

Unit 6: Topic 3 Area and volume

Area is the region covered by something, such as the top of table. It is typically described in square units. For example, New Zealand has an area of around 268 000 km2

Volume is the amount of space that something takes up and is normally described in cubic units. For example, the volume of this Rubik’s cube is 216 cm3

Calculating area

When we are calculating area, rectangles are the easiest shapes to work with because we just need to know the length and width (sometimes known as the breadth). We can follow the formula Area = length × width (A = l × w ) or Area = length × breadth (A = l × b ).

Guided practice

1 Use the formula to calculate the following areas. (They are not drawn to scale.)

Calculating volume

DRAFT

When we are calculating volume, rectangular prisms are the easiest to work with because we just need to know the length, width (sometimes known as the breadth) and height. We can follow the formula Volume = length × width × height (V = l × w × h).

2 Use the formula to calculate the volumes of the following rectangular prisms. (They are not drawn to scale.)

Independent practice

1 Calculate the area of the shaded parts of these diagrams. Use centimetres for the dimensions. (The shapes are not drawn to scale.) 9

2 Using B for the length of the base and H for the height of the triangle, circle any of the following formulae that could be used to calculate the area of a triangle.

a Area = B 2 × H

c Area = B × H 2

e Area = B × 2 H

3 Which other dimension would you need to know in order to find the area of this parallelogram? (It is not drawn to scale.)

4 Measure to find the area of this rhombus.

5 Fill in the gaps to show how to convert between units of area. a 1 cm2 = mm2 b 1 m2 = cm2 c 1 ha = m2

d To convert 5cm2 into mm2 I would multiply by

e To convert 8m2 into cm2 I would multiply by

6 Write Yes or No next to the following formulae to show whether they could be entered into cell C2 in the spreadsheet in order to calculate the area of a triangle.

a = (A2*B2)/2

b = (A2/B2)*2

c = (A2/2)*B2

d = (B2//2)*A2

Volume and capacity are related. Whereas volume is the amount of space something (like a box) occupies, capacity is the amount of something (usually a liquid) that can be poured into something. Litres and millilitres are typical units of capacity.

7 Using the conversion chart, fill in the gaps to convert between these units of volume and capacity.

DRAFT

8 How do you know that the volume of this prism is 0.125 m3?

What other measurement would be needed to be able to calculate the volume of this prism? 10 Calculate the volume of this right-angled triangular prism.

11 Calculate the volume of this triangular prism.

12 The Trans and the Smiths each have four people in the family. Each person showers for 5 minutes per day. The Tran family has a water-saving showerhead. It uses 8 L of water per minute. The Smiths have an old-style shower that uses 18 L per minute.

Complete the table to show how much shower water each family uses.

Extended practice

Finding the area of any shape on which rectangles or triangles can be drawn is relatively straight forward. Finding the area of a circle, however, is slightly more complicated. Over 2000 years ago, in Ancient Greece, Archimedes found that the circumference of a circle divided by the diameter always has the same answer: 22 7 . This became known as Pi and has the symbol π

The area of this circle is:

This can also be used to find the area of a circle. To find the area of a circle we multiply π by the square of the length of radius of the circle. We can see the calculation in a formula:

You may wish to use a calculator for the following.

1 What is the area of the following circles? They are not drawn to scale. Round to two decimal places.

2 Finding the volume of rectangular and triangular prisms is just about as easy as finding the area of a triangle or rectangle. The good news is, we can use the knowledge about the area of a circle to be able to calculate the volume of a cylinder. To find the volume of a cylinder we multiply by the square of the radius of the base and then multiply the answer by the height. We can see the calculation in a formula: Use the formula to find the volume of the following cylinders.

DRAFT

Unit 7: Topic 1 Polygons and circles

A polygon is any closed two-dimensional (2D) shape with straight lines. A circle, or any 2D shape with curved edges is not a polygon. In the living world, apart from a few animals such as bees and some spiders, humans are the only ones that seem to love polygons; we love right angles, especially in rectangles. There are many types of quadrilaterals, but the square is the cream of the crop. There is no other quadrilateral which has at least one attribute (or property) of every other quadrilateral.

Guided practice

DRAFT

1 a Here we can compare many types of quadrilaterals. Fill in the blanks. Are opposite sides equal? (Number of pairs?) Are opposite sides parallel? (Number of pairs?) How many sides are equal? Are adjacent sides perpendicular? Do the diagonals intersect? Do the diagonals bisect each other at right angles? Does it have rotational symmetry? (What is the order?) Name the shape.

b What property is common to all but one of the quadrilaterals?

c Comment on the difference between shapes C and F.

d Comment on a similarity and a difference between shapes A and E.

Independent practice

1

a Compare the following types of triangles. Fill in the blanks. Is it acuteangled? Is it obtuseangled? Is it rightangled? Is it a scalene triangle? Is it an isosceles triangle? Is it an equilateral triangle? Does it have rotational symmetry? Is (are) the longest side(s) opposite the widest angle(s)?

DRAFT

b Explain the connection between the longest side and the widest angle in each triangle.

2

Follow the instructions to draw this triangle.

• Using XY as a base line, draw a 5cm line at an angle of 60° from X.

• Label the new line XZ.

• Join points X and Z.

• Mark the sides that are of equal length.

What type of triangle is this?

3 a Follow the instructions to draw this quadrilateral.

• Using AB as a base line, draw line AC 4.5 cm long forming an angle of 60° upwards from point A.

• Using AB as a base line, draw line BD 4.5 cm long at an angle of 60° upwards from point B.

• Join points C and D with a straight line.

DRAFT

b Mark the sides that are parallel.

c What type of quadrilateral is this?

4

Follow the instructions to draw this quadrilateral.

• Measure WX and draw line WY the same length as WX at an angle of 70° from point W.

• Draw line XZ the same length as WX at an angle of 110° from point X.

• Join points Y and Z with a straight line.

X

b What type of quadrilateral is this?

c Draw in the diagonals and comment on the angles formed at the place where the diagonals cross.

Extended practice

DRAFT

You are perhaps familiar with many parts of a circle, such as the circumference, the diameter and the radius. For the next task you will need to use three more terms:

Chord: a straight line from one point on the circumference to another, so that there are two segments (parts) of the circle.

Segment: a region of the circle that is formed when a chord is drawn.

Tangent: a straight line outside a circle that touches, but does not cross, the circumference.

1

a Use a pair of compasses to draw a circle with a radius of 4 cm. Let the circumference of the circle touch, but not cut, the line below (AB).

2

b With the compasses at the same setting, put the point anywhere on the circumference and cut the circumference with two small arcs.

c Draw a straight line, CD, between the points where the two arcs cut the circumference.

d Label lines AB and CD correctly as “tangent” or “chord”.

e Label the minor and major segments of the circle.

a Use geometrical instruments to draw quadrilateral WXYZ, which is congruent to quadrilateral ABCD below but is the reflection of it.

DRAFT

b Use geometrical instruments to draw a design that is congruent to this one.

Unit 7: Topic 2

An angle is formed at the point where two straight lines meet.

∠ ABC is formed where lines AB and BC meet, making an acute angle. We can identify an angle by using the vertex and the letters at the end of the arms. This angle’s name is ∠ ABC. The vertex letter is always in the middle of the three letters. When straight lines intersect (cross), more than one angle is formed.

In this diagram, lines AB and CD intersect at point E, making two acute angles and two obtuse angles. The arcs show that ∠ AEC is the same size as ∠DEB. ∠ AEC is adjacent to ∠ AED. ∠ AEC is vertically opposite ∠DEB.

Guided practice

1 In the diagram above, which angle is:

a the same size as ∠DEB?

b vertically opposite ∠ AED?

c adjacent to ∠DEB?

2 Complete the table.

Independent practice

1 In this diagram, the two lines make more than one angle. ∠DBC is the marked angle. The adjacent angle is called a supplementary angle because the sum of two angles is 180º.

AB D C

a What is the name of the supplementary angle?

b Draw a single arc on the supplementary angle.

c Explain why the angle marked with two arcs in the diagram cannot be called ∠B.

2 If the sum of two angles is 90º, they are called complementary angles. In this diagram, ∠ ABC is a right angle. The sum of angles ∠DBC and ∠ ABD is 90º. So, we can call them complementary angles.

a What is the size of ∠ ABD?

DRAFT

b Name the complementary angles in ∠ XYZ?

3

Complete the table of information about the following angles.

Name the marked angles. Are the angles adjacent? Are the angles complementary? Are the angles supplementary? Calculate the size of the unknown angle.

DRAFT

4 In this diagram, there are two pairs of equal angles. The arcs show that the vertically opposite angles are the same size.

a Name two angles that are supplementary.

b If ∠ AEC = 30º, what is the size of ∠DEB?

5 Calculate the value of x °.

6 Name and calculate (do not measure) the size of the three other angles in this diagram.

A B C

Extended practice

DRAFT

Alternate angles are angles that occur on opposite sides of a line and have the same size.

Corresponding angles have the same relative position at different intersections.

1 In the diagram, identify the following angle pairs as adjacent, alternate, corresponding, vertically opposite, supplementary and/or equal.

(Note: More than one attribute may apply to each pair.)

a i ∠EFB and ∠FBC are

ii ∠ ABF and ∠DBC are

iii ∠BFG and ∠DBC are

iv ∠EFH and ∠FBG are

v ∠ ABD and ∠EFB are

b If ABD is 55°, then:

i

DBC = ii ∠FBC = iii

BFG = iv ∠ ABF =

2 Calculate and mark the sizes of the unknown angles in this parallelogram.

DRAFT

a ∠DAB b ∠ ADC c ∠DCB

3 Prove by measurement and calculation that the sum of the angles in this trapezium is 360°. Write M (measured) or C (calculated) next to each angle as you mark its size on the diagram.

Unit 8: Topic 1

Prisms and nets

A polyhedron is a 3D shape with flat faces that are polygons. The word comes from Greek words meaning “many faces”. There are many types of polyhedrons (or “polyhedral” as the plural is sometimes written.)

A prism is a polyhedron in which the two bases (ends) are the same shape and size. All the edges of a prism are straight. A prism gets its name from its bases.

In a right prism, the angles between the base and the other faces are right angles. This rectangular prism is a right prism.

In an oblique prism, the angles between the matching ends and the other faces are not right angles. This rectangular prism is not a right prism.

We don’t see many oblique prisms in the built environment!

Guided practice

1 E D C B A

a Which of the shapes are polyhedrons?

b Which of the shapes are prisms?

c Which of the shapes are right prisms?

d In which shape are none of the faces polygon?

2 We see many right prisms in the built environment. Why do you think that there are not many buildings that are oblique prisms?

3 A net in geometry is a pattern that can be folded to make a model of a 3D shape. This net is to be made into a dice. Remembering that the sum of the opposite faces on a dice is always seven, finish numbering the faces. 1 2

Independent practice

1 Compare the properties of 3D shapes by completing this table.

Shape Is it a right prism? Is it an oblique prism? No. of flat faces? No. of curved faces? Are all the faces congruent? Name A Yes No 6 0 Yes Cube

I

2 a Complete the table for objects A, B and I from question 1.

Shape No. of faces No. of vertices Total no. of faces and vertices No. of edges What is the connection between columns 4 and 5?

b A 3D shape has four faces and four vertices. How many edges does it have?

c What could the object be?

3 Complete the activities by studying the way these shapes are to be cut.

a Draw the cross-section if shape C were cut as shown in the direction of the arrow.

b The cut in which shape would result in this cross-section?

c In which shape would the cross-section not be uniform?

d A rectangular section could be a cross-section of all three objects. Explain why.

DRAFT

4 Draw a net for a right triangular prism with bases that are equilateral triangles. You may wish to practise on spare paper first. If you intend to actually make the prism later, don’t forget to add tabs so that the faces can be glued together.

Extended practice

1 The descriptions below will enable you to identify five different objects.

a Write the geometrical name of each object.

b Name something in the environment that is based on this shape. You may find it useful to sketch the shape in order to identify it.

Description Geometrical name A shape like this in the environment

A Its cross-section is uniformly circular.

B Its cross-section is uniformly triangular.

C It has a circular base and an apex that is at a uniform, fixed distance from the centre of the base.

D Its base is a polygon and its 5th vertex is at a fixed, uniform point from the centre of its base.

E Every point on its surface is at a fixed, uniform distance from its centre.

2 In question 2 of the Independent practice section, you looked at the connection between the number of edges on a 3D shape and the sum of its faces and vertices.

This is known as Euler’s Law and the formula (F + V − E = 2) holds true for every polyhedron.

Prove Euler’s formula by completing the following table. (You may find it useful to draw sketches.)

DRAFT

3 There is a special group of 3D shapes called The Platonic Solids. There are only five of these shapes. A cube is one of them. Carry out some research to find out what makes them special. As a clue, these are their nets:

DRAFT

Unit 8: Topic 2 Transformations

Shapes can be transformed in three main ways: translation, reflection and rotation

Translation:

Reflection:

Rotation:

DRAFT

They can also be transformed by size, scaling them up or scaling them down.

Decide which method has been used to transform the shapes in the following Ma – ori

2 We can show that an image has been enlarged or reduced by writing the scale factor in an abbreviated way. If it is three times larger than its original size, we write 3:1 and if it’s three times smaller 1:3. (The 1 indicates its original size).

The scale of the second dog on the grid at the top of this page is 2:1.

Write the scale of the third dog compared to its original size.

3 Draw transformation patterns using these shapes. Use different methods for each. Write the way it has been transformed below the pattern. (You may wish to use a combination of methods. For example, translation and reflection.)

Independent practice

DRAFT

1 Complete and describe these patterns. (There is no need to include the colours in your description.)

2

This is a version of a Tangram puzzle. The rectangle can be dissected (cut up) into 7 shapes. Patterns and pictures can be made using some, or all, of the dissected shapes.

Draw and cut up a Tangram rectangle on a piece of 12 cm × 6 cm grid paper. Use the shapes to create patterns. Draw them on grid paper. Copy and colour your favourite onto this grid.

3 Use these examples of Kowhaiwhai patterns as your inspiration to create your own transformation design. Use some of your own shapes if you wish. If you choose not to use the grid, use blank paper.

Extended practice

DRAFT

Lines can be transformed as well as shapes. The starting position of the blue star can be described by its number on the horizontal (x ) axis and its number on the vertical (y ) axis. The coordinate point is (−1,2).

It has been translated by moving it 5 places to the right along on the (x ) axis and 2 places up on the (y ) axis. The translated coordinate is (4,4).

The red line has been translated 5 places to the right along the (x ) axis.

1

a What is the coordinate for the blue circle?

b Translate the blue circle 3 points to the right and 1 place up.

c What is the translated coordinate for the circle?

d Move the hexagon by translating it to (3,1).

e Describe the translation of the hexagon.

2 The blue triangle has been translated on the grid. The original position is shown as A. The transformed position has a mark (Aʹ ) next to it to show that it is the transformed image.

a Describe the transformation of the triangle.

b Draw a second translation of triangle A by moving it 3 places to the right and 2 places down. Show that it is a second transformation by marking it Aʹʹ

3 Translate rectangle B by moving it 5 places to the right and 0 places up. Transform it also by a factor of 2:1.

DRAFT

Compass points

There are four cardinal points: north, east, south and west (N, S, E, W). The points in between, north-east, south-east, south-west, north-west (NE, SE, SW, NW) are called ordinal points.

Sometimes we need to tell the direction between the cardinal and ordinal points. Between north and north-east is called north-north-east (NNE) and between north-east and east there is east-north-east (ENE). The letter that goes first will always be the cardinal point. So, if we are looking between north and north-east, the cardinal point is north. So, we add another north (N), and the point is NNE. Between north-east and east, the cardinal point is east (E), and the point is ENE.

Guided practice

1 Fill in the blanks on the compass rose above.

2

The degree of turn between north and north-east is half a right angle, or 45º.

Sailors, and others who want to be exact in their directions, use degrees to identify direction. The amount of turn is always referred to in a clockwise direction, starting from north 0º.

Write in degrees to identify each of the following directions.

DRAFT

a south-east

b south-west

c west

d north-west

Independent practice

This is a map of most of the North Island. Use it to complete the activities.

Kerikeri

Whangarei

Mangawhai

Warkworth

Auckland

Hamilton

New Plymouth

Te Ika-a-Māui

/North Island

Rotorua

DRAFT

Whanganui

Palmerston

North Napier

Scale

100 km

Upper Hutt

Wellington

1

Auckland is in B6. In which grid square is:

a Wellington?

b Palmerston North?

c Tauranga?

d Gisborne?

Gisborne

Tauranga

2

Of the sixteen points on a compass rose, estimate the closest direction to the one you would be heading in if you were in a helicopter flying from:

a Rotorua to Gisborne.

b Auckland to Plymouth.

c Plymouth to Wellington.

d Wellington to Napier.

3 A 360° protractor would be helpful for the following. In order to give a helicopter pilot accurate directions, give an accurate compass heading in degrees for the four flights in question 2.

a Rotorua to Gisborne

b Auckland to Plymouth

c Plymouth to Wellington

d Wellington to Napier

4

Explain why the 16 main compass points are not used as directions by pilots.

DRAFT

5 If a pilot were using the following headings, which town would she be flying to?

a a heading of 305° from Taurunga

b a heading of 45° from Napier

c a heading of 215° from Hamilton

6 Which two towns are at a heading of 350° from Auckland?

7 Taking note of the scale on the map, which town is approximately:

a 150 km SW of Gisborne?

b 65 km NW of Palmerston North?

c 80 km at a heading of 87º from Hamilton?

8 Approximate the heading and distance from Auckland to Wellington.

9 As can be seen from the map, the driving distance from Auckland to Wellington is greater than the flying distance. Estimate the driving distance by looking at the roads marked on the map. Then check how close you are by carrying out some research.

10 Crowell, on the South Island, is said to be the furthest that anyone can get from the coast in New Zealand. On a map, find the distance and then write in which general direction you would be heading to get to the coast.

11 In which direction and how far would you need to travel to get to the nearest coast?

Extended practice

DRAFT

The Cartesian coordinate system

It is said that the French philosopher, Rene Descartes, came up with the idea of the Cartesian coordinate system after watching a fly flit from place to place on his ceiling. Using the centre of the ceiling as the origin point, he “split” the ceiling into four quadrants (quarters) and imagined a grid drawn on the ceiling. He could then use grid references to describe any place that the fly landed.

1 In the Cartesian coordinate system, both positive and negative number values are used.

a Remembering to use negative numbers where appropriate, write the coordinates of the points that are plotted on this number plane.

2

b Reflect the triangle and enlarge it by 2:1.

c Write the coordinate points for the new triangle.

DRAFT

a On a piece of grid paper, draw a number plane by copying the axes on this page. Then, draw a shape by following these coordinate points:

b Draw a second shape by enlarging the shape you drew by 2:1.

c Write the coordinate points for the second shape. Begin by plotting the line in quadrant 1.

Data sources

Data sources

Nowadays it seems that every online shopping experience or service is accompanied by a survey asking us for our opinion: How did we do?

This data can be used for various purposes. For example, businesses can use it to find out how they can improve. Advertisers might use it to promote their products. If there is a lot of data, it is stored in a data dictionary. Like a dictionary, it is organised so that it will be easy to find a particular entry later.

There are two main ways of carrying out a survey: a census survey and a sample survey. In a census survey, everyone is asked their opinion. In a sample survey, only some people are asked.

Guided practice

b Give a reason for your answer in Situation J. Unit 10: Topic 1

1 a Would a sample or a census survey be more appropriate in the following situations?

Situation Sample or Census?

A The New Zealand Warriors want to know what the fans think of their ideas for a new team uniform.

B The parent of five children wants to know whether they would prefer a holiday at the beach or in the mountains.

C A City Council wants to know what the residents feel about a new Skate Park.

D The manager of a soccer team wants to know on which evening the players prefer to train.

DRAFT

E The Principal of a school of 450 students wants to know whether the parents want the students to have more homework.

F A Football Coach in Gisborne needs to know whether the team wants to play in a weekend tournament in Wellington.

G The Principal of St Dominic’s school wonders whether the parents want the school starting and finishing times to change.

H A TV presenter wants to find out whether the public would prefer a male or a female Prime Minister.

I A car manufacturer wants to gauge public opinion on five new car body colours.

J The Highlands Council wants to know whether the residents of a village want a new Skate Park, and if so, where it should be built.

Independent practice

The teacher of a Year 8 class asks, “Do you think there is a correlation between a primary student’s height and age?” After a little discussion, they decide that there is. The teacher then asks, “What about their height and their hand span?”

A student asks, “Could we find out about their favourite colour too?”

The class decides to do a survey, collecting quite a few pieces of information. They want to include every year group. These are the survey topics they decide on: Age Height

1 a Why do you think a census survey would not be appropriate?

b How many students from each year group do you think they should survey?

c How do you think the student(s) should be chosen?

DRAFT

d In order to test their reaction time, a ruler is held between a student’s fingers and is suddenly let go. The point where they catch hold of the ruler shows how quickly they react. Do you think this is a fair test?

2

This information is from a five-year-old.

a Add your own information to the table.

3

b Imagine if this survey were carried out across the whole school, with four or five students from each age group taking part. What sort of data dictionary do you think would be appropriate for storing the data?

4

A spreadsheet would be a useful way to store the data. With your teacher’s permission, carry out the survey using data from five people in your class. Enter the information into a spreadsheet. Here is a fictitious example for a group of five-yearolds:

DRAFT

When you have collected the data, find the average height of your survey group like this: Auto-sum function

a Click on the cell below the height column. (In this example it is cell C8.)

b Click on the Formulas tab in the menu.

c Click on the Auto-sum function and choose Average. The cells containing relevant data above will be highlighted.

d Press Enter or Return and the average height will be displayed in cell C8.

5 When conducting a survey about preferences for a school camp, a teacher could ask a closed question (Do you want to go to Wellington? ), an open question (Where would you like to go for the school camp? ), or a multi-choice option (Which of these camp options would you prefer? )

a What type of question would be the most appropriate if the following information were required?

Information required:

A We need to decide on a class item for the school concert.

B The Principal wonders whether parents like the school banner.

C A new school doesn’t know whether the students want blue, brown, green or grey for the school uniform.

Most appropriate type of question

b Imagine you had to do the survey in Situation A, but you did not want thirty different ideas. Write a good question that would give you the information you needed.

Extended practice

1 With your teacher’s permission, carry out a sample survey of each year-group in your school along the lines of the survey in the Independent practice section.

a Some things to think about before you begin:

DRAFT

• Would this be more appropriate to carry out as a partner, small-group or individual task?

• Would it be quicker if one person/group surveys one age group, another surveys another, and so on?

• If so, how will we collate/share the information at the end?

• How many people from each year group should be surveyed?

• Who will choose the sample in each year group?

• Do we need to add to or perhaps amend the list of information to be collected?

• How can we ensure that the very young children do not feel scared about what we are doing?

2

• How can we do this so that we do not disrupt the learning time of other classes?

• How can we collect the data neatly and accurately?

b Once the data has been collected, it needs to be put into some sort of data dictionary. How do you think this should be done?

In this unit, there have been examples of data that might be collected in a school and used for an interesting purpose. Graphs and tables are often used to display data in a visual way, making it easier to interpret.

Think of an interesting “real-life” situation, such as people’s ages and the games they play, or the amount of time people spend on their homework and so on. Think of an effective way to collect the information and then present it in an appropriate way.

DRAFT

This graph clearly shows I am the world’s most popular politician

Unit 10: Topic 2

Representing and interpreting data

Choosing the right way to display data is as important as collecting it from the right place.

Imagine you have surveyed people about their favourite snack food. You could draw your own graph, but which type? You could also enter the data onto a spreadsheet and let it prepare the graph (chart) for you. However, you would still have to choose the correct way to display it.

Guided practice

1 A spreadsheet program will display the information in various ways. Which of the graphs above do you think display the data appropriately?

DRAFT

2 This frequency table gives information on how students at Wataki Primary School get to school. It can be displayed on a histogram. A histogram is a quick way to display data when you need to give an accurate (but not numerically precise) view of the results. The data is correctly represented in one of these histograms, but the axes are not labelled. By studying the shape of the histograms, circle the one that is correct.

3 Noah recorded the temperature at 8 am on every day in the month of July. Would a line graph or a sector (pie) graph be a more appropriate way to display the data? Give a reason for your choice.

Independent practice

1 Noah decided to represent the data he collected for the 8 am temperatures in July on a dot plot. This gives us information such as the most common temperature for the month, but what important information can we not identify?

8am temperature in July

2 Hannah’s teacher gives out tokens when students do the right thing. At the end of the week, they can exchange their tokens for “free time”. This table shows the amount of free time that students can have.

No. of tokens Mins. free time

5< tokens >15 5

15< tokens >25 10

25< tokens >35 15

35< tokens >40 20

40< tokens >45 25

45< tokens >50 30

Use the information to complete the step graph that has been started.

DRAFT

3

This line graph shows the monthly school canteen sales of ice blocks and soup. Use the information to complete the activities.

a When were the highest sales of: i ice blocks? ii soup?

b In which month do you think the soup machine was broken?

c In which month were 115 ice blocks sold?

d Estimate the number of ice block sales in August.

e What is the mean number of ice blocks sold per month?

f Sales of both soup and ice blocks were low in January. Give the most likely reason.

g The canteen manager can’t decide whether to continue with soup sales in summer. What would your advice be? (Give a reason.)

DRAFT

h The profit on each is ice block is 75c. How much did the canteen make on ice blocks during the year?

4 Maia’s family drove from Kerikeri to Wellington. The travel graph gives information about their journey. Use the graph to complete the activities.

a How long did the journey take from start to finish?

b At approximately what time did they turn around and return home?

c At what time did they take the longest of their three rest stops?

d What was their average speed between noon and 18:00?

e At approximately what time did they move into slow-moving traffic?

5 Olive’s Uncle Bill came from England for a visit. At the time of his visit, he knew that £1 UK was equal to NZ$2.50. He wanted to keep track of what he was spending and made a conversion graph between NZ$ and £. Use the graph to complete the activities.

a Her Uncle gave Olive a £10 note. How much was worth that in NZ$?

b He changed £200 into NZ$. How much did that convert to?

c Uncle Bill bought a pen for NZ$15. He could have bought the same thing in England for £7. Was it better value to buy it in England or New Zealand?

d Using the pronumerals x for £ and y for NZ$, complete the algebraic expression that gives the rule for the conversion graph: y = x ×

Extended practice

Points can be plotted on a scatter graph to show the correlation, or connection, between one set of information and another. For example, in the previous topic you collected information about students of various ages in your school. From the data dictionary, you could show the correlation (if any) between a child’s height and age. The ages can be presented along the x axis of a graph, and the heights along the y axis to show the correlation between the two.

This scatter graph has been made from information in a data dictionary. Its purpose is to show whether there is any correlation between a young person’s age and the amount of pocket money they have.

1 Write a sentence or two about what you think the red dotted lines show.

2 The three plotted points that have a red arrow next to them are called outliers. What does this mean and what information do you think it shows?

DRAFT

3 Use the data that you collected in Unit 10, Topic 1 about students of various ages in your school.

a Decide on pairs of topics that you think show a correlation between age and another topic. (For example, age and shoe size).

b Represent the data, one pair at a time, on a scatter graph. If it does show a correlation, mark it as in the graph above. Comment on any outliers that you may find.

c Repeat the process for a pair of topics that you think will show no correlation between them. (For example, eye colour and head circumference).

Unit 10: Topic 3 Critical analysis of data

It sometimes seems that everywhere we look there is someone trying to tell us what to think, trying to sell us something or trying to persuade us what to do. Do they really want us to believe them or to trust them? It depends. The supporters of English Premier League team Manchester City know that they are not going to persuade supporters of other teams; they sing for fun. However, what about advertisers or politicians? Can we believe them or trust them? We need to think critically about claims that are made.

Guided practice

1 In the cartoon above, could it be true? It might be. Who did he survey? What did he ask? Suppose he asked five of his friends, “Would I be a good leader?” Four said, “I suppose so.” One said, “No!”

a Was his claim true?

b In what way was it misleading?

DRAFT

2 A teacher asked some Year 8 students to choose the sport they would like to do. The teacher gave three choices. A sector graph of the results was made and presented to all 72 students. The results show that two thirds of Year 8 prefer cross-country.

a Fill in the blanks to show the number of students that, according to the graph, prefer each type of sport:

Cross-country

Gymnastics

80% of the people surveyed think that I would be a great leader!

Sport preferred by Y8 students

CITY… CITY the best team in the land and all the world!

b In reality, only six students were surveyed. How many chose each type of sport:

Cross-country Tennis

Gymnastics

c The teacher said to the students, “Since the graph shows that the majority of you prefer cross-country, it will be the sport for everybody next term.” Comment on the validity of the graph.

3 How could the survey about sport in question 2 have been improved? Comment on: a the type of survey (census or sample).

b the survey question.

Independent practice

1 The police in Christchurch were concerned about the number of people who were speeding. In one afternoon, they caught 48 speeders. The ages of drivers caught speeding are shown below.

DRAFT

a Complete the graph to show the results.

b Complete the stem-and-leaf plot to represent the same data

c Compare the two ways of showing the information in terms of:

• time spent to make the graph.

amount of detail the graph can show.

• aesthetics (the look ) of the graph.

2 A local newspaper saw the data and wanted to make a sensational headline from it. They wanted to show that people under 30 are dangerous drivers, They made a histogram from the results:

Comment on their claim: “Our research clearly shows that teenagers and people in their sixties are less careful than people in their fifties.”

3

20-somethings are the world’s worst drivers

<2020–2930–3940–4950–5960–6970–7980–89

The next day, the newspaper made further claims. The headline said: “40% of our population drives dangerously – GUESS WHO?”

They based claims on the information in the graph. The newspapers statisticians worked out the percentage from the data.

DRAFT

a How did they arrive at a figure of 40%?

b The editor wanted numbers to back up the claims that people in their 20s are not good drivers. The newspaper’s researchers set to work. Their rounded research numbers showed that there are around 700 000 New Zealanders out of a rounded 5 million in their 20s. Approximately what percentage is that?

c The next headline read: “Over 250 000 dangerous drivers on our roads – GUESS WHO?”

How did the researchers arrive at that figure?

4 Based on their “research” the newspaper article concluded: “People in their 20s make up almost half of our law-breakers.”

How is the headline misleading the readers?

5 Comment on another conclusion from the data that: “The safest age to be a driver is when you’re over 70.”

Extended practice

1 If a company tells a lie in its advertising, it is breaking the law. You may have seen signs outside a clothing shop like this:

a Why do you think the words up to are in smaller letters?

b How many of the items in the shop do you think are discounted by 70%?

c Why do you think shop owners show advertisements like that?

2 Some supermarkets have been found out trying to trick their customers. They put this sign next to some of the products.

DRAFT

a If the original price was $9.99 and there is a 15% saving, what will the new price be?

b The trick they played was to deliberately put the price up two weeks before the price drop week. Explain why the “bargain” might not really be a bargain.

c If the original price was $7.99, comment on the way that the store has mislead the customers.

3 Misleading advertising slogans can often be seen.

• Buy one, get one free (second item must be less than $5)

• Buy today – Go into our SUPER-DRAW (to win what?)

• Less sugar (than what?!)

• Fat Free (mineral water)

Carry out some research to find examples of false, or misleading examples.

DRAFT

Unit 11: Topic 1

Describing probabilities

You may have enjoyed a game such as Snakes and Ladders when you were young. In games like that, although there is a possibility that you will win, there is a probability that you will lose, especially if there are several players. Your chance of winning depends on the way that the dice lands. If you’re playing a game of tennis, on the other hand, then your chance of winning is based more on your skill level than luck.

When we are describing the probability of something happening, we can use words such unlikely, highly likely and so on. At other times it is important to be more precise. There are several ways, for example, to show numerical probability for a coin to land on heads, such as 50–50.

Guided practice

1 TV stations often predict the weather in terms of probabilities. Assign a percentage probability to each of the following predictions.

Weather prediction

A It might rain tomorrow and it might not.

B There’s a slight possibility of a shower in the morning.

C There’s a strong chance of a storm in the evening.

D There’s no sign of rain for the next week.

E There’s a good chance of strong winds later.

DRAFT

% Chance

2 The sample space of the way a coin can land is heads and tails, since there are just two possible outcomes. We can list the sample space for events. List the simple space for the following. The first one has been completed.

What are the possible outcomes for: Sample space a the coin?

Sample space = {heads, tails} b the die?

d choosing a particular bouncy ball?

3

What is the probability for each outcome of the situations in question 2? The first one has been completed. P() shows the probability of an event. For example, P(heads) means the probability of getting heads. When rolling a die, P(1) means the probability of getting 1.

Situation

Probability for each element

a Coin P (heads) = 1 2 , P (tails) = = 1 2

b Die

c Spinner

d Bouncy ball

Independent practice

1 The suits in a pack of cards are Hearts, Diamonds, Clubs and Spades

a List the sample space for the suits of a pack of cards.

b What is the probability for each element?

c Looking at each of the probabilities as a fraction, what is the sum of the four probabilities?

2 A computer randomly chooses letters from the word M I N I M U M

a List the sample space for the letters.

b What is the probability of the different letters being selected?

DRAFT

c Explain why the sum of the probabilities should be one.

d After 21 computer picks, how many of each letter would you expect there to be?

3 Try a chance experiment with the word M I N I M U M

a Write each of the seven letters on the face of seven identical, small pieces of paper or card (ensure that the writing cannot be seen from the reverse through to the front.)

b Shuffle the papers around face down.

c Have someone randomly choose a letter and make a tally mark on a tally sheet of the letter that has been picked.

Tally

d Shuffle the papers around again.

e Repeat steps c and d.

f Decide how many times the experiment should be repeated for the results to be accurate.

g Discuss the results with a partner.

4 The likelihood of something taking place can be shown by assigning it a numerical value between zero and one. Write an informal description for the probability of the following events.

What are the chances I will … Informal prediction

A visit a friend? 0.5

B play cricket tonight? 0

C receive extra homework today? 0.2

D receive a present this year? 0.95

E try my best in an assessment? 1

5 Using the information in question 2, assign a numerical value between 0 and 1 for the likelihood of each of the letters being chosen. Round decimals to an appropriate number of places. Ensure that the sum of the possibilities is 1.

DRAFT

6 If you were playing a game and needed a “six” on a die, the probability of getting the number you wanted would be one out of six. The chances of the number not being six would be five out of six.

We call these complementary events. We could write the complementary events for getting a six on a die like this: P(six) = 1 6 , P(not six) = 5 6

What are the complementary events for this spinner stopping on 3? 23 1

7 List the sample space for the following events.

a whether a baby is born a boy or a girl Sample space = { }

b 23 14 the way the pointer will stop c the result of a cricket match

d the day of the week of the next shower of rain

8 If the spinner in question 7 looked like this:

a how would it change the sample space?

b The probability for each element would change. Explain why and list the new probabilities.

Have you ever played a board game in which you needed to get a six and it never seemed to come up, no matter how many rolls of the dice you made? In those times it seems hard to believe that the six has the same chance as the other numbers. You probably know, however, that in board games where two dice are rolled together, some numbers do have less chance than others.

1 List the sample space for the numbers that can be obtained when two dice are rolled. Afterwards, list the probabilities for each number and finally comment on the results.

2 A car is about to go around a bend and will approach some traffic lights.

a List the sample space for the colours the lights could show.

b Explain why the probability of the colour being yellow is not 1 3

c Estimate the probability of the light being yellow.

3 Read the words and phrases that can be used to describe probability. Copy the corresponding letter onto the number line in the appropriate place. Two have been suggested for you.

A Impossible G A strong possibility

B Probable H Highly unlikely

C Certain I Highly likely

DRAFT

D Not a very good chance J Even chance

E Hardly any chance K Improbable

F A fairly good chance

00.10.20.30.40.50.60.70.80.91 A C

4

Express the probability of the following events occurring as a fraction, a decimal and a percentage.

Probability as a:

a Rolling a 2 on a die

b Getting a 4 on this spinner

c Getting a 2 on this spinner

d A coin landing on its edge

5 Challenge: Does anyone in your class share the same birthday as you? Did you know that in a class of 30, there is an almost 70% probability that 2 people will share the same birthday? In a group of 70, the percentage increases to 99.9%!

Try to find an easy-to-understand formula online for working out the probability of two people sharing the same birthday.

Answers

Unit 1: Topic 1

Guided practice

1 fifty-three thousand, six hundred and fiftyfour

2 2000 + 20 + 4

3 102 549

4 a 2.45, 100, 2024, 53 654

b 345, 34.5, 3.45

c 5 4 , 53, 52

5 a > b < c < d <

e It’s not possible to use either symbol because the numbers are the same size.

Independent practice

1 a twelve thousand, one hundred and forty-five

b twelve point one, four, five

c twelve thousand, one hundred and forty-five

d twelve point one, four, five

2 a 103 b 105 c 104

d 45 e 97

3 a fifty thousand b fifty million

c seven hundred thousand d one billion

4

a 5M

b 1.25M

c 1 2 of 5

e 5 ×

5 a five million

b fifty thousand

c five tenths

d fifty thousand

e five hundred thousand

f fifty million

g fifty billion

h five hundredths

6 a 0.025, 0.25, 2.5, 25, 255

b 0.35, 3.53, 3.55, 3500, 3.5M

c 70.005, 70.05, 75 000, 750K, 750M

d 0.47, 4.07, 4.7, 4.704, 7.4

7 a 355, 35, 3.5, 0.35, 0.035

b 8.5M, 8500, 8.58, 8.55, 0.85

c 250B, 20.5M, 250K, 25 000, 2005

d 30.5, 30.05, 3.05, 0.35, 0.035

8 a > b < c >

d > e < f >

Extended practice

1 a 2000 b 19 000 c 1000

d 33 000

2 a 32 800 b 32 830

3 Answers may vary. For example, thousand because that would take the amount up to $23 000.

4 a 39 000 b 41 000 c 26 000

d 451 000

5 a 200 000 b 400 000 c 300 000

d 1 100 000

6 a 1.8 b 0.5 c 37.1

d 8.0

7 a Answers may vary. For example, it is too difficult to measure it exactly.

b Answers may vary. For example, the orbit of the Moon is not a constant circle.

Independent practice

1 a 2 and 5, 10 = 2 × 5

b 3 and 3, 9 = 3 × 3

c 3 and 5, 15 = 3 × 5

d 3 and 7, 21 = 3 × 7

e 5 and 7, 35 = 5 × 7

f 3 and 13, 39 = 3 × 13

2 a 2 and 5 (20 = 2 × 2 × 5)

b 2 and 3 (18 = 2 × 3 × 3)

c 2 and 7 (28 = 2 × 2 × 7)

d 2 and 3 (36 = 2 × 2 × 3 × 3)

3 a 2 and 17 (68 = 22 × 17)

b 3 (27 = 33)

c 2 and 5 (40 = 23 × 5)

d 2 and 7 (56 = 23 × 7)

Extended practice

1 a 1, 2, 4, 8 and 1, 2, 3, 4, 6, 8, 12, 24

b Student circles 1, 2, 4, 8 for each number.

c Student puts a star over number 8.

d 8

2 a HCF = 8

b HCF = 7

c HCF = 2

d HCF = 6

3 a 3 4 b 2 3

c 1 5 d 4 7

4 35

Factors of 210: 1, 2, 3, 5, 6, 7, 10, 14, 15, 21, 30, 35, 42, 70, 105, 210

Factors of 315: 1, 3, 5, 7, 9, 15, 21, 35, 45, 63, 105, 315

Unit 1: Topic 2

Guided practice

Unit 1: Topic 3

Guided practice

1

2

c

d

e

3 Starting number What times itself makes the number? The square root of the starting number is: Write the number fact.

4

DRAFT

1

c

g

2

3

4

Unit 2: Topic 1

Guided practice

1 Problem My estimate

a

b 24 895 + 19 778 25 000 + 20

c

e 1 4 of 836 800 ÷ 4 = 200 209 Yes

f

h

i

2 a 3.92 b 32.76 c 43.81 d 70.44 e

j 99.99

Independent practice

1 Student circles d and e.

2 Student circles b, d and e.

3 25 000 km2

4 25 445 km2

5 Answers may vary. Check that the estimate matches the rounding. For example, I rounded to the nearest ten thousand. 270 000 + 240 000 = 510 000 km2

6 512 665 km2

7 11 000 km

8 Estimate: 1000 km, Actual: 1056.9 km

9 411 000 km

10 Estimate: 23 000 km, Actual: 22 950 km

Extended practice

1 a one third

b 32.5%. Methods may vary. For example (1693 ÷ 5214) × 100 = 32.4702 = 32.5% or (1 693 000 ÷ 5 214 000) × 100 = 32.4702 = 32.5%

Unit 2: Topic 2

Guided practice

1 a 184 b 900

c 570 d 3123

2 a 245 b 1230

c 600 d 8991

Independent practice

1 Students could share their solutions with the group.

a 160 b 300

c 180 d 210

2 Students could share their solutions with the group.

a 85 b 168

c 148 d 280

3 a 500 b 5000

c 5 tenths or 0.5 d 5000

4 a 360 b 37 × 20

Unit 2: Topic 3

Guided practice

1 a 2 b 2

c 3 d 2

2 a 8 b 1

c 0 d 7

3 a 5 b 9

c 8 d 9

4 a 1 b 5

c 2 d 5

5 dividend

Independent practice

1 C, A, B

DRAFT

c Answers may vary. Students could be asked to justify their response to others in the group.

2 104M (If 5% is 5.214M, then 1% is 1.0428M × 100 = approximately 104M)

3 210 000

4 a Wellington b 319.6%

5 Answers will vary. Students could be asked to justify their response to others in the group. For example, it was just over four times bigger in 2024 than it was in 1955. Or the population increased by around 1.3M between 1955 and 2024.

6 Answers will vary. Students could be asked to justify their response to others in the group. For example, the population changes from day to day.

7 approximately 1600 km by 448 km

+ 53 × 6

Extended practice

1 a 675 b 918 c 741

d 1786 e 1950 f 6132

2 No, because she has only saved 15 × 13 = $195.

3 a–c Answers will vary.

4 a 527 times b 1037 times

c 6222 times

5 a 426 L b Answers will vary.

2 a 9176 2 7 b 9816 1 2

c 7247 1 3 d 6593 1 3

3 a 7134.25 b 12 392.33

c 9423.63 d 8334.43

e 16 392.67 f 55 073.8

4 a 21 b 19

c 35 r1 d 28 r2

e 24 r3 f 37

g 235 h 326 r2 i 658 r3

Extended practice

1 a 12 b 26

c 28

2 True (68 × 2 = 136)

3 False (17 × 4 = 68 kg)

4 36 (145 ÷ 4 = 36.25)

5 5.14

6 7.83

7 a 9 (4000 ÷ 440 = 9.09)

b 114 (68 000 ÷ 595 = 114.3)

c 132 (145 000 ÷ 1100 = 131.8)

Unit 2: Topic 4

Guided practice

1 Answers will vary.

Independent practice

1 a 13 b 10

c 9 d 4

e 53 f 32

g 4 h 36

i 40 j 49

k 7 l 16

m 51 n 3

o 48 p 24

2 a 24 ÷ 4 + 2 ≠ 24 ÷ (4 + 2)

b 2 × 42 = 42 × 2

c 3 × 6 − 3 ≠ 15 + 2 × 5

d 5 × (4 + 1) = 52

e √9 × 32 ≠ 33 f 18 ÷ 2 + 42 ≠ 18 ÷ (2 + 42) g 7 + 3 × 2

3 a 15 ÷ ( 1 2 of 10) + 2 × 5

15 ÷ 3 + 2 × 5

5 + 2 × 5

5 + 10 15

b 3 × (2 + 4) + 15 ÷ 3

3 × 6 + 15 ÷ 3

18 + 15 ÷ 3

18 + 5

23

c 18 ÷ 9 + 1 2 of 10 − 2 × 3

18 ÷ 9 + 5 – 2 × 3

2 + 5 – 2 × 3

2 + 5 – 6

1

4 a 70 ÷ (10 + [3 + 2] × 5)

= 70 ÷ (10 + 5 × 5)

= 70 ÷ (10 + 25)

= 70 ÷ 35 = 2

b 5 × ([10 ÷ 2] + 22)

= 5 × (5 + 22)

= 5 × (5 + 4)

= 5 × 9 = 45

c 6 + (12 ÷ [8 - 22])2 – 1

= 6 + (12 ÷ [8 – 4])2 – 1

= 6 + (12 ÷ 4)2 – 1

= 6 + 32 – 1

= 6 + 9 – 1 = 15 – 1 = 14

Extended practice

1 40 + (1 4 of 40 – 2) – 3 × 3

= 40 + (10 – 2) – 3 × 3

= 40 + 8 – 3 × 3

= 40 + 8 – 9

= 48 – 9 = 39

She has $39 left.

2 a $82

3 × 12 + 40 + 4 × 1.5

= 36 + 40 + 4 × 1.5

= 36 + 40 + 6 = 82

b He saved $3.40.

3 × 12 + (40 – 1 10 of 40) + (4 × 1.5) + (4 × of 1.5)

= 36 + (40 – 4) + (4 × 1.5) + (4 × 1 10 of 1.5)

= 36 + 36 + (4 × 1.5) + (4 × 1 10 of 1.5)

= 36 + 36 + 6 + (4 × 1 10 of 1.5)

= 36 + 36 + 6 + (4 × 0.15)

= 36 + 36 + 6 + 0.6 = 78.6

3 $503.50

4000 − (3 × 1295 − [ 1 10 of 1295 × 3])

= 4000 − (3885 − 388.5)

= 4000 − 3496.5

= 503.50

Note: students may have learned that ‘of’ is worked through before ‘multiply’, giving the following steps:

4000 − (3 × 1295 − [ 1 10 of 1295 × 3])

= 4000 − (3 × 1295 − 129.5 × 3)

= 4000 − (3885 − 388.5)

= 4000 − 3496.5

= 503.50

4 = [(6 x 90) – (6 x 1 4 of 90)] ÷ 3 = [540 – (6 x 1 4 of 90)] ÷ 3 = [540 – (6 x 22.5)] ÷ 3 = [540 – 135] ÷ 3 = 405 ÷ 3 = $135

Unit 2: Topic 5

Guided practice

8 a colder b 7º

c warmer d 16º

9 a −2 b −5 c 1

d −9 e −3 f −1

Extended practice

1 a Singapore, 18º b Norway, 86º c Japan d 68º

2

e −31.1ºC

Date Credit $ Debit $ Balance $ June 1 500 500

June 3 195 160 535 June 5 235 245 525

June 7 305 420 410 June 10 95 540 −35

3 Answers will vary.

Unit 3: Topic 1

Guided practice

3 a 21%, 0.25, 210, 2.5 b 3%, 0.3, 30%, 1 3 c 12 1000 , 0.12, 12.5%, 1.2

Independent practice

1 a 10 20 = 1 2, 0.5, 50%

b 15 20 = 3 4 , 0.75, 75%

c 6 20 = 3 10, 0.3, 30%

d 18 20 = 9 10, 0.9, 90%

e 5 100 = 1 20, 0.05, 5%

1

2

Unit 3: Topic 2

6 a Answers may vary.

7 This could be a useful topic for discussion. For example, a $1M prize money divided between three winners.

Unit 3: Topic 3

Guided practice

1 a 468 and 46.8

b 744 and 74.4

c 1743 and 17.43

2 Student shades 3 8 of each rectangle and writes

Extended practice

Unit 3: Topic 4

Guided practice

1

3

Guided practice

1

b Answers will vary. For example, he owed $5 to Maia.

c Answers will vary.

2 Students could share and compare their ideas for the budget, perhaps being asked to justify their answers.

3 “Being in debt” means you owe a sum of money.

Independent practice

1 a Income: $1164.95, Expenditure: $1064.95

b Yes, because she earns more than she spends.

2

4

d This would be a good discussion point for the group. (The actual figures are fictitious, but it should prove to be a springboard for discussion about the way that it is possible for credit card spending to lead to bad debt.)

2 This would be another useful topic for discussion.

Unit 4: Topic 2

Guided practice

1 This would be a good topic for a group discussion.

2 a $5 b $8 c $7 d $5

e $4 f $3 g $2

3 Answers will vary.

4 a $0.45 b $0.75

c $0.40 d $1.25

Independent practice

1 a 1 10 b 1 10 c 1 5 d 1 10

e 1 100 f 1 4 g 1 8 h 1 5

2 a For example, find 10% then 5% and add together.

b For example, find 25% and then × 3.

3 a 73 100 b 17 100 c 31 100 d 43 100

4 a $1.10 b $1.90

c $52.00 d $5.50

e $4.80 f $9.60

5 Student circles c & e.

6 a $4.40 b $7.60 c $20.80

d $11.05 e $21.60 f $24.00

7 a & b $0.45

8 a $1.54 b $0.98

c $0.45 d $8.42

e $1.55 f $9.09

g $921.50 h $656.89

Extended practice

1 a Answers will vary. This is a good discussion point.

b one

2 Estimate: $800 Calculator answer: $825.55

3 a $1.08

b $12.52 ($12.50)

4 $49.07 ($49.05)

5 a 31.2 m

b x = 195 – 31.2 × 5

c 195 – 31.2 × 5 = 39 m d Tuesday 3

Unit 5: Topic 1

Guided practice

1 a b = 24 × a

b

3 a 19

b 23.13 recurring

c 271 2 d 132.135

4 17 and 19

5 a 8 b 20 c 9

6 a 5 b 4 c 8 d 6

Extended practice

1 98

DRAFT

2 406, 1792, 1001

3 319

4 8 × 40 + 366 = 406. 406 ÷ 7 = 58. So, 7 is a factor of 3668.

5 2996:

299 + 6 x 5 = 329

32 + 9 × 5 = 77

7 + 7 × 5 = 42

4 + 2 × 5 = 14

1 + 4 × 5 = 21

2 + 1 × 5 = 7

7 is a factor of 2996.

6 This is a good discussion point.

Unit 5: Topic 2

1 11, 19, 33, 71, 79

2 4 (2 is prime because its only 2 factors are 1 and itself.)

3 a 912, 735

b 2384, 3896

Independent practice

1 a 37 416, 9 999 990

b 475 362, 7 345 296

c 495 372

d 6 513 470, 37.35

e 950 724, 1 836 324

f 41 072, 65 392

Number of months a 1 2 3 4

Amount of growth (cm) b 24 48 72 96

c b = 24 × 12 = 288 cm

2 a Choice of pronumerals might vary, but should match answer to the next question: b = 20 × a

b

Number of hours a 1 2 3 4

Number of pizzas b 20 40 60 80

Independent practice

1 a 4 b 5 c 12 b 6

2 a 27 + (21 + 19) = 27 + 40 = 67

b 13 × (5 × 4) = 13 × 20 = 260

2

g 16 353, 484 209 h 2 001 390 i 4536, 38 052

Number Besides itself and one, circle any other factors (12 and under) Is it prime or composite?

105 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

127 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

297 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

181 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

223 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

287 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

323 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12

Composite: (3, 5, 7)

Prime

Composite: (3, 9, 11)

Prime

Prime

Composite (7)

Prime (but see below – Q3)

Unit 5: Topic 3

Guided practice

1 a 3w b 7b

c 10y d 1.5z

2 Answers will vary. For example, because the pronumeral x might be confused for a multiplication sign.

3 9 × w = 9w, 2 × b – 3 = 2b – 3, 2 + b × 3 = 2 + 3b, 9 × w × w = 9w2, 2 × b + 3 = 2b + 3

4 a 5a b 3x + 4y

c w d 2c + 9d

Independent practice

1 a 5(j + 3)

b 3(4 − b)

c a (b2 + 4)

d 3(5 – y )

e 3 × 4 × f (z – 2)

2 a y 5 b b 3 c 5 z d 2a 3

e ab 3 f c 2 g ac 4 h 3×4 4

3 a 12

b 5b − 10

c 2d − 4

d c + 5a + 20

4 a 4

b 4

c 12 ÷ 3 = 4

5 a 8 + 12 = 20

b 25 – 10 = 15

c 2d – 8 = 6 – 8 = –2

d c + 5a + 20 = 8 + 10 + 20 = 38

Extended practice

1 Answers may vary. For example, the pronumeral outside the grouping symbol needs to be multiplied by the pronumeral inside the grouping symbol only once.

2 a and b

3 c is not a correct formula because, if z = 4, the number of matchsticks would need to be 20 but it is not!

4 a both A and B b 21

5 Teacher to check.

Unit 5: Topic 4

Guided practice

1 a 5 b 10

Independent practice

1 a b +5 – 5 = 25 – 5. So, b = 20.

b y − 4 + 4 = 12 + 4. So, y = 16.

c m + 3 – 3 = 20 – 3. So, m = 17.

d n – 2 + 2 = 14 + 2. So, n = 16.

2 a x = 30

b x = 20

c x = 7

d x = 28

e x = −10

f x = 75

3 a 24

b 58

c 58

4 a 8a = 100. a = 100 ÷ 8 = 12.5. Noah is 12 1 2 years old.

b s 4 = 37. s = 37 × 4 = 148. Ari has 148 stickers.

Extended practice

1 a 13 b 25 c 8 d 2 e 11.5 f 9 g 1.5 h 2.5

2

3 Any number less than 4.

4 Because 14÷ 3 is less than 5.

5 a–c Both equations will solve the problem. The older girl is 10 and the younger is 7.

6 3(p + 2) = 21, 3p + 6 = 21, 3p = 21 – 6, 3p = 15, p = 5

Unit 5: Topic 5

Guided practice

1 a 16, 32, 64; double the previous number b 131, 100, 81; go down to the next square number

c 64 27, 8; go down by cubic numbers

4 a Either: y = 1 + (3 × z ) or y = 3 × z – (z – 1) b y = 1 + 30 × 3 = 91

5 a Yes, it is true substituting 16 for y : z = (16 − 3) 3 , = 15 3 = 5. Yes, 5 squares can be made from 16 sticks.

b There is not an exact number of squares. z = (17 − 1) 3 , = 16 3 = 5.33

c For example, the number of sticks has to be a multiple of 3, plus 1.

6 It would be a lot simpler. The formula would just become z = 3y

7 Students draw a pattern of squares in which there are no adjacent sides.

Extended practice 1

of

2 You need 5 for the first one, the 4 for every subsequent pentagon; 49

c 49

3 a 20, 35, 50, 65 b 5, 14, 41, 122

5

Independent practice

1 a Either: a = 1 + (b × 2) or a = 3 × b

(b – 1)

of matches used

3 Number of pentagons made Pattern of Pentagons

4 It is a straight line.

5 z = 4y + 1

Pattern of Squares

Number of matches used

of squares made

Unit 5: Topic 6

Guided practice

1 a B4

b Student writes 3 in A2 and 4 in B2.

2 Practical activity

Independent practice

1 & 2 Practical activity

3 a–d Practical activity

e The list would be alphabetical, but the numerical data wouldn’t match the original list (depending on the program, an error message may appear).

4 Practical activity

5 Add Subtract Multiply Divide Equals + * / Enter/ return

6–9 Practical activities

Extended practice

1–4 Practical activities

Unit 6: Topic 1

Guided practice

1 107

2 a 0.2 kg b 0.375 kg

c 1.25 kg d 0.001 kg

e 0.02 kg f 0.95 kg

g 1.75 kg h 0.01 kg

3 a 1300 mL b 1250 mL

c 350 mL d 2900 mL

e 50 mL f 10 250 mL g 5 mL h 100 000 mL

4 1325 L

Independent practice 1

Extended practice

1 a 3.6 m × 1200

3 Area needed is: 2 × 3.8 × 2.7 plus 1 × 0.59 × 2.4 (they have to buy the full piece for over the door and cut it down.) Total area of board = 21.936 m2. 21.936 × $39.50 = $866.47

Unit 6: Topic 2

2

2 a 12.5 seconds

b 8 m/ps

3 a walker: 40 seconds, Julia: 60 seconds

b walker: 2.5 m/ps seconds, Julia: 1.7 m/ps

4 9.58 seconds

5 & 6 Practical activity

7 a 1.5 km

b Her displacement is 50 m because she is only 50 m from her starting point.

Unit 6: Topic 3

Guided practice

1 a 12.5 cm2 b 12.25 cm2

2 a 45 cm3 b 140 cm3

c 128 cm3

Independent practice

1 a 30 cm2 b 30 cm2

c 28 cm2 d 30 cm2

2 Student circles all except E.

3 base length

4 b × h = 3 × 3 = 9 cm2

5 a 100 b 10 000

c 10 000 d 100

e 10 000

6 a Yes b No

c Yes d Yes

7 a 2 b 20 000

c 3.5 c 40 000

e 1 million f 5

g 2500

8 Answers may vary, e.g. I used the formula

l × w × h and the answer is 0.125.

9 the height

10 b × h 2 × l = 48 cm3

11 120 cm3

12

DRAFT

Guided practice

1 a 30 km (15 × 2 = 30) b 10 km (20 × 0.5 = 10)

2 12 km/h (24 ÷ 2 = 12)

3 1 1 4 hours (18 ÷ 12 = 1.5)

Independent practice

1 500 km

2 15 minutes

3 24 km/h

4 a 9 km/h

Extended practice

1 a 78.57 cm2 b 28.29 cm2

c 154 cm2

2 a 452.58 cm3

b 942.86 cm3

c 113.14 cm3

Extended practice

1 a 10 m/ps

b 16.7 m/ps

c 5 m/ps

Unit 7: Topic 1

Guided practice

1 a Number of pairs of equal sides Number of pairs of parallel sides Are all sides equal? Are adjacent sides perpendicular? Do the diagonals intersect? Do the diagonals bisect each other at right angles? Does it have rotational symmetry? (What is the order?) Name the shape.

a 2 Yes – 2 pairs Yes

b 0 1

c 0 0

d 2 2

e 2 2

f 0 0

g 2 2

h 2 0 no

b 4 angles and 4 sides

c e.g. F has a reflex angle.

d Both have 4 right angles but only the square has 4 equal sides.

Independent practice

kite

1 a Is it acuteangled? Is it obtuseangled? Is it rightangled? Is it a scalene triangle? Is it an isosceles triangle? Is it an equilateral triangle? Does it have rotational symmetry? Is (are) the longest side(s) opposite the widest angle(s)?

A

B

C

D

E

b e.g. If the triangle has a side or sides longer than the others, it (or they) are always opposite the widest angle(s).

2 equilateral triangle

3 Student draws and names a trapezium, and marks the pair of parallel lines.

4 Student draws and names a rhombus. The diagonals form four right angles.

Extended practice

1 a Minorsegment A B C D Chord Major segment Tangent

2 Teacher to check.

Unit 7: Topic 2

Guided practice

Independent practice

1 a ∠ ABD

b Student marks ∠ ABD.

c Because there are two angles that have B as their vertex.

2 a 55°

∠YXZ and ∠ XZY

3 Name the marked angles Are the angles adjacent? Are the angles complementary? Are the angles supplementary? Calculate the size of the unknown angle.

a ∠WXZ and ∠YXZ Yes No

b ∠ ABD and ∠CBD Yes Yes

c ∠VWX, ∠VWX and ∠ XWY Yes No

4 a either ∠ AEC and AED or ∠CEB and DEB b 30°

5 a 60° b 80°

c 70° d 270°

6 a 75° b 75° c 105°

Extended practice

1 a i alternate and equal ii supplementary and adjacent iii complementary and equal iv vertically opposite and equal v complementary and equal b i 125º ii 55º iii 125º iv 125º

2 a 110º b 70º c 110º

3

M or

Unit 8: Topic 1

1

DRAFT

b 8 – 4 = 6 edges

c triangular

3 a Student draws a

d Because the side faces of all prisms are rectangles.

4 Teacher to check.

Extended practice

1 Description Geometrical Name A shape like this in the environment

A Its cross-section is uniformly circular.

B Its cross-section is uniformly triangular. triangular

C It has a circular base and an apex that is at a uniform, fixed distance from the centre of the base.

D Its base has 4 vertices and its 5th vertex is at a fixed, uniform point from the centre of its base. square-based pyramid

E Every point on its surface is at a fixed, uniform distance from its centre. sphere

Independent practice

1 Is it a right prism?

B

C

D

E

F

G

3 The five Platonic solids are the tetrahedron (or triangular pyramid), cube, octahedron, dodecahedron, and icosahedron. Each of their faces is from the same type of polygon. All the edges are the same, and all of them join two faces at the same angle.

Unit 8: Topic 2

Guided practice

1 a rotation (reflection could be justified)

b translation

c reflection

2 1:2

3 Answers will vary. Students could share their responses.

Independent practice

1 Teacher to check patterns. (Shapes are coloured to simplify the identification of the patterns.) Examples of possible descriptions:

A The shape has been reflected horizontally on the first row. The second row is a vertical reflection of the first row.

B The shape is rotated through 180º clockwise on the first row (or has been reflected horizontally then vertically on the first row). The second row is a vertical translation of the first row.

2 Teacher to check.

3 Teacher to check.

Extended practice

1 a (−1,3)

b Student draws the circle at (2,4).

c (2,4)

d Student draws the hexagon at (3,1).

e 6 places to the right and 1 place down

2 Teachers to decide on level of accuracy.

a ESE b SSW

c SSE d NE

3 Teachers to decide on level of accuracy.

a 110° b 194°

c 166° d 42°

4 Answers may vary, e.g. because they would miss their destination if flying at night or in bad weather.

5 a Auckland

b Gisborne

c Plymouth

6 Mangawhai and Warkworth

7 a Napier

b Whanganui

c Tauranga

8 500 km at a heading of 180º

9 644 km (Google maps)

10 around 120 km north-west or south-east

11 Answers will vary.

Extended practice

1 a A = (3,9), B = (−7,4), C = (−8,−9), D = (0,−8), E = (7,−8) b

x –2–3–4–5

2 a It has been translated 2 places to the left and 1 place down.

b

c (4,0), (−4,) and (0, -8)

Unit 9: Topic 1

Guided practice

1 (clockwise from east) ESE, SSE, WSW, NNW

2 a 135° b 225°

c 270° d 315°

Independent practice

1 a B1 b C2

c D5 d F4

(8,0) – (0,8) – (−8,0) – (0,−8)

Unit 10: Topic 1

Guided practice

1 a Answers may vary, e.g. A: sample, B: census, C: sample, D: census E: discussion point, F: census, G: discussion point, H: sample, D: sample J: census. Possible discussion point.

b Answers may vary. Possible discussion point.

Independent practice

Extended

practice

1 a Practical activity

b This could be solved in a group discussion, with possibly each group entering its data on a class spreadsheet that would become the data dictionary. See Unit 10 Topic 2 for ideas on how to actually use the collected data.

2 Practical activity

3 a February, August

b September

c Dec

d 5

e 57.5

f school closed

g Possible discussion point.

h $517.50

4 a 12 hours

b 6:15 (approx.)

c 12:00

d 66.7 km/h

5 a $25

DRAFT

Unit 10: Topic 2

1 a because it would take too long

b Possible discussion point.

c Answers may vary, e.g. choose an “average” student.

d Possible discussion point. This is a common “reaction time test”. However, students may decide on a better test.

2 a Teacher to check.

b Answers may vary. For example, the sheets could be turned into a huge chart, or a spreadsheet could be used.

3 Practical activity

4 Practical activity

5 a Possible answers: A open, B closed, C multi-choice

b Answers may vary, possible discussion point. E.g. Would you prefer a dance, singing or drama item?

Guided practice

1 Students could be asked to justify their response. Most likely are column and pie (sector) graphs.

2 the middle image

3 A line graph would show the way the temperature changed day by day during the month.

Independent practice

1 The days on which each temperature occurred.

e approximately 17:10

b $500

c New Zealand

d y = 2.5x

Extended practice

1 They show that there is generally a correlation between the amount of pocket money and a young person’s age: the older they are, the more money they get.

2 The data lies outside the normal data. It shows that two young children get more than is normal and that one older person gets less than is normal for that age.

3 Students could make digital representations of the data and examples could be displayed. It may be interesting for them to share their results with other age groups in the school.

Unit 10: Topic 3

Guided practice

1 a Yes

b Answers will vary. E.g. He only asked a few friends.

2 a Cross-country: 48, Tennis: 12, Gymnastics: 12

b Cross-country: 4, Tennis: 1, Gymnastics: 1

c Potential discussion point. Students could share their opinions.

3 a Answers may vary. E.g. census or a larger sample.

b Answers may vary. Potential discussion point. Students could share their ideas. E.g. Make it an open question or, at least, give more options.

practice

Unit 11: Topic 1

Guided practice

Extended practice

1 Sample space = {2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12} (no possibility of 1)

Probabilities: P (2) = 1 36, P (3) = 1 18, P (4) = 1 12 , P (5) = 1 9 , P (6) = 5 36, P (7) = 1 6 , P (8) = 5 36, P (9) = 1 9 , P (10) = 1 12 , P (11) = 1 18, P (12) = 1 36

2 a Sample space = {red, yellow, green}

b Because the time on amber is less than for red or green.

c Answers will vary, e.g. 5%. Possible discussion point.

c Potential point for group sharing and discussion.

• The column graph takes much longer.

• The stem-and-leaf shows the ages of individuals.

• This is personal choice.

2 Answers will vary. For example, the sample of people who were caught speeding is not large enough for that conclusion.

3 a 19 out of 48 speeders were in their 20s which is almost 40%.

b 14%

c They calculated it from the % of speeders and the number of people who are in their 20s (40% of 700K = 280K).

2 b Sample space = {1, 2, 3, 4, 5, 6}

c Sample space = {2, 4, 6, 8}

d Sample space = {blue, red, yellow, green, green}

3 b P (1) = 1 6 , P (2) = 1 6 , P (3) = 1 6 , P (4) = 1 6 P (5) = 1 6 , P (6) = 1

d P (blue) = 1 5 , P (red) = 1 5 , P (yellow) = 1 5 , P (green) = 1 5

Independent practice

1 a Sample space = {Hearts, Diamonds, Clubs and Spades}

b P (Hearts) = 1 4 , P (Diamonds) = 1 4 , P (Clubs) = 1 4 , P (Spades)= 1 4

c 1

2 a Sample space = {M, I, N, U}

b P (M) = 3 7 , P (I) = 2 7 , P (N) = 1 7 , P (U) = 1 7

c Because the 7 possibilities must equal one whole.

d M: 9, I: 6, N: 3, U: 3

3 This could be a group activity with students sharing and discussing the results.

4 Answers could vary. For example: a fifty-fifty

b no chance

c not very likely

d almost certain e definitely

5

3 Answers might vary. Students could be asked to justify their responses in a group discussion.

4 a 1 6 , 0.17, 16.7% b 1 4 , 0.25, 25% c 1 3 , 0.33, 33 1 3 %

DRAFT

4 Answers will vary. For example, it’s too general and could suggest that people in their 20s are responsible for half of all lawbreaking.

5 Answers will vary. For example, there might not have been many people over 70 out driving that afternoon.

Extended practice

1 a So people will think that everything is 70% off.

b 1

c To get people to come into the shop.

2 a $8.50

b Because the original price was already more expensive than it was supposed to be.

c Because the old price was actually cheaper than the “bargain price”.

3 This could become a collaboration opportunity.

6 P(3) = 1 3 , P(not 3) = 2 3

7 a Sample space = {boy, girl}

b Sample space = {1, 2, 3, 4l}

c Sample space = {win, lose, draw}

d Sample space = {Days of the week}

8 a It wouldn’t change it.

b There are now more possibilities for 1. P (1) = 1 2 , P (2) = 1 6 , P (3) = 1 6 , P (4) = 1 6

5 Students could look at the method used at http://www.mste.uiuc.edu/reese/birthday/.