Teacher’s Pack




Teacher’sPlanningBook



Teacher’sResource Book



JuniorInfants
Teacher’sPlanningBook

JuniorInfants
Teacher’sResourcesBook
JuniorInfants
Teacher’s Pack








Teacher’sPlanningBook







JuniorInfants
Teacher’sPlanningBook

JuniorInfants
Teacher’sResourcesBook
JuniorInfants
Teacher’s Pack




Notetoteachers: Thecontentsshownindicatewhatisincludedinthissampleextract. While we have combinedthe resourcesintoasinglebooklethere,therewillbetwoseparate booksprovidedtoteacherswhoadopttheprogramme–a Teacher’s PlanningBookanda

ThePrimaryMathematicsCurriculum(PMC)hasfive Strands,whichare dividedinto StrandUnits:
Algebra DataandChanceMeasuresNumber ShapeandSpace
● Patterns, Rulesand Relationships
● Expressionsand Equations
● Data
● Chance
● Measuring
● Time
● Money
● UsesofNumber
● Numerationand Counting
● Place Valueand Base Ten
● Setsand Operations
● Fractions
● Spatial Awarenessand Location
● Shape
● Transformation
In MathsandMe,thecontentofeachunitisclearlyconnectedtooneormoreofthe Strands and StrandUnits.
EachoftheStrandUnitscontainsasetofLearningOutcomes.Learningoutcomesareusedtodescribethe expectedmathematicallearninganddevelopment foralllearnersattheendofatwo-yearstage,whendue accountistakenofindividualabilitiesandvaryingcircumstances.
Eachofthelearningoutcomesbeginswiththestem,‘Throughappropriatelyplayfulandengaginglearning experiences,childrenshouldbeableto...’Thisstememphasisestheimportanceofprovidingrichand engaginglearningexperiences.
In MathsandMe alllearningoutcomesarecoveredindepth.Theprogrammehasbeendesignedto includeawide rangeofrich,appropriatelyplayfulandengagingactivitiesandtasks,whichdemonstrate keypedagogicalpractices,andsupportchildrentowardsachievingthelearningoutcomesanddeveloping theirmathematicalproficiency.
The PMCissupported by thePrimaryMathematics Toolkit,whichincludestheProgressionContinua.The progressioncontinuaoutlineasamplelearningtrajectoryofMathematicsatprimarylevel.Theysuggest aseriesof learningexperiences whichchildrenmightengagewithastheydevelopanddeepentheir mathematicalknowledge,skillsanddispositions.Eachcontinuumdescribesthelearningjourneyacrosseleven ProgressionMilestones(a–k)intermsofmathematicalcontent(StrandUnits)andprocesses(Elements).
Takingintoconsiderationtheelevenprogressionmilestones(a–k),thevariousclasslevelsof MathsandMe have beendevelopedaroundcertainmilestones:
JuniorInfants ProgressionMilestonesbandc
SeniorInfants ProgressionMilestonescandd
FirstClass ProgressionMilestonee
SecondClass ProgressionMilestonef
Table2: MathsandMe ClassLevelsandtheProgressionContinua
Thatsaid, MathsandMe recognisesthatchildrenlearnanddevelopatdifferent rates.Therefore,whilethe contentoftheFirstClassbook, forexample,centresaroundsuggestedlearningfromProgressionMilestone e,itisalsoinfluenced by thestatementsinProgressionMilestonesdand f, tocater fortheneedsofallthe children. Teachersare recommendedtoexerciseprofessionaljudgementwhenmakingdecisionsastothe learningactivitiesandtaskswhicharemostappropriate forthechildrenintheirclassroom,andtoadapt accordingly.
ThePrimaryMathematics Toolkitoutlinesmathematicalconcepts.Theseareconsideredtobethe keyideas thatunderpineachlearningoutcome.Itisenvisagedthatchildrenwilldeveloptheirunderstandingofthese conceptsthroughengagingwiththemathematicalprocesses,asoutlinedintheelements.
In MathsandMe,mathematicalconceptsinformthedesignofunits,includingthemathslanguageto focus on,andinfluencetheprogressionofcontentfromoneclasstoanother.
A FocusofLearningidentifiesthepurposeofalessonand/ortheintendedlearningthatwilltakeplace.
In MathsandMe,the FocusofLearningineachlessonechoesthesuggestedlearningexperienceslisted intheprogressioncontinua,i.e.each focusoflearningiseitheranexact replicaofastatement,oris derivedfromoneormorestatements.
The focusoflearningisalsoconnectedtooneofthe fourelementsusingtheabbreviatedtitles.
Focusoflearning(withElements)
● Exploreshowthelayoutoforsizeofelementsinasethasnoeffectonthe overalltotal(conservationof number)(U&C)
● Subitises(looksatagroupofobjectsand realiseshowmanythereare,withoutcounting)numberof objectsinaset(U&C)
Digitalactivities:SubitisingandConservationof NumberA–E
MAM Routines: Reason& Respond/QuickImages
Concreteactivities:ConsolidationofNumber Pupil’sBookpage44:ConsolidationofNumber (0−8)
Childrendeveloptheirmathsskillsthroughprocessessuch asconnecting,communicating, reasoning,argumentation, justifying, representing,problem-solving,andgeneralising.The PMC(2023)uses fourelementstodefinetheseprocesses,and tocategorisethesuggestedlearningexperiencesgiveninthe progressioncontinua.
● Manipulatives (bears,counters, beads,links, interlocking cubes, 2-Dand3-Dshapes)
The PMCadvocatesthatteachers providechildrenwithrichmathematical learningexperiencesthatareplayfuland engaging,that reflect relevantpedagogical approachesandthatprovideopportunities forchildrentocollaborate,communicate mathematicalthinking,andexpresstheir understandinginmultiplewaysandin variouscontexts.
Table3:An overviewofelementsandabbreviations
In MathsandMe,the focusoflearningineachlessonisclearly connectedtooneoftheseelementsusingtheabbreviated title(seesecondcolumnof Table3).Andwhilethe focusof learningmayincorporatemorethanoneofthesecentral processes,itisthemostprominentelementthatisgiven. ©TheEducationalCompanyofIreland
Inlinewiththelearningoutcomes, mathematicalconceptsandsuggested learningexperiencesintheprogression continua, MathsandMe providesawide rangeofrichandmeaningfullearning experiences foreachlesson.Manyof thesearedesignedaroundthe Maths andMe Routines.
MathsandMe Routines areacollectionofplayful,engagingandinclusiveinteractionsthatpromote mathematicaltalk,thinkingandmodelingamongallchildren.These repeatable routineshave beenchosen astheyareproventoactivatepriorknowledge, fosterproductivedispositionsandprovidevaluable formativeassessmentopportunities forteachers.
Inadditiontosupportingthefive keypedagogicalpractices,whichwillbedescribedindetailinthenext section,the routinesalsosupport formativeassessmentandinclusivepracticesasdemonstratedin Table4.
Doesitsupport/promote…?
Sometimes,Never; TargetBoards
Chapter6ofthe PMCdescribesfive keypedagogicalpractices.Thesepracticesareacknowledgedasessential totheprovisionofqualitymathematicallearningexperiences.
The PMCemphasisesthat‘Dispositionsarenotstaticandcanbenurturedorchanged overtime’andacknowledgesthat‘attitudestomathematicsandvalues,bothathome andintheclassroom,alsohave astrongimpactonthedevelopmentofthechild’s productivedisposition formathematics’(p.28).
Thefundamentalaimof MathsandMe is forthechildtocometoappreciatemathsassomething thatispositiveand relevanttothem.Ifthishappens,itismorelikelythattheywillengagewithmaths inameaningfulway. Thisseriesaimstoachievethis by:
● Highlightingthe worldofmathsaroundthechildren −intheirschools,intheirhomes,intheirlives
● Using real-lifeexamples ofmathsinactionandsituationsthatchildrencareabouttomakemaths meaningfuland relevant
● Actively encouragingthepositiveinvolvementof families intheirchild’slearningandmathematical experiences
● Incorporatingfunandentertainingactivities,includingplay, role-playscenariosandengaginggamesto boostenjoymentwhilealso emphasisingcollaborativetasks overindividual work
● Includingtasksthatencourage activeparticipation,exploration,investigation,productivestruggle, risk-taking,creativestrategiesandperseverance,whichwill resultin asenseofpersonalsatisfaction intheiraccomplishments
● Providing scaffolding,encouragementandsupport,includingtimetothinkand reflect
● Enabling childagencyandthechild’s voice.
The PMCstatesthat‘MathematicalModelinginvolvesusingmathematicstodescribea problem-contextanddeterminemeaningfulsolutionstotheproblem.In formingmodels, childrenmightusephysicalactions,spoken words,objects,images(e.g.graphs,diagrams andpictures),symbolsorwritten words’(p.30).
MathsandMe enablesmathematicalmodelinginmanyways:
● Emphasisingthe importanceofthinkingtime toallowthechildrentomakesenseoftheirthinking
● Using speciallychosenquestionsandtasks toencouragethechildrentomodelmathematically
● Presentingmathsconceptsinmultipleways and/orusingmultiplevisual representationstointroduce thechildrentoawidervarietyofmodelsthantheymighthave encounteredorcreatediflefttotheir owndevices
● Encouragingthechildren tobeindividualandunique inhowtheyexpressand representtheirideasandthinking
● Promptingthechildrento challengeandtesttheir ownthinking andmodels,and thoseofothers
● Encouragingtheuseof physicalmodels (classroommaterialsandmanipulatives) tosupportmathematicalmodelingintheclassroom,andincluding representationsoftheseintheprintanddigitalmaterials
● Usingavarietyof conceptualandproceduralmodels todemonstrate differentapproachesandstrategiesandencouragechildrentodeveloptheir ownmodelsanduniquewaystoapproachcomputationsandtasks.
The PMCdescribesCognitivelyChallenging Tasks (CCTs)as‘richhigher-orderlearning opportunitiesthatshouldappropriatelystretchandchallengechildren’sconceptual understandingastheyencountersignificantmathematicalideasandsituations. Sometimes referredtoas low-thresholdhigh-ceilingtasks,thesetasksshouldprovide allchildrenwiththeopportunitytoaccessmathematics,whileofferingthepotential for deeperengagement’(p.31).
MathsandMe facilitatesCCTsintheclassroom by:
● Incorporatingchallengeslinkedtochildren’scurrentlevelofknowledgeandunderstanding,while providinganappropriatestretch
● Providingtaskswherethesolutionpathwayisnotimmediatelyobvioustothelearnerorwherethe solutionisnot reducedtoasetofstepsandprocedures
● Facilitatingchildrentocommunicateandexpresstheirideasopenly,allowingthemtodiscuss,compare, justifyandevaluatetheirideasorsolutions.
CCTshave beenincorporatedthroughouttheprogrammeanditscomponents.
The PMCdescribesMaths Talkas‘acollaborativeprocesswherechildren’sthinking, strategiesandideasarediscussed,sharedand/orexchanged.Thisallowschildrento reflectontheir ownunderstanding;define,presentandjustifytheirideas;makesenseof andcritiquetheir ownideasandthoseofothers;anddeveloptheirabilitytoexpressand articulatetheirthinking’(p.32).
Ineverylesson, MathsandMe providesideas forMaths Talkactivities,whichbeginwithappropriately challengingandengagingtasks.Bytheir verynaturetheseMaths Talkactivitiesalsoproviderichassessment opportunities,i.e.elicitingcurrentknowledgeandunderstanding,identifyingemergingconcepts.These activitiesemphasisetheprobingand responsiveaspectsofMaths Talk.Allofthe MathsandMe Routines promoteand fosterMaths Talk.Theuseofthese routinessupportsacultureof respectandrisk-taking,where children feelsafetosharetheirthinkingandareencouragedtolistentoandvaluetheideasofothers.
The PMCstatesthat‘Mathematicallearningcanbegreatlyenhancedinaplayenvironment thatisinteractive,engaging,inclusiveandsupportive;andthatprovidesopportunities for exploration,investigation,challenge,creativity,choiceandindependence.Playprovides acontext formathematicalthinkingandthedevelopmentofmathematicallanguageand concepts,withclearpotential forpromotingmathstalk’(p.29).
MathsandMe encouragesplayfulness by:
● Tappingintochildren’sinterestsandcuriositiesthroughengagingthemesand real-lifecontexts
● Suggestingideasandwaystointegratemathematicallearningwithplayfulactivitiesthroughouttheday
● SignallingopportunitieswherechildrenencounterMathematicsinspontaneousplayandexploration
● Emphasisingwaysto reinforcemathematicallanguageasitarisesthroughplay
● Facilitatingmultiplemeansofexpressionand representation
● Providingopportunities forchildrentoexploreandexperimentwithmathematicalideas
● Fosteringthecreationofasafespace forspontaneity,creativityandimaginativeplaywith mathematics
● Providingaccesstoawide rangeof resources,visualsupportsanddigital resources.
The PMCexplainsthat ‘Assessmentisanintegralpartoflearningandteaching.Itinvolvesteachersand children workingtogethertouseinformationtoinformandsupportlearningandteaching’(p.34).The PMC advocatesthreetypesofassessmentasbeingnecessary‘togainacomprehensivepictureofachild’sprogress andachievement’(p.35).Theseare:
● Intuitiveassessment:Unplanned,unrecorded,andongoing
● Plannedinteractions:Morevisible,maybe recorded,and relatedtolearningoutcomes/competencies
● Assessmentevents:Distinct,visible, recordedevents
Assessmentisintegratedthroughout MathsandMe.Thelearningexperiencesarestructuredinsuch awaythattheysimultaneouslypromotethedevelopmentofmathsskillsandfluency,andenablethe teachertogather, record,interpret,useand reportinformationaboutachild’sprogressandachievements. Theteachercanthen respondtotheinsightsgatheredfromtheassessmentsandadjusttheirteaching accordingly.
Theprogrammesupportsthethreetypesofassessmentoutlinedinthe PMCinthe followingways:
Intuitiveassessment Plannedinteractions Assessmentevents
● Unitplansprovide comprehensivebackground andrationale information forteachersonallthetopics covered,alongwith guidance andsupportsaroundcommon misconceptions,thus increasingteacherawareness andpreparednesstoobserve and respondtopupils.
● Lessonsaredesignedaround richlearningexperiences linkedtothe focusoflearning andlearningoutcomes.
● Lessonsuseanumberof MathsandMe Routineswhich posequestionsdesigned tostimulateclassroom conversations andtoscaffold learning.The regularityof theseensurethey become familiar,recognisable assessmentopportunities.
● Eachlessonplanhighlightsthe learningexperiencebest suitedtogenerating recordableassessmentdata.
● Pupil'sBooktasks provide furtherassessmentdata,as doesthe self-assessment feature.
● Mathsjournalling opportunitiesareoutlinedin thelessons.
● Eachunitcomeswitha FormativeAssessment ObservationsSheet for teachers.
● The ProgressAssessment Booklet providescheckupquestionslinkedtothe learningoutcomesofeach unit.Thesequestionscanbe usedasaplannedassessment and administeredasa traditionaltest,orusedasthe basisof agrouporwholeclass quiz
● A comprehensiverecord sheet enableseasyanalysisof assessmentdataatindividual, groupandclasslevel.
Thisguidewillwalk youthroughtheprogramme’s keycomponents,highlightingitsstructure,integrated approach,easeofuse,andthechoicesitofferstobothteachersandchildren.
The MathsandMe yearly overviewisdividedintomonths,thensubdividedinto units.Eachunitiseitheroneortwo weekslongandcoversaspecificareaof learning.Thecontentofeachunitisclearlyconnectedtooneormoreofthe StrandsandStrandUnits.
Review Weeksarealsoincludedinthe yearly overview,providing regular opportunities forchildrento reviewand reflectontheirlearning.
Unitsarethebuildingblocksof MathsandMe.Eachunitisbrokendownintoa numberoflessonplans,and followsasimilarstructure.Theinitiallessonactivates andassesseschildren’spriorknowledge,thesubsequentlessonsdevelopand progressthelearning,whilethefinallessonofeachunitprovidesavaluable opportunityto reviewand reflectviaamenuofoptions.
Let’sStrengthensuggestionsandmaterialprovidesadditionalsupporttochildren,whileLet’sDeepen suggestionsandmaterialprovideadditionalchallengeandextensionopportunities.
Eachlessonalso followsaclearstructure,whichisstraightforwardandeasyto follow, andprovidesflexibility andchoice forteachers.
Eachlessonstartswithwarm-upsuggestionstogetthechildren readytolearn. Theyaretypicallywhole-classactivities,whosepurposemightbeto:
● Provideanintroductiontothemainlesson
● Revisitconceptsthatthecurrentlessonwillbuildupon
● Reviewcontentcoveredpreviously.
Mainevent
Themainpartofthelessonincludesanumberoftasks,throughwhichthe childrenwillachievethe focusoflearning forthelesson.
Thesetasksmaybeconcreteactivities C ,digitalactivities D oractivitiesbased onprinted resources P
Somepointstonoteabout MathsandMe lessons:
● Emphasisonplayfulandengaginglearningexperiences
● Useofdigital resourcestoenhancethelearning
● Useof MathsandMe Routines:see At-A-GlanceGuide
● Support foralllearnersthroughLet’sStrengthenandLet’sDeepen features
● Formativeassessmentopportunitiesthroughout.
Optionalconsolidationandextensionopportunities
Eachlessonendswithachoiceoffurtherlearningexperiencestoconsolidateandextendthechildren’slearning.
To supportteachersinimplementingthe PMC,thecomponentsof MathsandMe have beendevisedtoserve variouspurposesandenhancetheteachingandlearningofmathsintheclassroom,widerschoolandhome environments.
Pupil’sBook
The MathsandMe Pupil’sBookisdesigned foruseafterengagementwith thelearningexperiencesoutlinedinthelessonplans.Itspurposeistwofold: toprovideanopportunitytoconsolidatethenewlearning,andtoprovidea recordofchildren’s work.
The following featuresareincluded:
● TryThis:providesoptional,cognitivelychallengingtasks forpupils
● Let’sPlay:incorporatesplayfulnessintomathsthroughengaginggames andinteractiveactivities
● MathsEyes:encourageschildrentolookaroundthemand recognise mathsinthe real world.
ThePupil’sBookalsocomeswiththe followingadditional resourcesto supportlearning:a MWBwithbranchingbondtemplate,atenframe,anda choiceoftwospinners forusewithgames.

The MathsandMe Home/SchoolLinksBook recognisestheimportanceofthe familyinthechild’slearning.Includedinthebookare‘Dear Family’notes for eachunit,whichprovidetipsonhowtosupportthechild,practicalactivitiesto becompletedathome,andQRcodesthatlinktodigital resourcessuitable for homeuse.Theactivitiesinthisbookhave beencreatedtobeapproachableand open-endedto facilitatechild-ledinvestigationandplayfullearning.
The MathsandMe teacher’s resourcematerials have beensplitintotwobooks:the Teacher’s PlanningBookandthe Teacher’s ResourcesBook.
The Teacher’s PlanningBookprovides comprehensiveteachingandplanning supportmaterialsinlinewiththe PMC.A yearly overview,unitplanswithshort-term planningandlessonplansareallincluded.
● The YearlyOverviewmapsouttheunits andstrandunitscovered overthecourse ofthe year.
● TheUnitPlansincludetheshort-term plan(fortnightlyplan)andadditional informationuseful forplanning,suchas commonmisconceptionsandmodelsand representationsused.









● TheLessonPlansoutlinethe focusof learning,learningexperiences,equipment needed,andmathslanguagethatwillbe focusedon.Fromthere,eachlessonisbrokendowninto WarmUpactivities,theMainEventandOptionalConsolidationandExtension Possibilities.
The Teacher’s ResourcesBookincludesalladditionalmaterialsoutsideofplanning.A rangeofphotocopiable materials(PCMs)are featuredhere,includinggeneralPCMs,Let’sStrengthenSuggestions for Teachers,Let’s StrengthenPCMsandLet’sDeepenPCMs.
ProgressAssessmentBooklet
The MathsandMe ProgressAssessmentBooklet featuresassessmentsthat covereachunit.Assessmentscomeinthe formofcheck-upquestionswhichare linkedtothelearningoutcomesofeachunit.Thebookletisdesignedinsucha waythatitcanbeeasilyusedaftereachunit,orduringeach review week.




The MathsandMe digital resourceswillbringmathstolife forchildren,andenhanceclassroomlearning by encouragingparticipationandpositiveengagement.The resourcesaredesignedtocater fordifferentlearning stylesandcontributetothewide rangeofrichandplayfullearningexperiencesintheprogramme.
Innovative,intuitiveandeasytonavigate,the MathsandMe resourceshave primarilybeendeveloped asteachingtools fortheinteractivewhiteboard(IWB)andcanbeusedinconjunctionwiththechildren’s mini-whiteboards(MWBs)whereappropriate.
Supportingthepedagogicalpracticespromotedinthe PMCandproviding variedlearningopportunities forchildren,the resources reflectthelesson’s focusoflearning,promoteMaths Talk,incorporatemathematicalmodeling andallow for formativeassessment.Many resourceshave beenspecifically designedaroundthe MathsandMe Routines,suchasConceptCartoon, Three-Act TaskandQuickImages.
Digital resourcesaccompanymostlessons. To provideguidance fortheintegrationofdigital resourcesin theclassroom,theyare referencedindetailthroughoutthelessonplans foreachunit,withsuggestions for discussionanddifferentiation.The resourcesincludethe following:
Animations
Featuringtheprogrammecharacters–Mia,Jay, Lexi,DaraandMontythedog –theanimationsbringmathstolife withcolourfulstories,songsandfun scenarios relevanttochildren



Videos
Immersiveandinterestingvideoswithdetailedconceptexplanationsandmeaningfulexamplesofmaths inactioninthe real world



Awide rangeofdigitalactivities–includingposters,slideshowsandinteractivegames–tosupport teachingandconsolidatelearning,encourageMaths Talkandpromoteactiveparticipation




Acomprehensivesetofbespokeinteractivemathstoolsandmanipulatives,includingMoney,Shapes,Clock, NumberSquare,Place Value,Dice,Spinnerandlotsmore!
Teacherscanaccessthe MathsandMe digital resourcesvia www.edcolearning.ie,whereadedicated websitewillhosttheprogramme’s keycomponents.Witheasy-to-usefilteringtosupportclassroom teachingandlessonplanning, mathsandme.ie willincludeinteractivee-books,digital resources,editable planningdocumentsandprintables(suchasMathsLanguageCards,ManipulativesCut-OutsandAssessment RecordSheets).
To supporthome/schoollinksandstationteachingintheclassroom,abankofdigital resources–suchas animationsandinteractivegames–willbemade availableviaafreePupil/ParentApp.
Unit8:
MathsandMe:JuniorInfants–Shor tTe rmPlanUnit8:Numbers6to8 (J anuary: We eks1&2)
Number>UsesofNumber;NumerationandCounting;SetsandOperations;Algebra> Pa ttern,Rulesand Re lationships
Strand(s)>Strandunit(s)
LearningOutcome ( s )T hroughappropriatelyplayfulandengaginglearningexperienceschildrenshouldbeabletodevelopan aw arenessthatnumbersha ve avarietyofuses;developan aw arenessthatthepurposeof countingistoquantify;usea ra ngeofcountingstrategies fo ra ra ngeofpurposes; re cogniseandunderstandwhathappenswhenquantities(sets)arepartitionedandcombined.
IntuitiveAssessment: re sponding toemerging misconceptions
PlannedInteractions: re spondingto insightsgleanedfrom children’s re sponsesto learningexperiences
Fo cusofLearning(withElements)
D Re ason& Re spondL1,3–9
C CountingObjectsL1
C Number Ta bleL1
P Story:‘SnowWhite’L1
C SoundofaNumberL2
C MakingSetsL2 P Book: HowtoCatcha Ra inbow L2
C MakingSetsandMatchingNumerals (0−8)L3
P NumberBingo3L3
P Book: SuzietheEight-Legged Pa inter L3
D QuickImagesL4
C ConsolidationofNumberL4
C CompositionofNumberL5–6
C MakingEquivalentandNon-equivalent SetsL7
AssessmentEvents: informationgathered fromcompletionof theunitassessment intheProgress AssessmentBooklet pagexx
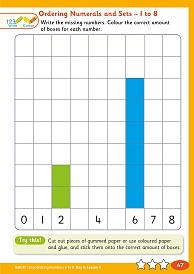
C OrderingNumeralsandSetsL8
D Ja y’ sSoccerPracticeGameL8
P Book: Six-DinnerSid L8
D Number Fo rmation0to8
C MakingNumeralsL9
P Book: OneMoleDiggingaHole L9
Print re sources
Pupil’sBookpages 43 –48
Home/SchoolLinksBookpagexx
PCMxx
Counting1to8: Re cites fo rwardtoatleast 10 (U&C); Re cognisesnumbers,initiallywithin 10 (U&C);Demonstratesan aw arenessof andusesnumeralsinpersonallymeaningfulcontexts (C ); At tendstonumeralsofsignificanceorimportancetothechild(U&C);Uses appropriatestrategiestofindouthowmany(A&PS)
MakingSets(0−8): Demonstratesan aw arenessofnumberandnumber wo rdsequencingthroughsong,stories,rhymesandgames (C ); Pa rt icipatesinactivitiesthatin vo lvecommunicatingaboutnumber(U&C);Usesideasaboutnumberandquantitytocommunicatewith others (C );Exploreshownumbersareused fo rcountingandthatthelastnumberinthecountindicatesthequantityofobjectsinaset(U&C)
MatchingNumeralstoSets(0−8): Re cognisesthatobjectsandsymbolscan re presentnumbers(U&C);Matchesnumeralsand number wo rdstosetsandtoothernumeralsinavarietyofcontexts(A&PS);Selectsandusesappropriatematerialstomakeavariety ofsets fo ragivennumber(A&PS);Sorts,groupsandarrangesmaterialsaccordingtocriteria(R);Establishesthat ze ro ,asanumeral, re presentsnothing/noneintermsofquantity(R)
ConsolidationofNumber(0−8): Exploreshowthela yo utoforsizeofelementsinasethasnoeffectonthe ove ra lltotal (conservationofnumber)(U&C);Subitises(looksatagroupofobjectsand re aliseshowmanythereare,withoutcounting)numberof objectsinaset(U&C)
CompositionofNumber(1−8): In ve stigatesvariousarrangementsofmanipulativestopromptdifferentmentalimagesofnumbers upto8,whiledevelopingasenseofeachnumber(R)
ExtendingCompositionofNumber(1−8): In ve stigatesvariousarrangementsofmanipulativestopromptdifferentmentalimagesof numbersupto 10 ,whiledevelopingasenseofeachnumber(R)
EquivalentandNon-equivalentSets(0−8): Showsanunderstandingofdifferencesinvalue(U&C);Usescomparativelanguage (more,less,same)tocomparesetstoatleast 10 (C );Usesappropriategesturesand wo rdstocon ve yandmakecomparisons (C ); Identifies, re cognisesandestimatesmoreorlessinthe re al-lifecontextand/orplay(R)
OrderingNumeralsandSets(1−8): Ordersnumeralsuptoatleast 10 (U&C);Orderssetswithoutcountingandchecks by counting (R);Explainsordinalityusingthelanguageofafter,beforeandin-between (C)
Wr itingNumbers0to8: Makesnumeralscreatively (C );Discusses,dr aw sandwrites re presentationsofnumbers1−8,using manipulatives (C );Explorestheuseofnumberandplaysgamesto ra ise aw arenessofnumberintheirenvironment(A&PS);Notices and re cognisestheuseofnumeralsaslabelsinthecontextofhome,theclassroomandtheschoolenvironment(U&C)
Re viewand Re flection: Re viewsand re flectsonlearning(U&C)
Ke y: Elements: (U&C)UnderstandingandCommunicating; (C )Communicating;(R) Re asoning;(A&PS)ApplyingandProblem-Solving.
LearningExperiences: C concreteactivity; D digitalactivity; P activitybasedonprintedmaterials, fo llo we d by lessonnumbers.
CM:CuntasMíosúil: pleasetickwhen yo u ha ve completedthe fo cusoflearning.
Lesson
1
2
3
4
5
6
7
8
9
10
ProgressionContinua
MathsLanguage
Equipment
InclusivePractices
See‘JuniorInfants MathsandMe ProgressionContinuaOverview’ foradetailedbreakdownofhowall progressioncontinuaarecovered.
See‘JuniorInfants MathsandMe MathsLanguageOverview’andindividuallessonplans.
See‘JuniorInfants MathsandMe MathsEquipmentOverview’andindividuallessonplans.
● SeeLet’sStrengthenandLet’sDeepensuggestionsthroughoutlessonplans.
● SeeUnit8Let’sStrengthenSuggestions for Teachers.
● SeeUnit8Let’sStrengthenPCM.
Integration
● SeeUnit8Let’sDeepenPCM.
Seeindividuallessonplans.
● Overthecourseofthe fortnight,thechildrenwillbecountingfrom1to 10,butthe focuswillbeonthe numbers6to8. Youwillbeassessingwhethertheyknowthesequenceinwhichtocount,areusingoneto-onecorrespondence(assigninganumbernametoeachobject,0−8),knowthatthelastnumberisthe totalcount,andunderstandconservationofnumber.
● Theywillbeintroducedtotheabstractprincipleofcounting(e.g.countingsounds),leadingtothembeing abletocreateamentalimageofanumberandmoreeasily‘counton’intheirhead.
● Itisof keyimportancethatchildrenseenumbersinthe‘real world’andthattheyapplytheirgrowing knowledgetoexperiencesoutsidetheclassroom.Theywilllistentostoriesaboutnumbertoextendtheir experience.
● Theywillbeginto recogniseandidentifythenewnumerals,andwritethenumerals1to8‘creatively’and onpaper,astheyconsolidatetheirexperienceofmatchingthenumeraltothecorrectnumberofobjects orsounds.
● Compositionofnumberallowsthechildrento‘breakup’anumberofobjects(1−8)andexplorethe differentcombinationstheycanmake.Seeingsmallernumberswithinthebiggernumbersolidifies comprehensionofthenumberitself,andwhatitis‘madeof’. Partitioningandcombiningbecomesa naturalprogressionwhenthechildrenhave already‘deconstructed’anumberofobjects.
Thethemeofthisunitis PictureBooksAboutNumber.Suggestions for relevantbooksareincluded inthelessonplans.
Thechildrenmay:
● Counttoomanyortoo fewobjects
● Countthesameobjectmorethanonce
● Repeatanumberwhilecountingobjectsormissanumber
● Not realise,whenusingthetenframe,thatthecounterscanbeinvaryingplacesintheframe,but canstillbesuccessfullycounted
● Becomeconfusedwith wordsthathave thesamesound,butdifferentmeanings(e.g. ate and eight)
● Mixuplettersymbolsandnumbersymbols(e.g.5andS,or8andB)
● Strugglewithnew vocabulary,suchas: lot, some, onemore, oneless.(Itis veryhelpfultomakeup smallstories forthisagegroup,e.g.‘There were sixdwarfs out forawalk,butthenonemorejoined them.Howmany were therethen?’)
● Strugglewithmakingthelinkbetweenthenumeral,thenumber wordandtheobjects(concrete andpictorial).(Engaginginmultiplehands-onactivitieswill reinforcethisconcept.)
● Numbershapes
● Fiveframes
● Tenframes
● Orderingframes
● Cuisenaire rods
● Sortingringsand/ordividers
● Two-sidedcounters
● Recites forwardtoatleast 10 (U&C)
● Recognisesnumbers,initiallywithin 10 (U&C)
● Demonstratesanawarenessofandusesnumeralsinpersonallymeaningfulcontexts (C)
● Attendstonumeralsofsignificanceorimportancetothechild(U&C)
● Usesappropriatestrategiestofindouthowmany(A&PS)
Digitalactivity:CountwithMonty
MAM Routine: Reason& Respond
Concreteactivity:CountingObjects(1−8)
Concreteactivity:Number Table
Story:‘SnowWhiteandtheSevenDwarfs’
Pupil’sBookpage 43:Counting1to8
● Manipulatives(bears, counters,beads,links, interlockingcubes,2-D and3-Dshapes)and collectionsofsmall objectsfromnaturewalks(leaves, twigs,pinecones,etc.)
● Story:‘SnowWhiteandtheSeven Dwarfs’
● Paintorplaydough
● six,seven,eight,in-between,next
D Digitalactivity:CountwithMonty
MAM Routine: Reason& Respond Usethedigitalcountingactivity. Tellthechildrento countwithMontyandhelphimnamethenumbers. Assessthe following:
● Aretheycountinginsequence(notmissingany number)?
● Aretheycountingeachnumber(oncenottwice)?
● Cantheystartatanumber(e.g.4)andcounton?
● Cantheystopatadesignatednumber(e.g. countto 7)?
● Canthey recogniseeachnumberanditsposition?
Theyarealsoansweringquestionssuchas:
● Isanyoneintheclassfive yearsold?Whereisthat numberonthesteppingstones?(in-between4 and6,after4,before6)
● Isanyone four/six yearsold?Whereisthat number?(in-between…and…,after…,before…)
● Whatagewill youbeon your next birthday?
● Doesanyonehave asister/brotherwhoisseven/ eight yearsold?
● Doesanyoneknowthenumberoftheirhouse?
● Isanyone’shousenumberbetween1and 10?
● Whatnumbercomes after 6?
● Whatnumbercomes before 8?
● Whatnumbercomes in-between 6and8?
Teachingtip:
Youcouldplaythisactivityeveryday over thecourseofthisunit.
C Concreteactivity:CountingObjects(1−8)
Thisactivityprovidesanopportunitytoassesseach child’sunderstandingofcountingobjects,as wellas thestrategiestheyareusing.Thechildrenarenot assigningatotalnumberinthisactivity, ratherthey are focusingonthecountandtheintroductionofthe numbers6,7and8.Distributemanipulativestoeach child,andtellthemtodothe following:
● Countasetof1,2,3,4,5objects(revision).
● Countasetof6,7and8objects.
Ensurethateachchildiscountingcorrectly (e.g.theymaybecountingintheirhead,but missinganobjectormissinganumberinthe sequence). At thisearlystageofcounting, mostchildrenwillbecountingaloud,pushing objectsawayfromthegroupofobjectsand assigninganumberastheypush/touch.
Assessthe following:
● Arethechildren repeatingone numberormissinganumberinthe sequence?
● Aretheysubitisingsmallernumbersofobjects, e.g. four.Whatistheirstrategy.Ask:Howdo you knowthereare fourobjects?(Dotheyseetwo andtwo?)
● Aretheytouchingeachobject once orarethey countingoneobjecttwice?
● Aretheyassigninganumbernameto eachobjectastheytouchit?
● Dotheyunderstandthatthe objectscanbemovedapart slightlyorcounted,e.g.topto bottom(inastackofcubes),andthey willstillgetthesamenumber?
● Aretheycountinginthecorrectsequence, includingthenumbers6, 7, 8?
● Dotheyunderstandthatthe final numberisthe total ofalloftheobjects?
Placeeightobjectsonthetable. Tellthechildren tocountthem.Move threeawayandask:
● Howmanyareleft?
Aftertheyhave countedfive,placethethree missingobjectsbackonthetableagain,andask:
● Howmanyaretherenow?
Dothechildrenneedto recount?
● Cantheycountobjectsthatareinanirregular arrangement(e.g. by puttingtwoobjectsand threeobjectstogether,andcountingthetotal/ puttingoneobject,threeobjectsandtwoobjects together,andcountingthetotal)?Theyarenot adding,butassemblingandcountinga random groupofobjects.
Askthechildrentogive you, forexample, four cubesfromasetofsixcubes.Dotheyneedto recounttheamountwhenaskedhowmanycubes theyhave given you?Dotheyknowhowmany cubesareleft(aftergiving you four)?Dothey needtocountorcantheysubitise?Whenthe fourmissingcubesare returnedtotheset,dothe childrenknowthattherearestillsixcubes?
C Concreteactivity:Number Table
YoumightliketosetupaNumber Table forthethree‘newnumbers’ overthecoming fortnight.The number6could feature fora few days, followed by thenumber 7, andthenthenumber8.Boththe numeralandthe wordcouldbe shown(e.g.6and six).Setsofsix objects(e.g.counters)couldbeshowninvarious arrays.Thenumbercouldalsobeshownonpaper, andusingconcretematerials,itemsfromthenature tableanditemschosen by thechildren.Theycould help youtosetupthetable,oronegroupcouldbe assignedtosetitup.
P Story:‘SnowWhiteandtheSevenDwarfs’ Readthestory‘SnowWhiteandtheSevenDwarfs’. Thechildrencouldthendraw,paintormake(with playdough)thesevendwarfs.
P Pupil’sBookpage 43: Counting1to8
Distributeadicetoeachpair. Eachchildshouldcolourthe setsintheir ownPupil’sBook.

RolePlay If youhave ashopsetupinthe PlayArea,ingroups,thechildrencould role-play shopkeepersastheycountoutsmallitems fortheir customersandputtheminabag.Thecustomers should recounttheitems,tomakesurethattheygot theamounttheyasked for.
Story Read‘The WolfandtheSevenLittleKids’, andthenactouttheeventsfromthestoryinthePE hall.Therecouldbea fewgroupswitha wolfand sevenkids.
MathsEyes Whatnumberscanthechildren seeinside/outsidetheclassroom?Ifitissomeone’s birthday, youcoulddrawattentiontothisandask themtobringinoneoftheirbirthdaycardswith theirageonit.Drawattentiontoanycounting opportunities, by countingoutsixpaintbrushes, sevencopies,etc.Countirregulargroups(e.g.six childrenaroundthesandarea).
Song Thiscountingsonghasacatchytune forthechildrentosingalongto: edco.ie/npzc
● Demonstratesanawarenessofnumberandnumber wordsequencingthroughsong,stories,rhymes andgames (C)
● Participatesinactivitiesthatinvolvecommunicatingaboutnumber(U&C)
● Usesideasaboutnumberandquantitytocommunicatewithothers (C)
● Exploreshownumbersareused forcountingandthatthelastnumberinthecountindicatesthe quantityofobjectsinaset(U&C)
Concreteactivity:Soundofa Number
Concreteactivity:MakingSets
Book: HowtoCatcha Rainbow by
NaomiJones● Cubesorbuttons
● Tinorjar
● Manipulatives(bears,counters, beads,links,interlockingcubes,2-D and3-Dshapes)andcollectionsof smallobjectsfromnaturewalks(leaves, twigs,pinecones,etc.)
● Book: Catcha Rainbow by NaomiJones
● Crayonsinthesevencoloursofthe rainbow
● Thereisnonewmathslanguage forthislesson.
C Concreteactivity:SoundofaNumber
Observethechildren’sunderstandingofthe abstractionprinciple(e.g.countingsounds).Ask themtocountalongwith youas youdropfivecubes orbuttons,one by one,intoatinorjar. Repeat,but
thistime,askthechildrentocountontheir own. Repeatagain,butthistime,stopatvariousnumbers andaskthechildrenhowmanytheyhave counted. Repeattheentiresequence,butwithdifferent sounds,suchasclapping,tappingorclicking. You
mightaskindividualchildrentomakethesoundwhile theotherscount. Youcouldalsoholdupanumber andtellthechildrentochooseasoundandmakethat soundastheycount.
Tellthechildrentoclosetheireyesandcountwith you,andthenontheir own.Stopatvariousnumbers andaskhowmanysoundstheyhave heard.The childrenshouldthencountintheirhead.Ask individualchildrenhowmanysoundshave theyheard.
Numbertennis: Yousay, ‘one’,thechildrensay, ‘two’. Yousay, ‘three’,theysay ‘four’.Continueup toten.
Makeapattern: Yousay, ‘one,two’,thechildren say, ‘three four’.Continueuptoten.
Startatanumber: Yousay, ‘five’,thechildrensay, ‘six’.Continueuptoten.
Pair work–ask:
● Can youtapapattern/rhythm (e.g.1,2;1,2)?
● Can yourpartnercopy yourtapping?
● Can youshareitwiththeclass?
C Concreteactivity:MakingSets(0−8)
Thisisanopportunitytoassesseachchild’s understandingofthecardinalprinciple(assigninga ‘specialnumber’or‘totalnumber’toasetofobjects). Distributemanipulativestoeachchild.Say/ask:
● Makeasetwith yourobjects.
● Howmanyobjectsaretherein yourset?Howdo youknow?(Dothechildrenknowthatthelast numbertheysaywhencountingisthetotal quantity?)
● Can youshowandtelltheclasshow you found outhowmanyobjectsarein yourset?
● Caneveryonemakeasetofsix?Howdid you makeasetofsix?Howdo youknowthatthere aresixobjectsin yourset?
● Caneveryonemakeasetof four?Can youturnit intoasetoffive?(Doanychildrenaddanother objecttotheirsetof fourordotheymakeanew setoffive?)
● Whocanmakeasetofseven?Counttheobjects in yourset.Put yourobjectsinaline(horizontally andthen vertically).Howmanyarethere?
● Can youturn yoursetofsevenintoasetofsix? Howwill youdothat?Do youneedtocount your objectsagain?
● Can youmakeasetofsevenagain?Howwill you dothat?
● Can youmakeasetof zero objects?No!
Let’sstrengthen: Chooseanumber(from yourclass-sized numbers)anddrawthecorrectnumberofobjects on your MWB forthatnumber.Whatnumberdid youchoose?
Let’sdeepen:
Taponthetable.Howmanytapsdid youdo?Make asetthathasthisnumberofobjects.
P Book: HowtoCatcha Rainbow by NaomiJones
Read HowtoCatcha Rainbow by NaomiJones,in whichFreyadoesherbesttofindthesevencolours ofthe rainbow. Thechildrencoulddrawtheseven coloursofthe‘perfect’ rainbowthatFreyafinally finds,usingcrayons. Youcouldintegratethisactivity withLiteracyandVisualArts.
Teachingtip:
A readingofthisstoryis availableat: edco.ie/56tg
GamesBank Play hopscotchfromthe GamesBank.
MathsEyes Say/ask:
● Lookaround/outsidetheclassroom.
● Whocanseeasetof…(e.g.4)?
● Are youcorrect? (Thechildshouldgoandcount thesetifitisinsidetheclassroom.)
● Cananyoneseeasetwithonemoreobject?
● Cananyoneseeasetof8?
● Let’sfindoutif you were correct.
● Recognisesthatobjectsandsymbolscan representnumbers(U&C)
● Matchesnumeralsandnumber wordstosetsandtoothernumeralsinavarietyofcontexts(A&PS)
● Selectsandusesappropriatematerialstomakeavarietyofsets foragivennumber(A&PS)
● Sorts,groupsandarrangesmaterialsaccordingtocriteria(R)
● Establishesthat zero,asanumeral, representsnothing/noneintermsofquantity(R)
D C P P
Learningexperiences
Animation:MontyNeedsHelp!
MAM Routine: Reason& Respond
Concreteactivity:MakingSetsand MatchingNumerals(0−8)
Game:NumberBingo
Book: SuzietheEight-Legged Painter by BethanyGum
● Manipulatives(bears,counters,beads,links, interlockingcubes,2-Dand3-Dshapes)andcollections ofsmallobjectsfromnaturewalks(leaves,twigs,pine cones,etc.)
● Stickynotes
● Book: SuzietheEight-Legged Painter by BethanyGum
● Playdough
● Eggcartons
● Pipecleaners
● PCM1
● Thereisnonewmathslanguage forthislesson.
D Animation:MontyNeedsHelp!
MAM Routine: Reason& Respond Playtheanimation,inwhichMontyishavingtrouble countinghisbones,treatsandtoys,andthechildren
musthelphim.Whentheyhave helpedhimtocounta setcorrectly,theymusthelphimtoassignthe correctnumber.
C Concreteactivity:MakingSetsandMatching Numerals(0−8)
DistributemanipulativesandacopyofPCM1−Small Numerals0to8toeachchild.Thisactivityprovides anopportunitytoassesseachchild’sunderstanding ofNumber(0−8).Askthechildrento:
● Matchonenumeral,e.g.6,toanothernumeral6, andsaythenumbername.
● Makeavarietyofsets(usingthesameor differentobjects,andarrangingthemindifferent ways) forthenumbers2,3,4,5,6, 7, 8.
● Makesetswithdifferent-sizedobjects(e.g.aset ofsixsmallleavesandasetof fourlargeleaves). (Assesswhetherthechildrenthinkthatthe‘large’ setof fourhas‘more’.)
● MatchthenumeralsfromPCM1tosets (0−8objects).
● Countasetofobjectsandchoosethecorrect numeral fortheset.
● Chooseanumberfrom0to8andmakethe equivalentsetofobjects.Can youmakeasetof objects for0?
Unit8: Numbers6to8
● Exploreconservationofnumber(bycounting, for example,sixobjects,assigningthenumbername, andmovingtheobjects,butnot recounting).
Ask:
● Haseveryonegotacollectionof objects?
● Can youmakeasetwithoneobjectandasetof sixobjects? (Continuewithdifferentamounts for twosets.)
● Putthetwosetstogether.Howmanyobjects haveyougot?(Dothechildren counton or count all oftheobjects?)
Askthechildrentodrawasetofobjects forachosen number.
Let’sdeepen:
DistributesmallnumeralsfromPCM1andacopy eachofPCM2–Setsof1−5,andPCM3−Setsof 6−8toeachchild.Thechildrenshouldmatch numeralstothesets.
Let’sstrengthen:
UsingsmallnumeralsfromPCM1,canagroupof children(workinginpairs),matchnumeralsto makepairs(e.g.7matchedto 7)?Whichtwo childrenhave madethemostpairs?
P Game:NumberBingo
Thechildrenplaybingoingroupsofsix.Distributea bingocardfromPCMs4to6–NumberBingoCards andSmallNumeralsfromPCM1toeachplayer. The
numbersonthecardsareshownusingdifferent representations,includingdots,tallymarks,ten framesandfingers.When youcalloutanumber, e.g. 7, thechildrenshouldlook fora representation of7ontheircardandplacethecorrectnumeralon it.Thefirstplayertocovertheircardshouldshout, ‘Bingo!’If you wouldliketoinvolvethewholeclass, theothergroupsmaketheir ownnumber representationsontheirtable(usingmanipulatives).
P
Let’sstrengthen:
Aneasier version wouldinvolveusingcountersor stickynotestocoverthebingocard,insteadof numerals.
SuzietheEight-Legged Painter by BethanyGum
Read SuzietheEight-Legged Painter by Bethany Gum,inwhichSuziethespidermeetssomeinsects whoareafraidofherbecauseshehaseightlegs insteadofsix.Thisstorycouldleadtoadiscussion abouthowmanylegsdifferentanimalshave, for example:
● Howmanylegsdoesanant/agiraffe/abirdhave?
● Whichhasmorelegs:anantoraspider/anantor agiraffe?
● Doesanyanimalhave three/five/sevenlegs?
Whynot?
● Thechildrencouldthenmakeaspider,usingplay dough,aneggcartonandpipecleaners.
RolePlay If youhave ashopsetupinthePlay Area,askthechildrentosubitiseandthencount amountsofitemsintheshop.
PizzaSlices Youwillneedtwopaperplatesper child,smallnumerals1to8(seePCM1),glue,and scissors.Beforedistributingtheequipment,draw eightequalsectorsoneachpaperplate,giving you two‘eight-slicepizzas’perchild. Tellthechildrento gluethesmallnumeralsontooneoftheir‘pizzas’ (onenumeralperslice).Next,theyshoulddrawdots (‘pepperonipieces’):onedotononeslice,twodots onanotherslice,threedotsonanotherslice…andso on,uptoeight.Theyshouldthencuttheirpepperoni pizzaintotheeightslices,andplaceeachslice over thecorrespondingnumeralontheirotherpizza.
Bingo PlaythedigitalBingogame fornumerals
0to8.Beforeplayingthegame,printthedigital bingocardsandgiveeachchildacard.ClicktheBingo buttonto releaseaballandcontinueto releaseballs untilachildfillstheircardtowinthegame.
● Exploreshowthelayoutoforsizeofelementsinasethasnoeffectonthe overalltotal(conservationof number)(U&C)
● Subitises(looksatagroupofobjectsand realiseshowmanythereare,withoutcounting)numberof objectsinaset(U&C)
Digitalactivities:SubitisingandConservationof NumberA–E
MAM Routines: Reason& Respond/QuickImages
Concreteactivities:ConsolidationofNumber
Pupil’sBookpage44:ConsolidationofNumber (0−8)
● Manipulatives (bears,counters, beads,links, interlocking cubes, 2-Dand3-Dshapes)
● Thereisnonewmathslanguage forthislesson.
D Digitalactivities:SubitisingandConservation ofNumberA–E
MAM Routines: Reason& Respond/Quick Images
UsetheQuickImagesactivitiesandtheslideshows. Intheseactivities,thechildrenaresubitisingamounts onfiveframes,ontenframes,indifferentarraysand scatteredat random.Dothey realisethatitiseasier tosubitiseandcountwhentheobjectsareinlines (arrays)?Theywilllookattwodifferentsetsand respondtothequestion:‘Whatisthesameandwhat isdifferent?’
Teachingtip: Foreachslideorimage,ask:
● Doeseachsethave thesameamount?
Somechildrenmaythinkthatbecausethe objectsarespreadout,ortheobjectsare larger,thatthesethasagreater number ofobjects.
C Concreteactivities:ConsolidationofNumber Fortheseactivities,thechildrenshouldallhave the sametypeofmanipulative(e.g.counters).
Activity1:Subitising
DistributemanipulativesandafiveframefromPCM7 –FiveFramestoeachchild.Say/ask:
● Pickupahandfulofcounters.Can you guess how many youhave?
● Put yourcounterson yourfiveframe.
● Countthem.Howmanydo youhave?
● Howdid youshow yourset/countersonthefive frame?(e.g. for fourcounters,twocounters,then agap,thentwomorecounters)
● Can youpickup about fivecounters?Countthem.
● Putthemon yourfiveframe.Howmanydid you pickup?
Unit8: Numbers6to8
● Whopickedupexactlyfive?
● Optionalquestion:Whopickedup‘more’than five?‘Less’thanfive?
Let’sdeepen:
Usealargernumberofmanipulativesalongwith thetenframes,ifthechildrenare ready.Ask/say:
● Can youpickupaboutsevencounters(etc.)? Countthem.Putthemon yourtenframe.
● Howmanydid youpickup?Whopickedup exactlyseven?
● Optionalquestion:Whopickedupmorethan seven?Lessthanseven?
● Putsomecounterson yourtenframe.Guess howmanythereare.
● Nowcountthem. Were youcorrect?
Activity2:ConservationofNumber Tellthechildrentoput some countersintheirfive frame.Ask:
● Howmanycountersarethereon yourfiveframe?
● Howdo youknow?
● Can youmove thecounterson yourfiveframe (i.e.tonewpositions)?
● Howmanycountershaveyougotnow?(Dothe childrenneedto recount?)
Youmightliketotrytheabove activities without the ‘scaffolding’ofthefive/ten
frames, by askingthechildrentosubitisea random numberofmanipulativesandthencountthem.They shouldthenarrangethemanipulativesindifferent ways(vertical,horizontal,straightline,crookedline, arraysand/orpatterns).Assesswhetherthechildren needto recounteachtime.
Youmightliketodrawsomeoftheoptionsabove (verticalline,horizontalline,straightline, crookedline,arraysand/orpatterns)ontheIWB forchildrenwhomightneedinspirationorhelpin arrangingtheircounters.
Youmightliketotrytheseactivitiesusingmixed manipulatives(e.g.bigbearsandsmallbears,or largetrianglesandsmallsquares).Thechildren couldmake,e.g.asetoffivesmallbearsandaset offivebigbears.Askthemifthesetshave the samenumber.Spreadoutoneofthesetsandask thequestionagain.
P Pupil’sBookpage44:
Number(0−8)

Animation:BirthdayCake
MAM Routine: Reason& Respond
MathsStations:CompositionofNumber(1−8)
● Birthdaycandles (orcrayons)
● Manipulatives (bears,beads,links, interlockingcubes,2-D and3-Dshapes)
● Sortingringsordividers
● Thereisnonewmathslanguage forthislesson.
D Animation:BirthdayCake
MAM Routine: Reason& Respond
Teachingtip:
Understandingcompositionofnumbermeans thatthechildunderstandsthatnumbersare madeupofsmallernumbers.Theycan ‘seethe smallernumbers’insidethelargernumber.
Startwithsmallernumbers, forexample:1to5. Bringfivechildrentothefrontoftheclass.Ask:
● Howmanychildrenarehere?
Assesswhoneedstocountandwhocan subitise.Counteachchildandmove them aside,showingthatanumberismadeup of‘ones’.Thenshowthatthenumbers/childrencan jointogethertomakethesmallernumbersinthe biggernumber(e.g.1and1makes2;1and1and1 makes3. We putthe2and3togethertomake5.)
Ask:
● Howcould we breakupthisgroupoffive?(Start withmakingfiveseparate‘ones’ofthelineof children.)
● Let’sputthegroupoffivebacktogether.Could we breakthisgroupoffiveupinadifferentway? (Wecouldputthese fourchildrentogetherand move oneapart.)
● Isthereanotherway?(onefirstandthen four)
● Isthereanotherway?(twofirstandthenthree)
Continueuntilallthecombinationshave been explored.
Nowplaytheanimation.Promptthechildrento singalongwiththesong.Thecompositionofthe candlesonthebirthdaycake keepschanging;usethe animationtoexplorethebreak-up/compositionof numberswiththechildren.
Explorethecombinationof‘0and5’if appropriate for yourclass.
C MathsStations:CompositionofNumber(1−8)
Group1: Distributebirthdaycandles(orcrayons)and acopyofPCM9–BirthdayCaketoeachchild. Tell thechildrentousethePCMtomakecombinationsof sixonthecake.Allowthemtoengageinfreeplay first,makingtheir owncombinations.Next,askthem tomakesixofthesamecolourcandles/crayons. Then,askthemtousecandles/crayonsoftwo differentcolourstomakedifferentcombinations. (Alternatively,theycouldusecandles/crayonsoftwo differentsizes,oruseamixtureofcandles/crayons.) Theycould recordtheir favouritecombination by
drawingthecandles/crayonsonthePCM.
Groups2,3and4: Distributemanipulatives,sorting rings/dividersandsmallnumeralsfromPCM1toeach child.Allowthechildrentoengageinfreeplay, beforeaskingthemtomakecombinationsofthe numbers4to8,beginningwith4(3and1;2and2; 0and4;1and3;4and0).Theyshouldplacethe correctnumeralbesideeachsortingring,andexplain thecombinationstheyhave made.
Group5: Distributemanipulatives,small numeralsfromPCM1andacopyofthe tenframestoeachchild.Assesshowthe
childreninteractwiththemanipulativesandten framesbeforegivingthemanydirection. Tellthemto placeasmallnumeral5infrontofthemontheir table,andmakedifferentcombinationsofthat number,usingtheirtenframesandmanipulatives. (Move ontonumerals6,7andthen8oncetheyhave completedtheactivity for5.)
Dothechildrenunderstandthatthe manipulativescanbepositionedondifferent areasofthetenframe,butstillmakeupthe samenumber?
GamesBank Play‘HowManyBearsintheCave?’ fromtheGamesBank.
GamesBank PlaydominoesfromtheGames Bank.
FormtheNumber Thisactivityprovidesgood integrationwithPE.Dividetheclassintogroupsof one,two,threeand four.Calloutanumber(e.g.6). Thechildrenmust formthisnumber(twogroupsof three;agroupof fourandagroupoftwo,etc.).They shouldcount themselves toensurethatthetotalis correct.Willthey remembertocountthemselves?
GamesBank Play‘ShaketheCup’fromthe GamesBank.
NumberShapes Distributenumbershapes fornumbers1to8andsmallnumerals(seePCM 1)toeachgroup.Allowthechildrentoexplorethe equipment forthemselves(e.g.todiscoverhow differentnumbershapesfittogetherortwonumber shapesfitontopofone).Askthemtoplacethe numeral5infrontofthemandmakenumber-shape combinations forthisnumber.Theyshouldexplore the different combinationsthattheycanmake.They mightliketo recordtheirfindingsinamanneroftheir
choosing,butprovideguidanceifneeded.Continue withnumbers6,7andthen8.
Let’sdeepen:
Askthechildrentomakeasetofsixobjects.They shouldsubitiseandthencounttheobjects. Tell themtoputtwooftheobjectsinabowl.Ask:
● Howmanydid youputinthebowl?
● Howmanyareleft?
Arethechildrensubitisingtheamountcorrectly ordotheyneedtocount?
Ask:
● Can youmakeasetofeightobjectsquickly? Countandseeif youarecorrect.
● Can youmakesmalleramountswith yoursetof eightobjects?
● Can youmake another setofeightobjects? Makesmalleramountswiththissetofeight objects.
Didthechildrenmake thesame smallersets, e.g.2and2and2and2,ordidtheymake, e.g.4and4? Youcouldpromptthemtotry differentarrangements.
Digitalactivity:CompositionofNumber–
TenFrames
MAM Routine: Reason& Respond
MathsStations:CompositionofNumber(1−8)
Pupil’sBookpage45:ExtendingComposition ofNumber(1−8)
Teachingtip:
● Manipulatives(bears, beads,links,interlocking cubes,2-Dand3-Dshapes)
● Numbershapes
● Two-sidedcounters(redand yellow)
● Cuisenaire rods
If youdonothave NumberShapesin yourschool,afreeprintable versionis availableat: edco.ie/ynyv
● Thereisnonewmathslanguage forthislesson.
D Digitalactivity:CompositionofNumber–
TenFrames
MAM Routine: Reason& Respond Playtheslideshow, whichshowsdifferentcompositions ofthesamenumber.Thechildrenareasked:
● Whatisthesame?
● Whatisdifferent?
Thechildrenmustsubitiseandthencounteach number.Observewhetherthechildrencan voicethe difference, forexample:
● Thetoptenframehassixcounters.Ithasthree redcountersandthree yellowcounters.
● Thebottomtenframealsohassixcounters,butit has four redcountersandtwo yellowcounters.
Teachingtip:
Youmightliketo replaythisslideshow overa fewdays,asitwillbechallenging forsome childrenatfirst.
C MathsStations:CompositionofNumber1to8
Groups1and2*: Distributemanipulatives, smallnumerals(seePCM1)andten framestoeachchild.Allowthechildren toengagewiththeequipmentindependently. Dotheytryto replicatewhatwasontheIWB? Tell themtoplaceanumeral4infrontofthemontheir table,andmakedifferentcombinationsofthat number,usingthetenframesandmanipulatives. Continuewithnumbers5,6,7andthen8.Assess whetherthisgroupcan verbalisethedifference betweentwodifferentcombinations forthesame numbere.g. for6(2bluecountersand4 redcounters or3bluecountersand3 redcounters).
(* YoumightlikeGroup3todothisactivityas well,if numbershapesarenot available.)
C Group3: Distributenumbershapes fornumbers 1to8andsmallnumerals(seePCM1)toeach
child.Allowthechildrentoexplorethe equipment forthemselves(e.g.todiscoverhow differentnumbershapesfittogetherortwo numbershapesfitontopofone). Tellthemto placethenumeral5infrontofthemandmake number-shapecombinations forthisnumber. Theyshouldexplorethemany different combinationstheycanmake.Theymightliketo recordtheirfindingsinamanneroftheir choosing,butprovideguidanceifneeded. Continuewithnumbers6,7andthen8.
Group4: Distributetwo-sidedcountersandten framestoeachchild. Tellthechildrentomake numbersoftheirchoosing.Theycoulddraw/write eachnumbertheyaremakingonastickynoteor their MWB.Aftertheymakeacombination,they couldturn oversomeofthecountersandmakea differentcombination.
Group5: DistributeCuisenaire rodsandsmall numerals(seePCM1)toeachchild.Allowthe childrentoexplorethepropertiesofthe rods for themselves.Ask:
● Whatcan youtellmeaboutthis rod(e.g.thesixunit rod)?(Itisthesameasonepurpleandone red rod.)
● Howmanyofthewhite rod(theone-unit rod)are inthe red/purple rod?
● Placethenumeral6on yourtable.Howmany wayscan youmakethis rod(thesix-unit rod)? (sixwhite rods;one red rodandonepurple rod,
etc.Theycould recorde.g.allthecombinations for6ontheir MWB.)
P Pupil’sBookpage45: ExtendingComposition ofNumber(1−8)

EggCartons Distributeaneggcarton, beadsorplaydoughandsmallnumerals(seePCM1) toeachchild.Thechildrenshouldplaceanumeral besidetheeggcartonandmakecombinationsofthat numberusingbeadsorplaydoughoftwodifferent colourbeads.
Hangersand Pegs Thechildrencouldusea clotheshangerandclothespegsoftwodifferent colourstomaketheir own versionofa rekenrek.
GamesBank Play‘Throw theDice’fromthe GamesBank.
CompositionofNumber Thisdigital multiple-choiceactivitycanbeusedtoexplore differentcompositionsofnumber.
● Showsanunderstandingofdifferencesinvalue(U&C)
● Usescomparativelanguage(more,less,same)tocomparesetstoatleast 10(C)
● Usesappropriategesturesand wordstoconveyandmakecomparisons (C)
● Identifies, recognisesandestimates‘more’or‘less’inthe real-lifecontextand/orplay(R)
D C P
Digitalactivities:More,Less,theSameA–C
MAM Routine: Reason& Respond
Concreteactivity:MakingEquivalentand Non-equivalentSets
Pupil’sBookpage46:EquivalentandNonequivalentSets(0−8)
● Manipulatives(bears, counters,beads,links, interlockingcubes,2-D and3-Dshapes)and collectionsofsmall objectsfromnaturewalks(leaves, twigs,pinecones,etc.)
● alot,some,onemore,oneless
Teachingtip:
Usethislanguageateveryopportunitythroughouttheday/week, forexample:
● Lilyhassixmarkersand youhave fivemarkers.Whohasmore?Howmanymore?Whohasless?
● Therearesixbuttonson Karim’scoatand fourbuttonsonJack’scoat.Whosecoathasmore buttons?Whosecoathasless?
D Digitalactivities:More,Less,theSameA–C MAM Routine: Reason& Respond
Playthemultiple-choicegames,whichinitially focus ontheconceptofequivalentandnon-equivalent sets.Insomecomparisonsitisvisuallyobviouswhich sethasmore.Thechildren’sattentionisdrawntothis aspectofcounting.Alsocovered:
● Whichsethasless?
● Whichtwosets(outofthreesets)have thesame amount?
Teachingtip:
Thegamesillustratethe needtocount when thedifferenceincardinalityisnotobvious,as opposedto,e.g.asetofeightobjectsanda setoftwoobjects,whereitis visuallyobvious toachildwhichsethasmore,andthatoneset has‘alot’more.
C Concreteactivity:MakingEquivalentand Non-equivalentSets
Distributemanipulatives,smallnumerals(seePCM1) andtenframestoeachchild. Tellthechildrento maketwosets:onewitheightobjectsandonewith twoobjects.Ask/say:
● Whichsethasmore?Howdo youknow?Do you need tocount?Countandcheckthat you were right.
● Whatcan yousayaboutthisset(thesetwith eight)?Doesthissethave ‘alot’morethanthe otherset?
● Whichsethasless?Howdo youknow?Do youneed tocount?Countandcheckthat you were right.
● Whatcan yousayaboutthisset(thesetwith two)?Doesonesethave ‘alot’lessthanthe other?Assesswhetherthechildren realisethereisnonecessitytocount whenthedifferenceintheamountis visuallyobvious.Canthey verbalisewhythereis noneedtocount? Forexample:‘Itlookslikethis sethasmore;Idon’tneedtocount;itlookslike thissethasless.’
Manychildrencannotgrasptheconceptof‘less’ immediately.Theterms‘more’and ‘same’occur intheireverydaylife,sotheyunderstandthese concepts,but‘less’canbechallenging.Some explicitteachingtimemayneedtobespenton thisterm.
Tellthechildrentomaketwosets:onewithfive objectsandonewith fourobjects. Wouldthis work?
−Canthechildrenvisuallyseewhichsethasmore? (No,becausetheamountsaretoosimilar.)Ask:
● Whichsethasmore?Do youneedtocount? Countandcheckthat you were right.
● Whatcan yousayaboutthisset(thesetoffive)? Doesithave ‘alot’morethantheotherset,or just ‘some’more?
Howmanymoreobjectsdoestheothersethave?
● Whichsethasless?Do youneedtocount?Count andcheckthat you were right.
Unit8:
● How would youmakethesetsthesame?(Add onemoretothesetof fourortakeawayonefrom thesetoffive.)
Tellthechildrentoplacethecorrectsmallnumeral besideeachsettheyaremaking,toconsolidate givingeachsetits‘numbername’.Theycouldthen usethetenframestoshowequivalentandnonequivalentamounts.
Pair work: Onechildshouldmakeanumberontheir tenframe.Theirpartnercanmakeanumberwithone more;thesamenumber;anumberwithoneless.
P Pupil’sBookpage46: Equivalentand Non-equivalentSets(0−8)

Book Read More by EmmaChichesterClark, whichexplorestheconceptof‘more’.
GamesBank Play‘OneMore!’fromthe
GamesBank
Nature Walk Goonanaturewalk,if feasible. Collectpinecones, feathers,leaves,twigs,etc.,and usethesetomake ‘sets’backintheclassroom,.
NumberShapes Distributenumbershapes for numbers1to8andsmallnumerals(seePCM1)to eachgroup.Ask:
● Can youshowmeanumbershapewith four holes?Can youfindanothernumbershapethatis thesame?Can youfindnumber shapes thatwill ‘make four’?(e.g.twoshapeswithtwoholes)
● Can youshowmeanumbershapethathasmore holes/lessholes/onemorehole/onelesshole?
● Can youputthecorrectnumeralbesideeach numbershape?
Pair work Onechildhas,e.g.anumbershape withsixholes.Theirpartnermustswapthemthe equivalentamount,usingtwonumbershapes.
Let’sdeepen:
Onechildhas,e.g. two numbershapestotalling seven.Theirpartnermustswapthemthe equivalentamount,usingtwonumbershapes.
MoreorLess– TenFrames Playthisdigital multiple-choicegametocomparesetsusingtenframes.
Digitalactivity:OrderingNumeralswith
Book: Six-DinnerSid by
47:OrderingNumerals andSets(1−8)
● Thereisnonewmathslanguage forthislesson.
D Digitalactivity:OrderingNumeralswithMonty MAM Routine: Reason& Respond
Playtheinteractivegame,inwhichthechildrenhave toarrangethecones(withthenumerals1to8)inthe
correctorder.Observewhetherthechildrencanput thenumeralsinthecorrectorder.Aresomechildren havingdifficulty?
C Concreteactivity:OrderingNumeralsandSets Distributemanipulatives,numbershapes, Cuisenaire rodsandsmallnumerals(see PCM1)toeachchild.Askthechildrento arrangethenumerals1to8inorder,horizontallyor vertically(oroneway, andthentheotherway),on theirtable.Next,tellthemto:
● Placethecorrectnumberofmanipulatives(e.g. links)besideeachnumber(countingcarefully).
● Subitiseamountsandputthesetsinorder,then counttocheckifthey were correct.
● Maketowersofinterlockingcubes,placingthe correctnumberofcubesbesideeachnumber.
● Placethecorrectnumbershapeabove each number.
● PlacethecorrectCuisenaire rodabove each number.
Askthechildrentoputtheirnumerals1to8inorder (withoutusingmanipulatives).Say/ask:
● Placeonenumeralonits own.Whatcomesnext/ before?Assesswhetherthechildrencanputthe numeralsandthenthesetsinthecorrectorder (andthenassignthecorrectnumbertoeachset) oraresomechildrenhavingdifficulty?
● Placetwonumerals(e.g.6and8)ontheir own. Whatnumeralcomesinbetween?
● Placethenumerals2and6onthetable.What numbersaremissing?Can youputtheminorder?
● Putthenumeral7onits own.Lineupallofthe numbersthatcomebeforethisnumber.
● Putthenumeral3onits own.Lineupallofthe numbersthatcomeafterthisnumber.
● Placethenumerals6and4onthetable.Putthem inorder:whichcomesfirst?Whichisthesmallest/ largestnumber?
● Placethenumerals6,4and8onthetable.Put theminorder:whichcomesfirst?Whichisthe smallest/largestnumber?
Promptthechildrentousethe followinglanguage: before,after,in-between.Ask:
● Whatcan youtellmeaboutthisnumber(e.g.6)? (Itcomesafter5,before 7, inbetween5and 7.)
● WherecouldIputthenumber7?
Inpairs,thechildrenmixupthenumerals 1to8andthenarrangetheminthecorrectorder. ChildAclosestheireyeswhileChildBtakesaway anumeral.CanChildAsaywhichnumeralis missingwhentheyopentheireyes?
DistributemanipulativesandacopyofPCM 10 −OrderingFrametoeachchild.Theordering framehasthenumerals1to8alongthebottom. Thechildrenplacethecorrectnumberofobjects ineachcolumn.
Thechildrencouldmakeapatternusing interlockingcubes.Examples: forthenumber4− one red,onegreen,one red,onegreen; forthe number6−one red,oneblue,one red,oneblue, one red,oneblue.
Let’sDeepenPCM– WouldThis Work?The childrenusethePCMtopotentiallydiscoverthat ABpatternsonly‘work’ forevennumbers.Dothey realisethatthepatterndoesnot‘work’ for number 7, butit works fornumber8?
Playtheinteractivegameinwhichthechildrenfillin theblanktocompletealineofnumberedcones.
P Book: Six-DinnerSid by IngaMoore Read Six-DinnerSid by IngaMoore,whichtellsthe storyofacatwhogoestosixdifferenthousesand eatssixdifferentdinnersaday.
Teachingtip:
A readingof Six-DinnerSid is available at: edco.ie/5ew4
P Pupil’sBookpage 47: OrderingNumerals andSets(1−8)
GamesBank Play‘PiedPiper’fromthe GamesBank.
VisualArts Thechildrencoulddraw/paint/build thesixhousesthatSidthecatvisits.Theycoulduse
smallnumerals1to6(seePCM1)toputthecorrect numberoneachhouse.
● Makesnumeralscreatively (C)
● Discusses,drawsandwrites representationsofnumbers1−8,usingmanipulatives (C)
● Explorestheuseofnumberandplaysgamesto raiseawarenessofnumberintheirenvironment(A&PS)
● Noticesand recognisestheuseofnumeralsaslabelsinthecontextofhome,theclassroomandthe schoolenvironment(U&C)
Digitalactivity:WhichNumberAmI?
MAM Routine: Reason& Respond
Animations:Number Formation–0to8
Concreteactivities:MakingNumerals
Pupil’sBookpage48: WritingNumbers0to8
● Thereisnonewmathslanguage forthislesson.
● Classroom-sizednumerals(oradisplayontheIWB)
● Playdough
● Sandarea
● Buttonsand/orpebbles
● Linksand/orinterlockingcubes
● Cordand/or wool
● Countersand/orbeads
D Digitalactivity:WhichNumberAmI?
MAM Routine: Reason& Respond Playtheinteractivegame,whichslowly revealseach numeralfrom0to8.Thechildrenmustguesswhich
numberwillbe revealed.Askthechildrensaythe nameofthenumberassoonasthey recogniseit.
D Animations:NumberFormation–0to8
Playthenumeral formationanimations foreach numeral.Theydescribethe formation veryclearly.
C Concreteactivities:MakingNumerals
Begin by tellingthechildrentotracethenumerals 0to8ontheirtableorintheairwiththeirfinger. Drawtheirattentiontotheclass-sizednumerals(or displayontheIWB),whichtheycanuse for reference. Describethe formofeachnumeral,oraskachildto describeit, forexample:
● Howdo we makea6?Startatthetop…
● Howdo we makea7?
● Howdo we makean8?
Teachingtip:
To bemathematicallycorrect,thechildrenare ‘forminganumeral’,butthephrase‘makea number’willbemore familiartothem.
Distributetheequipmentlistedbelowtoeachgroup.
● Group1:playdoughand/oraccesstothesand area(todrawnumeralsinthesandwiththeir finger)
● Group2:pebblesand/orbuttons
● Group3:linksand/orinterlockingcubes
● Group4:cordand/or wool
● Group5:countersand/orbeads
Instructthechildrentomakethenumbers0to8 innumericalorder,usingtheirequipment.Ask eachgroup:
● Whichnumbersareeasiesttomake?(Perhaps1,4 and7areeasiest,becauseofthestraightlines.)
Teachingtip:
Youmightliketomixuptheequipment, andaskthechildrentoseeiftheyfindit easierto formthenumeralswithdifferent combinationsofequipment. Forexample, ifusingcubesandcord,theycouldusethe cubes forthestraightpartsofanumeral andthecord forthecurvingparts. Forming numeralsinthiscreativewayhelpschildren tointernalisetheshapeand formofeach numeral.
Holduptheclass-sizednumeral6,andask:
● Can youuse your…(e.g.playdough)tomakethis number?
● Whatnumbercomesafter6?
Holduptheclass-sizednumeral 7, andsay:
● Use your…(e.g.playdough)tomakethisnumber.
Ask/say:
Holdupsixfingers,andask:
● Howmany?(6)
● Howmanychildrenarethereatthistable?(Hold uptheclass-sizednumeral.)Makethisnumber.
● Whatageare you?(Holduptheclass-sized numeral.)Makethisnumber.
● Howmanywindowsarethereintheclassroom? (Holduptheclass-sizednumeral.)Makethis number.
● Whatnumbercomesinbetween6and8?(Hold uptheclass-sizednumeral.)Makethisnumber.
● WhatnumberamImakingintheair/onthewall? (Holduptheclass-sizednumeral.)Makethis number.
● Whichnumberisbigger:3or6?(Holdupthe class-sizednumeral.)Makethisnumber.
● Whichnumberisless:8or4?(Holduptheclasssizednumeral.)Makethisnumber.
DistributeacopyeachofPCMs11−13–Large DottedNumerals6to8toeachchild.The childrenshouldtrace overthenumeralsand practisewriting6,7and8.
Onechildinagroupshouldchooseanumeral andwriteitontheir MWB.Usingmanipulatives, everyotherchildmakesasetcontainingthat numberofobjects.
Let’sdeepen:
Thechildrenshouldwritethenumerals0to8 intheircopy.
P Pupil’sBookpage48: WritingNumbers0to8

MathsEyes Askthechildrenlook fornumerals 0to8insideandoutsidetheclassroom. Tellthemto beonthelook-out fornumeralsathomeandontheir waytoschool,and reportbacktotheclass.
GamesBank Play‘NumberWhisper’fromthe GamesBank.
BodyNumerals Thisactivityprovidesgood integrationwithPE. Tellthechildrentomakespecific numeralsonthefloorofthePEhall,usingtheirbody. Startwiththeeasiestone−thenumeral1.They couldalsodothisingroups.
Carryoutawarm-upactivityof yourchoicefromoneofthelessonsinthisunit.
Choosefromthismenuofactivityideas,orchoose your ownwaytobeststructurethislastlessontosuit your needsandtheneedsof yourclass.
Mathsstory
Readoneofthesuggestedstoriesthat youmaynot have hadtimeto read.
Mathslanguage
Askthechildrentousetheirfingersto formthe numerals6,7and8.Theymightneedhelpfroma partner.Promptthemtousethe followinglanguage: after,before,inbetween.Theycouldalsodrawthe numbersontheir MWB.
ProgressAssessmentBooklet
Completequestionsxx−xxonpagexx.Alternatively, thesecanbelefttodoaspartofabigger review duringthenext review week.
Identifychildrenwhomightbenefitfromextra practicewithsomeofthe keyconceptsorskills inthisunit.ConsulttheUnit8Let’sStrengthen Suggestions for TeachersortheLet’sStrengthen PCM forparalleltasks.
Let’splay!
PlayoneofthegamesorPEactivitiesthat youdid notgetachancetotryout.
Choralcounting
Chantthenumbers1to 10 or revisittheanimation titled‘CountwithMonty’.
Mathseyes
Tellthechildrentofindnumbersandquantitiesof objects(6−8)insideandoutsidetheclassroom.
UsetheLet’sDeepenPCM.
Tracethenumerals.Drawyourownseto
Placethecorrectnumberof objectsormanipulativesinthe frames.
Choose2colourstomakeapattern foreachnumber.Forexample,the dinosaurs fornumber 4 couldbecolouredred,blue,red,blue.Doesyour pattern‘work’ foreverynumber?Whichnumbersdoesit‘work’ for?
Class Teachers: Usetheseactivitiesalongsidethespecifiedlessons,tostrengthentheunderstandingof children,as required.Manyoftheseactivitiescouldalsobeusedasastation.
SpecialEducation Teachers: Usetheseactivitiestosupportchildren(pre-teachingor re-teaching)inin-class and/orwithdrawalsessions.
Accuratecountingisa keyskill forchildrentolearnfromanearlyage.When youngchildrenbegincounting,they count‘byheart’or‘by rote’.Theycansaythenumbernamesinorderbecausetheyhave memorisedthe wordsand theirsequence.Childrenthenneedtoengagewiththefivecountingprinciples.Thefiveprinciplesare:
Stableorderprinciple
● Inordertomasterthisprinciplethechildrenneedtobeabletocountinthecorrectorder.Thechildrencanpractise by learning numberrhymesandsongs,andlisteningtonumberstories.Theycancountduringtheschooldayandathome.Theycanplay countinggamessuchasHopscotch.InUnit8,thechildrenneedtobeabletocountupto8.Iftheycancountupto8,theycan thentrytocount8objects.
One-to-oneprinciple
● Oncethechildcancountinthecorrectorder(andrealisethatthenumbersmuststayinthatorder),theyneedtobeableto assignanumber(verbally)toeachitem.Whencountingobjects,thechildrencouldfirstlineuptheobjects.Thiswillhelpthem toassignanumbertoeachobjectandkeeptrackoftheircount.‘Slowandsteady’isthekeyphrase;thechildrencarefullytouch eachobjectandsayitsnumbername.Theymightliketomovetheobjectaside.Ifthisprocessisnotpractised,thechildmay countoneobjecttwiceorusethesamenumberforafewobjects.
Cardinalprinciple
● Thisprincipleinvolvesthechildknowingthatthefinalnumberinthecountisa‘special’number.Thisspecialnumbertellsthem ‘howmany’objectsthereareintheset.Thechildcannowanswerthequestion:‘Howmany?’Encouragethemtoarticulatethe answerinfulle.g.‘Thereare5bears.’Plentyofcountingofconcretematerialsshouldbepractised,withthequestionbeing posed:Howmany?Andthefullanswerbeinggivenbythechild.
Abstractionprinciple
● Apre-requisiteformasteringthisprincipleisunderstandingthattheobjectsinasetcanbedifferentcolours,shapes,sizes. Forexample:Thechildcouldpractisecountingasetof6thatcontains:1yellowbear,2differentcolourcircles,1button,1cube, 1link.Cantheyanswerthequestion:Howmany?Thereare6objects.Theycanthenmoveontoabstractcounting,e.g.sounds (coinsbeingdroppedintoabowl);tappingonadesk;thenumberoftimesachildcanhopononefoot;thepostersonthewall, etc.Thechildrencouldpractisethisprinciplebycountingrandomobjects,e.g.theitemsontheirdesk(pencils,eraser,copy, twocrayons).InthePEhall,theycouldcounthowmanytimestheycanthrowandcatchabeanbag.Inpairs,onechildcloses theireyeswhiletheotherchilddropscubesintoabowl.
● Practiceactivitiescouldincludeinitiallycountingasetof concrete objects.Thechildrencounttheobjectsfromlefttoright, righttoleft.Theyarrangetheobjectsverticallyandcountfromthebottomup,thenfromthetopdown.Theycouldarrangethe objectsinaroughcircleandcountthem.
● Thisprincipleisalignedwithconservationofnumber.Thechildrenunderstandthatevenwhenthecountedobjectsaremoved, thetotalcountremainsthesame.Practisethisconceptbyaskingthechildrentocountasetofobjectsandgiveyouthefinal count.Movetheobjectsandaskhowmanytherearenow.Thechildrencouldthentrycountingobjectsthatcannotbetouched, e.g.chartsonthewall.Howmanyarethere?Whichwaydidyoucountthem?Canyoucounttheminadifferentway?Didyou getthesameanswer?
MatchingNumerals(0to8)toSets
● Havingconsolidatedthefiveprinciplesofcounting,thechildrencouldmoveontopractisingassigningnumeralstosetsof objects.Startwiththesmallernumbers(1to5).Thechildrencountouttwoobjects.Whichnumbermatchesthisset?Canthe childselectthecorrectnumberandmatchittotheset?Continueupto5.Showthechildanumber,e.g.5.Cantheyidentifythe numberandcountoutfiveobjectstomatchthenumber?Ifappropriate,continuewiththenumbers6,7,8.
CompositionofNumber(2to8)
● Useonlyonetypeofmanipulativeatfirst,e.g. redcubes.Givethechildrenasetof, forexample,3 redcubes.Howmany?Ask themto‘move/divide/separate’theobjects,e.g.2and1;1and2.Theycounttheindividualcubesorcubeandthenputthem all‘backtogetheragain’.Howmany?Continueupto8ifthatisappropriate for yourgroup. Trygivingthechildren5objects (youcouldusetheSmall World Farmanimals).Howmanyanimals?Thereare5animals.Can youmove/divide/separatethem? Howcould youdothat?(2sheepand3cows;Ibiganimaland4smallanimals). Trythiswithnumbers4to8(as youdeem appropriate).Usethetenframe,double-sidedcounters,andsmallnumerals(2to8)toshowcompositionifthechildrenare ready forthisstage.
EquivalentandNon-EquivalentSets
● Emphasisethemathslanguagehere,e.g.the words‘more’,‘less’,‘thesame’.Childrenfinditeasiertounderstandtheconcept of‘more’sobeginwithsetsthathave ‘more’. Trytousethelanguageduringtheday, e.g.‘Whohas more Lego/grapes/crayons?
Whohas less?’Intheseinstances,thechildrenwillbesubitisingandmakingavisualdecision. Forconcreteactivities,cubesand Cuisenaire rodsare verysimpleandclearintermsofequivalenceandnon-equivalence.‘More’,‘less’,and‘thesame’canbe determinedvisuallyandthenchecked by counting,e.g.thecubesintwotowersside by side.Thechildrencouldcountthecubes intwosetsfirst,thenmaketwotowerstocompare‘height’andcountagain.Ensuretheyusetheirmathslanguagetoanswer yourquestion:
—Whichtowerhasmorecubes?Thisonehasmorecubes.
—How do youknow? (Thisisa keyquestiontoascertainwhetherthechildrenunderstandtheconceptofonetowerhaving morecubesthantheothertower. Theyarenotcountingallthecubesinonetowerbutnoticingthedifferencebetween them.)
● Thechildrencouldstart by puttingajumbledupsetofnumbersinorder.Whichnumbergoesfirst?Next?Thenputsetsof2,3,4 objectsonthedesk.Canthechildputthesetsinorder?
● Thechildrencouldfirst formthenumeralscreatively;theyuseplaydough,straws/piecesofstraws,beadsonstring.Inengaging intheseactivities,thechildrennoticetheshape(curves/lines)ofeachdifferentnumeral.Theycoulduselargenumeralsand coverthemwithcubes/smallmanipulatives.Theyusethe raisednumeralsintheirPupil’sBook(ordraw thenumeralsinthe sandbox,and youmayhave theMontessoriSandpaperNumbers).Whenthechildrenarecomfortablewritingthenumerals ‘creatively’,theycouldtrywritingthenumber1withacrayon,thenapencil.Move ontotheothernumbersas youseefit.Use Number FormationRhymestotakeany‘pressure’outofwritingthenumbers.
Notetoteachers:
Numberof players: 2to10
Youneed: Hopscotchgrid,smallobjectperchild(e.g.small stone)
Howtoplay: Mark outahopscotchgridintheyardorPEhall (oruseacommercialversion).Thechildrenplay hopscotchandpractisetheircountingskills.Each childtakesaturntothrowasmallobject(e.g. astone)ontooneof thesquaresonthegrid.If theysucceed,theythenstartcountingasthey hopontoeachsquare.Theyhopoverthesquare withtheirobjectonit.Theymusthoptotheend of thegridandback againwithouthoppingon aline,missingasquareorlosingtheirbalance. If theydoso,theymustwaittheirturnagain (butthistimetheystartattheirobject).The childwholandstheirstoneback onthestarting squarewins.
Strengthen: Usenumbers1to8 forthegrid.
Deepen: Usenumbers1to10 forthegrid.
Title Throw theDice (orSpintheSpinner)
Numberof players: Groupof children,e.g.6–8
Youneed: Diceorspinner,2-sidedcounters(8counters each),ten frame, five frame,smallsticker
Howtoplay: Onechildthrowsthedice.Theotherchildren eachmakethenumberthrown(ontheirten frameusingtwo-sidedcounters).The firstchild tomaketheirnumbercorrectlywins.Theyplay again.
Strengthen: Thechildrenusetheir five frametomakethe numbers(coverthenumber6onthedicewitha sticker).
Deepen:
Eachchildtakesaturntomakeanumberon theirten frame.Theotherchildrenclosetheir eyeswhilethenumberisbeingmade.Theyopen theireyesandthe firstchildtosaythenumber correctlywins.
Title OneMore!
Numberof players: Wholeclass
Youneed: PEhall
Howtoplay: BringthechildrentothePEhallandcallout anumber,e.g.sevenThechildrenmust form groupsof seven.Callout:‘Onemore!Make (eight!)’Thegroupsmustadd onemore totheirnumber.Childrenwhoareleftoverand cannotmakeupagroupof eightareout.The childrendispersearoundthehall.Keepcalling outdifferentnumbersuntilonlyonegroupisleft. Theyarethewinners!
Strengthen: Usesmallernumbers,e.g.6orless.
Deepen: Callout‘Oneless!’insteadof ‘Onemore!’
Day1,Lesson1: Counting1to8
● Recites forwardtoatleast 10 (U&C)
● Recognisesnumbers,initiallywithin 10 (U&C)
● Demonstratesanawarenessofanduses numeralsinpersonallymeaningfulcontexts (C)
● Attendstonumeralsofsignificanceor importancetothechild(U&C)
● Usesappropriatestrategiestofindouthow many(A&PS)
Day2,Lesson 2:MakingSets (0−8)
● Demonstratesanawarenessofnumberand number wordsequencingthroughsong, stories,rhymesandgames (C)
● Participatesinactivitiesthatinvolve communicatingaboutnumber(U&C)
● Usesideasaboutnumberandquantityto communicatewithothers (C)
● Exploreshownumbersareused forcounting andthatthelastnumberinthecount indicatesthequantityofobjectsinaset (U&C)
Day3,Lesson 3:Matching Numeralsto Sets(0−8)
● Recognisesthatobjectsandsymbolscan representnumbers(U&C)
● Matchesnumeralsandnumber wordstosets andtoothernumeralsinavarietyof contexts(A&PS)
● Selectsandusesappropriatematerialsto makeavarietyofsets foragivennumber (A&PS)
● Sorts,groupsandarrangesmaterials accordingtocriteria(R)
● Establishesthat zero,asanumeral, representsnothing/noneintermsof quantity(R)
Day4,Lesson4: Consolidation ofNumber (0−8)
● Exploreshowthelayoutoforsizeof elementsinasethasnoeffectonthe overall total(conservationofnumber)(U&C)
● Subitises(looksatagroupofobjectsand realiseshowmanythereare,without counting)numberofobjectsinaset(U&C)
Day5,Lesson5: Compositionof Number(1−8)
● Investigatesvariousarrangementsof manipulativestopromptdifferentmental imagesofnumbersupto8,whiledeveloping asenseofeachnumber(R)
Day6,Lesson
6:Extending Compositionof Number(1−8)
Day 7, Lesson7: Equivalentand Non-equivalent Sets(0−8)
● Investigatesvariousarrangementsof manipulativestopromptdifferentmental imagesofnumbersupto 10,while developingasenseofeachnumber(R)
Day8,Lesson
8:Ordering Numeralsand Sets(1−8)
● Showsanunderstandingofdifferencesin value(U&C)
● Usescomparativelanguage(more,less, same)tocomparesetstoatleast 10(C)
● Usesappropriategesturesand wordsto conveyandmakecomparisons (C)
● Identifies, recognisesandestimates‘more’or ‘less’inthe real-lifecontextand/orplay(R)
● Ordersnumeralsuptoatleast 10 (U&C)
● Orderssetswithoutcountingandchecks by counting(R)
● Explainsordinalityusingthelanguageof after,beforeandin-between (C)
Day9,Lesson 9: Writing Numbers0to8
● Makesnumeralscreatively (C)
● Discusses,drawsandwrites representations ofnumbers1−8,usingmanipulatives (C)
● Explorestheuseofnumberandplaysgames to raiseawarenessofnumberintheir environment(A&PS)
● Noticesand recognisestheuseofnumerals aslabelsinthecontextofhome,the classroomandtheschoolenvironment (U&C)
Day 10,Lesson 10: Reviewand Reflect
● Reviewsand reflectsonlearning(U&C)