9.HYPERBOLIC PARABOLIC MODEL-GRID SHELL STRUCTURE

9. HYPERBOLIC PARABOLIC MODEL-GRID SHELL STRUCTURE

As we know from mathematical diagrams, the hyperbolic paraboloid is a ruled surface, which means that we can create it using only straight lines even though it is curved. Fig. 9.1 shows our models of a hyperbolic paraboloid made of fifteen straight 30cm and 90cm long wood elements. First, we created an equilateral triangle using three elements of our straight timbers. Then we used this triangle as a template to form two skewers at a 60-degree angle, completing 2/3 of another equilateral triangle. Connecting the first triangle by one skewer to the second triangle, created together a regular tetrahedron shape. Further, we marked the edges of the tetrahedron in regular 4 cm intervals to connect the skewers between the marks. Then we connected a skewer to the first marks on each of the edges. In another step, we created another layer of skewers so that they would overlap. We kept connecting skewers by moving one interval mark on each edge. The overlap got greater each time. To reach the goal we had to use two of the other edges to form the other ruling lines.

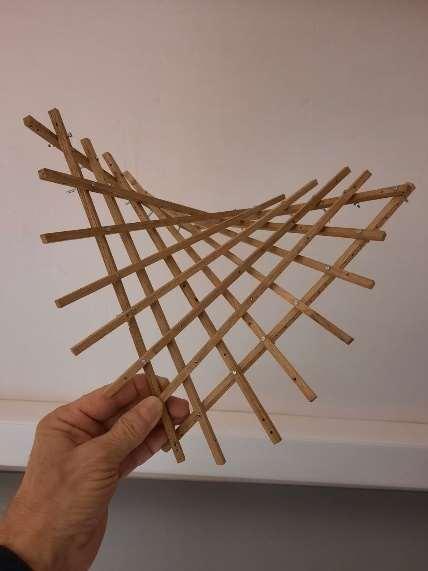
Fig. 9.1. Hyperbolic Paraboloid Model (Shawkat, 2021)

REFERENCES

Otto, F., Trostel, R. (1967). Tensile Structures, vol 2, MIT Press R.B. Fuller, R. Marks, The dymaxion world of Buckminster Fuller, New York, Anchor Books, 1973.

R.B.Fuller, Tensile-Integrity Structures. U.S. Patent 3,063, 521, 13 November 1962. K. Snelson, Continuous Tension, Discontinuous Compression Structures. U.S. Patent 3,169, 611, 16 February 1965. D.G. Emmerich, Construction de Reseaux Autotendantes. French Patent 1,377, 290, 28 September 1964.

Shawkat, S. (2019). The Art & Engineering of Lightweight Structures. Tribun EU, Brno, 308 pp. Shawkat, S. (2021). Structural icons for architects, Tribun EU, Brno, 840 pp. Shawkat, S. (2021). Time Space Existence 2021, European Cultural Centre, Grafiche Veneziane Venice, Italy, pp. 550. Shawkat, S. (2019). Behavior of Steel Structures. Tribun EU, Brno, 312 pp. Bach, K., Burkhardt, B., Otto F. (1988). IL 18, Seifenblasen, Karl Kramer Verlag,Stuttgart, 400

pp. Foster, B. (1998). The Integration of Large Fabric Structures within Building Projects including the Significance of Design and Procurement Methods. Proceedings of IASS – LSAA Symposium Foster, B., Mollaert, M. (2004). European Design Guide for Tensile Surface Structures. Tensinet, Brussels.

Schleicher, S., Lienhard, J., Fleischmann, M. (2010). Forschungspavillon ICD/ITKE, Detail, online: http://www.detail.de/artikel forchungspavillonuniversitaet- stuttgart 26600 De.htm Bletzinger, K.U., Firl, M., Linhard, J., Wüchner R. (2008). Optimal shapes of mechanically motivated surfaces.

In Computer Methods in Applied Mechanics and Engineering. Bletzinger, K.U., Wüchner, R., Daoud, F., Camprubi, N. (2005). Computational methods for form finding and optimization of shells and membranes. In Computer Methods in Applied Mechanics and Engineering, Vol. 194 Bletzinger, K.U. (2014). Structural Optimization, Lecture Notes, TU Munich, Bletzinger, K.-U. Ramm, E. Structural optimization as tool for shape design, in: Hirsch, C. et al., (Eds.), Numerical Methods in Engineering 92, Elsevier, Amsterdam, New York, 1992 Motro, R. (1992). Tensegrity systems. The state of the art. Int. J. Space Struct. 1992, 7, 75–83.

Motro, R. (2003). Tensegrity. Structural Systems for the Future; London, UK. Imprint: Butterworth-Heinemann, ISBN: 9780080542348 Chilton, J. C., Choo, B. C. and Yu, J. (1994). Morphology of reciprocal frame 3-dimensional grillage structures. In Spatial Lattice and Tension Structures, Proceedings IASS-ASCE (Abel, J. F., Leonard, J.W. and Penalba, C. U., eds), Atlanta, GA, pp. 1065–1072. Chilton, J.C., Choo, B. S. and Popovic,O. (1995). Reciprocal frames past, present and future. In Proceedings of the International Conference in Lightweight Structures in Civil Engineering (Obrebski, J. B., ed.), Warsaw, Poland, September, pp. 26–29. Magat-Magdalena Burska, Warsaw, Poland.

I. Peterson, Fragments of Infinity, A Kaleidoscope of Math and Art, John Wiley & Sons, 2001, p. 138-141.

V. Petresin, R. Laurent-Paul, “The Double Möbius Strip Studies”, Nexus Network Journal, Vol. 4, 2002. Z. Hadid, The Complete Buildings and Projects, Thames, and Hudson, 1998, pp. 110-115. J. Thulaseedas and R. J. Krawczyk, Möbius Concepts in Architecture, College of Architecture Illinois Institute of Technology Chicago, IL, 60616, USA, info on https://arch.iit.edu/ Kolar, V., Nemec, I., Kanicky, V. (2001). FEM principy a praxe metody konecnych prvku, Computer Press, Brno, 401 pp. Sabmeethavorn A. (2013). Studio Detailing and Patterning, IMS Dessau, Germany Senk, P. (2018). Capsules: a typology of other architecture, Routledge, London, 207 pp. Pottmann H 2007 Architectural Geometry Bentley Institute Press 1st edition Meades J. (2008). “Zaha Hadid: The First Great Female Architect”. Intelligent Life Magazine. Accessed April 18th, 2012. http://moreintelligentlife.com/story/zaha-hadid. Michaud J. (2011). “Zaha Hadid: The Lady GAGA of Architecture”. The New Yorker. Accessed June 25th, 2012. J. Thulaseedas and R. J. Krawczyk, Möbius Concepts in Architecture, College of Architecture Illinois Institute of Technology Chicago, IL, 60616, USA, info on https://arch.iit.edu/ C. J. Mathews, Some Novel Möbius Strips, Mathematical Teacher, Vol. 65, 1972, p. 123-125.

Other books by Sabah Shawkat

1. Design of reinforced concrete members (2000) 2. Železobetónové konštrukčné sústavy (2002) 3. Deformation behaviour of reinforced concrete beams (2011) 4. Konštrukčné projekty (2014) 5. Structural design I (2014) 6. Structural design II (2015) 7. Architektonika (2015) 8. Structural design III (2015) 9. Structural projects (2016) 10. Element design to shape a structure I. (2016) 11. Element design to shap e a structure II. (2017) 12. Inžinierske drevené konštrukcie (2017) 13. The art of structural design (2017) 14. Lightweight steel structures (2018) 15. The art and engineering of Lightweight Structures (2019) 16. Art in/of Nature (2020) 17. Application of structural system in building design (2020) 18. Structural icons for architects (2021) 19. Construction in architecture (2022) 20. Lightweight structures from the perspective of formfinding geometry (2022) 21. Free Form Geometry in Architecture (2022)