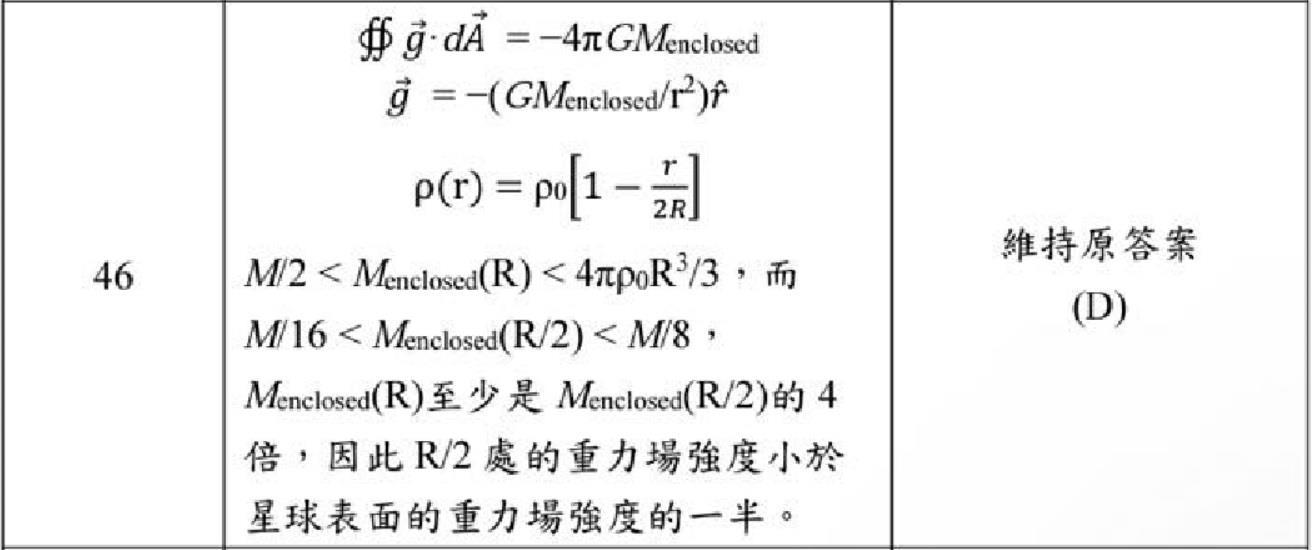
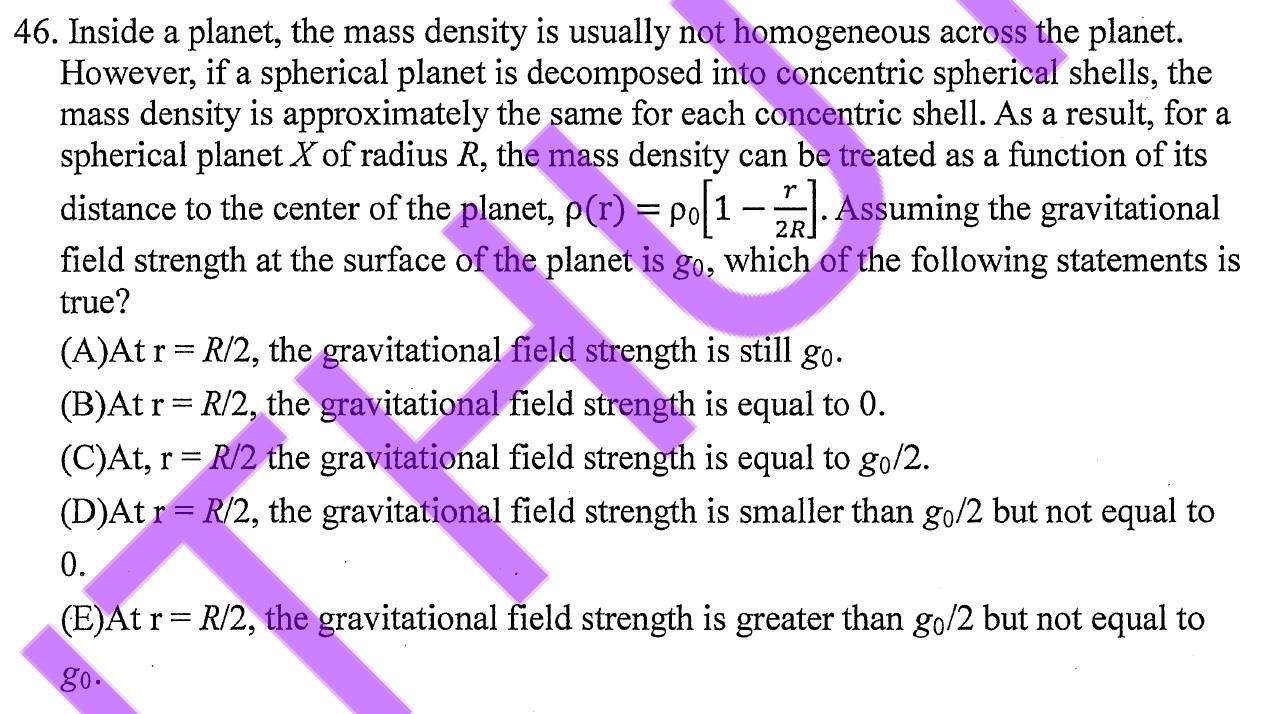
爭議題 – 高斯定理之引力場強 Gauss’s Law of Gravity
清華大學學士後醫學院 112 年度物理試題 46 吳祖諺 筆
The mass density inside a planet is generally not uniform. However, the mass density of the concentric shells of a spherical planet is approximately the same when the planet is decomposed into concentric spherical shells. As a result, for a spherical planet �� of radius ��, the mass density can be treated as a function of its distance to the center of the planet
��(��)=��0 (1 �� 2��)
Assuming the gravitational field strength at the planet’s surface is ����>�� ≝��0. Show that at �� = 1 2 ��, the gravitational field has a magnitude smaller than half that of planet ��'s surface but is not zero, i.e., ����>½�� < 1 2��0 and ����>½�� ≠0
星球的整體內部一般擁有非均勻的質量密度,然而若是將球體分成多重同心球殼的話, 每個同心球殼內的質量密度皆大略相同。假設有顆星球是一個半徑為 �� 、質量為 ���� 的非 均質球體,吾人稱之為星球 �� 。其質量密度為 ��(��)=��0 (1 �� 2��) 且 ��0 為常數,�� 為距離
星球 �� 中心位置之距。試證明當同心球體的半徑變為星球 �� 的半徑之一半時,其重力場 強度會比在星球 �� 表面的重力場強度之一半弱,即 ����>½�� <½��0 且 ����>½�� ≠0。
Analysis:
A planet’s density usually increases as we go inwards from the crust to core Suppose that a planet called �� is a sphere of radius �� and that its density �� is modeled by ��(��)=��0 (1 �� 2��)
Where �� is the distance from the center, ��0 and �� are constants. The approximate volume of a spherical shell of radius �� and thickness ���� is given by ���� =4����2���� so the mass in this shell is given by ���� =��(��)���� =4����0 (1 �� 2��)��2 ���� Therefore, the total mass in general is given by the integral:
For the total mass of the planet �� with radius �� =��, we have:
For the total mass of the inner spherical shell with radius ��
, we have:
The original question is presented as follows:
With a hint provided as follows:
*The above says that ��������(��) is at least 4 times of ��������
and hence